A >200 meV Uphill Thermodynamic Landscape for Radical Transport in Escherichia coli Ribonucleotide Reductase Determined Using Fluorotyrosine-Substituted Enzymes

Abstract

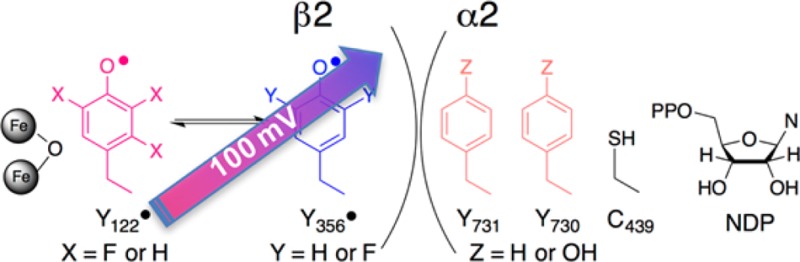
Escherichia coli class Ia ribonucleotide reductase (RNR) converts ribonucleotides to deoxynucleotides. A diferric-tyrosyl radical (Y₁₂₂•) in one subunit (β2) generates a transient thiyl radical in another subunit (α2) via long-range radical transport (RT) through aromatic amino acid residues (Y₁₂₂ ⇆ [W₄₈] ⇆ Y₃₅₆ in β2 to Y₇₃₁ ⇆ Y₇₃₀ ⇆ C₄₃₉ in α2). Equilibration of Y₃₅₆•, Y₇₃₁•, and Y₇₃₀• was recently observed using site specifically incorporated unnatural tyrosine analogs; however, equilibration between Y₁₂₂• and Y₃₅₆• has not been detected. Our recent report of Y₃₅₆• formation in a kinetically and chemically competent fashion in the reaction of β2 containing 2,3,5-trifluorotyrosine at Y₁₂₂ (F₃Y₁₂₂•-β2) with α2, CDP (substrate), and ATP (effector) has now afforded the opportunity to investigate equilibration of F₃Y₁₂₂• and Y₃₅₆•. Incubation of F₃Y₁₂₂•-β2, Y₇₃₁F-α2 (or Y₇₃₀F-α2), CDP, and ATP at different temperatures (2–37 °C) provides ΔE°′(F₃Y₁₂₂•–Y₃₅₆•) of 20 ± 10 mV at 25 °C. The pH dependence of the F₃Y₁₂₂• ⇆ Y₃₅₆• interconversion (pH 6.8–8.0) reveals that the proton from Y₃₅₆ is in rapid exchange with solvent, in contrast to the proton from Y₁₂₂. Insertion of 3,5-difluorotyrosine (F₂Y) at Y₃₅₆ and rapid freeze-quench EPR analysis of its reaction with Y₇₃₁F-α2, CDP, and ATP at pH 8.2 and 25 °C shows F₂Y₃₅₆• generation by the native Y₁₂₂•. F_nY-RNRs (n = 2 and 3) together provide a model for the thermodynamic landscape of the RT pathway in which the reaction between Y₁₂₂ and C₄₃₉ is ∼200 meV uphill.

Introduction

The E. coli class Ia ribonucleotide reductase (RNR) contains two homodimeric subunits, α2 and β2, and functions as an α2β2 complex.^1,2 Its active cofactor is a diferric-tyrosyl radical (Y₁₂₂•) unit buried within β2. This cofactor generates a transient thiyl radical (C₄₃₉•) in α2^3,4 which initiates reduction of the four nucleotides (CDP, GDP, ADP, and UDP) to their corresponding 2′-deoxynucleotides (dNDP), with the specificity of reduction dictated by the appropriate allosteric effector (ATP, TTP, dGTP, and dATP).⁵⁻⁸ During each turnover, Y₁₂₂• reversibly oxidizes C₄₃₉ via multiple proton-coupled electron transfer (PCET) steps through a pathway involving aromatic amino acid residues Y₁₂₂ ⇆ [W₄₈] ⇆ Y₃₅₆ in β2 to Y₇₃₁ ⇆ Y₇₃₀ ⇆ C₄₃₉ in α2. Currently, there is no direct evidence for the involvement of W₄₈ in RT.⁹⁻¹¹ In the wild-type (wt) RNR, only Y₁₂₂• is observed in the presence of substrates (S) and effectors (E); there has been no detectable electron delocalization over the other pathway tyrosines.¹² In this paper, we present the first insight into the thermodynamic landscape of the RT pathway within β2. Site-specific replacement of either Y₁₂₂ or Y₃₅₆ with fluorotyrosines (F_nY, n = 2 and 3) in combination with pathway-blocked α2 mutants (Y₇₃₁F-α2 or Y₇₃₀F-α2)/CDP/ATP and X-band electron paramagnetic resonance (EPR) spectroscopy¹³ provides evidence for equilibration of Y₁₂₂• with Y₃₅₆• as a function of temperature and pH. These studies have allowed estimation of ΔE°′(Y₃₅₆•–Y₁₂₂•) of ∼100 mV.

Detection of low concentrations of any pathway radical in the wt RNR system is challenging due to rate-limiting conformational changes and the substantial overlap in the EPR spectra of the Y•’s.¹⁴ Initial attempts to address if Y₁₂₂• equilibrated with the pathway tyrosines (Y₃₅₆, Y₇₃₁, and Y₇₃₀) utilized the ability to collapse the Y• doublet EPR spectrum into a singlet with β-methylene-deuterated ([β-²H₂]) Y’s.^12,14 β2 containing globally incorporated [β-²H₂]Y’s was reacted with α2 containing protonated Y’s, dCDP, and TTP.¹² These conditions promote α2β2 complex formation¹ but prevent turnover, thus potentially allowing equilibration of the pathway Y•’s. Unfortunately, no unlabeled Y• signal could be detected; the EPR spectrum of Y• in the α2β2 complex was identical to that in free β2.¹²

Recently, we showed that the reaction of NO₂Y₁₂₂•-β2 (3-nitrotyrosine at position 122), which is predicted to be 200 mV more difficult to oxidize than Y at pH 7.0,^15,16 with wt-α2, CDP, and ATP generates a new Y•, localized to Y₃₅₆.¹⁷ Using 3,5-difluorotyrosine (F₂Y) at Y₇₃₁ (or Y₇₃₀) we demonstrated that Y₃₅₆• equilibrated with F₂Y₇₃₁• or F₂Y₇₃₀•.¹⁴ The analysis was facilitated by the unique F₂Y• features arising from ¹⁹F and ¹H-β hyperfine interactions that are observed in both the low- and high-field regions of the EPR spectrum.^11,13 This spectroscopic handle gave us the first opportunity to investigate the effect of the protein environment on the reduction potentials of the pathway Y•’s. Quantitation of Y₃₅₆• in β2 and F₂Y₇₃₁• (or F₂Y₇₃₀•) in α2 by EPR spectroscopy allowed estimation of a ΔE°′(Y_731/730–Y₃₅₆) of ∼100 mV.¹⁴ The thermodynamic landscape of the RT pathway constructed from these studies is shown in Figure 1. We proposed that the overall RT pathway in wt RNR is thermodynamically uphill and driven forward by the nucleotide reduction process, specifically the rapid irreversible cleavage of the C2–OH bond¹⁸ of the substrate and loss of water (10⁶–10⁹ s^–1)¹⁹⁻²¹ in the active site of α2. Equilibration of the pathway Y’s could be measured because oxidation of Y₃₅₆ by NO₂Y₁₂₂• is irreversible. Unfortunately, this same feature prohibited use of NO₂Y₁₂₂•-β2 to monitor equilibration of NO₂Y₁₂₂• and Y₃₅₆•.

Figure 1.

Proposed thermodynamic landscape of the PCET pathway at 25 °C and pH 7.6. The overall reaction is proposed to be thermodynamically uphill and driven forward by the rapid irreversible loss of water from NDP substrate in the active site of α2. No direct evidence is available for the presence of a discrete W₄₈ radical intermediate. Studies performed on NO₂Y₁₂₂•-β2 determined the relative reduction potentials of Y₃₅₆, Y₇₃₁, and Y₇₃₀.

To obtain insight over the entire thermodynamic landscape of RNR, ΔE°′(Y₁₂₂–Y₃₅₆) must be defined. A recently engineered β2 containing 2,3,5-trifluorotyrosine (F₃Y) at position 122 provides an avenue to assess the ΔE°′(Y₁₂₂–Y₃₅₆) energetics.^13,22 The reaction of F₃Y₁₂₂•-β2, α2, CDP, and ATP results in rapid formation of dCDP concomitant with accumulation of Y₃₅₆• (20–30 s^–1). In contrast to NO₂Y₁₂₂•-β2, however, we have demonstrated that Y₃₅₆• can reoxidize F₃Y₁₂₂ and that this reoxidation process is rate-limiting for subsequent turnovers.²² The reversible nature of Y₃₅₆ oxidation in F₃Y₁₂₂•-β2 has led to the studies described herein and provided the opportunity to investigate the relative reduction potentials of F₃Y₁₂₂• and Y₃₅₆•.

In this work, we report the temperature (2–37 °C) and pH-dependent (6.8–8.0) quantitation of F₃Y₁₂₂• and Y₃₅₆• in the reaction of F₃Y₁₂₂•-β2, Y₇₃₁F-α2 (or Y₇₃₀F-α2), CDP, and ATP by EPR spectroscopy. At pH 7.6 and 25 °C, ΔE°′(F₃Y₁₂₂•–Y₃₅₆•) values of 20 ± 10 and 5 ± 7 mV are observed in the reactions with Y₇₃₁F-α2 and Y₇₃₀F-α2, respectively. The ability to equilibrate F₃Y₁₂₂• and Y₃₅₆• with Y₇₃₁F-α2 also provided the opportunity to investigate the fate of the Y₃₅₆ proton upon oxidation of this pathway Y. A plot of the log([Y₃₅₆•]/[F₃Y₁₂₂•]) versus pH provides a slope of 1.2 ± 0.2 at 25 °C, consistent with rapid release of the Y₃₅₆ proton to solvent. With a knowledge of the pH dependence of the F₃Y₁₂₂•/Y₃₅₆• equilibration, we have implemented an experimental design to determine the thermodynamic difference between Y₁₂₂ and Y₃₅₆. Increasing amounts of Y₃₅₆• are observed with increasing pH. Additionally, by choosing an appropriate pH the reduction potential of F₂Y can be tuned to be essentially equal to that of Y,²³⁻²⁵ but oxidized F₂Y• has the potential to be spectroscopically observable because of the ¹⁹F hyperfine features.¹³ Thus, the ability of Y₁₂₂• to oxidize F₂Y incorporated in place of Y₃₅₆ (F₂Y₃₅₆-β2) was tested. Rapid freeze-quench (RFQ)-EPR spectroscopy of the reaction between F₂Y₃₅₆-β2, Y₇₃₁F-α2, CDP, and ATP at pH 8.2 and 25 °C revealed F₂Y₃₅₆• at 3 ± 1% of the total radical concentration. This observation provided a ΔE°′(F₂Y₃₅₆•–Y₁₂₂•) of 70 ± 5 mV, which along with our recent measurement of the reduction potential of F₂Y in a protein environment^23,25 gives an estimate of ΔE°′(Y₃₅₆•–Y₁₂₂•) of ∼100 mV at pH 7.6. The results of the site specifically incorporated unnatural amino acids described herein together with our previous studies allow us to propose a thermodynamic landscape for the RT pathway in the E. coli class Ia RNR that is ∼200 meV uphill between Y₁₂₂ and C₄₃₉.

Materials and Methods

Materials

(His)₆-Y₇₃₁F-α2,²⁶ (His)₆-Y₇₃₀F-α2,²⁶ wt-α2 (specific activity of 2500 nmol/min/mg),²⁶ tyrosine phenol lyase,²⁷ F₂Y,²⁸ and F₃Y²⁸ were isolated; apo F₃Y₁₂₂-β2 was expressed, isolated, and reconstituted²² as previously reported. F₂Y₃₅₆-β2 (0.7 Y•/β2) was available from an earlier study.²⁹ CDP and ATP were purchased from Sigma-Aldrich. Assay buffer consists of 50 mM HEPES pH 7.6, 15 mM MgSO₄, and 1 mM EDTA unless otherwise specified. In all studies, the temperature was controlled using a Lauda RM6 circulating water bath. The reference spectrum for F₃Y₁₂₂• and its simulation were recently reported.³⁰ The reference spectrum for Y₃₅₆•, which was obtained as the signal averaged sum of the Y₃₅₆• difference spectra, is in agreement with the previously reported spectrum.¹⁷

Hand-Quench EPR Analysis of Y₃₅₆• Formation as a Function of Temperature

Assay mixtures containing a final volume of 250 μL with 25 μM Y₇₃₁F-α2, 1 mM CDP, and 3 mM ATP in assay buffer were incubated in a water bath set between 2 and 37 °C. F₃Y₁₂₂•-β2 (0.8 F₃Y•/β2) was added to a final concentration of 25 μM to initiate each of the reactions. The reaction mixtures were then transferred to X-band EPR tubes maintained in the water bath, and the samples were frozen in liquid isopentane (−140 °C) at 20 s (or 1 min) and analyzed by X-band EPR spectroscopy The EPR parameters were as follows: microwave frequency 9.45 GHz; power 30 μW; modulation amplitude 1.50 G; modulation frequency 100 kHz; time constant 40.96 ms; and conversion time 20.48 ms. Three independent sets of experiments were carried out.

Analysis of EPR Data

Two different methods, A and B, were used for quantitation of the two radicals due to the small changes in the EPR spectra associated with the changes in T and pH (section described subsequently), the complexity of the spectra, and the half-sites reactivity of RNR (that is, 50% of the starting F₃Y•/β2 remains unchanged). The data shown in the Results section were analyzed by method A, chosen for visualization purposes. Both methods of analysis provide similar outcomes and are summarized in Tables S1 and S2. The total spin remained unchanged in all the samples throughout the analyses. The ΔE°′(F₃Y₁₂₂•–Y₃₅₆•) was calculated based on the two quantitation methods described below and using

1

where K_eq = [Y₃₅₆•]/[F₃Y₁₂₂•], R is the ideal gas constant, T is the temperature (K), and F is Faraday’s constant.

Method A: Quantitation of F₃Y₁₂₂• and Y₃₅₆• in β2 as a Function of Temperature

Each EPR spectrum was normalized to have the same intensity in the low-field features associated with F₃Y₁₂₂•. In this representation of the spectra, the intensity of F₃Y₁₂₂• remains constant, allowing easier visualization of the Y₃₅₆• signal that grows in with increasing temperature. Using the low-field features in the spectrum of F₃Y₁₂₂•, F₃Y₁₂₂• was subtracted from each composite spectrum. The amount of Y₃₅₆• remaining was determined by double integration.¹³ The Y₃₅₆• spectrum observed for each sample was identical by this method.

Method B

A detailed description of data analysis by method B is presented in the Supporting Information. In the first step, the baseline was removed from each spectrum with a second-order polynomial fit. In the second step, the 50% signal from F₃Y₁₂₂• that remains in the composite spectra due to half sites reactivity was subtracted using the F₃Y₁₂₂•-β2 reference spectrum (Figure S1A). The resulting composite spectra show the interconversion between F₃Y₁₂₂• and Y₃₅₆• as a function of temperature (Figure S1B), free from the complications caused by half sites reactivity. However, this subtraction increases the noise level of the spectra, so the relative amounts of F₃Y₁₂₂• and Y₃₅₆• cannot be determined reliably by eye. Therefore, a script was written in Matlab 2016a to automatically subtract out the remaining F₃Y₁₂₂•. The amount of remaining F₃Y₁₂₂• was determined by adjusting the intensity of the F₃Y₁₂₂•-β2 reference spectrum (Figure S1C) until the least-squares difference between the reference spectrum and the composite spectra in the g-value interval between 2.0363 and 2.0390 (this defines the highest S/N region of the low-field F₃Y₁₂₂• features) was minimized. The amount of Y₃₅₆• after subtracting out the remaining F₃Y₁₂₂• was determined by double integration. The Y₃₅₆• spectrum determined by this method was the same in each sample (Figures S1D and S2).

Temperature-Dependent Equilibration of F₃Y₁₂₂• and Y₃₅₆• within the Same Sample

To support equilibration between F₃Y₁₂₂• and Y₃₅₆• in β2 (at 25 °C) as described above in the Y₇₃₁F- and Y₇₃₀F-α2 reactions, the EPR spectrum of the 20 s sample was first recorded. Each sample was then thawed by submersion into a room-temperature water bath and was then incubated in a 2 °C water bath for 15 s followed by refreezing and reacquisition of the EPR spectra. The samples were thawed again and then placed in a 25 °C water bath for 15 s, refrozen, and the EPR spectrum rerecorded. Quantitation of Y₃₅₆• and F₃Y₁₂₂• was performed as described above.

RFQ-EPR Analysis of Y₃₅₆• Formation as a Function of Temperature

RFQ experiments were performed on an Update Instruments 1019 syringe ram unit and a model 715 syringe ram controller (ram speed 1.25 cm/s). F₃Y₁₂₂•-β2 (70 μM, 0.8 F₃Y•/β2) and CDP (2 mM) in assay buffer in one syringe were mixed with Y₇₃₁F-α2 (70 μM) and ATP (6 mM) in a second syringe and incubated at varying temperatures (2–37 °C) for either 4 or 10 s. The reaction mixture was then sprayed into liquid isopentane,³¹ and the crystals were packed into EPR tubes for analysis by X-band EPR spectroscopy. A packing factor of 0.60 ± 0.02 was calculated for F₃Y₁₂₂•-β2. Data acquisition and analysis were performed as described for the hand-quench (HQ) method.

HQ-EPR Analysis of Y₃₅₆• Formation as a Function of pH

Y₇₃₁F-α2 (25 μM), F₃Y₁₂₂•-β2 (25 μM, 0.6–0.8 F₃Y•/β2), CDP (1 mM), and ATP (3 mM) were combined in 50 mM MES (pH 6.8) or HEPES (pH 7.0–8.0), 15 mM MgSO₄, and 1 mM EDTA and incubated at 5 or 25 °C. Reaction mixtures were transferred to X-band EPR tubes also maintained in the water bath and frozen in liquid isopentane (−140 °C) within 20 s (or 1 min) for analysis by X-band EPR spectroscopy using methods A and B described above. The data were fit to

2

where K = [Y₃₅₆•]/[F₃Y₁₂₂•].

RFQ-EPR Analysis of the Reaction of F₂Y₃₅₆-β2, Y₇₃₁F-α2, CDP, and ATP

Y₇₃₁F-α2 (80 μM) and 6 mM ATP in 50 mM TAPS pH 8.2, 15 mM MgSO₄, and 1 mM EDTA in one syringe was rapidly mixed at 25 °C with an equal volume of F₂Y₃₅₆-β2 (80 μM, 0.7 Y•/β2) and CDP (2 mM) in the same buffer in the second syringe. The reaction was aged for 10, 20, or 40 s, quenched in liquid isopentane, and analyzed by X-band EPR spectroscopy as described above. The EPR parameters were as follows: microwave frequency 9.45 GHz; power 30 μW; modulation amplitude 1.50 G; modulation frequency 100 kHz; time constant 163.8 ms; and conversion time 20.48 ms. The total number of scans were 700 (10 s sample), 600 (20 s sample), and 560 (40 s sample). The simulations were carried out using EasySpin v5.0.18³² in Matlab R2015b. The g-values (2.0073, 2.0044, and 2.0022) and β-¹H hyperfine tensor (54, 52, and 54 MHz) were fixed in the simulations using previously reported values for Y₃₅₆• in the reaction of NO₂Y₁₂₂•-β2 with Y₇₃₁F-α2¹⁴ and the ¹⁹F and β-¹H hyperfine values of F₂Y₁₂₂•.³⁰

Results

Temperature-Dependent Distribution of F₃Y₁₂₂• and Y₃₅₆• in β2 in the Presence of CDP, ATP, and Y₇₃₁F-α2 (or Y₇₃₀F-α2)

We have recently shown that the reaction of F₃Y₁₂₂•-β2, wt-α2, CDP, and ATP generates a kinetically and chemically competent Y₃₅₆• that can reoxidize F₃Y₁₂₂.²² We hypothesized that if we carried out the same experiment with a block in the pathway (Y₇₃₁F-α2 or Y₇₃₀F-α2)¹³ then equilibration of F₃Y₁₂₂• and Y₃₅₆• could be measured by EPR spectroscopy as a function of temperature, allowing determination of ΔE°′(F₃Y₁₂₂•–Y₃₅₆•). F₃Y₁₂₂•-β2, CDP, and ATP were incubated with Y₇₃₁F-α2 at varying temperatures from 2 to 37 °C for 20 s or 1 min. The samples were then frozen in liquid isopentane and examined by X-band EPR spectroscopy. Analysis of the EPR spectra at the chosen times showed no differences between the two time points, suggesting that the reaction mixture had equilibrated. The data from the 20 s incubation time is presented herein. No loss of total spin was observed between the two time points or between the different temperatures.

Interpretation of the EPR data requires consideration of the contributions of each radical and the complexities associated with E. coli RNR. First, Figure 2 shows a 1:1 mixture of F₃Y₁₂₂• (pink) and Y₃₅₆• (blue). The dotted vertical lines assist visualization of the features associated with Y₃₅₆• that minimally overlap with those associated with F₃Y₁₂₂•. Second, reduced amounts of F₃Y₁₂₂• and Y₃₅₆• arise from unique features of the E. coli class Ia RNR. The amount of F₃Y₁₂₂• is typically 0.6–0.8 per β2 (instead of the theoretical 2 F₃Y•/β2), with active β2 containing a F₃Y₁₂₂• in each β monomer.²² Furthermore, while the active form of RNR is α2β2, the enzyme exhibits half-sites reactivity where only one of the two Y₁₂₂•’s (one α/β pair) is active at a time.²² A consequence of these phenomena is the presence of 50% of the total spin as residual F₃Y₁₂₂• in all reaction mixtures. Thus, the data shown in Figures 3, S3, and S4 are presented using method A described in the experimental section to allow the small changes in the amounts of Y₃₅₆• as a function of temperature (2, 5, 8, 10, 12, and 15 °C) to be more clearly observable. With method A, the spectra have been manipulated such that the amount of F₃Y₁₂₂• remains constant, while Y₃₅₆• grows in as a function of temperature. In this analysis method, each spectrum is normalized to have the same intensity in the low-field F₃Y₁₂₂• features. As shown by the dotted line in Figure 3A,B, increasing amounts of Y₃₅₆• can then be observed between 2 and 15 °C. Two additional replications of this experiment are shown in Figure S3. The changes in the spectra directly correlate with increasing amounts of Y₃₅₆• from 17 ± 5% (average of three trials at 2 °C) to 31 ± 2% (average of three trials at 15 °C) of total spin; the quantitation of these data is summarized in Table S1. In contrast with these observations, minimal changes are visualized in the composite EPR spectra recorded between 15 and 37 °C (Figure S4 and Table S1). The average amounts of Y₃₅₆• in the three experiments are shown in Table S1 (31 ± 2% at 15 °C and 33 ± 1% at 37 °C). The percentage Y₃₅₆• of total spin as a function of temperature is shown in Figure 4 (pink dots). A break in the curve is observed at ∼15 °C, and the amount of Y₃₅₆• does not appear to change significantly from 15 to 37 °C.

Figure 2.

X-band EPR spectra of equimolar concentrations of F₃Y₁₂₂• (pink) and Y₃₅₆• (blue). All spectra presented subsequently are additive and contain the same concentration of F₃Y₁₂₂• and increasing amounts of Y₃₅₆•. The dotted lines highlight the regions of the spectrum where the changes that occur upon Y₃₅₆• formation are most apparent.

Figure 3.

Composite EPR spectra of the F₃Y₁₂₂•-β2/Y₇₃₁F-α2/CDP/ATP reaction as a function of temperature (2–15 °C). The composite spectrum at each temperature was acquired on three independently prepared samples. (A and B) Low- and high-field regions of the spectra for trial 1 are shown here. The color code is described in panel A. Trials 2 and 3 are shown in Figure S3. The composite EPR spectra collected between 15 and 37 °C are shown in Figure S4. (C and D) Low- and high-field regions of a simulated spectrum of a reaction mixture containing 50% each of F₃Y₁₂₂• and Y₃₅₆•. The spectrum was generated by adding the individual spectra of F₃Y₁₂₂• and Y₃₅₆• (Figure 2). The dotted lines identify spectral features that are characteristic of Y₃₅₆•.

Figure 4.

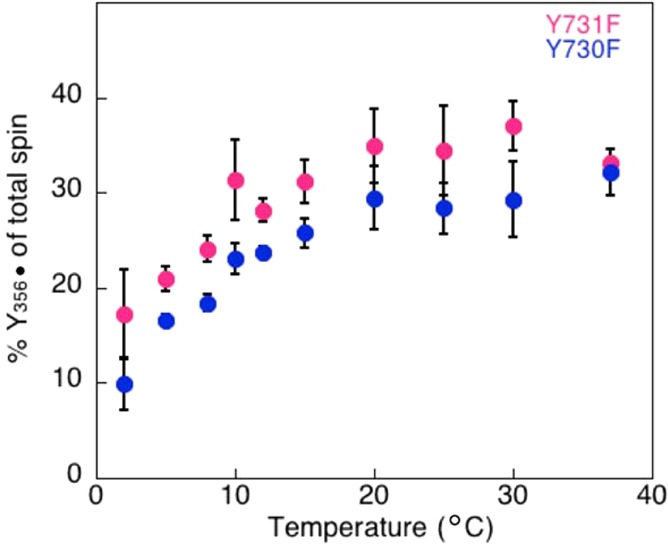
Temperature dependence (2–37 °C) of Y₃₅₆• formation in the reaction of F₃Y₁₂₂•-β2, CDP, ATP, and Y₇₃₁F-α2 (pink) or Y₇₃₀F-α2 (blue). Each data point represents the average of two (blue) or three (pink) independent trials.

Control Experiments to Support F₃Y₁₂₂•/Y₃₅₆• Equilibration

Two types of experiments were carried out to provide further support for the equilibration of F₃Y₁₂₂• and Y₃₅₆•. Previous studies on adenosylcobalamin (AdoCbl) class II RNR³³ have shown that slow quenching of samples by hand shifts the equilibrium relative to rapid freezing methods. Thus, changing ratios of F₃Y₁₂₂• and Y₃₅₆• by RFQ would support equilibration of the two radical states. Preliminary experiments revealed no spin loss and minimal changes in the EPR spectra of samples quenched at 4 and 10 s using the RFQ method. The time scale for quenching was chosen based on kinetic experiments performed with F₃Y₁₂₂•-β2 and wt-α2.²² Thus, subsequent RFQ samples were quenched at 10 s. The results of these experiments are shown in Figure S5 and summarized in Table S1. The amount of Y• observed by RFQ is 5–10% higher than that recorded by the HQ method. However, similar trends are observed between the RFQ-EPR and HQ samples. Increasing amounts of Y₃₅₆• are observed between 2 and 15 °C, whereas the spectra collected between 15 and 37 °C show minimal changes in the percentage of Y₃₅₆• (Table S1 and Figure S6). The RFQ and HQ methods together support equilibration of F₃Y₁₂₂• and Y₃₅₆• and the ability to shift the equilibrium between the two radical states based on the quenching method.

A second experiment to support equilibration between F₃Y₁₂₂• and Y₃₅₆• was carried out as described in the Materials and Methods section. In this experiment, the EPR spectrum of a single sample that was equilibrated at 25 °C was first measured and the sample thawed, equilibrated at 2 °C, and reanalyzed by EPR spectroscopy. The sample was then thawed a final time, shifted back to 25 °C, and the EPR spectrum was recorded. The composite EPR spectra are shown in Figure S7A,B, and the amounts of Y₃₅₆• ascertained from these spectra are summarized in Table S3. The total spin changed minimally and the ratio of the two radicals shifted with temperature as predicted by the trend observed in Figure 4. The data together support equilibration of F₃Y₁₂₂• and Y₃₅₆• with an unusual temperature dependence.

Effect of the F Block at Residue 731 in α2 on the F₃Y₁₂₂•/Y₃₅₆• Equilibrium

Recent high-field (HF)-EPR spectroscopy experiments indicate that the electrostatic environment of Y₃₅₆• changes in a reaction containing Y₇₃₁F-α2 relative to wt-α2.¹⁴ Differences in reactivity between wt-α2 and Y₇₃₁F-α2 are also recorded for photo-RNR, which contains a [Re^I] photooxidant appended to the C-terminal tail of β2 (S₃₅₅C).^34,35 We therefore posited that the block at 731 could perturb the reduction potential of Y₃₅₆• compared to the wt enzyme. The equilibration experiments were repeated with Y₇₃₀F-α2, and as seen in Figure 4 (blue dots), variations can be observed between Y₇₃₁F-α2 and Y₇₃₀F-α2, with the former construct generating slightly higher amounts of Y₃₅₆•.

Calculation of ΔE°′(F₃Y₁₂₂•–Y₃₅₆•) from the Y₇₃₁F and Y₇₃₀F-α2 Studies

To calculate the reduction potential difference between F₃Y₁₂₂• and Y₃₅₆•, the ln K_eq ([Y₃₅₆•]/[F₃Y₁₂₂•]) observed in the Y₇₃₁F and Y₇₃₀F-α2 reactions at 25 °C by the HQ method were used (eq 1); ΔE°′(F₃Y₁₂₂•–Y₃₅₆•) at 25 °C is 20 ± 10 and 5 ± 7 mV, respectively. We note again the unusual temperature dependence of the Y₃₅₆• amounts with a break at 15 °C. A similar temperature dependence has been noted for steady-state dNDP formation in a 1976 study by vön Dobeln and Reichard.³⁶ The cause(s) of the break in Figure 4 and in the previous activity studies are unknown but are likely related to RNR conformational changes that rate-limit RT and nucleotide reduction.

Equilibration of F₃Y₁₂₂• and Y₃₅₆• as a Function of pH and Rapid Proton Exchange with Solvent during Y₃₅₆ Oxidation

The equilibration of F₃Y₁₂₂• and Y₃₅₆• described above gave us the opportunity to investigate the fate of the proton released upon Y₃₅₆ oxidation. Two scenarios for this proton transfer (PT) can be envisioned (Scheme 1). In one case, the proton from Y₃₅₆ is transferred to an amino acid residue (X) and is sequestered from solvent. In the second case, the proton is in rapid exchange directly with solvent; the initial proton acceptor could be an amino acid residue (Y, Scheme 1) or a water cluster. For PT to X, the amount of Y₃₅₆• would be independent of pH, while for PT to Y/solvent log([Y₃₅₆•]/[F₃Y₁₂₂•]) would be directly proportional to the pH with a slope of 1. It has been previously proposed that the conserved E₃₅₀ in β2 functions as the proton acceptor for Y₃₅₆.^10,37 The location of E₃₅₀ within the C-terminal tail at the α2/β2 interface remains unknown, but its importance to catalysis has been demonstrated by site-directed mutagenesis studies.^29,37

Scheme 1. Proposed Models for the Fate of the Y₃₅₆ Proton.

(A) The proton released from Y₃₅₆ is accepted by an amino acid residue (X) and is not solvent-exchangeable. (B) The proton is in fast exchange with solvent. The initial proton acceptor (Y) is either an amino acid residue or water.

To gain insight into the PT pathway at Y₃₅₆, a series of studies were undertaken. F₃Y₁₂₂•-β2, Y₇₃₁F-α2, CDP, and ATP were combined in designated assay buffers (pH 6.8–8.0), incubated for 20 s or 1 min at 25 °C (or 5 °C), quenched by hand, and analyzed by EPR spectroscopy. The analysis was first carried out using method A. As with the temperature dependent studies, no variations in total spin were recorded, and no differences were observed between the spectra of samples incubated for 20 s and 1 min, observations consistent with a reaction at equilibrium. The spectral changes are shown in Figure 5A,B, and the dotted line shows an increase in the amount of Y₃₅₆• as the pH is increased. The composite spectra for a second trial are shown in Figure S8 (see Figure S9 for the 5 °C data), and the average amounts of Y₃₅₆• from the two experiments are reported in Table S2. Figure 6A shows the percentage of Y₃₅₆• for the pH range 6.8–8.0 at 25 °C (see Figure S10A for data from pH 6.8–7.8 for 5 °C). The percentage of Y₃₅₆• at pH 6.8 and 7.0 are very low (Table S2), and the percentage of Y₃₅₆• above pH 8.0 at 25 °C and pH 7.8 at 5 °C does not change. The maximum amounts of Y₃₅₆• at 25 °C (43%) and 5 °C (31%) reflect the equilibrium concentrations of Y₃₅₆• at each temperature.

Figure 5.

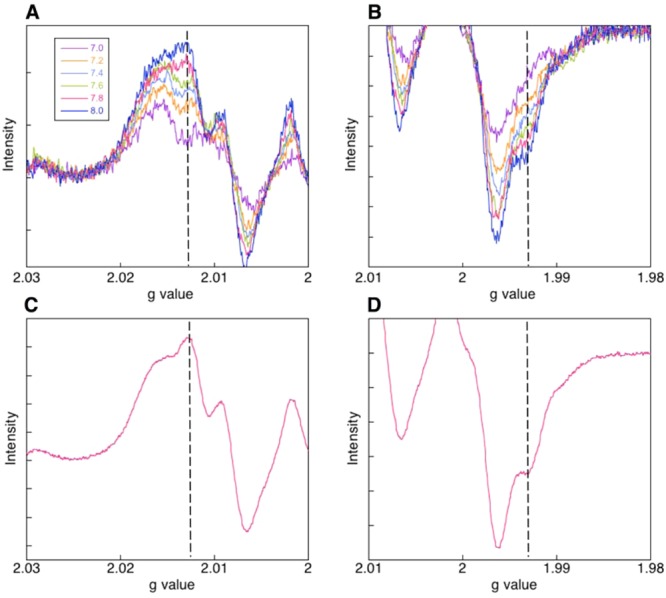
Composite EPR spectra of the F₃Y₁₂₂•-β2/Y₇₃₁F-α2/CDP/ATP reaction at 25 °C as a function of pH. The composite spectrum at each pH was acquired on two independently prepared samples. (A and B) The low- and high-field regions of the spectra for trial 1 are shown here. The colors represent different pH values as described in panel A. Trial 2 is shown in Figure S8. (C and D) Low- and high-field regions of a simulated spectrum of a reaction mixture containing 50% each of F₃Y₁₂₂• and Y₃₅₆•. The spectrum was generated by adding the individual spectra of F₃Y₁₂₂• and Y₃₅₆• (Figure 2). The dotted lines identify spectral features that are characteristic of Y₃₅₆•.

Figure 6.

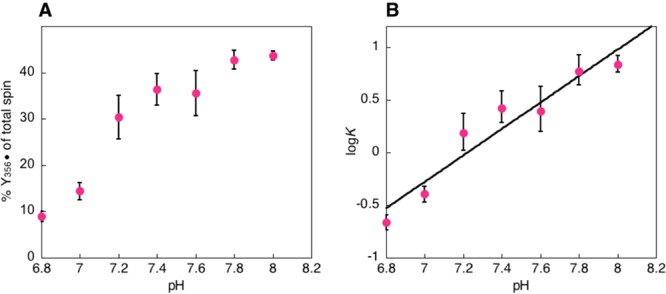
pH dependence of Y₃₅₆• formation in the reaction of F₃Y₁₂₂•-β2/Y₇₃₁F-α2/CDP/ATP at 25 °C. (A) Percentage Y₃₅₆• of total spin as a function of pH. (B) log K as a function of pH where K is the ratio of Y₃₅₆• to F₃Y₁₂₂•. The observed pH dependence of slope 1.2 ± 0.2 supports that the Y₃₅₆• proton is in fast exchange with solvent.

The dependence of log([Y₃₅₆•]/[F₃Y₁₂₂•]) on pH at 25 and 5 °C are shown in Figures 6B and S10B, respectively. A slope of 1.2 ± 0.2 is measured at 25 °C (1.0 ± 0.1 at 5 °C) supporting the model in which the proton from Y₃₅₆ is in fast exchange with solvent at both temperatures. Y₃₅₆• formation is favored more at 25 °C compared to 5 °C, an observation that is in accordance with our temperature-dependent distribution between the two radicals (Figure 4).

Equilibration of Y₁₂₂• and F₂Y₃₅₆• Using F₂Y₃₅₆-β2/α2/CDP/ATP

Although the above studies allowed establishment of ΔE°′(F₃Y₁₂₂•–Y₃₅₆•) in F₃Y₁₂₂•-β2, the ΔE°′(Y₁₂₂•–Y₃₅₆•) in wt RNR, which is essential for understanding the thermodynamics of the RT pathway, remains unknown. The pH studies described above show that maximum Y₃₅₆• is generated with F₃Y₁₂₂•-β2 at pH 8.0 or greater and 25 °C. Recent studies suggest that the difference in reduction potential between Y and F₂Y at position 356 at pH 8.2 is small (<10 mV)²⁵ and that the activity of F₂Y₃₅₆-β2 at this pH is 50% of the wt activity.²⁹ The pK_a of F₂Y₃₅₆ is estimated to be 7.6 at position 356;¹⁵ thus, at pH 8.2, >80% of F₂Y₃₅₆ is in the deprotonated state. Due to the ability to detect small amounts of F₂Y• utilizing its unique spectroscopic features in the low- and high-field regions of the EPR spectrum, we carried out the following experiment in the hope of obtaining insight about ΔE°′(Y₁₂₂•–Y₃₅₆•). F₂Y₃₅₆-β2, Y₇₃₁F-α2, CDP, and ATP were reacted at pH 8.2 for 10, 20, or 40 s, and the reaction was quenched using the RFQ instrument and analyzed by EPR. Quenching on the millisecond time scale was used to avoid potential shifting of the equilibrium observed with hand quenching (Table S1 and Figure S6).³³

The RFQ-EPR data for the reaction at 20 s are shown in Figure 7, and the 10 and 40 s data are shown in Figure S11. A view of the entire spectrum is shown in the inset in Figure 7. The results reveal small features on the low- and high-field sides that suggested the presence of F₂Y₃₅₆•.¹³ The resolved hyperfine splittings were simulated with the “pepper” module of EasySpin as described in the Methods section. From the initial simulations, it was recognized that the β-¹H hyperfine parameters matched the doublet splitting on the high-field side of the spectrum, confirming the identity of this radical species as F₂Y₃₅₆•. The interdoublet splitting was reproduced with two equivalent ¹⁹F couplings having an A_zz of 147 MHz.^13,30 The sharpness of the 3,5-¹⁹F features are similar to those previously reported for the other pathway residues F₂Y₁₂₂,¹³ F₂Y₇₃₁,¹⁴ and F₂Y₇₃₀¹⁴ reflecting a rigid conformation constrained by the protein environment. The A_zz value for F₂Y₃₅₆• is slightly weaker than those reported previously for the other F₂Y•’s (Table S4) and will be of importance when structural insight is obtained.

Figure 7.

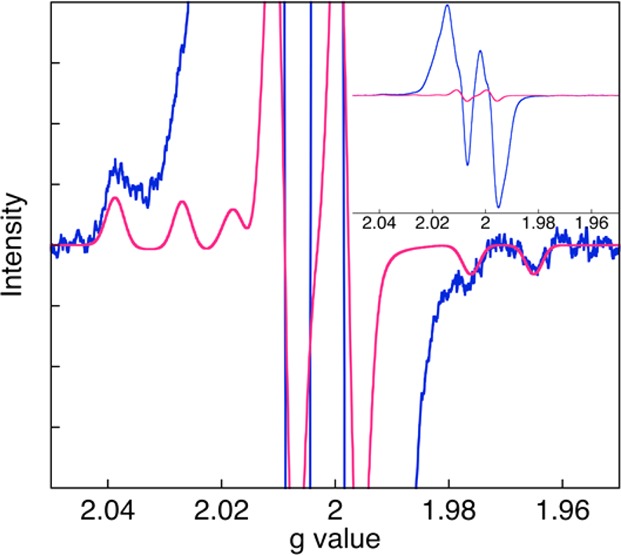
Reaction of F₂Y₃₅₆-β2, Y₇₃₁F-α2, CDP, and ATP monitored by RFQ-EPR spectroscopy. Expanded view of the overlay of the EPR spectrum of the reaction mixture quenched at 20 s (blue) with the simulated spectrum of F₂Y₃₅₆• (pink). The inset shows the full spectrum. The EPR spectra of reaction mixtures quenched at 10 and 40 s are shown in Figure S11.

The amount of F₂Y₃₅₆• was similar at all three time points and was approximated from the simulated spectrum by matching the signal intensities of the wing features in the experimental and simulated spectra and comparing the double integral of the two. The greatest source of error in the analysis comes from the intrinsic line broadening factor (17 ± 4 MHz) used in all simulations.¹⁴ The amount of F₂Y₃₅₆• in the 20 s sample was quantitated as 3 ± 1% of total spin. This amount of radical reflects ΔE°′(F₂Y₃₅₆•–Y₁₂₂•) of 70 ± 5 mV, which in combination with our reduction potential studies^24,25 allows calculation of ΔE°′(Y₃₅₆•–Y₁₂₂•) of ∼100 mV at pH 7.6 (Figure 8).

Figure 8.

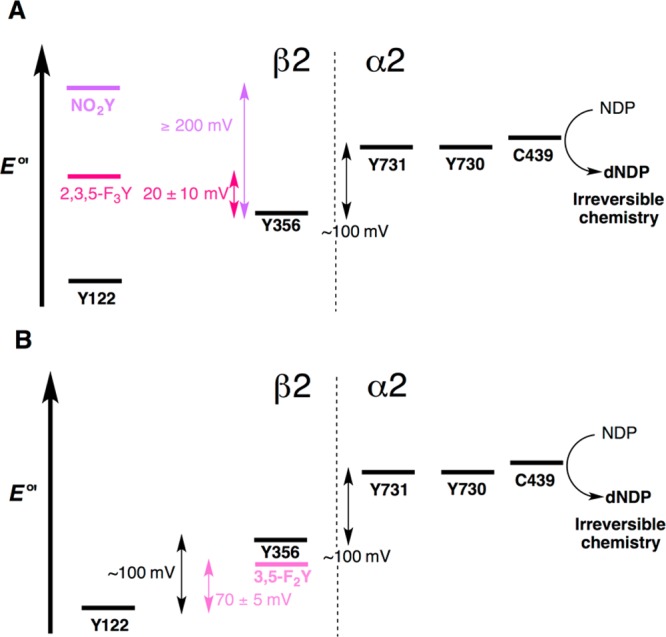
Current thermodynamic landscape of the PCET pathway at 25 °C and pH 7.6. (A) Studies performed on F₃Y₁₂₂•-β2 described in this work provided an estimate of the relative reduction potentials of F₃Y₁₂₂ and Y₃₅₆. (B) Studies performed on F₂Y₃₅₆-β2 provided an estimate of the relative reduction potentials of Y₁₂₂ and Y₃₅₆. W₄₈ has been removed from the landscapes for the sake of clarity.

Discussion

RNRs are divided into three classes based on the metallo-cofactor used for thiyl radical formation.⁶ All classes of RNR initiate nucleotide reduction by thiyl radical mediated 3′-H atom abstraction from the substrate.¹⁸ The reducing equivalents for the reaction are provided by oxidation of a pair of cysteines in the active site,³⁸⁻⁴⁰ with a subtype of the class III enzyme which uses formate as the reductant as the sole exception.⁴¹ The class II RNR utilizes adenosylcobalamin as a cofactor,³ whereas the class III system uses a stable glycyl radical to generate the transient thiyl radical.⁴² These observations raise the issue of why and how a 35 Å oxidation process evolved in the class I RNR⁹ instead of a direct H atom abstraction process that is used by the other classes.¹⁸ The turnover number for deoxynucleotide formation (2–10 s^–1)⁴³ and the large distance between Y₁₂₂• and C₄₃₉ in the class Ia RNR^9,44 require intermediates in the oxidation process and raise the question of how the thermodynamic and kinetic landscape of this process has evolved to maintain balanced dNTP pools and avoid self-inactivation. Investigation of this oxidation process has proven challenging primarily due to the slow rate-limiting conformation changes that occur in the α2β2 complex subsequent to S/E binding and prior to RT.⁴³ Furthermore, the substantial overlap of the EPR spectra of Y•’s would make identification of these species challenging even if the rate-limiting step could be altered.

Thermodynamic Landscape of the RT Pathway within the β2 Subunit

Recently we have assembled the diferric-NO₂Y₁₂₂• cofactor (t_1/2 of 40 s at 25 °C) in the β2 subunit of RNR. NO₂Y• is ∼200 mV more oxidizing than Y•¹⁶ and has provided insight about the thermodynamic landscape for the RT pathway in two ways. When NO₂Y was substituted in place of each Y_x in the pathway (Figure 1, where x = 122 and 356 in β2 and 731 and 730 in α2), the resulting mutants were all catalytically inactive.¹⁵ Thus, perturbation of the reduction potential by +200 mV is sufficient to shut down the RT pathway. This observation supports previous proposals about the extent to which uphill steps can be accommodated in electron transfer (ET) pathways in general,^45,46 and in RNR specifically.^46,47 NO₂Y substitution at each position also allowed assessment of the protein environment perturbation of the pK_a of the phenol, relative to the pK_a in solution. Positions 356, 731, and 730 were found to be minimally perturbed (+0.4, 1.0, and 1.2 units) and position 122 was found to be greatly perturbed (greater than +3 units).¹⁵ We assume that a similar position-dependent perturbation occurs with the F_nY’s incorporated at 356, 731, and 730. However, given the unique environment of Y₁₂₂ (hydrophobic and adjacent to the diferric cluster), this assumption cannot be made.

The ability to generate NO₂Y₁₂₂• in β2 allowed observation of the equilibration of the pathway tyrosyl radicals: Y₃₅₆•, F₂Y₇₃₁•, or F₂Y₇₃₀•. This observation was fortuitous as the equilibration arose from several unanticipated consequences of NO₂Y₁₂₂• substitution. First, this mutant uncoupled the conformational gating masking the wt RT process. DeoxyCDP and Y₃₅₆• formed during reverse RT occurred at 100–300 s^–1,¹⁷ much faster than the wt turnover of 5 s^–1.⁴³ Although Y₃₅₆• was generated rapidly, it was unable to reoxidize the NO₂Y^– phenolate formed during forward RT (Scheme 2). Thus, a block in the pathway occurred without additional mutations. We note that in wt RNR there is evidence to suggest that a proton is delivered to Y₁₂₂• from the water on Fe1 in the cluster during forward RT (Scheme 2).⁴⁸ In the case of NO₂Y, this does not occur, and the phenolate is formed. It is likely that the water on Fe1 remains protonated providing insight into the relative pK_as of Y₁₂₂ and Fe1–H₂O. Since the NO₂Y phenol has a pK_a of 7.1, this raises issues about the protonation state of F₃Y₁₂₂• (pK_a of phenol is 6.4) on reduction during forward RT (Scheme 2).

Scheme 2. First PCET Step in the RT Pathway of E. coli Class Ia RNR.

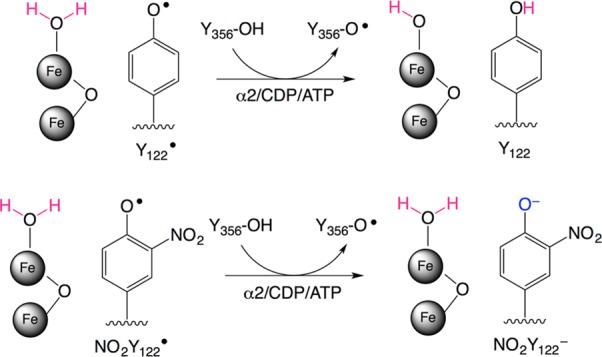
(A) In the wt-β2/α2/CDP/ATP complex, PT from Fe1–H₂O to Y₁₂₂• occurs concomitant with ET from Y₃₅₆ to Y₁₂₂•. (B) In the NO₂Y₁₂₂•-β2/α2/CDP/ATP complex, ET from Y₃₅₆ to Y₁₂₂• generates the NO₂Y^– phenolate. RT initiation in F₃Y₁₂₂•-β2 is proposed to generate the F₃Y₁₂₂^– phenolate (Scheme 1).

Due to the inability to investigate equilibration of Y₃₅₆• with Y₇₃₁• and Y₇₃₀• in wt RNR, F₂Y was inserted in place of either Y₇₃₁ or Y₇₃₀, providing access to the unique EPR spectroscopic features of F₂Y•.¹⁴ These experiments showed the presence of 10–15% F₂Y₇₃₁• (or F₂Y₇₃₀•). A knowledge of the pK_a perturbation of ∼1 unit at these positions¹⁵ in conjunction with differential pulse voltammetry (DPV) studies on the N-acetyl-3,5-difluoro-l-tyrosinamide²⁴ provided an estimate of 85–95 mV for the reduction potential difference between Y₇₃₁• (or Y₇₃₀•) and Y₃₅₆•. This calculation agreed with the results from a second experiment where NO₂Y₁₂₂•-β2 was reacted with [β-²H₂]Y-α2 and probed for variations in the EPR spectrum. Temperature dependent studies provided the ΔE°′([β-²H₂]Y•–Y₃₅₆•) of ∼100 mV (Figures 1 and 8). These studies together showed that the RNR protein environment perturbs F₂Y and Y in a similar fashion and that F₂Y is a good probe for the reduction potential of both Y₇₃₁ and Y₇₃₀.

More recently, we have reported the detailed kinetic analysis of the F₃Y₁₂₂•-β2/α2/CDP/ATP reaction.²² This reaction generates a kinetically and chemically competent Y₃₅₆• at 20–30 s^–1, which in contrast to Y₃₅₆• generated by NO₂Y₁₂₂•-β2 is capable of reoxidizing F₃Y₁₂₂. The reoxidation process is conformationally gated and rate-limiting for subsequent dCDP formation and only observed after several turnovers upon exhaustion of the reducing equivalents. The observation of both radicals (F₃Y₁₂₂• and Y₃₅₆•) and activity required that we utilize a pathway block in order to monitor equilibration. Y₇₃₁F-α2 (Y₇₃₀F-α2) served that purpose as our previous studies showed that these mutants still allow Y₃₅₆• generation.¹³

To quantitate the reduction potential increase that occurs upon replacement of Y₁₂₂ with F₃Y₁₂₂, it is important to determine whether the latter is reduced to the phenol or phenolate (F₃Y₁₂₂ vs F₃Y₁₂₂^–) during RT (Scheme 2). We favor the model where F₃Y₁₂₂^– is generated upon RT. In support of this proposal is the observation of NO₂Y₁₂₂^– in the NO₂Y₁₂₂•-β2 experiments.¹⁷ The solution pK_a of NO₂Y is 7.1,¹⁶ and the visualization of NO₂Y₁₂₂^– can be rationalized if Fe1–H₂O has a pK_a between 8.0 and 10.0. Although ferric iron typically reduces the pK_a of bound water,⁴⁹ di-iron clusters have been known to shift this value into the physiological pH range (pH > 7.0)⁵⁰ in a protein-environment-dependent manner. The diferric cluster environment in the class Ia RNR is unique and as noted above perturbs the pK_a of Y₁₂₂ by >3 units.¹⁵ If the pK_a of Fe1–H₂O is perturbed to >8.0, then initiation of the reaction with F₃Y₁₂₂• would primarily result in the generation of F₃Y₁₂₂^–. The protonation state of F₃Y₁₂₂, while favored to be deprotonated, is unknown and is under investigation.

The potential difference of ∼20 mV calculated between F₃Y₁₂₂• and Y₃₅₆• (Figure 4) makes generation of F₃Y₁₂₂^– an appealing model. We predict that ΔE°′(NO₂Y₁₂₂•/NO₂Y₁₂₂^––Y₃₅₆•/Y₃₅₆) is ≥200 mV, owing to the inability of Y₃₅₆• to reoxidize NO₂Y^–. With these two values, we can estimate ΔE°′(NO₂Y₁₂₂•/NO₂Y₁₂₂^–– F₃Y₁₂₂•/F₃Y₁₂₂^–) as greater than or equal to ∼184 mV. This calculation agrees with the predicted potential difference between these two analogs based on the solution DPV data collected on the protected amino acids (∼180 mV).²⁴ Unfortunately, we cannot at present directly extrapolate the potential difference calculated between NO₂Y₁₂₂•/NO₂Y₁₂₂^– (or F₃Y₁₂₂•/F₃Y₁₂₂^–) and Y₃₅₆•/Y₃₅₆ to Y₁₂₂•/Y₁₂₂. This is primarily due to the unique nature of residue 122’s environment compared to that of the other pathway Y’s. The Y₁₂₂ site is not in equilibrium with solvent⁴⁸ over the time course of our experiments (<20 s); its reduction potential is pH-independent and is directly determined by the dielectric constant of the protein environment. Due to these reasons, we turned our attention to an alternate way to monitor equilibration of Y₁₂₂• and Y₃₅₆• where the native Y₁₂₂• remains intact but Y₃₅₆ is replaced with F₂Y₃₅₆.

Our observations with NO₂Y₁₂₂•-β2¹⁴ and the pH-dependent studies reported herein suggest that ΔE°′(Y₁₂₂•/Y₁₂₂–Y₃₅₆•/Y₃₅₆) can be easily extrapolated from ΔE°′(Y₁₂₂•/Y₁₂₂–F₂Y₃₅₆•/F₂Y₃₅₆^–). The proton from F₂Y₃₅₆ is in rapid exchange with solvent (Figures 6B and S10B), and at an appropriate pH, we predict that its reduction potential is a good approximation of Y₃₅₆. The reaction of F₂Y₃₅₆-β2/Y₇₃₁F-α2/CDP/ATP was carried out at pH 8.2 to maximize the chances of observing the F₂Y₃₅₆• signal and revealed similar amounts of F₂Y₃₅₆• at 10, 20, and 40 s, supporting equilibration. The observed percentage of F₂Y₃₅₆• (3%) provides an estimation of ΔE°′(F₂Y₃₅₆•/F₂Y₃₅₆^––Y₁₂₂•/Y₁₂₂) of ∼70 mV. At pH 8.2, the reduction potentials of the F₂Y₃₅₆•/F₂Y₃₅₆^– and Y₃₅₆•/Y₃₅₆ couples are predicted to be roughly the same.^24,25 At pH 7.6, the standard assay conditions, the reduction potential of Y₃₅₆ is expected to increase by ∼30 mV,^24,25,51 providing a ΔE°′(Y₃₅₆•–Y₁₂₂•) of ∼100 mV (Figure 8B). Finally, we note that our data taken together propose that at 25 °C and pH 7.6 F₃Y₁₂₂ is ∼120 mV more oxidizing than Y₁₂₂ within the RNR protein environment. This difference is 10 times greater than we had originally predicted based on the solution DPV data collected on the N-acetyl-fluoro-l-tyrosinamide derivatives.¹¹ We note that this original prediction assumed that both F₃Y• and Y• are reduced to the corresponding phenols during turnover.

Relationship between the Thermodynamic Landscape and Kinetics

It is important to note that the equilibration studies described in this work were performed under nonturnover conditions (with Y₇₃₁F-α2 or Y₇₃₀F-α2). Thus, a key issue to address is whether the protein environment can alter the thermodynamic landscape to lower ΔE°′(Y₃₅₆•–Y₁₂₂•) and facilitate turnover. Although this is a likely possibility, we argue that oxidation of Y₃₅₆ by Y₁₂₂• must be uphill even under turnover conditions. Evidence for this conclusion is provided by our combined studies with wt RNR,⁴³ F₃Y₁₂₂•-β2,²² and NO₂Y₁₂₂•-β2.¹⁷

In the case of wt RNR, investigation of RT has been hindered by the inability to monitor Y₁₂₂• disappearance and reappearance during turnover.⁴³ To account for this observation, we have previously modeled that the reverse RT process in wt RNR in which Y₃₅₆• reoxidizes Y₁₂₂ must be downhill and rapid (10³ s^–1).⁴³ In the case of F₃Y₁₂₂•-β2, we have measured formation of Y₃₅₆• (20–30 s^–1) and demonstrated that reoxidation of F₃Y₁₂₂ by Y₃₅₆• is slow (0.4–1.7 s^–1) and rate-limiting for multiple turnovers.²² In the NO₂Y₁₂₂•-β2 system, Y₃₅₆• accumulates (100–300 s^–1) due to the inability of this pathway radical to reoxidize NO₂Y^– subsequent to the first turnover.¹⁷ Taken together, these studies suggest that Y₃₅₆• can be observed during turnover only when reverse RT is slowed down (F₃Y₁₂₂•-β2) or completely inhibited (NO₂Y₁₂₂•-β2) and is partly a result of the potential difference between Y₁₂₂ and Y₃₅₆. DPV studies have estimated that reduction potential increases in the order Y < F₃Y < NO₂Y.^16,24 In accordance with this prediction, the rate constant for forward RT that generates Y₃₅₆• increases with increasing driving force, whereas the rate constant for reverse RT decreases with driving force, reinforcing our model that oxidation of Y₃₅₆ by the native Y₁₂₂• is uphill. We have previously proposed that the conformational change that triggers RT targets the initial PT step from Fe1–H₂O to Y₁₂₂• (Scheme 2).⁴⁸ Uncoupled PT and ET in NO₂Y₁₂₂•-β2, and potentially F₃Y₁₂₂•-β2, suggest that we may have overcome this conformational gating and obtained direct insight into the thermodynamic effect of replacing Y₁₂₂ with these unnatural analogs. Further support for this model is obtained when the forward RT rate constants in NO₂Y₁₂₂•-β2 and F₃Y₁₂₂•-β2 are predicted using the Moser–Dutton equation⁵² (eq 3) for dependence of k_ET on distance (R) and driving force (ΔG).

3

Assuming identical distances and reorganizational energies (λ) for ET in NO₂Y₁₂₂•-β2 and F₃Y₁₂₂•-β2, the individual expressions for log k_ET can be combined to assess the effect of the driving force differences (ΔG, 200 mV vs 20 mV, Figure 8A) on k_ET. The net equation requires an estimation of λ; by varying the reorganizational energy from 0.7 to 1.4 eV,⁴⁵k_ET in NO₂Y₁₂₂•-β2 was calculated to be 9- to 11-fold faster than k_ET in F₃Y₁₂₂•-β2. This approximation is similar to our experimental data (5- to 15-fold) supporting the idea that the driving force dictates the kinetics in these mutant RNRs and further that both NO₂Y₁₂₂• and F₃Y₁₂₂• are reduced to the corresponding phenolates during RT.

Based on our static thermodynamic picture constructed from the studies with NO₂Y₁₂₂•-β2 and those reported herein, we propose that the landscape from Y₁₂₂ to Y₇₃₀ is ∼200 meV uphill (at 25 °C and pH 7.6, Figure 8B). The landscape between Y₇₃₀ and 3′ hydrogen atom abstraction from the nucleotide must further be taken into account to make deoxynucleotides. Electrochemical measurements on the cysteine within glutathione and Y have revealed similar midpoint potentials at pH 7.0,⁵³ providing an estimation of ∼0.04% C₄₃₉• formation in the α2β2 complex. Given the predicted rate constant for H₂O loss from the 2′ position (10⁶–10⁸ s^–1)¹⁹⁻²¹ of the nucleotide, the rate of this reaction using 0.04% C₄₃₉• would be ∼10²- to 10⁴-fold faster than conformationally gated nucleotide reduction (2–10 s^–1).⁴³

The above calculation assumes that the reaction landscape is isoenergetic subsequent to generation of Y₇₃₁•. However, DFT calculations performed on the individual crystal structure of α2 and on model systems have provided an estimate of ∼120 mV for ΔE°′(C₄₃₉•–Y₇₃₀•)^54,55 and ∼90–260 mV for 3′ H atom abstraction by C₄₃₉•.^6,56−58 If the measured ΔE°′(Y₇₃₀•–Y₁₂₂•) of 200 mV is reflective of the thermodynamic landscape under turnover conditions, then we estimate that the combined steps of C₄₃₉ oxidation and 3′ H atom abstraction must be <200 meV uphill to maintain a turnover number of >10 s^–1.

The DFT calculations were based on a structure of α2 alone with poor electron density for the substrate and in the absence of allosteric effector. It is likely that the RT pathway and the active site in α2 will be conformationally altered in the active α2/β2/S/E complex. Furthermore, uphill reactions can be partially compensated for by decreasing the ET distance between donor and acceptor^45,46 and in the case of PCET reactions by controlling the positioning of the proton acceptor. The distances between Y₁₂₂, Y₃₅₆, and Y₇₃₁ remain unknown because of the disordered C-terminal tail of β2. Thus, structures of the α2β2 subunit interface and knowledge of how these structures are altered in the presence of S and E binding to α2 are crucial to understanding the overall landscape of the reaction and the tuning of the individual steps in the RT process. Nonetheless, we believe from the studies described herein, that the overall reaction from Y₁₂₂• reduction to 3′-hydrogen atom abstraction of NDP is uphill and driven forward by rapid and irreversible loss of H₂O from the NDP (Figure 8).¹⁹⁻²¹

PCET across the β/α Interface Involves Fast Proton Exchange between Y₃₅₆ and Solvent

The equilibrium between F₃Y₁₂₂• and Y₃₅₆• as a function of pH has further provided important insight about the fate of the Y₃₅₆ proton upon its oxidation. It was originally proposed that a specific sequestered amino acid residue within β2 functioned as the proton acceptor.¹⁰ However, the slope of 1 associated with a plot of log([Y₃₅₆•][F₃Y₁₂₂•]) versus pH (Figure 6B) is consistent with the rapid exchange of the Y₃₅₆ proton with solvent at the subunit interface either through an amino acid residue or a water cluster functioning as the initial proton acceptor (Scheme 1). Three distinct types of experiments are currently the basis for favoring the latter possibility.^{29,35,37,59,60}

The most compelling support for this model has been the work of Bennati and co-workers using multifrequency EPR and [²H]-electron–nuclear double resonance (ENDOR) spectroscopic methods on mutant RNRs containing the radical trap, 3-aminotyrosine (NH₂Y). This unnatural amino acid has site specifically replaced Y₃₅₆, Y₇₃₁, or Y₇₃₀, leading to accumulation of NH₂Y• in each case upon incubation with the second subunit, S, and E.^26,61 HF-EPR studies on NH₂Y•’s,^55,59 specifically the g_x component of their g tensors, revealed that the electrostatic environment of all three NH₂Y•’s are perturbed, but that of NH₂Y₃₅₆• is perturbed to a greater extent than either NH₂Y₇₃₁• or NH₂Y₇₃₀•. In contrast with NH₂Y₇₃₁• or NH₂Y₇₃₀•, no moderate hydrogen bonding interactions were observed with NH₂Y₃₅₆• by HF-[²H] ENDOR spectroscopy.⁵⁹ The studies together led to the proposed importance of water clusters in proton removal at the subunit interface.⁵⁹

Using a very different approach, recent studies have been carried out with photo-RNRs in which a photooxidant is attached site specifically to residue 355 in β2 and F_nY (n = 2 or 3) or W replaces Y₃₅₆. In the presence of α2, S, and E and with light initiation, these constructs exert significant control in facilitating PT during oxidation of residue 356, shuttling reactive intermediates between the subunits and in the case of W, rapid PT out of the α/β interface.^35,60

Finally, prior to the studies reported herein, the conserved residue E₃₅₀ located on the flexible C-terminal tail of β2 near Y₃₅₆ in sequence space, was considered to be the most likely amino acid candidate that could function as a proton acceptor for Y₃₅₆. Mutation of E₃₅₀ to A abolished RNR activity,³⁷ an observation we have confirmed.^11,29 However, using our ability to incorporate F_nY analogs in place of RNR pathway residues, we have shown that E₃₅₀ is likely not the proton acceptor for Y₃₅₆, but that its essentiality stems from its involvement in subunit interaction and in the protein conformational gate for RT initiation.²⁹ The experiments presented herein, the E₃₅₀ studies,²⁹ the EPR and ENDOR results,⁵⁹ and the photo-RNR experiments^35,60 together support fast proton exchange between Y₃₅₆ and solvent via water during PCET across the interface.

Summary

Using site specifically incorporated F₃Y and F₂Y in place of β2 residues 122 and 356, respectively, and taking advantage of the unique EPR features of F_nY• relative to Y•, we have measured the thermodynamic landscape within β2 in the α2β2 complex. These results, when combined with similar types of experiments examining the relative reduction potentials of Y₃₅₆, Y₇₃₁, and Y₇₃₀, provide us with the overall thermodynamic landscape that is uphill by >200 meV and is unprecedented in biology. Why would such a design evolve when other classes of RNRs avoid long-range RT by direct hydrogen atom abstraction from the cysteine by their active cofactors? We propose that the enzyme exerts significant kinetic control over radical initiation. RT in class I RNRs plays a very important role in the fidelity of DNA replication and repair by regulating the relative ratios of the dNDP (and hence dNTP) pools and the absolute amounts of these species. This process is largely controlled by binding the appropriate S/E pairs in α2, 40–50 Å removed from the site of RT initiation by the diferric-Y• cofactor.⁴⁸ Subtle changes that occur on S/E binding are thus likely to modulate the reduction potential of residues within the wt RT pathway. All of the experiments conducted to determine the thermodynamic landscape summarized in Figure 8 have been performed with different types of pathway blocks, which are likely to have subtle conformational effects on radical initiation. The proposed uphill nature of the pathway would prevent accumulation of reactive pathway radical intermediates and minimize self-inactivation during the radical initiation process. The connection between our current unprecedented and unexpected thermodynamic measurements and conformational gating of RNR activity by S/E binding is the major focus of our efforts.

Supporting Information Available

The Supporting Information is available free of charge on the ACS Publications website at DOI: 10.1021/jacs.6b08200.

• Temperature dependence of Y₃₅₆• formation; temperature-dependent equilibration of F₃Y₁₂₂• and Y₃₅₆• in the reaction of F₃Y₁₂₂•-β2, CDP, ATP, and Y₇₃₁F-α2 or Y₇₃₀F-α2; hyperfine values for β-¹H and ¹⁹F of F₂Y• at different positions on pathway; analysis by method B for one trial of the F₃Y₁₂₂•-β2/Y₇₃₁F-α2/CDP/ATP reaction as a function of temperature and one trial of the F₃Y₁₂₂•-β2/Y₇₃₁F-α2/CDP/ATP reaction as a function of pH; composite EPR spectra of the F₃Y₁₂₂•-β2/Y₇₃₁F-α2/CDP/ATP reaction as a function of temperature (2–15 °C) and the F₃Y₁₂₂•-β2/Y₇₃₁F-α2/CDP/ATP reaction as a function of temperature (15–37 °C); temperature dependence of Y₃₅₆• formation monitored by RFQ-EPR spectroscopy and in the reaction of F₃Y₁₂₂•-β2/Y₇₃₁F-α2/CDP/ATP as determined by HQ- and RFQ-EPR spectroscopies; temperature-dependent equilibration of F₃Y₁₂₂• and Y₃₅₆• in the reaction of F₃Y₁₂₂•-β2, CDP, ATP, and Y₇₃₁F-α2 or Y₇₃₀F-α2; composite EPR spectra of the F₃Y₁₂₂•-β2/Y₇₃₁F-α2/CDP/ATP reaction at 25 °C and the F₃Y₁₂₂•-β2/Y₇₃₁F-α2/CDP/ATP reaction at 5 °C as a function of pH; pH dependence of Y₃₅₆• formation in the reaction of F₃Y₁₂₂•-β2/Y₇₃₁F-α2/CDP/ATP at 5 °C; reaction of F₂Y₃₅₆-β2, Y₇₃₁F-α2, CDP, and ATP monitored by RFQ-EPR spectroscopy (PDF)

Author Present Address

K.R.R.: Moderna Therapeutics, 200 Technology Square, Cambridge, MA 02139, United States.

Author Present Address

Y.W.: Metabolic Engineering Research Laboratory, 31 Biopolis Way, Nanos #01–01, Singapore 138669.

The authors declare no competing financial interest.