Running Technique is an Important Component of Running Economy and Performance

ABSTRACT

Despite an intuitive relationship between technique and both running economy (RE) and performance, and the diverse techniques used by runners to achieve forward locomotion, the objective importance of overall technique and the key components therein remain to be elucidated.

Purpose

This study aimed to determine the relationship between individual and combined kinematic measures of technique with both RE and performance.

Methods

Ninety-seven endurance runners (47 females) of diverse competitive standards performed a discontinuous protocol of incremental treadmill running (4-min stages, 1-km·h⁻¹ increments). Measurements included three-dimensional full-body kinematics, respiratory gases to determine energy cost, and velocity of lactate turn point. Five categories of kinematic measures (vertical oscillation, braking, posture, stride parameters, and lower limb angles) and locomotory energy cost (LEc) were averaged across 10–12 km·h⁻¹ (the highest common velocity < velocity of lactate turn point). Performance was measured as season's best (SB) time converted to a sex-specific z-score.

Results

Numerous kinematic variables were correlated with RE and performance (LEc, 19 variables; SB time, 11 variables). Regression analysis found three variables (pelvis vertical oscillation during ground contact normalized to height, minimum knee joint angle during ground contact, and minimum horizontal pelvis velocity) explained 39% of LEc variability. In addition, four variables (minimum horizontal pelvis velocity, shank touchdown angle, duty factor, and trunk forward lean) combined to explain 31% of the variability in performance (SB time).

Conclusions

This study provides novel and robust evidence that technique explains a substantial proportion of the variance in RE and performance. We recommend that runners and coaches are attentive to specific aspects of stride parameters and lower limb angles in part to optimize pelvis movement, and ultimately enhance performance.

Running is a fundamental form of human locomotion, a very popular physical activity and the most ubiquitous movement pattern in sports. Distance running performance depends on sustained, predominantly aerobic, energy production and the conversion of this energy into forward movement, called running economy (RE). As running is a relatively unconstrained movement with numerous degrees of freedom, individual runners achieve forward locomotion using diverse “techniques,” with evidence for large inter-individual variation in stride patterns (11,34) and lower limb kinematics (1). Despite this variability and an intuitive relationship between running technique and both performance and economy (3), very little robust objective information exists regarding the influence running technique has on performance and/or economy.

Although there has been extensive research into running biomechanics for more than a century (for an historical review, see Cavanagh [10]), whether running kinematics significantly influence or are related to performance remains unknown. To the authors' knowledge, the only study that has directly examined the relationship between running kinematics and performance found no significant associations in 16 moderate to high-level runners (46). A few small studies have compared groups of sub-elite runners with subelite athletes, finding no (12) or subtle (27) differences in kinematic variables.

RE, typically defined as the energy/oxygen cost of running a given distance at submaximal velocity (42), is known to be an important determinant of distance running performance (14,21) and also to vary widely between runners (>30% [31]). Investigations into the relationship between RE and technique have typically been small cohort studies (n < 25 [45,47]) of relatively homogeneous groups of runners (37,38,45), limited kinematic measurements and methods (e.g., stride parameters [16,37,38]), or measurements of RE and kinematics at different speeds or under different conditions (34,47). Consequently, the overall relationship between specific kinematic variables and RE and performance remains opaque, such that a recent review of strategies to improve RE provided no recommendations with regard to running technique (5).

Despite the lack of consensus in the literature, we postulated that five aspects of kinematics may influence RE and performance: stride parameters, lower limb angles, vertical displacement of the body (vertical oscillation), changes in horizontal velocity during ground contact (braking), and trunk and pelvis orientation (posture). With regard to stride parameters, shorter ground contact time (GCT) has been associated with better RE (34,38) and poorer RE (16). Similarly, the relationships between RE and stride rate (SR) and its reciprocal stride length are equally unclear (r > 0.6 [45] vs NS [38]), whereas other temporal factors, including swing time (SWT [38]) and duty factor (DF), have received little or no attention. The configuration of the lower limb at touchdown might also be expected to be a critical aspect of running technique (8). This includes the relatively simplistic classification of footstrike type (16,37), which can be more precisely measured as foot angle at touchdown, as well as the angles of the shank and thigh. Since RE has been suggested to relate to the stiffness of the stance leg (15), and motion of the swinging leg may contribute ~20% to the cost of locomotion (30), the minimum knee joint angles during stance and swing phases might also be key variables.

As a substantial proportion of work during running is involved in opposing gravity, it has been widely postulated that the amplitude of vertical oscillation of the center of mass (CM) (3,39) and/or surrogate body segments (e.g., pelvis or head [47]) may be related to RE and performance. A smaller CM vertical oscillation has been reported to be associated with better RE (20,45), but other authors have not found any relationship (46). In addition, changes in horizontal velocity of the CM reflect braking/deceleration upon landing that necessitates energetically expensive reacceleration to maintain a constant velocity (20). Paradoxically, the only evidence to date reported a greater change in horizontal velocity of the CM to be related to better RE (47). Finally, some studies have hypothesized that posture could influence economy and performance, such as forward lean of the trunk and transverse plane rotation of the pelvis (46), but with no convincing evidence to date.

Williams and Cavanagh (46) conducted the most thorough kinematic study of RE to date, dividing 31 runners into low, medium, and high RE groups and reporting differences between at least two of the groups for 4 of ~50 kinematic variables. Kinematic measures were derived from 100 Hz cine film recordings of a single stride of overground running and related to RE measurements made during treadmill running on a different day. With contemporary 3-D automatic motion capture methods, more thorough measurements (higher frequency, more rigorous marker sets, multiple strides, and larger sample size) of specific hypothesized kinematic variables are possible at a range of running speeds simultaneous to RE measurements. In addition, RE can be most accurately assessed as the locomotory energy cost (LEc) of running as this is not confounded by individual differences in substrate metabolism (18,42). These methodological improvements may further illuminate the relationships of kinematics with RE and, importantly, also kinematics and performance.

The aim of this study was to determine the relationships between specific kinematic measures of running technique and both RE and performance. Given that RE and performance are known to be influenced by a range of anthropometric (44), physiological (41), and intrinsic musculoskeletal variables (4), the contribution of technique would seem to be relatively modest. In this case, a statistically robust investigation dictated the recruitment of a large heterogeneous cohort, including recreational and elite runners, to provide a range of running techniques, economy, and performance.

METHODS

Participants

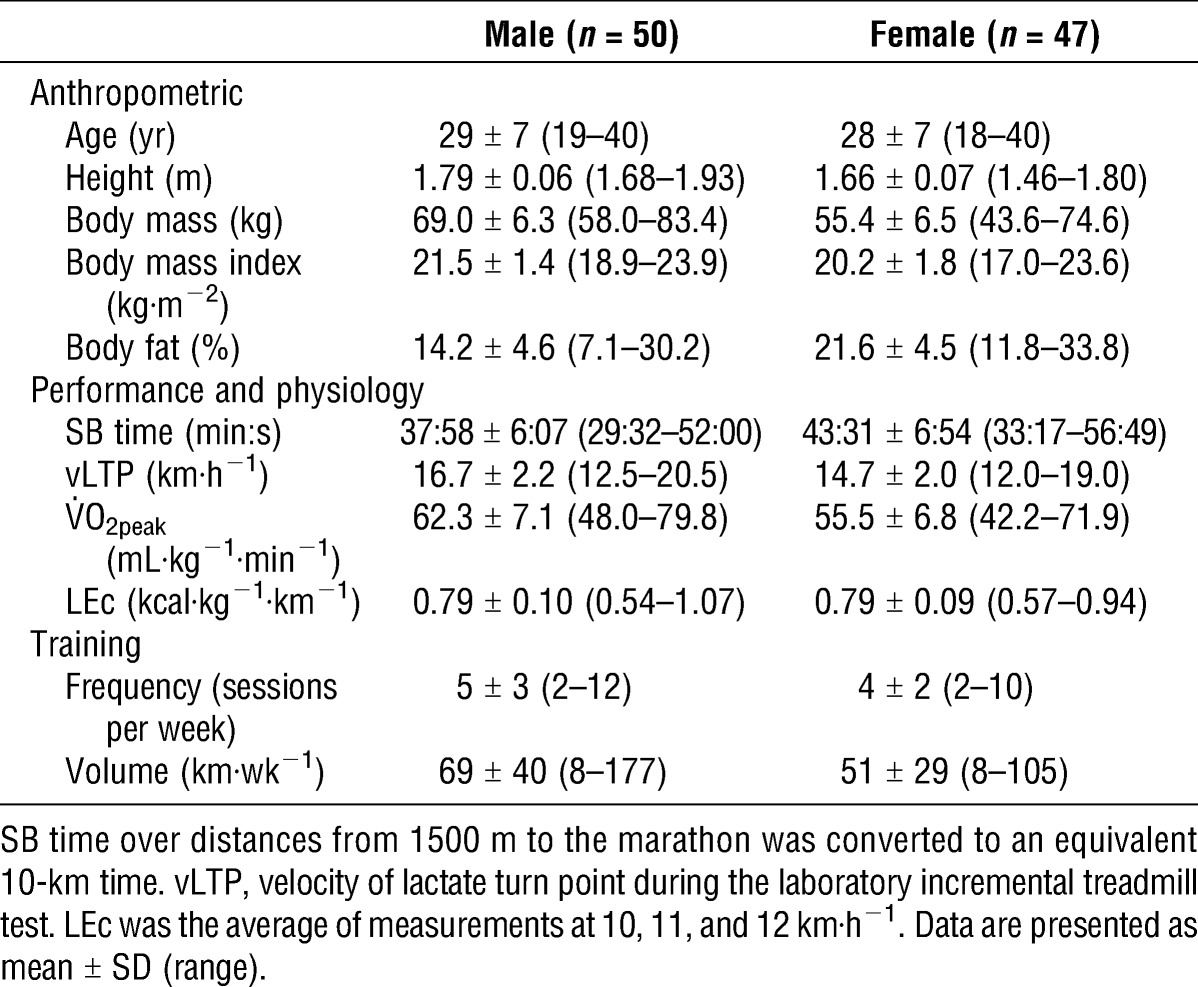
Ninety-seven healthy endurance runners volunteered and provided written informed consent before their participation in this study, which was approved by the NHS National Research Ethics Service and the Loughborough University Ethics Committee. All participants were regular runners (two times or more per week) and considered running to be their primary sport or physical activity. Runners of a wide range of performance standards were recruited (Table 1) according to their best running performance in the previous 12 months (season's best [SB] time), specifically their highest International Association of Athletics Federations (IAAF) points score for distances between 1500 m and the marathon in UK Athletics–sanctioned track and road races. All SB times were converted to an equivalent 10 km time using IAAF points scores (22). The cohort included 29 elite runners (males, SB time <31 min, n = 15; females <35 min, n = 14) and 68 recreational runners (males ≤52 min, n = 35; females ≤57 min, n = 33). All participants were required to have a body mass index of <24 kg·m⁻² and be free from moderate (previous 3 months) and minor (previous 1 month) musculoskeletal injury. Individual training history (typical training volume as kilometers and sessions per week) and SB time were collected by questionnaire, with the latter verified via official event results.

TABLE 1.

Descriptive characteristics of the participants.

Study Design

Participants visited the laboratory on two occasions separated by ≤14 d. All tests were completed in the morning (0730–1200 h) at a laboratory temperature of 18°C–20°C. Participants were instructed to arrive at the laboratory well hydrated, having avoided strenuous activity for 36 h, alcohol for 24 h, and caffeine ingestion for 6 h before testing. During the first session, whole-body superficial anthropometric measurements were obtained before participants performed a familiarization run, extensively practicing mounting and dismounting the moving treadmill belt for 30 min while wearing the mask required for respiratory measurements. The second session involved measurements of body mass and height, an assessment of body composition via dual-energy x-ray absorptiometry, an incremental treadmill running test, and a submaximal discontinuous incremental protocol of 4-min stages, immediately followed by a continuous protocol performed to volitional exhaustion. During the submaximal running protocol, there were recordings of three-dimensional full-body kinematics using an automatic motion capture system, respiratory gases to determine energy cost, and blood lactate ([La]_b) to determine the velocity of lactate turn point (vLTP). To focus the number of kinematic variables examined, literature and logical deduction were used to select 24 variables, from the five categories described, most likely to be related to performance and/or RE. In the case of vertical oscillation and horizontal velocity, we deliberately examined four similar measures to find the most powerful predictor of economy/performance from these categories. In addition to LEc (RE) and SB time (performance), vLTP, which is considered a powerful integrative physiological marker of performance dependent on V˙O_2max, fractional utilization, and RE (6,17,33), was used as an intermediate outcome variable (i.e., between RE and performance).

Anthropometry and body composition

Whole-body segmental anthropometric measurements involved a trained assessor taking 45 measurements of each participant according to a reduced set inertia model (49). These were used to calculate participant-specific segmental inertia parameters for 17 body segments (Inertia, Visual 3D; C-Motion, Germantown, MD) and used in the determination of joint center locations as well as segmental and whole-body CM positions. Height was measured using a stadiometer (Harpenden Stadiometer; Holtain Limited, Crymych, UK), and after voiding their bladder and bowel, each participant's body mass was recorded using digital scales (Seca 700; Seca, Hamburg, Germany). Whole-body dual-energy x-ray absorptiometry scans (Lunar iDXA; GE Healthcare, Madison, WI) were conducted using a standardized protocol while participants lay supine on the scanner, which was calibrated daily, and wore minimal clothing, typically running shorts and a vest.

Submaximal and maximal running trial

All participants wore running shorts and the same model of neutral racing flat running shoe (New Balance RC 1400 v2; New Balance, Boston, MA). Females were asked to wear a sports bra or a tight fitting crop top, and men were asked to run without a top. Fifty-six retroreflective markers were placed over joint centers and body landmarks so that they could be tracked by automatic motion capture. For the determination of breath-by-breath gas exchange and ventilation rates, participants wore a low-dead space mask and breathed through an impeller turbine assembly (Jaeger Triple V; Jaeger, Hoechberg, Germany). The inspired and expired gas volume and the concentration signals were continuously sampled, the latter using paramagnetic (O₂) and infrared (CO₂) analyzers (Jaeger Vyntus CPX; Carefusion, San Diego, CA) via a capillary line. Before each test, the gas analyzers were calibrated with gases of known concentration (16% O₂ and 5% CO₂) and ambient air, and the turbine volume transducer was calibrated using a 3-L syringe (Hans Rudolph, Kansas City, MO). The volume and the concentration signals were time aligned, accounting for the transit delay in capillary gas and analyzer rise time relative to the volume signal.

A calibrated “oversized” treadmill (Venus T200; H/P/Cosmos, Nussdorf-Traunstein, Germany; 854-kg treadmill mass, 2 × 0.75 m belt space, 11-kW motor, and 0–40 km·h⁻¹ speed range) was used throughout this study. Treadmill stiffness has recently been found to influence RE measurements (43), and qualitatively our treadmill is relatively stiff compared with more compliant smaller treadmills. After >15 min of quiet standing (while Vicon markers and face mask were applied), participants stepped on to the treadmill and stood in a standardized relaxed posture with hands resting on the handrails for 2 min while standing energy cost was measured. Participants then adopted a modified anatomical reference position: standing upright on the stationary treadmill belt with their feet shoulder width apart, facing directly forward, with their elbows flexed to 90° so that their forearms were pointing anteriorly. A 1-s recording was then taken using a 10-camera motion capture system (Vicon Nexus; Oxford Metrics Ltd., Oxford, UK) operating at 240 Hz. Data points for each marker location were averaged over this period and used as a representative recording of body configuration during quiet standing for the normalization of some kinematic variables (see next section) and defining segment poses. Right-hand laboratory and body segment coordinate systems were defined (x mediolateral, positive x to the right; y anteroposterior, positive y to the front; z superoinferior, positive z upward) from the perspective of an individual standing on the treadmill, with variable name abbreviations containing x/y/z as appropriate.

Participants performed an incremental running protocol, initially a discontinuous submaximal protocol that transitioned after vLTP into a continuous maximal protocol. Participants started at 8 km·h⁻¹ (males) or 7 km·h⁻¹ (females) with increments of 1 km·h⁻¹, and the treadmill incline was 0%. The submaximal protocol consisted of 4 min of running at each speed, followed by 30 s rest during which time a capillary blood sample of ~30 μL was obtained from the fingertip for analysis of [La]_b (YSI 2300; Yellow Springs Instruments, Yellow Springs, OH). The submaximal protocol progressed until [La]_b had risen by >2 mmol·L⁻¹ from the previous stage (or exceeded 4 mmol·L⁻¹), when participants began a continuous treadmill test. In this continuous test, the treadmill speed increased by 1 km·h⁻¹ every 2 min until volitional exhaustion. Respiratory gas data were recorded throughout the treadmill run, and motion capture data were recorded for 15 s starting ~30 s into each stage of submaximal running.

Data Analysis

Pulmonary gas exchange

Breath-by-breath V˙O₂ data were initially examined to exclude errant breaths (>4 SD from the local mean) caused by coughing, swallowing, etc. Subsequently, the breath-by-breath data were converted to second-by-second data using linear interpolation. Oxygen consumption (V˙O₂), carbon dioxide production (V˙CO₂), ventilation rate (V˙_E), and RER were quantified for the final 60 s of each stage of submaximal running and for 30 s during the second minute of quiet standing. V˙O_2peak was determined as the highest 30 s moving average.

vLTP

vLTP was identified via a derivation of the modified Dmax method (9). Briefly, a fourth-order polynomial curve was fitted to the speed–lactate relationship. Lactate threshold was identified as the final stage preceding an increase in [La]_b >0.4 mmol·L⁻¹ above the baseline level (lowest moving average of three values), and a straight line was drawn between the lactate threshold and the last 4-min stage of running (i.e., a rise of >2 mmol·L⁻¹ or exceeding 4 mmol·L⁻¹). Finally, vLTP was defined as the greatest perpendicular distance between this straight line and the fourth-order polynomial to the nearest 0.5 km·h⁻¹.

RE

The average second-by-second V˙O₂ and V˙CO₂ during the final minute of each submaximal running stage, and at rest during quiet standing, were used to calculate energy expenditure. Updated nonprotein respiratory quotient equations (35) were used to estimate substrate use (g·min⁻¹). The energy derived from each substrate was calculated by multiplying fat and carbohydrate use by 9.75 and 4.07 kcal, respectively (23). Absolute energy expenditure was calculated as the sum of the energy derived from fat and carbohydrate at rest and during each running velocity ≤ vLTP, and with an RER value of <1.00, to ensure an insignificant anaerobic contribution to energy expenditure. Energy expenditure at rest during quiet standing was subtracted from the running measurements to calculate the LEc. Subsequently, LEc was expressed in kilocalories per kilogram per kilometer (42). LEc data were averaged across the three highest common velocities that had valid energy cost data for all runners: 10, 11, and 12 km·h⁻¹. Runners for whom 12 km·h⁻¹ was >vLTP, or RER ≥1.00 at this velocity, were excluded from the analysis.

Kinematic variables

The raw marker data were initially labeled in Vicon Nexus with a combination of spline (small gaps) and pattern (larger gaps) filling (the maximum filled gap length was set to 10 frames). A bidirectional low-pass Butterworth filter (fourth-order, 15-Hz cutoff from residual analysis [48]) was applied to individual marker trajectories to remove high-frequency noise. The labeled and filtered data were then exported to Visual 3D (version 5.01; C-Motion, Oxford, UK) where a 17-segment model of the body was defined, from which three-dimensional joint centers and joint angles could be obtained. The model outputs were exported to Matlab (R2015b; Mathworks, Natick, MA), where algorithms were developed to calculate each of the key variables.

Ten consecutive strides were analyzed from the 15 s of data captured at each velocity. The instants of touchdown and toe-off for each step were identified using an optimized algorithm method based on analysis of >500 ground contacts on a force platform for various running speeds and footstrike patterns (19), which was an extension of previous methods (2,28). Initially, touchdown and toe-off windows were defined to limit the search regions: the touchdown window started when the anterior–posterior velocity of the heel marker changed from positive to negative and ended at the next minima in vertical heel position, and the toe-off window started 100 ms after touchdown and ended at the first of either the vertical toe position exceeding 0.1 m or reaching a maximum. Then within these windows, the instant of touchdown was defined by an algorithm identifying the first occurring peak of the vertical acceleration of the heel or first metatarsal head markers to account for all footstrike types. The toe-off algorithm used the time of peak vertical jerk (first derivative of acceleration) for the toe marker (positioned on the distal end of the hallux). In comparison with a force plate gold standard, this method resulted in touchdown, take-off, and GCT with mean offsets of +3.1, +2.1, and −1.1 ms, respectively, and root mean square errors for a single footstrike of 8.3, 5.6 and 9.0 ms, respectively (19).

On the basis of these events, the data were split into steps (e.g., right foot touchdown to left foot touchdown) and strides (e.g., right foot touchdown to right foot touchdown). Thereafter, kinematic variables were averaged across 10 strides, or 20 steps for each participant (i.e., both legs) at each running velocity up to and including their vLTP. Table 2 lists the 24 kinematic variables in five categories and their abbreviations. All length-dependent kinematic variables were normalized to standing height. The kinematic variables were then averaged across the three fastest common velocities (10, 11, and 12 km·h⁻¹) in the same way as the LEc measurements. If a variable was not recorded for a runner at one of these speeds but values existed at adjacent speeds, the missing value was estimated by linear interpolation. If this was not possible then the runner was removed from the analysis of this variable.

TABLE 2.

Descriptive kinematic data during steady state running.

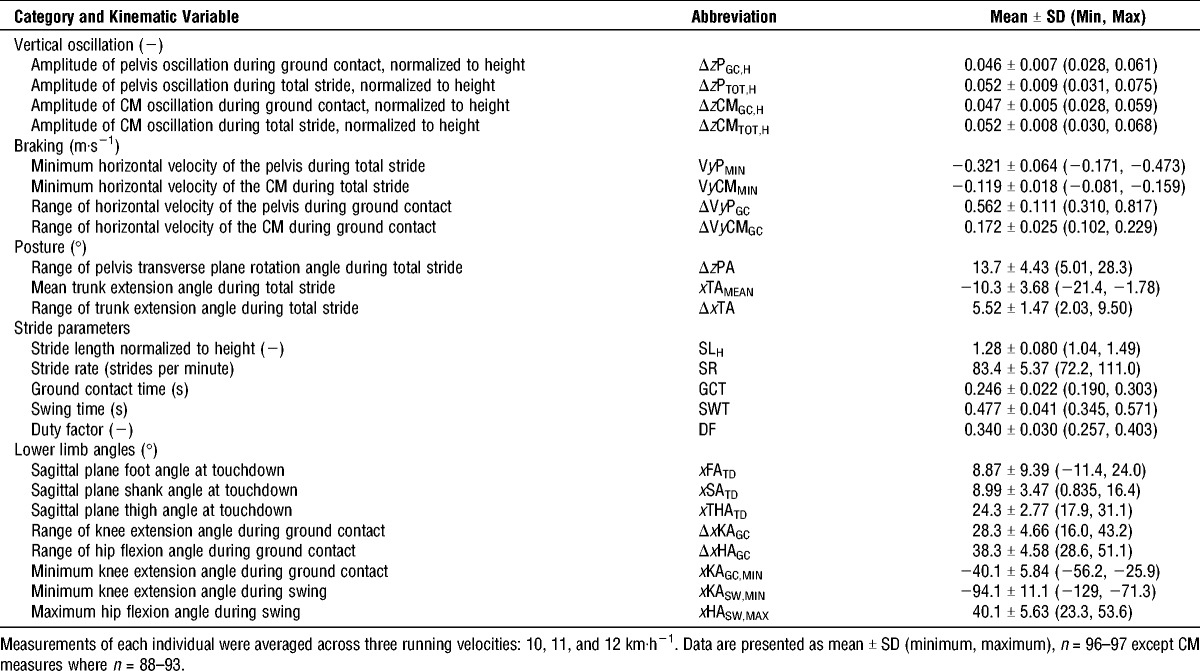
The measures of vertical oscillation were the vertical range of motion of the pelvis and CM during ground contact (ΔzP_GC,H and ΔzCM_GC,H) and throughout the whole stride (ΔzP_TOT,H and ΔzCM_TOT,H), and these measures were all normalized to height. Braking measures were minimum horizontal velocity of the pelvis and CM (VyP_MIN and VyCM_MIN) relative to mean anterior–posterior velocity during that step (i.e., to account for any net anterior–posterior movement relative to the treadmill belt) and the range in anterior–posterior velocity of the pelvis and CM during ground contact (ΔVyP_GC and ΔVyCM_GC). Trunk posture was assessed with mean forward lean of the trunk, relative to quiet standing (xTA_MEAN, i.e., average throughout the stride with flexion being negative); range of motion of trunk forward lean (ΔxTA); and range of transverse plane rotation of the pelvis during a complete stride (ΔzPA).

The ground contact events were used to define five temporal–spatial stride parameters: stride length normalized to height (SL_H), SR, GCT of each step, SWT between toe-off and touchdown of the same foot, and DF (the fraction of the stride cycle that was step GCT; Table 2). Eight measurements of lower limb angles were extracted (Fig. 1). Segment positions at touchdown (foot xFA_TD, shank xSA_TD, and thigh xTHA_TD) were determined relative to quiet standing. The range of motion of the knee (ΔxKA_GC) and hip (ΔxHA_GC) joints during ground contact was assessed as the difference between the most flexed and the most extended positions between touchdown and toe-off. The minimum knee joint angle (i.e., most flexed position) during ground contact (xKA_GC,MIN) and swing phases (xKA_GC,MIN) and the maximum (most flexed) hip angle (xHA_SW,MAX) were also calculated relative to quiet standing (with knee flexion as negative and hip flexion as positive).

FIGURE 1.

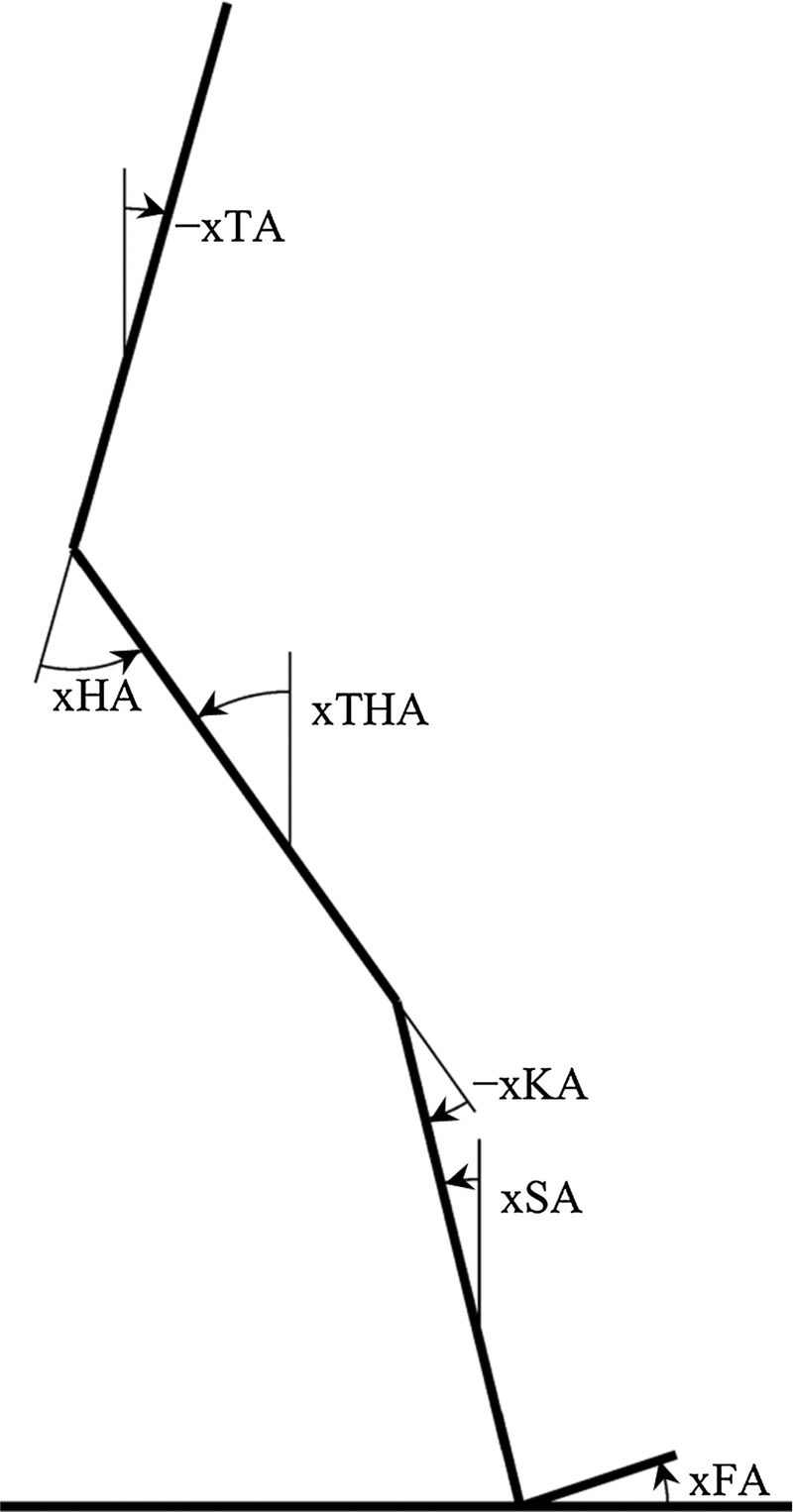
A stick figure illustrating the measurements of lower limb angles. Counterclockwise rotations from the anatomical position were defined as positive.

Statistical Analysis

Sex-independent measures of SB time (SB time-z) and vLTP (vLTP-z) were calculated as sex-specific z-scores (difference in standard deviations of each participant from the sex-specific mean, with faster [shorter] times and faster vLTP values given positive z-scores). LEc was found to be sex independent (males vs females, t-test P = 0.902). The relationships between kinematic variables and sex-independent measures of LEc, vLTP-z, and SB time-z were first assessed as bivariate relationships with independent Pearson's product moment correlations. P values were corrected for multiple tests using the Benjamini–Hochberg procedure (7) with a false detection rate of 5%, and significance was defined as adjusted P < 0.05. Kinematic variables significantly correlated with a specific outcome (e.g., LEc, vLTP-z, and SB time-z) were subsequently included in a stepwise multiple linear regression to calculate the variance explained by the best combination of kinematic variables. Of the four similar measures of vertical oscillation and horizontal velocity, the strongest of these measures/predictors was selected as the criterion measure of this category of kinematic variables and included in the regression analyses (when significantly correlated with the outcome). For inclusion in the regression equation, each additional kinematic variable had to significantly increase the explained variance (adjusted R², P < 0.05). Variables were excluded if the variance inflation factor >2.5; thus, the reported models all had a variance inflation factor of <2.5. All statistical analysis procedures were performed with IBM SPSS Statistics Version 21 (IBM Corp., New York, NY). Data are presented as mean ± SD.

RESULTS

Interindividual Variability in Running Performance, Economy, and Kinematics

SB time, expressed as an equivalent 10 km time, varied substantially (40:14 ± 7:20, range = 29:32–56:49 min:s, coefficient of variation [CV] = 17.8%), and there was also similar variability in vLTP (15.7 ± 2.3, range = 12.0–20.5 km·h⁻¹, CV = 14.7%; Table 1). Once resting energy cost was subtracted, LEc at 10–12 km·h⁻¹ varied by almost twofold (0.79 ± 0.09, range = 0.54–1.07 kcal·kg⁻¹·km⁻¹, CV = 11.7%) in this heterogeneous cohort, and this measure was independent of body mass (r = 0.078, P = 0.45).

Some runners exhibited more than twice the vertical oscillation of other runners, for all four measures (Table 2). Similarly braking measures displayed a 2.0- to 2.8-fold range, with VyP_MIN showing the greatest range. SR, averaged for 10–12 km·h⁻¹, varied from 72 to 111 strides per minute, although stride length normalized to height (SL_H) showed slightly less variability (1.04–1.49). GCT, SWT, and DF varied ≥1.6-fold. Orientation of the lower limb segments at touchdown varied by 33° (xFA_TD), 16° (xSA_TD), and 23° (xTHA_TD). Range of motion of the knee (ΔxKA_GC) and hip (ΔxHA_GC) during ground contact varied by 27° and 22°, respectively. Minimum knee joint angle during ground contact and swing phases varied by 31° (xKA_GC,MIN) and 57° (xKA_SW,MIN), and maximum hip joint angle during swing (xHA_SW.MAX) by 30°. The range of transverse plane pelvis rotation varied from 5° to 28°, the average forward lean of the trunk varied by ~20°, and the range of motion of forward lean of the trunk varied by 7°.

Bivariate Relationships between Kinematics and RE, vLTP, and Performance

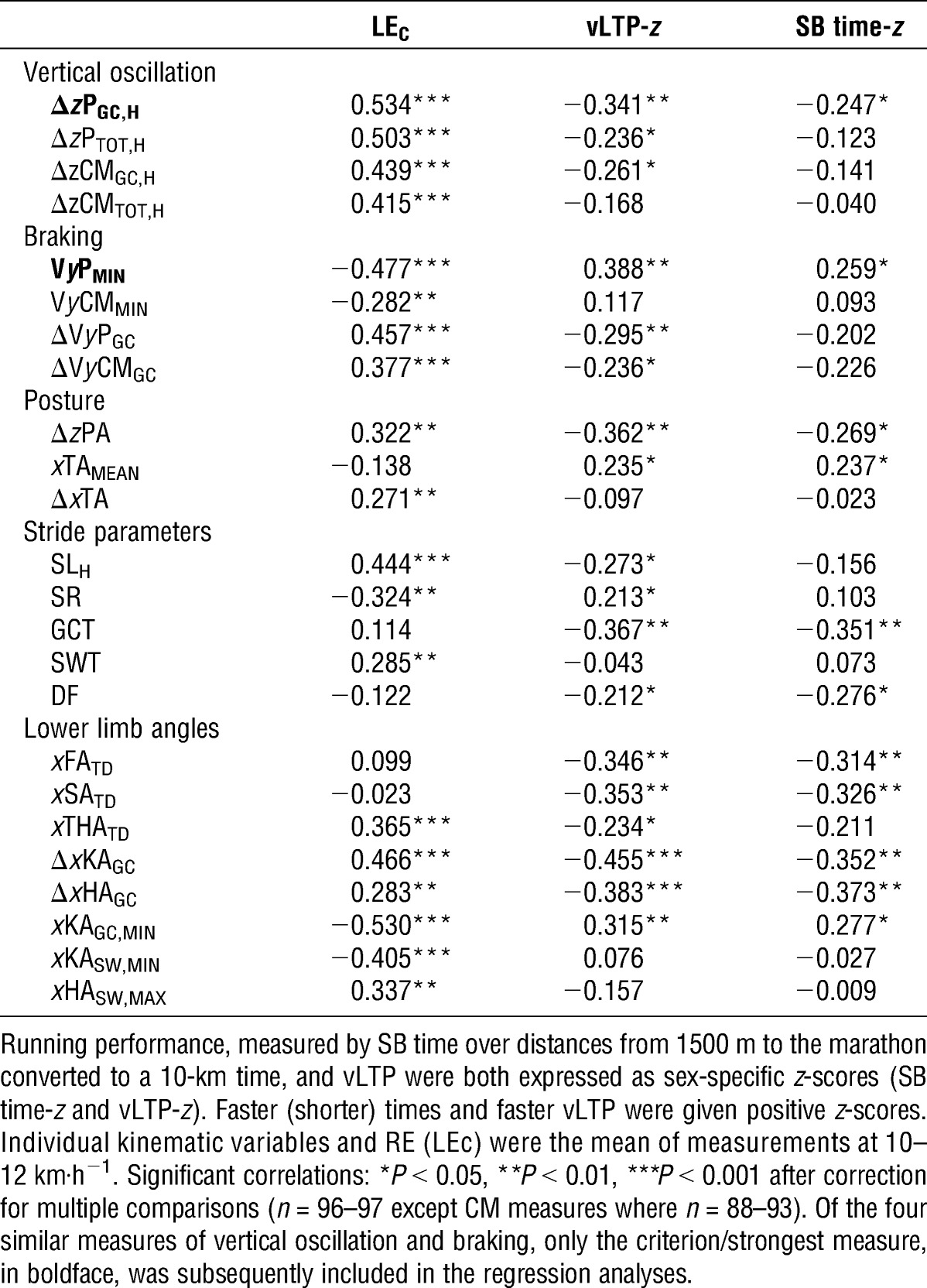
Of the 24 kinematic variables assessed, 19 were correlated with LEc, 18 with vLTP-z, and 11 with SB time-z (Table 3). These relationships were weak to moderate (|r| ≤0.534).

TABLE 3.

Correlation coefficients between kinematic variables, and RE, running performance and vLTP as a physiological marker of performance.

Vertical oscillation

Of the four measures of vertical oscillation, pelvis oscillation during ground contact normalized to height (ΔzP_GC,H) was the variable most strongly associated with all three outcomes, such that larger oscillation was related to greater energy cost, lower vLTP, and worse performance (LEc, r = 0.534, P < 0.001; vLTP-z, r = −0.341, P = 0.002; SB time-z, r = −0.247, P = 0.036; Fig. 2A). Therefore, ΔzP_GC,H was the criterion measure of vertical oscillation included in the regression analyses. Other measures of vertical oscillation were not associated with running performance, although for all four measures of vertical oscillation, larger oscillation was related to greater energy cost (r = 0.415–0.534, all P < 0.001).

FIGURE 2.

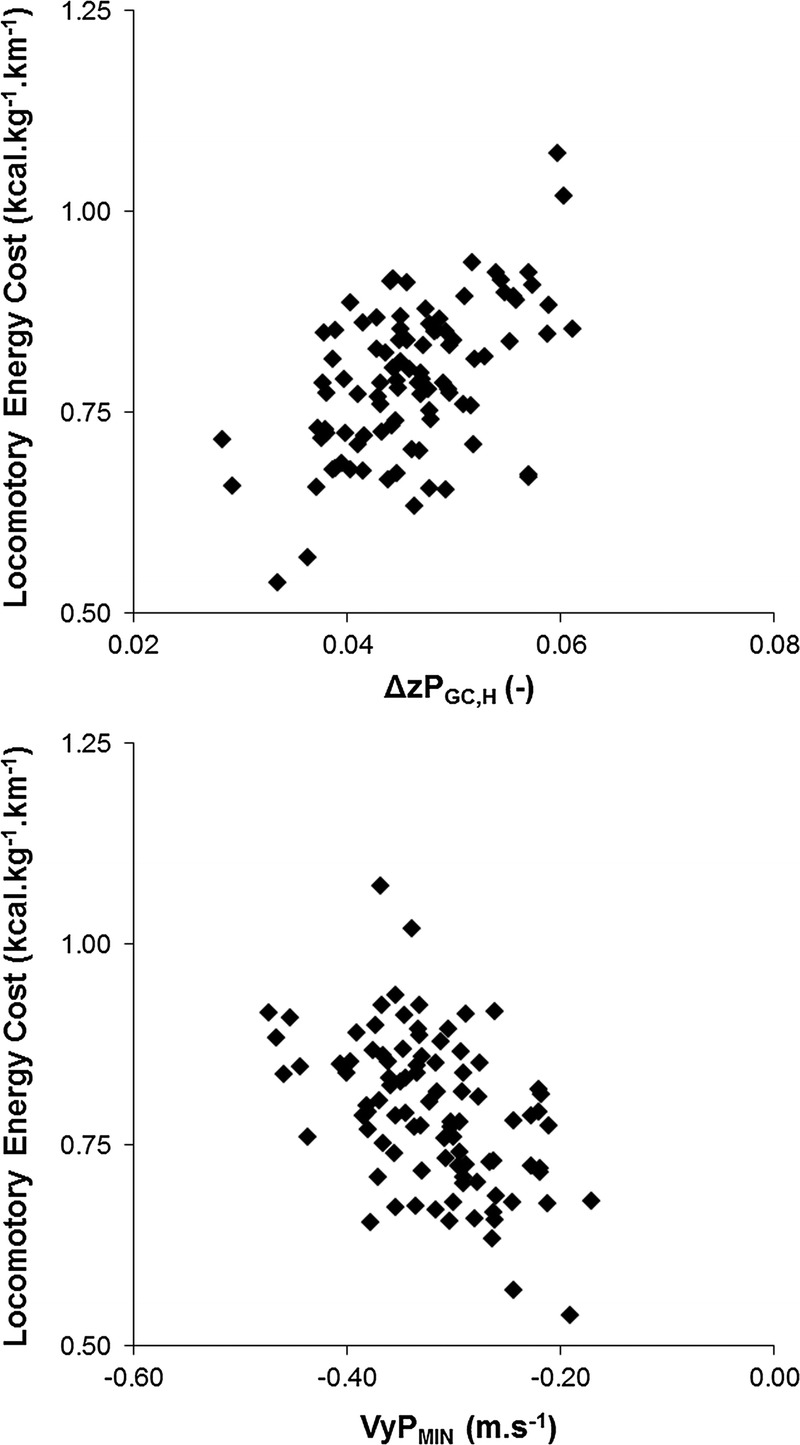
Scatter plots showing the criterion variables of vertical oscillation (ΔzP_GC,H, r = 0.534, P < 0.001) and braking (VyP_MIN, r = 0.477, P < 0.001) that were most strongly related to LEc.

Braking

Minimum horizontal velocity of the pelvis (VyP_MIN) was the measure of braking that was most strongly correlated with all three outcomes, with a lower minimum velocity (i.e., more deceleration/braking) related to higher energy cost, lower vLTP, and worse performance (LEc, r = −0.477, P < 0.00; vLTP-z, r = 0.388, P = 0.001; SB time-z, r = 0.259, P = 0.029; Fig. 2B). Hence, VyP_MIN was the criterion measure of braking included in the regression analyses. All four measures of braking were correlated with LEc, but none of the other measures of braking were related to performance.

Posture

Greater transverse plane rotation of the pelvis (ΔzPA) was related to greater energy cost, lower vLTP, and worse performance (LEc, r = 0.322, P = 0.002; vLTP-z, r = −0.362, P = 0.001; SB time-z, r = −0.269, P = 0.024). A greater range of forward/backward trunk lean (ΔxTA) was associated with higher energy cost, but not performance. A more upright/extended trunk posture (xTA_MEAN) correlated with better running performance but not LEc.

Stride parameters

A shorter GCT and the related variable of lower DF were not associated with LEc, but both were associated with vLTP-z (GCT, r = −0.367, P = 001; DF, r = −0.212, P = 0.049) and SB time-z (GCT, r = −0.351, P = 0.003; DF, r = −0.276, P = 0.021). The other stride variables showed no relationships with performance, although a shorter stride length normalized to height (SL_H) and higher SR both correlated with better LEc (r = 0.44 and r = −0.32, respectively).

Lower limb angles

A more extended knee during both ground contact and swing phases was associated with lower LEc (xKA_GC,MIN r = −0.530; xKA_SW,MIN r = −0.405). Lower ranges of motion of the knee and hip joints during ground contact were also correlated with better LEc (ΔxKA_GC r = 0.466; ΔxHA_GC r = 0.283). In addition, analogous range indices, i.e., less hip flexion during the swing phase (xHA_SW.MAX, r = 0.337) and smaller thigh angle at touchdown (xTHA_TD Fig. 1; r = 0.365), were also related to better LEc. Five measures of lower limb angles were associated with performance and vLTP. Greater foot and shank angles at touchdown (xFA_TD and xSA_TD; Fig. 1) and greater ranges of motion of the knee and hip during ground contact (ΔxKA_GC and ΔxHA_GC) were all correlated with worse performance and slower vLTP (SB time-z and vLTP-z; r = −0.211 to −0.455), whereas a more extended knee during ground contact (xKA_GC,MIN) was associated with better performance and faster vLTP (SB time-z r = 0.277; vLTP-z r = 0.315).

Multiple Linear Regression for RE, vLTP, and Performance

The regression model found 39.4% of the variance in LEc was explained by a combination of three variables (ΔzP_GC,H 27.7%; xKA_GC,MIN 9.2%; VyP_MIN 2.5%; Fig. 3). The regression analysis for performance found 30.5% of the variance in SB time-z to be explained by a combination of four variables (xSA_TD 10.0%; VyP_MIN 9.9%; DF 6.4%; xTA_MEAN 4.2%; Fig. 3). The same four variables also combined to explain 41.8% of the variance in vLTP-z (xSA_TD 19.0%; VyP_MIN 14.2%; DF 4.5%; xTA_MEAN 4.1%; Fig. 3).

FIGURE 3.

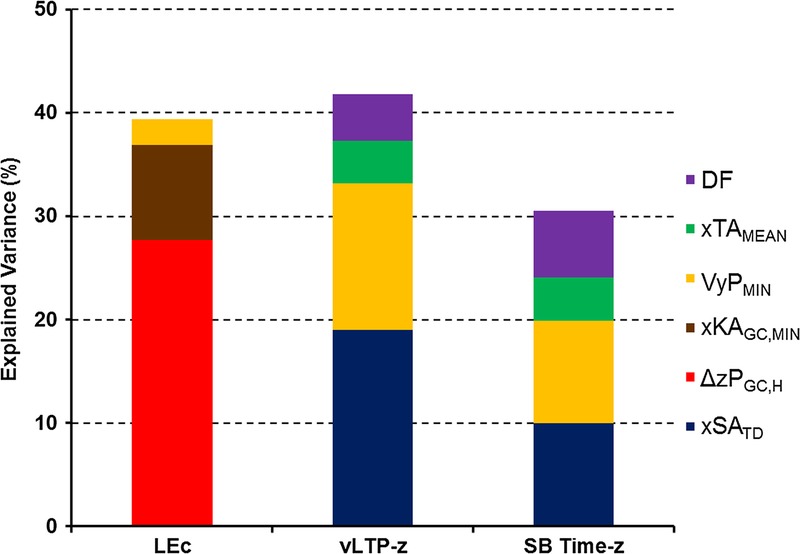
Kinematic determinants of RE, vLTP, and performance from stepwise multiple linear regression analyses. Running performance, measured by SB time over distances from 1500 m to the marathon converted to a 10-km time, and vLTP were both expressed as sex-specific z-scores (SB time-z and vLTP-z). RE was assessed as LEc. Kinematic measures and LEc were the average of measurements at three running velocities (10–12 km·h⁻¹).

DISCUSSION

To investigate the relationship between running kinematics, and economy, vLTP, and performance, this study assessed a large number of runners of heterogeneous performance standard, using thorough contemporary measurements of RE (energy cost) and kinematics (10 strides at each speed) concurrently at a range of speeds and with data averaged across several similar speeds (10–12 km·h⁻¹). This facilitated investigation of a focused set of hypothesis-driven kinematic variables and represents a substantial improvement in scale and rigor compared with previous investigations. Consequently, numerous kinematic variables were found to be associated with RE, vLTP, and performance (LEc 19 variables; vLTP 18 variables; SB time 11 variables). Independent regression analyses revealed that three variables (vertical oscillation of the pelvis normalized to height, minimum knee joint angle during ground contact, and minimum horizontal velocity of the pelvis) explained 39% of the variability in LEc. In addition, a consistent set of four variables (minimum horizontal velocity of the pelvis, shank angle at touchdown, DF, and forward lean of the trunk) combined to explain both a remarkable 31% of the variability in running performance (SB time) and also 42% of the variability in vLTP, a physiological marker of performance. Previous research has not found any kinematic variables to be related to performance and only unconvincing evidence for isolated variables to be related to economy. Therefore, this study provides novel and robust evidence for the importance of overall running technique as an important component of RE and performance. Furthermore, it highlights, for the first time, the significance of several individual kinematic variables; for example, minimum horizontal velocity of the pelvis, which contributed to all three regression models.

The current cohort had a wide range of SB times (performance variability) and also exhibited substantial variability in the majority of kinematic measures, although mean values were comparable with previous reports (1,46). RE measured as LEc showed a large twofold range across the whole cohort, which appears noticeably greater than previous reports primarily because the current study removed resting energy cost to isolate the energy cost of running/locomotion (i.e., LEc). For comparison, the oxygen cost of running at 10–12 km·h⁻¹ in this cohort was 200.3 ± 16.7 mL·kg⁻¹·km⁻¹ (range, 160.6–248.8 mL·kg⁻¹·km⁻¹), and thus similar to a previous 0% gradient report (24). Although we used an established correction for multiple correlations (7), given the number of tests performed, we are cautious about overinterpreting isolated, significant, yet weaker correlations. Therefore, in our interpretation, we have focused on variables with stronger correlations that contributed to the regression models or were related to multiple outcomes, or were part of a cluster of related variables, to reduce the possibility of highlighting false positives.

The consistency of the stronger kinematic correlates (e.g., GCT, VyP_MIN, and ΔzP_GC,H), and the selection of the same four variables within the regression models for actual performance (SB time) and vLTP, as an integrative physiological marker of performance, reinforces the validity of the findings. The physiological marker of performance was correlated with a wider range of kinematic variables and had a stronger regression model than actual performance (vLTP, 18 correlations and 42% of the variance explained vs SB time, 14 correlations and 31% of the variance explained), likely because of the controlled conditions and concurrent measurement of vLTP as opposed to the variable races, conditions, and timing (preceding 12 months) of SB time.

Braking

One of the novel findings of the current study was the relationship of minimum velocity of the pelvis (VyP_MIN), as the criterion measure of braking, with RE (r = −0.477), vLTP (r = 0.388), and actual performance (SB time r = 0.259), and that this variable also contributed to the explained variance in all three regression models (SB time 14.2%, vLTP 9.9%, and LEc 2.5%). Braking has been widely hypothesized as a possible determinant of RE and performance (20,25,26,34,39), although the only limited evidence to date surprisingly reported a greater change in horizontal velocity of the CM to be related to better RE in 14 elite female runners (47). This is clearly in contrast to the findings of our larger more rigorous investigation. In addition, for the elite runners in our cohort (n = 29), measurements of VyP_MIN at 10–12 km·h⁻¹ were correlated with LEc (r = 0.587, P = 0.001) and vLTP (r = 0.658; P < 0.001). Therefore, the current comprehensive study convincingly demonstrates the importance of minimizing braking (i.e., reducing VyP_MIN) and thus fluctuations in horizontal velocity to optimize RE and performance.

The minimum velocity of the pelvis (VyP_MIN), rather than the CM (VyCM_MIN) or the change in velocity of the pelvis (maximum-minimum, e.g., ΔVyP_GC), was the strongest index and thus the criterion measure of horizontal velocity fluctuation. This may be because during initial ground contact, VyP_MIN better reflects the magnitude of any braking effect of the stance leg, and/or the swinging leg, on the pelvis. By contrast, CM velocity during initial ground contact may be less sensitive to these braking effects because of the movement of the other limbs, torso, and head. The relationship of braking with RE and performance is not thought to be due to the act of braking per se, which involves passive impact forces and eccentric muscle activity. Rather, greater braking during the initial phase of ground contact subsequently necessitates the generation of larger horizontal propulsive forces in compensation to reaccelerate during the remainder of the step and maintain the prescribed running velocity. The generation of propulsive force involves energetically expensive concentric contractions (36) and appears to be a major component of the metabolic cost of running (13), and thus the influence of braking on economy and performance is entirely logical.

Vertical oscillation

Vertical displacement of the pelvis during ground contact (ΔzP_GC,H) was the criterion measure of vertical oscillation as it was consistently the strongest correlate of economy, vLTP, and performance. It is possible that vertical oscillation of the pelvis better reflects the stiffness of the leg during the stance phase, whereas the vertical oscillation of the CM may be sensitive to, and thus potentially confounded by, movements of the arms, trunk, and head. Alternatively, it is possible that the importance of CM oscillation is obscured by any noise/error in the position and/or anthropometry of the 17 body segments. Nonetheless, the finding that improved outcome measures were related to reduced vertical displacement of the pelvis during ground contact is entirely consistent with the idea that minimizing vertical oscillation (3,38,46) and having a stiffer leg (15) are beneficial for RE and performance.

The finding of a relationship between vertical oscillation and performance (r = −0.247 to −0.341) appears to be entirely novel, although surprisingly ΔzP_GC,H did not contribute to the regression models for performance or vLTP. ΔzP_GC,H was related to LEc (r = 0.534), explaining 28% of the variance within the regression model, indicating that vertical oscillation may be an important determinant of RE. Although a relationship between vertical oscillation and RE has been widely postulated, the evidence has been opaque (related, 19.45; unrelated, 46). Given imperfect elastic energy return, greater vertical oscillation necessarily involves more work performed against gravity, and therefore the observation of greater vertical oscillation being detrimental to economy and performance appears highly plausible.

Posture

Holding the trunk posture steady in the sagittal and horizontal planes during the running motion (i.e., lower range of motion) was related to better economy and to a lesser extent performance, highlighting the detrimental influence of these extraneous movements. Forward lean of the trunk (xTA_MEAN) was unrelated to LEc but was weakly correlated with, and contributed to the regression model of, actual performance (r = 0.24; 4.2%). By contrast, previous work found forward lean of the trunk to be associated with RE, but not performance, in a smaller cohort (46).

Stride parameters

A shorter GCT and the related lower DF were correlated with better running performance and vLTP (GCT r = −0.351 to −0.367; DF r = −0.212 to −0.276). Surprisingly, it was DF, not GCT, that contributed to the regression models for performance (SB time 6.4%) and vLTP (4.5%) rather than GCT. However, neither of these variables was associated with LEc. Previous studies have reported shorter GCT to be associated with better (34,38) and poorer RE (16), which in the context of our findings appear to be spurious results from small groups of runners. The discrepancy between the association of GCT with performance, but not RE, is surprising given that the assumed mechanism by which kinematic factors affect performance is via RE. This might suggest that either short GCT or low DF values are by-products rather than determinants of running performance (i.e., a coincidental association), or more likely that the ideal kinematics for running performance are determined by optimizing factors beyond simply minimizing LEc. For example, minimizing neuromuscular activation, muscular force, or stress has been suggested to explain why the preferred pedaling rate of cyclists is substantially higher than the energetic optimum (~90 vs 60 rpm (32)). Further credence has been added to this hypothesis in simulations of running, where minimizing muscle activations led to results that were more similar to measured performances than minimizing the cost of transport (29). However, the present study provides the first robust empirical evidence that these factors seem to be important in determining running kinematics in practice.

By contrast, a shorter stride (SL_H) and a higher SR were related to LEc, perhaps suggesting that overstriding leading to an uneconomically low SR is a common technique. A recent review concluded that a higher SR may be a consequence of increased stance leg stiffness leading to reductions in energy absorption at the main joints and reduced vertical oscillation of the CM (40), which is consistent with the findings of this study.

Lower limb angles

Greater foot and shank segment angles at touchdown (xFA_TD and xSA_TD; Fig. 1) and knee and hip range of motion during ground contact (ΔxKA_GC and ΔxHA_GC) were negatively related to running performance and vLTP. This may be due, at least in part, to a more anteriorly extended leg at touchdown, and greater range of movement during ground contact being associated with a longer GCT (all four of these lower limb angles were significantly correlated with GCT; r = 0.346–0.638, P ≤ 0.001). In fact, these four variables included the strongest kinematic correlates of SB time (ΔxHA_GC, r = −0.373) and vLTP (ΔxKA_GC, r = −0.455). Thus, these were initially the first variables entered in the regression model; however, they were subsequently removed from the model presumably because other overlapping factors explained a greater proportion of the variance. Nonetheless, shank angle at touchdown (xSA_TD) explained 10.0% of the variance in performance (SB time) and 19.0% of the variance in vLTP. Conversely, a smaller previous study found shank angle at touchdown to contribute to RE, but not performance (46).

Lower limb angles reflecting greater knee and hip movement were associated with higher LEc (6 variables). Of these variables, the strongest correlates of LEc were those reflecting flexion of the knee during ground contact (xKA_GC,MIN r = −0.53; ΔxKA_GC r = 0.466) with xKA_GC,MIN explaining 9.2% of the variance in LEc within the regression analysis. This is again intrinsically linked to leg stiffness because greater lower limb joint flexion during stance may reflect more energetically expensive eccentric followed by concentric muscle activity, rather than relatively efficient isometric contractions (36).

Greater flexion of the knee and hip of the swinging leg was associated with higher LEc (xKA_SW,MIN r = −0.405; xHA_SW,MAX r = 0.337) but not performance. Flexion at these joints may also have contributed to the braking effect on the pelvis described previously. This is interesting given that “picking up” the knees and feet are popular coaching points. However, the current results indicate that excessive knee and hip flexion leads to unnecessary energy expenditure at the speeds considered in this study.

Overall influence of running technique

Running kinematics clearly changes with speed, and therefore to avoid this confounder, we examined running kinematics at the highest range of common speeds (10–12 km·h⁻¹) before significant fatigue or anaerobic metabolism in all individuals (i.e., ≤vLTP), which was necessarily quite different to race pace particularly for the elite runners in our cohort. That kinematics at 10–12 km·h⁻¹ was related to economy measured at these same speeds was perhaps expected, but more unexpected was the relationship between kinematics at 10–12 km·h⁻¹ and performance at speeds that for some runners were substantially higher. This might suggest that running technique relative to other runners is consistent across speeds; that is, runners with the smallest shank angle at touchdown at 10–12 km·h⁻¹ also have smallest value at higher speeds. Alternatively, it is also conceivable that running technique is sensitive to relative intensity and that a low vLTP, close to 10–12 km·h⁻¹, exerts an influence on running technique compared with individuals with a higher vLTP and could potentially produce a coincidental relationship between kinematics and performance. However, because 75% of the cohort had a vLTP of ≥14 km·h⁻¹, it seems unlikely that fatigue or metabolic factors had a strong influence on running kinematics measured at an average 11 km·h⁻¹. Finally, it is possible that kinematics at speeds closer to race pace may have an even stronger relationship with performance, but this possibility has not been examined, and future studies could concentrate on higher standard runners to derive valid kinematics at higher speeds.

The large number of correlations and the relatively small number of variables within the regression model (e.g., LEc 19 correlations, three variables in the regression) demonstrates the extent of overlap among the kinematic variables and suggests that consideration of isolated variables should be treated with caution. This extensive overlap is to be expected as the different elements of running technique are inevitably inter-related. In particular, pelvis movements are necessarily consequent to the actions of the lower limbs, specifically lower limb angles and stride parameters (which are themselves interrelated). Although the purpose of this study was not to examine the inter-relationship of the diverse kinematic variables we assessed, it was notable that VyP_MIN, ΔzP_GC,H, and ΔzPA were all related to both SL_H (r = 0.358–0.659; P < 0.01) and ΔKA_GC (r = 0.359–0.548; P < 0.01). Furthermore, although the focus of this study was clearly on kinematics, it should be recognized that kinetics, including ground reaction forces and net joint torques, anthropometry, and tissue mechanics, are important underpinning aspects of the kinematic observations in this study. For example, the alignment of the resultant ground reaction force vector may be crucial in minimizing the joint moments and thus muscle forces (8,13).

Running technique is presumed to influence performance via RE, one of many factors affecting performance. Therefore, it is logical that kinematic variables explained a smaller proportion of the variance in performance (SB time 31%) compared with LEc (39%). However, the higher proportion of vLTP that was explained by kinematic variables (42%) compared with LEc was surprising, given that vLTP is a physiological marker of performance considered to be dependent on the integrative combination of V˙O_2max, fractional utilization, and LEc (6). This might suggest that kinematic variables are either coincident with, or determinants of, other aspects of performance. This study was not able to quantify the extent of cause and effect in any of the relationships examined. It is possible that other variables not included in the current study (e.g., anthropometric factors such as segmental mass and inertia) could influence both our predictor (kinematics) and outcome (RE and performance) variables and thus contribute to the relationships we have observed. However, on the basis of the diversity of kinematic variables between runners, the intuitive influence of technique on economy and performance (3), the logical rationale for the influence of some of the variables identified (e.g., vertical oscillation and braking as discussed previously), and the magnitude of the observed relationships, it seems likely that there is a causal component to these relationships.

Hence, we recommend consistent forward velocity of the pelvis, with minimal vertical oscillation and transverse rotation for enhancing economy and performance, and it may be that improving the stride parameters (DF, GCT, and SL_H) and lower limb angles identified (xSA_TD, ΔxKA_GC, and ΔxHA_GC xKA_GC,MIN) may help to enhance these aspects of pelvis movement. Given the apparent importance of technique to performance, it is recommended that runners dedicate an appropriate proportion of their preparation to technical development. Future work should examine the plasticity of overall running technique in response to acute and chronic interventions, with particular reference to the specific variables we have identified, to establish the extent to which technique, and ultimately economy and performance, can be enhanced. In addition, the nature of these relationships should be explored, specifically whether they are causative or simply correlates of other influential variables.

In conclusion, the current study provides novel and robust evidence that running technique explains a substantial proportion of the variance in RE (39%) and performance (31%). It is therefore recommended that runners and coaches be attentive to stride parameters (lower DF, shorter GCT, and shorter stride length) and lower limb angles (more vertical shank and plantarflexed foot at touchdown, and a smaller range of motion of the knee and hip during stance) in part to optimize pelvis movement (minimal braking, vertical oscillation, and transverse rotation), and ultimately enhance performance.