Biexponential T_1ρ Relaxation Mapping of Human Knee Cartilage in-vivo at 3T

Abstract

Purpose

To demonstrate the feasibility of biexponential T_1ρ relaxation mapping of human knee cartilage in-vivo.

Methods

A 3D customized Turbo-Flash sequence was used to acquire T_1ρ-weighted images from healthy volunteers employing a standard 3T MRI clinical scanner. Series of T_1ρ-weighted images were fitted using monoexponential as well as biexponential models with two- and four- parametric non-linear approach, respectively. Non-parametric Kruskal-Wallis and Mann-Whitney U statistical tests were used to evaluate the regional relaxation and gender differences respectively with a significance level, p-value of 0.05.

Results

Biexponential relaxations were detected in the cartilage of all volunteers. Short/long relaxation components of T_1ρ were estimated to be 6.9/51.0ms respectively. Similarly, the fractions of short/long T_1ρ were 37.6/62.4%. The monoexponential relaxation of T_1ρ was 32.6ms. The experiments showed good repeatability with coefficient of variation (CV) of less than 20%

Conclusion

Biexponential relaxation model better fit to T_1ρ relaxation decay than monoexponential model in the knee cartilage^(R2.4.1). Biexponential T_1ρ components could potentially be used to increase the specificity to detect early osteoarthritis by measuring different water compartments and their fractions.

Graphical abstract

In this study, we demonstrated the feasibility of in-vivo biexponential T1ρ relaxation mapping of knee articular cartilage of 8 healthy volunteers using 3T MRI in clinically feasible scan times. Our preliminary results show that the biexponential model may better represents the T1ρ relaxation decay in articular cartilage. It is possible distinguish between tightly bound and loosely bound water compartments associated with macromolecules in the articular cartilage.

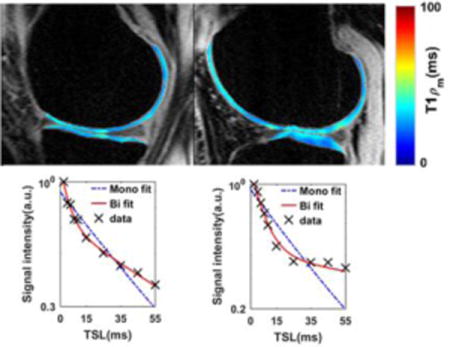
1 INTRODUCTION

Osteoarthritis (OA) is a degenerative, heterogeneous, and multifactorial disease that is mainly identified by the progressive loss of hyaline articular cartilage¹. The symptoms include joint pain, limited range of motion, stiffness, and swelling^2,3. Several methods including plain radiographs, microfocal radiographs, radionuclide imaging, arthrography, computed tomography (CT), ultrasound, arthroscopy and magnetic resonance imaging (MRI) of articular cartilage have been used to detect OA^4,5 among these methods, MRI shows more sensitivity in detecting Osteoarthritis⁵. Different MRI techniques have been proposed to detect OA⁶. The standard techniques for imaging cartilage are fat-saturated T₂-weighted, proton density-weighted fast spin echo (FSE) sequences, and T₁-weighted spoiled gradient echo (SPGR) sequences⁷. However, these standard techniques are insensitive in detecting the early stage OA⁸.

Articular cartilage is a connective tissue of arthrodial joints that is composed of a dense extracellular matrix (ECM). ECM is principally composed of collagen (~20%), proteoglycans (PG, ~5%), and water (~75%)^9,10. Early stage OA is associated with loss of PG, increase in water content, and changes in the structure of collagen fibers^{(R2.4.2)11,12}. Spin-lattice relaxation in the rotating frame (T_1ρ) has been extensively used for quantifying the biochemical changes associated with PG, water content and disruption of collagen and anisotropy^8,13–16. Different water compartments in articular cartilage lead to different T_1ρ relaxation components_. As a result of the change in the biochemistry of cartilage and increase in water content due to OA, an elevated T_1ρ relaxation time is expected. T_1ρ relaxation time was measured in-vivo and the relationship between the elevation of T_1ρ and cartilage degeneration has been demonstrated in previous studies^{8,11,13,17–20}. T_1ρ is found to be sensitive to the slow motional interactions between local macromolecular environments and bulk water²¹, and as a result to proteoglycan content in articular cartilage²².

Different components of relaxation can be identified by MR relaxation experiments on cartilage^23–26, such as T1_ρ from collagen or proteoglycan (PG) macromolecules, from fragmented PG molecules, from water molecules trapped within collagen fibrils, and from free water molecules^(R2.4.3). Hence, biexponential fitting could potentially provide more information on different water compartments than monoexponential fitting. To the best of our knowledge, in all of the previous in-vivo studies^{8,11,13,14,17,20}, T_1ρ was described by a monoexponential decay model, which shows the mean of relaxation time from all of the water compartments. In addition biexponential studies have been limited to ex-vivo cartilage tissue specimens or animal studies^23,26 and biexponential T1ρ has yet to be reported in-vivo. The goal of this paper is to demonstrate the feasibility of in-vivo biexponential analysis of T_1ρ relaxation times of articular cartilage in the human knee joint using 3T MRI in clinically feasible scan times.

2 METHODS

2.1 Monte Carlo Simulations

The mono- exponential T_1ρ (T_1ρm) relaxation can be estimated by fitting the signal intensities of each pixel at different spin-lock durations (S (TSL)) to:

$$S(\mathit{TSL})=A_m\exp (-\frac{\mathit{TSL}}{T_{1\rho m}})$$ [1]

Similarly, the biexponential relaxation components were estimated using:

$$S(\mathit{TSL})=A_s\exp (-\frac{\mathit{TSL}}{T_{1\rho s}})+A_l\exp (-\frac{\mathit{TSL}}{T_{1\rho l}})$$ [2]

Where TSL is the spin-lock duration, T_1ρs and T_1ρl correspond to the short and long relaxation time components, respectively. The weightings (fractions) of the short (A_s) and long components (A_l) are usually reported in percentage as a_s % = 100 × A_s/(A_s + A_l) and a_l % = 100 × A_l/(A_s + A_l), respectively.

Monte Carlo simulations²⁷ were performed to determine a set of TSLs sufficient for estimating four parameters in a^(R2.4.4) biexponential model (two relaxation times and the corresponding fractions), as well as covering the possible T_1ρ values in the cartilage. For^(R2.4.5) given signal to noise ratio (SNR) the smaller set of TSLs is desired to minimize the total acquisition time.

To perform the simulation, a set of signals was generated with different set of spin‐lock durations (TSL) with known T_1ρ components fractions (assuming A_s + A_l = 1) and time constants. A random noise with normal distribution N(0, σ) was added to the signal. The SNR is defined as 1/σ²⁸. The relaxation components were then estimated by fitting the biexponential model to the noisy signals. The process was repeated for 1000 independent noise trail and the mean absolute percentage error (MAPE) was calculated as:

$$\mathit{MAPE}=\frac{100}{n}{\displaystyle \sum \frac{\left|y_a-y_e\right|}{y_a}}$$ [3]

Where y_a and y_e are the actual and estimated values, respectively and n is the total number of noise trail (n = 1000) in Monte Carlo simulation.

2.2 T_1ρ-weighted MRI Acquisition

T_1ρ-weighted MR images were acquired on a 3T whole body clinical MRI scanner (Prisma, Siemens Healthcare, Erlangen, Germany) with a 15-channel Tx/Rx knee coil (QED, Cleveland OH). A 3D Cartesian turbo-Flash sequence was customized to enable T_1ρ imaging with varying spin-lock durations.

The most basic T_1ρ preparation module consists of a 90° RF pulse along the x-axis to tip the magnetization to the transverse plane, a long continuous spin-lock pulse along the y-axis to lock the magnetization in the transverse plane, and finally a second 90° RF pulse in the opposite direction of the first one (−x) to tip the magnetization back to its original direction before the readout. However, in practice, the actual spin-lock field strength and direction are affected by the field inhomogeneities. Any B_o or B₁ inhomogeneity causes the magnetization to deviate from the transverse plane which leads to a complicated magnetization evolution instead of the exponential decay related only to T_1ρ relaxation time²⁹. Several methods were proposed in the literature to compensate for the field inhomogeneities^30–36. The paired spin-lock pulse method^35,36 which was used in this study tries to self-compensate the B₁ inhomogeneities by changing the phase of spin-lock pulse. In this method, the spin-lock pulse was divided into four segments with alternative phase to compensate for B₁ inhomogeneities and a refocusing (180°) pulse was applied between two pairs to compensate the B₀ inhomogeneities, respectively. The T_1ρ imaging pulse sequence diagram is shown in Figure 1. A long delay time was added after each phase line acquisition for T₁ recovery.

Figure 1.

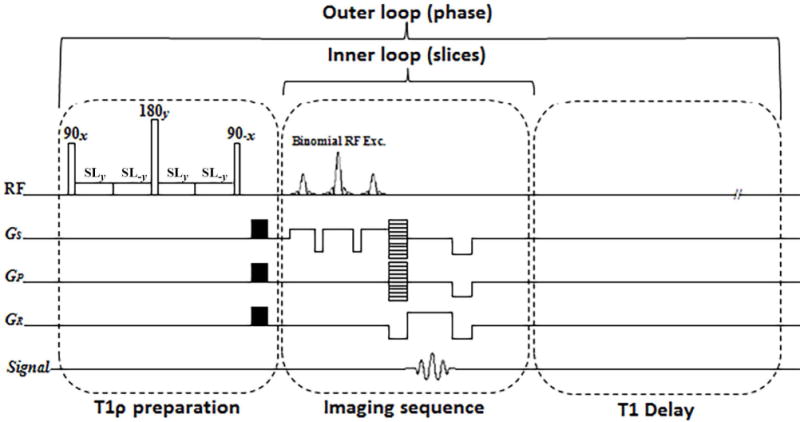
The imaging sequence timing diagram consists of T_1ρ preparation, 3D turbo-Flash readout, and T1 recovery delay. To compensate the effect of B₁ inhomogeneities, the spin-lock pulse (SL) was divided into four segments with alternative phase. A 180° refocusing pulse was applied between two pairs for B₀ inhomogeneity compensation. One phase line from all slices was acquired after applying the preparation module (inner loop). After T₁ restoration delay the preparation module was applied again to acquire another phase line (outer loop).

To estimate T_1ρ relaxation, multiple sets of 3D scans were acquired with different spin-lock durations (TSL). The sequence acquisition parameters were: TR/TE 1500ms/4ms, flip angle 8°, matrix size 256 × 128 × 64, spin-lock frequency=500Hz, slice thickness = 2mm, field of view (FOV) = 120mm², and receiver bandwidth = 515 Hz/pixel.

2.3 Ex-vivo Bovine Cartilage Study

Fresh bovine patellae cartilage specimens (n = 3, ~6months old) were obtained from a slaughterhouse (Max Insel Cohen, Inc., Livingston, NJ) and equilibrated in phosphate-buffered saline (PBS) for one hour prior the MRI study. The tissue was covered with parafilm to avoid dehydration during the scans. T_1ρ-weighted images were acquired from 3 specimens. The scans were taken in axial plane at 15 different TSLs including 0.5/2/4/6/7/8/10/12/15/20/25/35/45/55/65ms. To investigate the effect of reduced SNR due to GRAPPA^(R2.4.6)-parallel imaging³⁷ on the estimated relaxations time, the images were acquired with acceleration factor of AF = 1(fully sampled), 2, 3 and 4. The total acquisition time for 3D data set with 15 TSLs were 46, 27, 22, and 18 minutes for AF = 1 to 4 respectively.

2.4 In-vivo knee Study

Eight healthy volunteers (n=4 females, and n=4 males) were recruited for this study with a mean age of 30±4 years, mean weight of 63±15 kg, and mean height of 169±12cm (female volunteers: mean age of 26 ± 3 years, weight of 52 ± 6 kg and height of 159±6 cm; male volunteers: age of 30±3 years, weight of 74±12 kg and height of 177±3cm). The study was approved by the institutional review board (IRB) and all eight volunteers provided written informed consent prior to MRI scanning.

T_1ρ-weighted images were acquired in sagittal plane using a binomial water excitation pulse as RF excitation at 10 different TSLs including 2/4/6/8/10/15/25/35/45/55 ms. The GRAPPA parallel imaging method with the acceleration factor of AF= 3 was used to decrease the total acquisition time from 30 to 15 minutes for in-vivo imaging.

The acquired T_1ρ-weighted scans were analyzed using a custom-written script in MATLAB (R2016a, The MathWorks Inc., Natick, MA, USA). T_1ρ relaxation maps were calculated pixel by pixel over five consecutive slices in five regions of interest (ROI): medial-tibia (MTC), medial-femoral (MFC), lateral-tibia (LTC), lateral-femoral (LFC), and Patellar (PC) cartilages (Figure 4).

Figure 4.

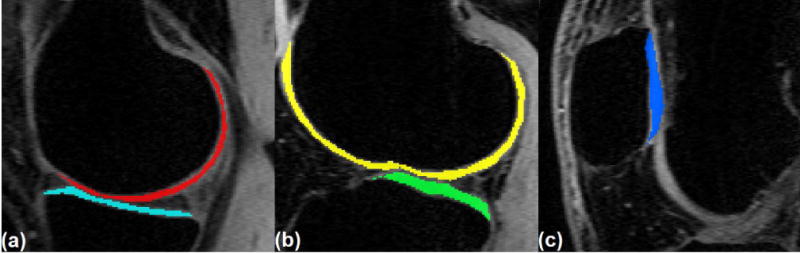
Regions of interest (ROI) for T1ρ mapping (a) medial femoral cartilage (MFC) in red, medial-tibial-cartilage (MTC) in cyan, (b) lateral-femoral cartilage (LFC) in yellow, lateral-tibial cartilage (LTC) in green, and (c) patellar cartilage (PC) in blue.

In the final biexponential fitting maps, the pixels that were not satisfied the following condition was excluded from the map³⁸.

$$4\times T_s<T_l$$ [4]

Statistical Analysis

The mean values of T_1ρ were calculated across the volunteers in each ROI. Then, a non-parametric Kruskal-Wallis test was applied to assess the significance of the difference in relaxation times between different ROIs (MTC, MFC, LTC, LFC, and PC). Moreover, Mann-Whitney U test was applied on each ROI to compare between male and female volunteers. In the statistical test, P = 0.05 was considered as the threshold to reject the null hypothesis.

Repeatability

To investigate the repeatability, the experiment was repeated on three volunteers on two different days with two weeks gap. The mean and standard deviation (SD) of T_1ρ relaxation times were calculated for each volunteer across two-session and the coefficient of variation (CV) is calculated as CV = SD/Mean to assess intra-subject repeatability.

To evaluate the inter-subject repeatability, the root mean square CV is calculated as:

$$\mathit{rmsCV}-\sqrt{({\displaystyle \sum _{i=1}^{N}C{V}_i^2})/N}$$ [5]

Where CV_i is the CV of an individual volunteer and N is the total number of volunteers (N=3).

3 RESULTS

3.1 Monte Carlo Simulations

The results of Monte Carlo simulations showed that the estimation error for short and long relaxations and their fractions decrease by increasing the number of TSLs (Figure 2.a) at the cost of longer total acquisition time. As shown in Figure 2a, the estimation error for 10 TSL points is less than 10% for all parameters and the improvement using 15 TSL points is not significant considering the fact that the total acquisition time will increase by 50%. Hence, 10 TSL points was selected for the rest of the simulations as well as in-vivo studies.

Figure 2.

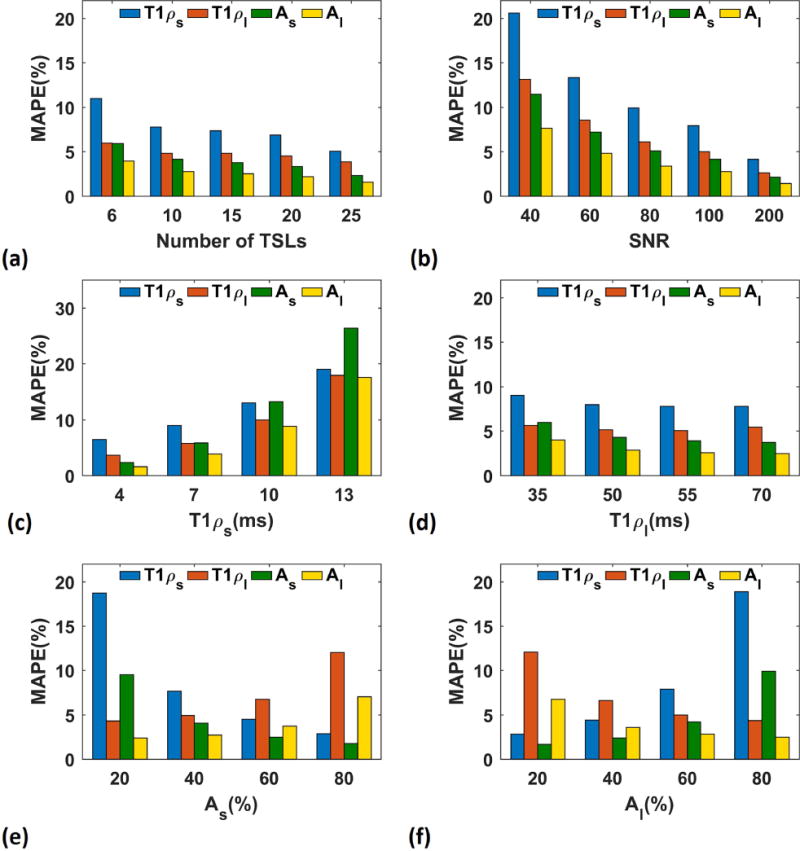
Monte Carlo simulations. Effect of different parameters on biexponential model (a) the estimation mean-absolute percentage error (MAPE) decreases by increasing the number of TSLs, (b) higher SNR leads to less estimation error, (c) the estimation is more accurate for shorter short component (T_1ρs), (d) the estimation is more accurate for larger long component (T_1ρl), and (e, f) the component with larger fraction (short component, A_s, in (e) and long component, A_l in (f)) has better estimation than the component with shorter fraction.

As shown in Figure 2.b, higher SNR leads to smaller error. The SNR of the in-vivo knee cartilage are around 60 so about 15% error for short component and less than 10% for the long component and fractions are expected in in-vivo study based on the simulation result. As the ranges of the components are different in different tissues and subjects, we investigated the effect of each parameter on the biexponential model. As shown in Figure 2.c and Figure 2.d, the error is higher for longer short components while the longer long component can be estimated more accurately. Moreover, as shown in Figure 2.e and Figure 2.f, the component with larger fraction has smaller error than the component with smaller fraction.

3.2 Ex-vivo bovine cartilage Study

Figure 3a–c shows an example of T1ρ maps of a bovine patella. Comparing the relaxation times estimated from scans with different GRAPPA acceleration factor showed that there was about 5% difference between the estimated values from fully sampled data and GRAPPA with acceleration factor of 3 (Figure3.d) while the total acquisition time decreases more than 50% from 32 to 15 minutes. Figure 3.e shows the difference between the estimation using 15 TSLs as reference and 10 and 6 TSLs. There is less than 5% difference between the estimated value using 15 and 10 TSL points which confirms our simulation study. Hence; 10 TSL points were selected as a good tradeoff between time and accuracy for in-vivo knee studies.

Figure 3.

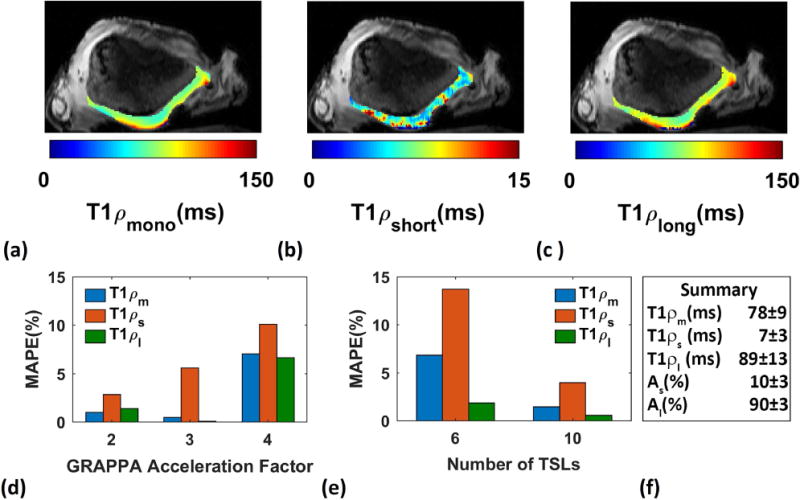
Ex-vivo study on bovine patella. (a) mono (b) short (c) long components spatial T1ρ maps, (d) the estimated parameters from scans acquired with AF 3 have less than 5% difference from the fully sampled scans, (e) the estimated relaxations using 10 TSLs has less than 5% difference from the estimated relaxations using 15 TSL points, and (f) summary of estimation.

3.3 In-vivo Knee Study

Examples of T_1ρ maps in medial, lateral and patellar cartilages as well as the locations of pixels with biexponential decay are shown in Figure 5.

Figure 5.

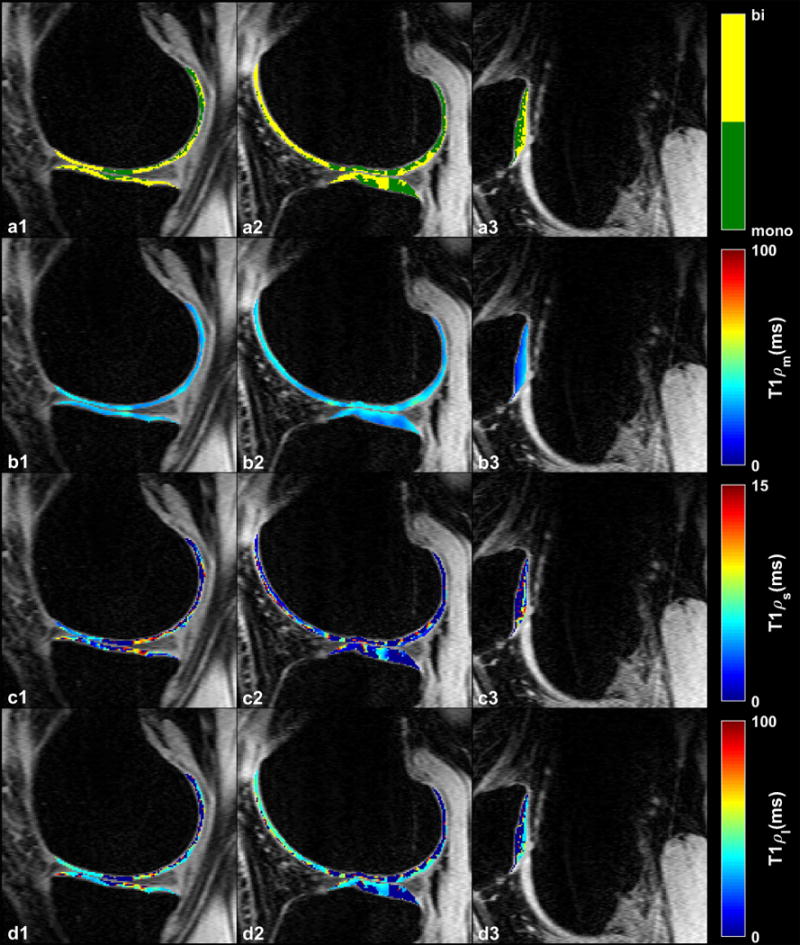
Representative examples of T1ρ maps in (a1–d1) medial (a2–d2) lateral and (a3–d3) patellar cartilages. (a1–a3) Binary maps show the distribution of the pixels with monoexponential and biexponential fitting. (b1–b3) Calculated monoexponential T1ρ map (T1_ρm). (c1–c3) Biexponential short (T1_ρs) and (d1–d3) long (T1_ρl) T1ρ maps.

T_1ρ decay in 42% of the pixels in all ROIs was biexponential. The summary of T_1ρ descriptive statistics over 8 volunteers are shown in Table 1. The short component has a lower fraction (37.6%) than the long component (62.4%). We expect that the short component is related to water tightly bound to PG and collagen macromolecules while the long component corresponds to the water loosely bound to macromolecules.

Table 1.

Summary of mean values for the monoexponential, short, and long biexponential components of T_1ρ and the ratio of biexponential pixels to a total number of pixels calculated in five ROIs.

ROI		Tmono (ms)	Tshort (ms)	Tlong (ms)	as (%)	al (%)	Ratio (%)
MTC	Mean	33.24	7.98	47.88	36.86	63.14	46
	SD	6.95	1.48	4.50	1.48	4.50	18
	R2	0.96	0.98	0.98	–	–	–
MFC	Mean	38.72	7.98	53.14	32.78	67.22	34
	SD	5.12	1.95	6.77	1.95	6.77	19
	R2	0.95	0.97	0.97	–	–	–
LTC	Mean	34.17	6.04	48.92	39.77	60.23	44
	SD	4.13	0.87	5.20	0.87	5.20	17
	R2	0.95	0.97	0.97	–	–	–
LFC	Mean	35.62	6.67	49.83	32.98	67.02	33
	SD	6.14	1.56	8.96	1.56	8.96	10
	R2	0.91	0.94	0.94	–	–	–
PC	Mean	29.39	5.53	45.27	45.63	54.37	53
	SD	6.50	1.62	8.98	1.62	8.98	17
	R2	0.95	0.96	0.96	–	–	–
Global	Mean	34.228	6.84	49.008	37.604	62.396	42
	SD	5.768	1.496	6.882	1.496	6.882	16.2
	R2	0.944	0.964	0.964	–	–	–

SD: Standard Deviation

R²: Adjusted R square shows the goodness of fit

^(R2.4.7)The deviation of data points from the straight line shows the existence of more than one exponential term. Moreover, the smaller residuals of biexponential model (Figure 6e–h) confirm that. a biexponential fit is better than monoexponential fit. ^(R2.4.7)

Figure 6.

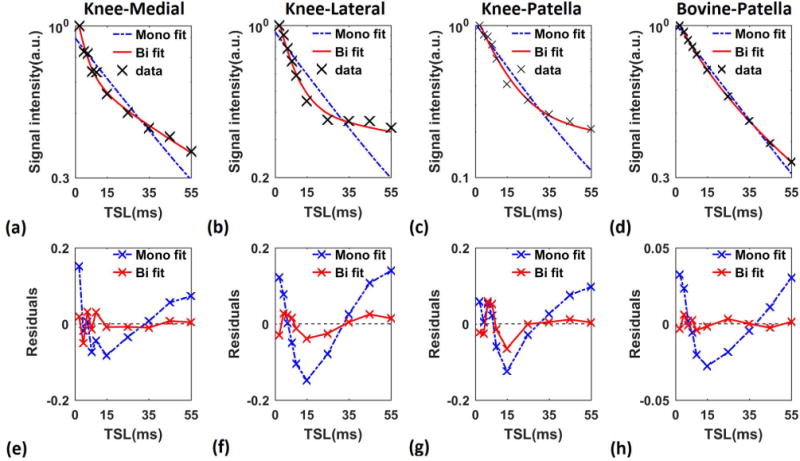
Mono- vs biexponential fitting. T1ρ decay in logarithmic scale and the fit residuals of representative pixels in (a, e) medial (b, f) lateral and (c, g) patellar cartilage of human knee and (d, h) bovine patella. The deviation of the data from the straight line (a–d) shows the existence of more than one exponential term. The smaller biexponential fit residual in comparison with mono exponential (e–h) the biexponential model fits better to the data than the monoexponential model.

Figure 7 shows the mean mono- and biexponential components of T_1ρ among all volunteers as well as male and female volunteers. The Mann–Whitney U test results showed no significant difference between male and female for each component per ROI.

Figure 7.

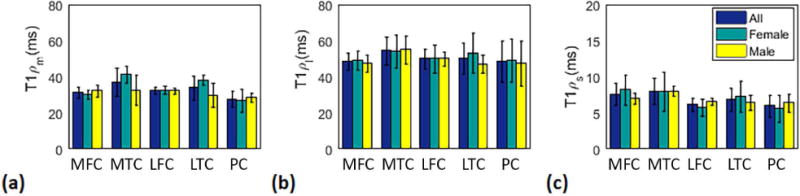
Mean estimated (a) mono (b) long and (c) short T1ρ in female (N=4), male (N=4), and all volunteers (N=8) in the medial-femoral, (MFC), medial-tibial (MTC), lateral-femoral (LFC), lateral-tibial (LTC), and patellar (PC) cartilage.

The Kruskal-Wallis test result showed a statistically significant difference (P = 0.017) in monoexponential T_1ρ between medial-tibial and patellar cartilage. No statistically significant difference was observed in biexponential components in different ROIs.

The intra-subject repeatability (i.e. repeating the experiment on the same subject) described by the coefficient of variation (CV) is shown in Table 2. The experiments showed good repeatability in the entire region (CV < 20%). No statistically significant difference was observed between CVs in different ROIs.

Table 2.

The Intra-subject repeatability.

	T1ρm			T1ρs			T1ρl			a1s			a1l
ROI	Mean (ms)	SD (ms)	CV (%)	Mean (ms)	SD (ms)	CV (%)	Mean (ms)	SD (ms)	CV (%)	Mean (ms)	SD (ms)	CV (%)	Mean (ms)	SD (ms)	CV (%)
MTC	29.5	0.7	2.4	7.4	0.3	3.8	43.0	2.8	6.6	34.5	4.9	14.3	65.5	4.9	7.6
MFC	36.5	2.1	5.8	7.2	0.7	9.8	55.5	2.1	3.8	36.0	0.0	0.0	64.0	0.0	0.0
LTC	31.5	0.7	2.2	6.7	0.4	6.3	47.0	1.4	3.0	38.5	4.9	12.9	61.5	4.9	8.0
LFC	26.0	0.0	0.0	7.3	1.6	21.3	50.5	4.9	9.8	47.0	2.8	6.0	53.0	2.8	5.3
PC	32.0	1.4	4.4	5.1	1.0	19.4	40.5	3.5	8.7	40.5	4.9	12.2	59.5	4.9	8.3

The inter-subject repeatability was measured as rmsCV (Figure 8). Long T_1ρ relaxation has less variability (mean: 7%, range: [9%-18%]) than the short T_1ρ relaxation (mean: 17%, range: [~15%-19%])), probably because the short component is more sensitive to change and also has larger potential differences between volunteers³⁹

Figure 8.

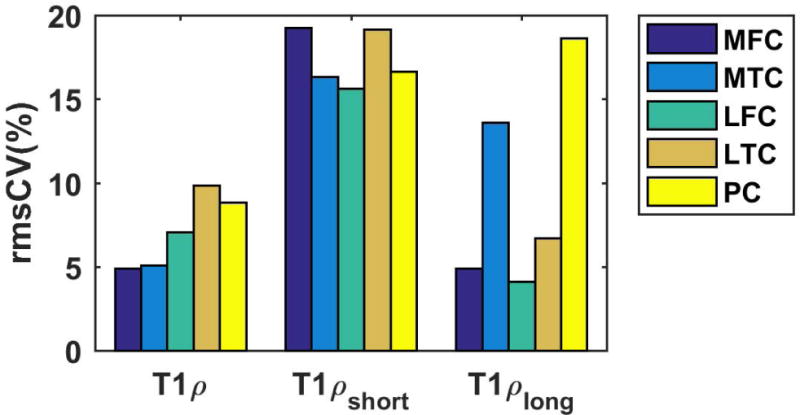
Inter-subject repeatability. The root mean square coefficient of variation (rmsCV) is less than 20% in all the ROIs.

The highest rmsCV of monoexponential T_1ρ was observed in lateral-tibial (~10%) cartilage, while the highest rmsCV of short and long components were in medial-femoral (~19) and patellar cartilage (~18) respectively.

4 DISCUSSION

In this paper, we present a 3T MRI technique for in-vivo, bi-component T_1ρ analysis of articular cartilage. Five ROIs were defined in the articular cartilage of knee joints and T_1ρ were measured in each ROI. The results showed that biexponential fitting may better present and distinguish the different relaxation times in the cartilage due to different water compartments associated with PG and collagen macromolecules.

As demonstrated by Redfield⁴⁰, in the presence of a spin-lock RF field, the spins will interact with different internal Hamiltonian such as chemical shift, J-coupling, chemical exchange, and dipole-dipole interaction^40–41. In general, the interaction can be classified into three categories (1) scalar coupling, (2) dipole–dipole, and (3) chemical exchange processes. Depending upon the tissue environment, several mechanisms may contribute differently to the T1ρ relaxation. This contribution, R_1ρ (1/ T_1ρ), can be expressed as⁴¹:

$$R_{1\rho }(f_1)=R_{1\rho }^{FD}(f_1)+R_{1\rho }^{\mathit{Diff}}(f_1)+R_{1\rho }^{Ex}(f_1)+R_{1\rho }^{\mathit{RDI}}(f_1)$$ [6]

Where R_1ρ^FD is the dipolar relaxation due to molecular rotational processes (fluctuating dipolar fields), R_1ρ^Diff is the diffusion contribution, R_1ρ^Ex is related to exchange processes associated with water protons and other exchangeable protons on macromolecules, and R_1ρ^RDI is the contribution due to residual static dipolar coupling⁴¹.

In the cartilage, T_1ρ relaxation is predominately affected by spin exchange-mediated relaxation due to glycosaminoglycans (GAG) (e.g. −OH and −NH); residual dipolar interaction of water molecules associated with collagen and it is influenced by the orientation of cartilage⁴¹

The estimated monoexponential T_1ρ range in this study was comparable^(R2.4.8) to the other studies^42–45. Some of the previous studies reported a higher value of T_1ρ^{8,11,15,42,46} .The difference is probably due to the partial volume effects (PVE) and limited temporal points. The smaller slice thickness (2mm) in our experiments leads to less PVE and as a result more accurate estimation of relaxation times in comparison with the other studies with a slice thickness of 3mm or 4mm. Moreover, we mapped the relaxation times using 10 data points (TSL), in contrast to other studies which used only 4 or 5 data points^42–44. Hence, the lower slice thickness and more temporal points of our study result in more accurate T_1ρ estimation. However, the total acquisition time in our experiment is higher than previous works since we acquired more data points. We plan to reduce the total acquisition time by employing compressed sensing^47,48 in addition to parallel imaging in the future.

To the best of our knowledge, biexponential T_1ρ measurement has only been done on tissue specimens or animal models^23,49. For example, a biexponential analysis of T_1ρ in rat muscles was reported by Yuan et al⁴⁹. The mono, short and long T_1ρ were measured as (~30 – 33 ms), (~9–11ms) and (~37 – 41ms), respectively. The short and long fractions were (~12–20%) and (~80–88%). 25 temporal points from TSL = 1ms to 60 ms were acquired in this study at the cost of increasing the total acquisition time to ~30 minutes⁴⁹. Our T_1ρ estimations using 10 TSLs acquired in 15 minutes scans are in good agreement with this study considering the difference between knee articular cartilage and rat muscle tissue⁴⁹ The cartilage extracellular matrix is mainly composed of water (~75%), type II collagen (~20%) and PG (~5%), while the rat skeletal musclemainly consists of both intra and extracellular water (~75%), different types of proteins (~20%, Myosin, Actin, Tropomyosin/Troponin, Myoglobin etc) and other components (~5%, salts, phosphates, ions, glycogen and macronutrients). The difference in chemical compositions may be attributed to differences in relaxation times and corresponding fractions between rat skeletal muscle and articular cartilage ^(R2.1).

The short component is thought to be related to the water tightly bound to PG and collagen macromolecules while the long component corresponds to water loosely bound to macromolecules. Further investigation is warranted for evaluation of sensitivity and specificity of bicomponent T_1ρ for cartilage GAG in different disease models (Knee OA and cartilage repair).

Li et al.⁴⁵ investigated the repeatability of T_1ρ relaxation time measurement and the rmsCV of 4.8%–8.8% for T1ρ has been reported across all the ROIs. Their numbers are in agreement with our experiments where the rmsCV of 4.9%-9.85% was measured for monoexponential T_1ρ. Mosher et al⁹. investigated the reproducibility of measuring T_1ρ in healthy control group and osteoarthritis (OA) patients (T_1ρ rmsCV: 5.74%-13.58% in healthy volunteers). Similar results were also observed in our study. The good agreement was also observed if we compare the rmsCV in different ROIs. Note that the reproducibility was evaluated in Mosher study by acquiring the data from different sites while we investigated the repeatability by acquiring all the data on the same scanner.

The T_1ρ imaging sequences are SAR intensive due to the application of long spin-lock pulse^50,51. SAR increases as square of RF pulse flip angle⁴¹ which is related to amplitude and duration of the spin-lock pulse. Longer TR is needed for satisfying the SAR limitation. In our proposed method, the long TR (1500ms) used for T₁ recovery also eliminates the SAR Problem. Real time SAR monitoring during the in-vivo scans showed that it was always below the limit.

There were a number of limitations to our study. We had a small number of healthy volunteers.^(R2.4.9) Moreover, biexponential condition of 4×T_1ρs < T_1ρl may produce some bias. We chose this condition based on the suggestion by Juras et. al³⁸ and confirmed it with our own experiments.

Field inhomogeneities may also produce some complications since the T_1ρ imaging is very sensitive to B₀ and B₁ inhomogeneities. To compensate this effect, we used the refocusing pulse (B₀ inhomogeneity compensation) and paired spin-lock pulse with phase alteration (B₁ inhomogeneity compensation) in T_1ρ module. In addition, the manual shimming was required before acquiring the scans.

Additionally, the magic angle effect can influence T_1ρ value due to the dipolar interactions of fiber orientation with respect to B₀. As shown by Henkelman et al.⁵², in bovine articular cartilage, the biexponential behavior disappeared when the tissue’s orientation to B₀ is about 55°. To diminish the magic angle effect, the spin-lock frequency of at least 1–2 kHz is required⁵³ which is not feasible for an in-vivo study. Moreover, at the high spin-lock frequency, as reported in an ex-vivo study²³ only the monoexponential behavior can be observed. Hence, we chose a 500Hz spin-lock frequency as a good trade-off between reducing the influence of residual dipole interactions and observing the biexponential behavior.

Finally, only asymptomatic volunteers were scanned in this study. We plan to further investigate the biexponential T_1ρ relaxation times in OA patients.

5 CONCLUSION

We have demonstrated the feasibility of biexponential T_1ρ analysis of human cartilage in-vivo on a 3T MRI scanner with clinically feasible scan times. Our preliminary results suggest that biexponential T_1ρ components could potentially be used to increase the specificity to detect early osteoarthritis by measuring different water compartments and their fractions. No statistically significant difference was observed in biexponential components in different ROIs and between genders^(R2.3).

Abbreviations

AF

Acceleration factor

CV

Coefficient of variation

ECM

Extracellular matrix

FOV

Field of view

FSE

Fast spin echo

GAG

Glycosaminoglycans

GRAPPA

Generalized auto-calibrating partial parallel acquisition

LFC

Lateral femoral cartilage

LTC

Lateral tibia cartilage

MAPE

Mean absolute percentage error

MTC

Medial tibial cartilage

MFC

Medial femoral cartilage

OA

Osteoarthritis

PBS

Phosphate-buffered saline

PC

Patellar cartilage

PG

Proteoglycan

PVE

Partial volume effects

ROI

Region of interest

R²

Adjusted R square

SPGR

Spoiled gradient echo

SD

Standard deviation

S(TSL)

Signal intensity at different spin-lock durations

TSL

Spin-lock pulse duration