I_h interacts with somato-dendritic structure to determine frequency response to weak alternating electric field stimulation

Abstract

Transcranial current stimulation (tCS) modulates brain dynamics using weak electric fields. Given the pathological changes in brain network oscillations in neurological and psychiatric illnesses, using alternating electric field waveforms that engage rhythmic activity has been proposed as a targeted, network-level treatment approach. Previous studies have investigated the effects of electric fields at the neuronal level. However, the biophysical basis of the cellular response to electric fields has remained limited. Here, we characterized the frequency-dependent response of different compartments in a layer V pyramidal neuron to exogenous electric fields to dissect the relative contributions of voltage-gated ion channels and neuronal morphology. Hyperpolarization-activated cation current (I_h) in the distal dendrites was the primary ionic mechanism shaping the model’s response to electric field stimulation and caused subthreshold resonance in the tuft at 20 ± 4 Hz. In contrast, subthreshold I_h-mediated resonance in response to local sinusoidal current injection was present in all model compartments at 11 ± 2 Hz. The frequencies of both resonance responses were modulated by I_h conductance density. We found that the difference in resonance frequency between the two stimulation types can be explained by the fact that exogenous electric fields simultaneously polarize the membrane potentials at the distal ends of the neuron (relative to field direction) in opposite directions. Our results highlight the role of I_h in shaping the cellular response to electric field stimulation and suggest that the common model of tCS as a weak somatic current injection fails to capture the cellular effects of electric field stimulation.

NEW & NOTEWORTHY Modulation of cortical oscillation by brain stimulation serves as a tool to understand the causal role of network oscillations in behavior and is a potential treatment modality that engages impaired network oscillations in disorders of the central nervous system. To develop targeted stimulation paradigms, cellular-level effects must be understood. We demonstrate that hyperpolarization-activated cation current (I_h) and cell morphology cooperatively shape the response to applied alternating electric fields.

INTRODUCTION

In transcranial current stimulation (tCS), weak electric fields generated by currents applied through scalp electrodes are used to modulate endogenous brain dynamics in animals (Chan et al. 1988; Chan and Nicholson 1986; Deans et al. 2007; Fröhlich and McCormick 2010; Jackson et al. 2016; Ozen et al. 2010; Reato et al. 2013; Schmidt et al. 2017) and humans (Helfrich et al. 2014; Herrmann et al. 2013; Kasten and Herrmann 2017; Vossen et al. 2015; Zaehle et al. 2010). Transcranial direct current stimulation (tDCS) generates static electric fields, whereas transcranial alternating current stimulation (tACS) is used to apply oscillatory electric fields. These techniques are presently under investigation for potential therapeutic use in depression, schizophrenia, and other neurological and psychiatric disorders (Berlim et al. 2013; Gluckman et al. 1996; Gluckman et al. 2001; Johnson et al. 2013; Kuo et al. 2014; Ngernyam et al. 2013; Vosskuhl et al. 2015).

Previous studies have examined the effects of both tDCS and tACS at multiple spatial scales. At the network level, tDCS increases cortical excitability (Dougherty et al. 2014; Nitsche and Paulus 2000), and tACS modulates network activity through population synchronization, state-dependent modulation of oscillations, and resonance (Alagapan et al. 2016; Ali et al. 2013; Berzhanskaya et al. 2013; Fröhlich and McCormick 2010; Park et al. 2005; Reato et al. 2013; Reato et al. 2010). At a cellular level, the neuronal response to tDCS has been thoroughly investigated, including characterization of membrane polarization, biphasic modulation of excitability, and long-term synaptic effects (Arlotti et al. 2012; Bikson et al. 2004; Chan et al. 1988; Chan and Nicholson 1986; Lafon et al. 2017; Rahman et al. 2015; Rahman et al. 2013; Yi et al. 2014). In general, the membrane polarization at a specific subcellular location in response to spatially and temporally uniform electric fields depends on the proximity to the terminals of the cells (Arlotti et al. 2012; Bikson et al. 2004, 2013; Chan et al. 1988; Chan and Nicholson 1986; Rahman et al. 2013, 2015). Cell morphology plays an important role in neuronal response to external electric fields (Arlotti et al. 2012; Chan and Nicholson 1986; Radman et al. 2009; Tranchina and Nicholson 1986). The main targets of tCS are likely layer V pyramidal neurons due to their distinct somato-dendritic axis; the effect (measured by membrane potential deflection) of an applied electric field linearly scales with the length of the elongated apical dendritic tree (Joucla and Yvert 2009; Radman et al. 2009; Rahman et al. 2015). Despite previous work describing changes in neuronal membrane voltage in response to alternating electric fields (Cavarretta et al. 2014; Deans et al. 2007; Migliore et al. 2017), the biophysical mechanisms shaping the frequency response remain unknown.

To address this gap, we used a published multicompartmental model of a mammalian neocortical L5 pyramidal neuron (Larkum et al. 2009) to investigate the cellular effects of weak oscillatory electric fields, such as those used in tACS. We focused on the frequency dependence of the subthreshold response to applied electric fields and its underlying biophysical mechanisms.

MATERIALS AND METHODS

Multicompartmental model.

The NEURON simulation environment, accessed through its Python interface, was used for all simulations (Hines and Carnevale 2006). We adapted a published biologically and morphologically realistic model of a rat neocortical layer V pyramidal neuron (ModelDB a.n. 124043, cell 070603c2) (Larkum et al. 2009) for our simulations. This multicompartmental model featured 116 apical dendrites and 70 basal dendrites modeled with 11 segments per compartment for a maximum segment length of 10 µm. Temperature was set to 34°C in the model, and the simulations were run with a time step of 0.1 ms.

The original model distinguished “trunk,” “hot zone,” and “tuft” regions of the apical dendritic tree based on their different biophysical properties, especially the distribution of ionic conductances. We refer to these groupings in our results. Per Larkum et al. 2009, most of the apical dendrites were assigned to the three regions as follows; dendrites between 0 and 500 µm from the soma were designated part of the trunk and dendrites >500 µm away from the soma (location of apical nexus) were designated part of the tuft. Within the tuft, dendrites between 500 and 800 µm from the soma were also designated part of the hot zone due to their high concentration of calcium conductances.

The model featured passive and active ionic conductances, including: Hodgkin-Huxley-style sodium and potassium channels, calcium-dependent potassium channels, fast-inactivating potassium channels, slow noninactivating potassium channels, voltage-dependent calcium channels, and hyperpolarization-activated cation current (I_h). I_h in the model was designed according to Magee 1998 for distal dendrites and distributed in the tuft region, with a uniform conductance density of 10 pS/µm² and a reversal potential of −34 mV.

Electric field stimulation.

Extracellular electric fields were applied to the model by procedurally updating the external electric potential of each segment using the NEURON extracellular mechanism at each time point (Arlotti et al. 2012; Cavarretta et al. 2014; Migliore et al. 2017). Fields were designed according to the “quasi-uniform” assumption (Bikson et al. 2013) such that the extracellular potential V_ext(x,y,z) at a given compartment was a simple function of the spatial position $\overrightarrow{x}\left(x,y,z\right)$ along the direction of the time-dependent applied field ${\overrightarrow{E}}_{\text{ext}}$:

$$V_{\text{ext}}\left(x,y,z,t\right)=-\overrightarrow{x}\left(x,y,z\right)·{\overrightarrow{E}}_{\text{ext}}\left(t\right)\mathrm{.}$$

Static and oscillatory electric fields were introduced with fixed and sinusoidal waveforms, respectively. For most simulations, field strength was limited to 5 mV/mm peak to peak such that the response of the model remained subthreshold. This is within the lower limit of values used in in vitro experiments (Bikson et al. 2004; Chan and Nicholson 1986; Lafon et al. 2017) but larger than those used in vivo (Huang et al. 2017). A wide range (1–1,000 Hz) of frequencies was applied to fully characterize high-frequency cellular response. The orientation angle of the field is reported as degrees from the somato-dendritic axis; this axis was visually estimated and shown to be aligned to the y-axis in Figs. 1, 2, and 4. Static fields were applied for 1,000 ms, whereas oscillatory fields were applied for the largest integer multiple of the stimulation period ≤5,000 ms. Sinusoidal current injections were simulated using the IClamp mechanism in NEURON. Current amplitudes were limited to 200 pA, and injection durations were determined in the same fashion as for oscillatory fields.

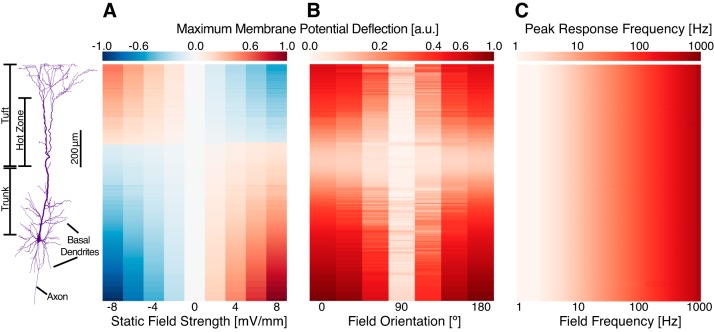
Fig. 1.

Cellular effects of electric fields. A: a static electric field, oriented in parallel to the somato-dendritic axis of the model, induced membrane polarizations in each segment dependent on field strength and spatial location. Results are unity-normalized. B: oscillatory field stimulations maximally polarized the model when oriented in parallel to the somato-dendritic axis (0 and 180°). Select compartments, such as basal dendrites extending perpendicular to the somato-dendritic axis, were maximally stimulated when the field was oriented perpendicular to the axis. Results are unity-normalized. C: the peak response frequency of membrane potential oscillations followed the frequency of stimulation in all compartments.

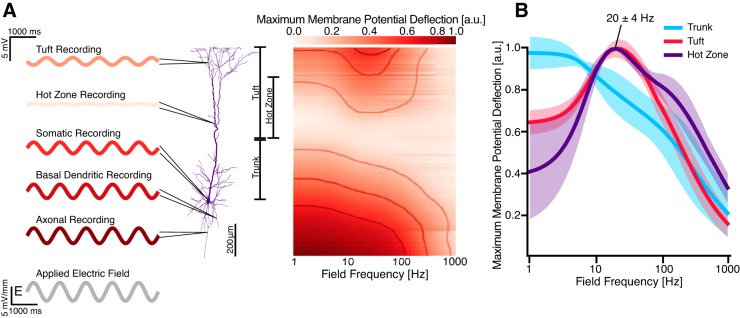
Fig. 2.

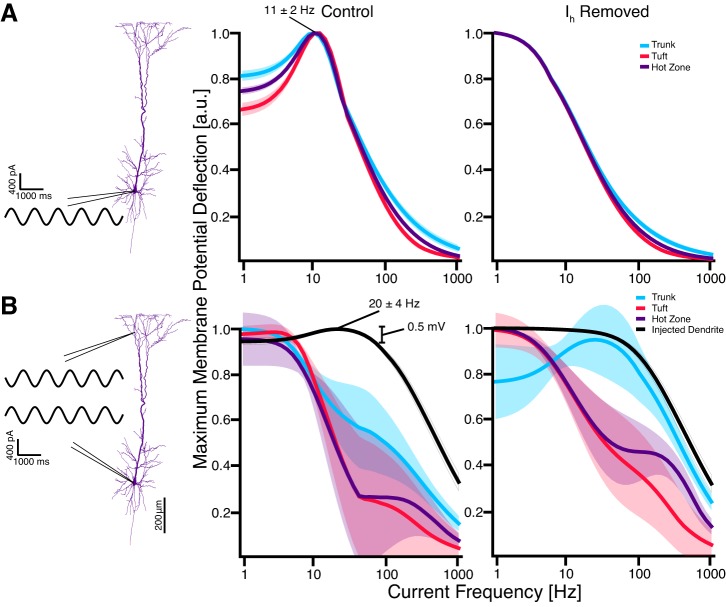
Frequency-dependent subthreshold response to oscillatory electric fields. A: sinusoidal electric fields with frequencies ranging from 1 to 1,000 Hz were applied to the neuron along the somato-dendritic axis, and the normalized maximum membrane potential deflection was determined and color-coded at each modeled segment for each frequency. Example waveforms show voltage traces for selected compartments for 1-Hz stimulation. The basal dendrites, soma, and axon of the model exhibited a low-pass response to the stimulus; the apical tuft and hot zone exhibited a band-pass response. Actual measured membrane polarizations were on the order of 0.0–2.0 mV; results were converted to arbitrary units via unity normalization. B: aggregated membrane potential deflections in the apical dendritic tree. Subthreshold resonance centered at 20 ± 4 Hz occurred in the apical tuft and hot zone. At resonance, membrane polarization in the tuft and hot zone is elevated by 0.3–0.5 mV. Data are plotted as means ± SD for each group. Compartment recordings are individually unity normalized to highlight filtering features.

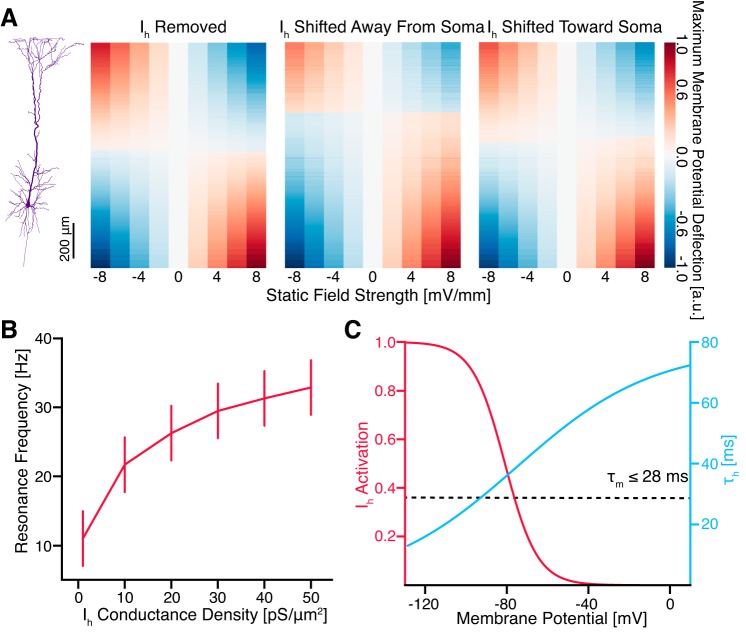
Fig. 4.

Manipulation of I_h conductance density altered cellular response to applied fields. A: removal of I_h abolished the asymmetric response of the neuron to static electric fields such that minimal membrane polarization occurred halfway between the axonal and apical ends of the model (left). Limiting I_h to compartments >850 µm from the soma likewise shifted the minimal membrane polarization point away from the soma (middle), whereas insertion of I_h in all compartments >100 µm shifted this point toward the soma (right). I_h conductance density was maintained at a uniform 10 pS/µm² in each case. Results are unity normalized. B: increasing I_h conductance density increased the resonance frequency observed in the tuft and hot zone. Uncertainties are estimated by the greater of the standard deviation of observed resonance frequencies in each compartment and the spacing of field frequencies simulated. C: the activation kinetics of the modeled I_h explain the results in A and B. Specifically, changes in membrane potential near rest (approximately −60 mV) alter I_h activation to oppose the change. The membrane time constant of the model is <28 ms, mediating the high-pass filtering of I_h.

Data analysis.

Results were analyzed with custom code written in Python. Membrane potential deflections were calculated by subtracting the mean resting value of the membrane potential of each segment in the absence of stimulation from the corresponding voltage traces under stimulation. For static field stimulations, the magnitude and sign of the membrane potential deflection was recorded; for oscillatory field stimulations, only magnitude was recorded. We estimated the power spectrum of individual voltage traces using Welch’s method, recording the peak response frequency as the maximum-power frequency.

Membrane potential deflections are reported primarily using a simple normalization scheme to aid feature extraction. For simulations using a static electric field, membrane potential deflections were unity-normalized such that mean values ranged from −1 to 1; for oscillatory electric fields, membrane potential deflections were unity-normalized such that mean values ranged from 0 to 1. To compare filtering properties of multiple compartments in the apical dendritic tree, membrane potential deflections for each compartment were individually unity-normalized as described above. This was done to avoid confusion over the response magnitude of individual compartments, as the magnitude of membrane polarization can vary significantly at different locations that otherwise exhibit similar filter responses. Membrane polarizations never exceeded 10 mV and, for most simulations, were on the order of 0.0–2.0 mV.

When reporting resonance frequencies, we estimated uncertainties as the greater of either the standard deviation of the peak frequency or the spacing of stimulation frequencies we examined in the simulations. The latter was frequently larger. To help visualize the response of the entire model to different stimulus paradigms, we present the results as a heat map, with the x-axis corresponding to the relevant stimulus parameter, the y-axis corresponding to the spatial location of a given compartment along the somato-dendritic axis, and the color of the rectangle corresponding to the relevant response feature. Rows have uneven heights because the compartments are not spaced evenly; notably, the axonal compartments have a wide spacing, whereas the portion of this plot corresponding to the tuft is quite dense. Separation of compartments was achievable because no compartments share the exact same coordinate along the somato-dendritic axis.

RESULTS

Static electric fields.

We validated our implementation of electric field stimulation by comparison with previously reported results. For the case of stimulation by a static electric field, we observed an asymmetric biphasic modulation of membrane potential (Fig. 1A), consistent with previous observations of dipole formation in neurons exposed to electric fields; for an electric field oriented along the somato-dendritic axis, hyperpolarization of the basal region was accompanied by a depolarization of the apical region and vice versa (Arlotti et al. 2012; Chan et al. 1988; Lafon et al. 2017; Radman et al. 2009; Rahman et al. 2015; Rahman et al. 2013). We confirmed that the magnitude of membrane polarization at a given compartment was linearly correlated to field strength. Compartmental polarizability was 0.3–0.6 mV per mV/mm for a static field, which was at the upper limit of in vitro observations for cortical neurons in rats (Bikson et al. 2004; Deans et al. 2007; Radman et al. 2009).

Oscillatory electric fields.

We applied an oscillatory electric field with a frequency of 10 Hz to the model at various angles of incidence (Fig. 1B) and showed that, consistent with previous in vitro and modeling results (Bikson et al. 2004; Radman et al. 2009; Rahman et al. 2015; Tranchina and Nicholson 1986), maximal membrane potential effects were observed when the electric field was oriented parallel to the somato-dendritic axis. Compartments oriented away from the somato-dendritic axis were maximally stimulated at non-zero orientations (Fig. 1B).

We were also interested in the ability of different compartments to follow changes in electric fields, especially for higher frequency stimulations, so we examined the peak frequency of membrane potential fluctuations in each compartment under a wide range of stimulation frequencies. We found that external electric fields induced membrane voltage oscillations with the same frequency as the stimulation across all compartments in the model for all stimulation frequencies tested (Fig. 1C).

Frequency-dependent responses.

To quantify the frequency-dependent response of the model, we measured the maximum membrane potential deflection at each compartment from its corresponding baseline under application of fields of varying frequency (Fig. 2A). As in previous studies, the amplitude of membrane potential deflections at most compartments increased with proximity to the two ends of the somatodendritic axis (Arlotti et al. 2012; Rahman et al. 2015; Tranchina and Nicholson 1986). With the exception of compartments in the apical tuft, all compartments exhibited a low-pass behavior, responding maximally to low-frequency stimulation. In the apical tree, however, some compartments demonstrated a band-pass response at 20 ± 4 Hz. Separating the compartments into the previously prescribed groupings revealed that this band-pass response was isolated to the tuft (which includes the hot zone) of the apical tree (Fig. 2B).

I_h-mediated subthreshold resonance.

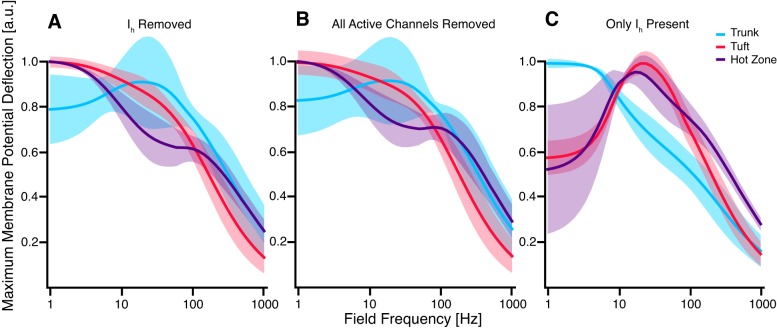
I_h contributes to subthreshold resonance in the theta range (4–10 Hz) in response to local current injections and synaptic inputs (Engel et al. 2008; Hu et al. 2002; Hutcheon et al. 1996; Hutcheon and Yarom 2000; Leung and Yu 1998; Rotstein 2015; Schmidt et al. 2017; Ulrich 2002). To test whether I_h explains the band-pass response we observed, we removed I_h from the model. As a result, subthreshold resonance in both tuft and hot zone dendrites was abolished (Fig. 3A). Removing the remainder of the active ionic mechanisms without altering the passive leak channels in the model did not cause a substantial change of the frequency response of the model (Fig. 3B). Finally, removing all active ionic mechanisms except for I_h preserved subthreshold resonance in the tuft and hot zone (Fig. 3C). These findings indicate that I_h is both necessary and sufficient for the observed subthreshold resonance and that it is the primary ionic mechanism shaping the frequency-dependent response of the modeled cell.

Fig. 3.

Hyperpolarization-activated cation current (I_h) in tuft and hot zone dendrites mediated resonance at 20 Hz. A: upon removal of I_h from the model, apical dendrites previously exhibiting resonance at 20 ± 4 Hz showed a low-pass response. Interestingly, trunk compartments gained a slight resonance upon removal of I_h, although the resonance frequency varied widely between compartments (±50 Hz). B: a similar response was observed upon removal of all active ionic mechanisms (leaving passive leakage channels). C: reinserting I_h as the only active conductance caused subthreshold resonance at 17 ± 7 Hz for hot zone dendrites and 22 ± 4 Hz for tuft dendrites.

Manipulation of I_h distribution further altered the response of the neuron (Fig. 4). Removing I_h abolished the spatial response asymmetry of the model (Fig. 4A, left). Shifting the distribution of I_h away from (Fig. 4A, middle) or toward (Fig. 4A, right) the soma likewise shifted the point of zero polarization away from or toward the soma, respectively. Increasing I_h conductance density increased the average resonance frequency observed in the tuft and vice versa (Fig. 4B). These effects can be explained by the kinetics of the modeled I_h (Fig. 4C) and are discussed further below.

The subthreshold resonance peak in response to electric field stimulation occurred only at the hot zone and tuft of the dendrites and at a higher frequency than expected based on studies using somatic current injections to characterize the effect of I_h (Alagapan et al. 2016; Engel et al. 2008; Hu et al. 2002; Leung and Yu 1998; Ulrich 2002). To investigate whether specific properties of I_h in the model could account for this discrepancy, we injected subthreshold sine-wave currents into different compartments. We observed subthreshold resonance at 11 ± 2 Hz in all compartments in response to somatic or apical current injections (Fig. 5A, middle) and showed that this resonance was I_h dependent (Fig. 5A, right). These results demonstrate that the I_h-mediated resonance observed in both electric field stimulation and single current injection is affected differently by the distinct localizations of each stimulation type: for example, the global effect of electric field stimulation vs. the local effect of current injections. Therefore, we hypothesized that the simultaneous depolarization and hyperpolarization of the dendritic tree and soma during electric field stimulation explains the difference in peak response frequency. To test this hypothesis, we mimicked the main effect of electric field stimulation by simultaneously applying two out-of-phase current injections at the soma and a distal apical dendrite (Fig. 5B). This stimulation scheme resulted in a low-pass response in most of the apical tree and in resonance at 20 ± 4 Hz in the injected dendrite (Fig. 5B, middle). Five different distal apical dendrites distributed throughout the tuft were tested, with the same result. This resonance peak was abolished by deletion of I_h (Fig. 5B, right).

Fig. 5.

Subthreshold resonance frequency in response to local current injections was different than the response to external field stimulation. A: somatic (or apical; not shown) sinusoidal current injections showed a I_h-dependent subthreshold resonance at 11 ± 2 Hz in all compartments of the model (only apical dendrites shown). Current injection magnitude was limited to achieve similar membrane polarizations as observed under electric field stimulation. Results are individually unity normalized, as previously described. B: the addition of a second current injection of equal magnitude in a distal apical dendrite, out of phase with the first to mimic the bidirectional polarizations induced by external electric fields, induced a resonance effect in the injected apical dendrite (n = 5; left). The magnitude of this resonance (∼0.5 mV) is comparable with that observed under external electric field stimulation (0.3–0.5 mV; see Fig. 2B). This induced resonance was also I_h dependent (right). Results are individually unity normalized, as previously described.

DISCUSSION

High-pass filtering by I_h causes subthreshold resonance.

I_h functions as a high-pass filter of membrane potential fluctuations (Dickson et al. 2000; Hu et al. 2002; Hutcheon et al. 1996; Hutcheon and Yarom 2000; Lüthi and McCormick 1998; Magee 1998; McCormick and Pape 1990; Nolan et al. 2007; Rotstein 2015; Rotstein and Nadim 2014). Briefly, when the cell is hyperpolarized, I_h activates, increasing cation influx and depolarizing the cell, and when the cell is depolarized, I_h deactivates, decreasing cation influx and hyperpolarizing the cell (Fig. 4C). Then, because the I_h activation time constant is larger than the membrane time constant of the model (≤ 28 ms) for relevant subthreshold membrane potentials (Fig. 4C), I_h channels will have time to respond to and suppress the membrane potential oscillations at low frequencies (Hu et al. 2002). Combined with the intrinsic low-pass filtering of the cell membrane, I_h high-pass filtering creates subthreshold resonance that tunes neurons to specific firing frequencies (Engel et al. 2008; Hutcheon and Yarom 2000; Pike et al. 2000; Rotstein et al. 2005; Schmidt et al. 2017; Stark et al. 2013). Increasing I_h conductance density suppresses membrane fluctuations at a wider range of low frequencies, thereby increasing the resonance frequency we measured in the tuft.

The activation properties of I_h also explain its role in establishing the asymmetry of the polarization in response to an applied electric field; membrane polarizations are opposed in compartments expressing I_h and relatively unaffected elsewhere, leading to an asymmetric cellular response where basal compartments exhibit a higher polarization than apical ones containing I_h. Therefore, when I_h distribution is shifted away or toward the soma, the center of the dipole induced across the neuron is likewise shifted.

Previous studies demonstrating I_h-mediated resonance have examined local stimuli such as synaptic inputs and current injections (Hu et al. 2002; Rotstein et al. 2005; Schmidt et al. 2017; Stark et al. 2013). Here, we demonstrated that the same biophysical substrate gives rise to resonance in response to electric field stimulation, albeit with a pronounced shift in resonance frequency that results from the opposing polarization of the distal ends of the neuron.

Implications for tACS.

Our modeling results emphasize several points that must be considered when discussing the rational design of tACS paradigms. First, at the network level, tACS likely entrains endogenous network oscillations (Alagapan et al. 2016; Ali et al. 2013; Berzhanskaya et al. 2013; Fröhlich and McCormick 2010; Park et al. 2005; Reato et al. 2010, 2013). Our results demonstrate subcellular frequency tuning in layer V pyramidal cells. Given the differential functional roles of the various neuronal compartments, such differences in response properties to tACS may lead to complex modulation patterns of electric signaling within individual neurons and, therefore, the networks that they form. The implications for network-level target engagement by tACS need to be explored in future studies.

Second, in many network-level modeling studies of tACS effects, single- or two-compartment models are employed with somatic sinusoidal current injections to simulate the effects of tACS (Ali et al. 2013; Park et al. 2005). Our findings suggest that this approach may miss important interactions between ionic mechanisms and cellular morphology that determine individual neuron resonance and, therefore, network resonance.

Third, tACS at higher frequencies than standard EEG frequency bands (e.g., 140 Hz) appear to modulate cortical excitability (Moliadze et al. 2010; Siebner and Ziemann 2010). However, our results indicate that stimulation at this frequency will have a reduced cellular-level effect in all neuronal compartments. Further investigation of potential alternative mechanisms that mediate this change in excitability are warranted based on our findings.

Finally, HCN channel mutations have been implicated in certain neurological and psychiatric disorders that are proposed targets of tCS, such as epilepsy and neuropathic pain (DiFrancesco and DiFrancesco 2015; Gluckman et al. 1996, 2001; Herrmann et al. 2015; Ngernyam et al. 2013; Postea and Biel 2011; Reid et al. 2012). These mutations may change the effects of tCS on individual neurons, altering the efficacy of tCS techniques in modulating cortical dynamics in these disorders. Further study of the effects of these mutations on neuronal response to tCS will be valuable for treatment development.

Limitations.

As every scientific study, our work has limitations. First, the model used exhibited a uniform distribution of I_h in distal dendrites, consistent with previous work (Harnett et al. 2015). However, the original model does not feature the exponential increase in HCN channel density from the soma to the start of the apical tuft observed in vitro and in vivo (Harnett et al. 2015; Kole et al. 2006; Lörincz et al. 2002; Migliore and Shepherd 2005). To address this concern, we modified the model to exhibit an exponential I_h distribution according to Kole et al. 2006 and observed results consistent with our previous findings, namely, that the resonance frequency in each compartment slightly increased as I_h conductance density in that compartment increased (data not shown). This modified model response was otherwise unchanged.

Second, the model used here featured a shortened axon spanning <500 µm, much shorter than the typical length of many millimeters (Arlotti et al. 2012; Oberlaender et al. 2011). However, we doubled the length of the model’s axon and observed no change in the filtering response other than a slight increase in the magnitude of membrane potential polarization (0.1–0.3 mV; data not shown).

Third, we considered exclusively the role of ionic mechanisms and other biophysical properties intrinsic to the single neuron in determining the response to oscillatory electric field stimulation; however, synaptic modulation is an important aspect of the mechanism of action of tCS under in vitro and in vivo conditions (Bikson et al. 2013; Cavarretta et al. 2014; Huang et al. 2017; Rahman et al. 2015). Future studies should incorporate synaptic inputs, especially to investigate suprathreshold neuronal response and tACS-driven alteration of individual neuron spiking behavior.

Finally, the model we used contained only two ionic mechanisms that were significantly active in subthreshold membrane potentials: I_h in the tuft and I_m in the soma. Modifying I_m had little to no effect on our results (data not shown), but this may be due to its limited distribution in the model. Indeed, our results are limited by any approximations used in constructing the model or its ionic mechanisms. Additionally, our results do not reflect the contribution of any ionic mechanisms present in L5 pyramidal neurons in vivo that are not included in the model used.

Conclusions.

We measured the effects of static and oscillatory electric fields on a model of a rat neocortical L5 pyramidal neuron, focusing on the frequency-dependent subthreshold response. We found that I_h in the distal dendrites of the apical dendritic tree were the primary ionic mechanisms shaping cellular response to tACS. This current was necessary and sufficient for a subthreshold resonance in the apical tuft at 20 ± 4 Hz that was distinct from the subthreshold resonance at 11 ± 2 Hz caused by single somatic or apical sinusoidal current injections. We used a simple model of biphasic membrane potential modulation via two distal sinusoidal current injections to illustrate that the simultaneous stimulation of distal regions of the cell under exogenous electric fields contributes to this difference in resonance frequencies. In conclusion, our work illustrates an important limitation of the prevalent model of tACS as a modulation of solely somatic membrane voltage and emphasizes the potential of multicompartment modeling in dissecting the biophysical mechanisms of tCS.

GRANTS

This work was supported in part by the National Institute of Mental Health of the National Institutes of Health (NIH) under award nos. R01-MH-111889 and R01-MH-101547 (principal investigator: Flavio Fröhlich). The content is solely the responsibility of the authors and does not necessarily represent the official views of the NIH. This project was also supported in part by the Tom and Elizabeth Long Excellence Fund for Honors administered by Honors Carolina.

DISCLOSURES

F. Fröhlich is the founder, chief scientific officer, and majority owner of Pulvinar Neuro.

AUTHOR CONTRIBUTIONS

E.H.T., E.N., and F.F. conceived and designed research; E.H.T. performed experiments; E.H.T. and E.N. analyzed data; E.H.T., E.N., and F.F. interpreted results of experiments; E.H.T. and E.N. prepared figures; E.H.T. and E.N. drafted manuscript; E.H.T., E.N., and F.F. edited and revised manuscript; E.H.T., E.N., and F.F. approved final version of manuscript.