Significance
An inverse Leidenfrost state can happen when ambient-temperature drops are deposited on liquid nitrogen, as the bath evaporation generates a vapor film that maintains drops in levitation. Contrary to what is seen on solids, drops levitating on a cryogenic liquid exhibit counterintuitive dynamics: They are spontaneously self-propelled (and glide in straight lines) and keep levitating, even after cooling down to a temperature equal to that of the bath. This spontaneous self-propulsion in a cryogenic environment—that lasts for tens of minutes—can be seen as an efficient way to freeze and further transport biological materials (such as cells or proteins) or chemicals without contamination or risk of heat degradation.
Keywords: drops, self-propulsion, inverse Leidenfrost effect, liquid nitrogen bath
Abstract
When deposited on a hot bath, volatile drops are observed to stay in levitation: the so-called Leidenfrost effect. Here, we discuss drop dynamics in an inverse Leidenfrost situation where room-temperature drops are deposited on a liquid-nitrogen pool and levitate on a vapor film generated by evaporation of the bath. In the seconds following deposition, we observe that the droplets start to glide on the bath along a straight path, only disrupted by elastic bouncing close to the edges of the container. Initially at rest, these self-propelled drops accelerate within a few seconds and reach velocities on the order of a few centimeters per second before slowing down on a longer time scale. They remain self-propelled as long as they are sitting on the bath, even after freezing and cooling down to liquid-nitrogen temperature. We experimentally investigate the parameters that affect liquid motion and propose a model, based on the experimentally and numerically observed (stable) symmetry breaking within the vapor film that supports the drop. When the film thickness and the cooling dynamics of the drops are also modeled, the variations of the drop velocities can be accurately reproduced.
When deposited on a hot solid, volatile drops can levitate over a cushion of their own vapor—a phenomenon extensively described by J. G. Leidenfrost (1) in the 18th century. Being insulated from the substrate by a vapor layer, the Leidenfrost drops have a lifetime of the order of a few minutes (2). Moreover, in the absence of friction, they do not only glide at the slightest inclination, but also bounce (3), jump (4), or oscillate (5), rich dynamics (6) that make the control of such drops a problem. On solid substrates, addition of a well-chosen texture can efficiently guide drops, as first demonstrated by Linke et al. (7): Asymmetric textures can redirect the vapor flow below the liquid (8), which generates self-propulsion. This is used to efficiently guide or even entrap levitating drops (9–11) or solids (12). However, controlling drop motion seems more complex on deformable substrates such as liquid baths, where Leidenfrost levitation also occurs (13–17). The liquid surface, resisting the weight of the drops, is notably deformed (18, 19), but this does not impact drop mobility, as there is no contact drag (20, 21). The suspended drops were observed to sometimes glide for tens of seconds (14, 16, 17, 22, 23) and have to be trapped to perform some measurements (13).
In this work, we consider the dynamics of ethanol or silicone-oil droplets deposited on a liquid-nitrogen bath, in an “inverse” Leidenfrost scenario (24), where vapor generated by the bath maintains drops above the pool. We show that, contrary to what is seen on solid substrates, a spontaneous symmetry breaking occurs that leads to a self-propelling state—a phenomenon that we investigate experimentally. Using simulations, we demonstrate that the movements arise from a difference in the film thickness between the front and the back of the drop, which we use to model the gliding dynamics.
Experiment
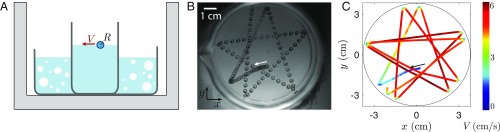
Liquid nitrogen is a cryogenic liquid with boiling temperature of −C and low latent heat of vaporization Lv = 2x105 J/kg. Its evaporation is fast enough so that, when a drop at ambient temperature approaches a nitrogen bath, the generated vapor cushion can maintain the drop in the Leidenfrost state (16, 22, 23). As opposed to more usual Leidenfrost situations (1–3) where vapor is produced by the levitating objects, here, vapor comes from the bath so that the drops keep a constant radius over time. However, the drops continuously cool down (below their freezing point), until their temperature reaches that of the bath, which sometimes causes their sinking (16, 22). To avoid ebullition within the pool, we followed Adda-Bedia et al. (16) by placing the central bath (with diameter = 7.6 cm) at the center of a sacrificial bath of liquid nitrogen, itself inside an homemade polystyrene cryostat. As schematized in Fig. 1A, the sacrificial bath is continuously boiling, which maintains a nitrogen atmosphere in the box. The residual evaporation of the central bath (at 0.1 L/h, due to radiative heat exchanges at the top) does not disturb the liquid surface that remains perfectly still. Drops of ethanol (density = 789 kg/, specific heat = 2,400 J/kgK−1 at C), or silicone oil ( = 930 kg/, = 1,600 J/kgK−1) with radii ranging from 0.65 to 1.8 mm are formed from calibrated needles and released 1 cm above the bath surface. The chosen liquids have low freezing temperatures (C), which limits their freezing in the needles. Moreover, such drops keep a smooth spherical shape when they freeze, which does not always happen for water drops (25). Once released, the drops, denser than liquid nitrogen (density = 808 kg/), initially sink, but nitrogen evaporation generates a buoyant force that almost immediately pushes them back to the surface, where they remain (22). Drop trajectory and velocity are recorded from the top at typically 125 fps, and the origin of time is chosen as soon as Leidenfrost levitation happens.
Fig. 1.
(A) Schematic of the experimental setup: A drop with radius is deposited on a liquid-nitrogen bath. To avoid ebullition of the central bath, it is placed in a styrofoam box and maintained at the center of a sacrificial bath for which evaporation maintains a nitrogen atmosphere in the box. Drop trajectory and velocity are recorded from the top. (B) Chronophotography of the successive positions (separated by 80 ms) of an ethanol drop ( = 1.5 mm) seeded with particles. The white arrow indicates the initial position and movement of ethanol. (C) Trajectory of the center of mass of the same drop in the x–y plane in a longer time interval. The color indicates the drop velocity, varying from (dark blue) to cm/s (dark red).
Fig. 1B shows the first 15 s of motion of an ethanol drop with radius = 1.5 mm seeded with particles—two successive images are separated by 80 ms. The white arrow indicates the initial position and direction of the drop. Ethanol, initially at rest, slowly accelerates and starts hovering on the bath in straight lines. This regular movement is only disturbed by almost-perfect reflections close to the edges of the beaker, producing a remarkable star-shaped trajectory. The droplet is initially subjected to strong internal motion (that can be seen in Movie S1) that vanishes as the liquid cools down and freezes (which happens between the second and the third bouncing)—with no visible impact on its movement. As is visible in Movie S1, the surface of the bath remains still as the drop hovers above it. The drop velocity , of a few centimeters per second, is small enough not to generate any stationary wake (21). In Fig. 1C, the trajectory of the drop center of mass is plotted: The position (x, y) = (0, 0) is the center of the beaker, and the black circle corresponds to the edge of the bath. The color code indicates the drop velocity . Initially 0 (dark blue), increases up to 6 cm/s (dark red) after the 4th bouncing and then slowly diminishes to reach 4 cm/s after the bouncing. Interestingly, the propulsion mechanism is not disturbed by the successive rebounds: In the first seconds, the drop keeps accelerating even after turning back close to the edge. The setting in motion of the drops is observed for every liquid tested (ethanol, silicone oil, propanol, butanol, pentanol, and water), provided the drops are small and light enough to be supported by the liquid-nitrogen bath. Depending on the first incident angle of the drop with the wall, trajectories vary from diagonals (for a perfectly normal incidence) to stars with varying numbers of branches—as in SI Appendix, Fig. S1—up to triangles, pentagons, and circles (for a tangent impact). Finally, it can be noted that self-propulsion is also seen for millimeter-sized particles (polyethylene spheres), although for a much shorter duration. Similarly to frozen drops, the solid particles do not exhibit any rotational movement while gliding (as in Movie S2).
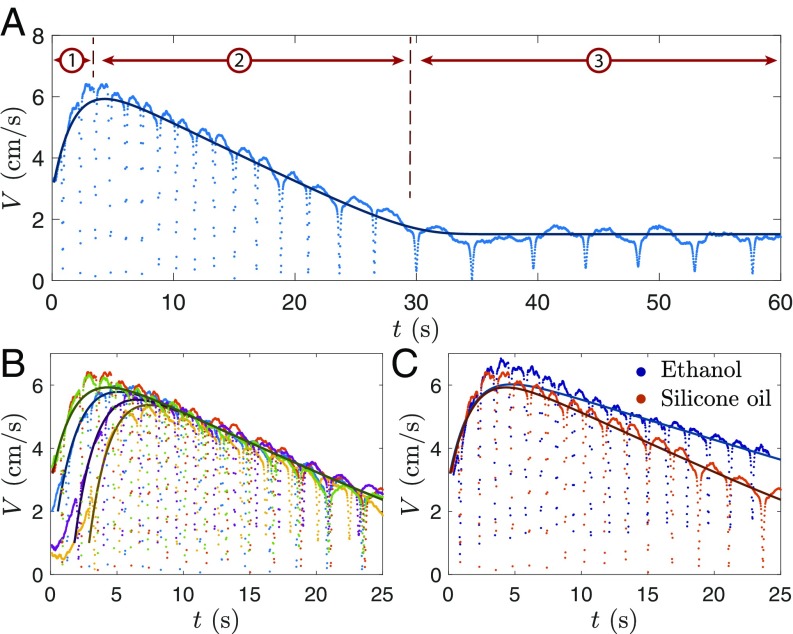
The velocity dynamics is even more intriguing. Fig. 2A shows for a silicone-oil drop ( = 1.4 mm) as it glides on the bath (see also Movie S3). After falling from the needle, the drop sinks and resurfaces with an initial velocity = 3.2 cm/s and immediately accelerates. The shape of results from the combination of two effects. First, at each rebound, the velocity decreases and rises up again to the same value—indicating elastic bouncing. The drop bounces 23 times during its 60 s lifetime: Each event can be distinguished individually in Fig. 2A. Second, exhibits very regular variations on a longer timescale (variations that are quite undisturbed by the repeated bounces) and that we call here the velocity amplitude . is highlighted by the black line (which is the numerical solution of Eq. 5) and can be decomposed in three phases, numbered on Fig. 2A: (i) An acceleration phase (for s) where the drop velocity amplitude increases from = 3.2 to 6.5 cm/s; (ii) a deceleration phase (for 5 s s) that lasts five times longer than the acceleration, and during which decreases linearly with time; and (iii) a constant velocity phase (30 s s) with cm/s. This third phase can sometimes last several minutes, until an outside event (a small movement of the liquid surface or an encounter with a floating ice crystal) makes the drop sink. The levitation time is much longer than the expected Leidenfrost duration, which is of the order of s for millimeter-sized drops initially at ambient temperature (16). It can also be noticed that drop immersion after >30 s hardly generates any boiling (Movie S4), which indicates that the particle temperature is then close to the vaporization temperature of the bath.
Fig. 2.
Drop velocity. (A) Velocity of a drop of silicone oil ( = 1.4 mm) gliding on a liquid-nitrogen bath, as a function of time . The numbers mark the three phases of movement: acceleration, deceleration, and constant velocity. The corresponding movie is Movie S3. (B) Comparison of the velocity of five identical silicone-oil drops ( = 1.4 mm) deposited slightly differently on the bath. (C) Velocity of ethanol (blue dots, specific heat = 2,400 J/kgK−1) and silicone-oil drop (orange dots, = 1,600 J/kgK−1) with similar radius ( = 1.4 mm) and initial velocity. In all images, the darker lines are the numerical solution of Eq. 5, with identical prefactors = = 15 and = 1.45 m.
Fig. 2B shows five velocity plots (colored dots) obtained by repeating the same experiment (silicone oil, = 1.4 mm) but varying the height at which the drops are deposited. If the velocity amplitudes of drops with identical initial velocity perfectly overlap (as for the green and red curves), varying the initial conditions impacts the acceleration phase. In particular, it can be noted that sometimes (yellow and purple plots), the drops do not accelerate immediately but exhibit an erratic motion at low velocity ( 1 cm/s) for the first seconds before starting to self-propel. Interestingly, this does not impact the second phase of the drop movement (the deceleration), where all plots perfectly overlap. Finally, Fig. 2C compares the velocity profiles of ethanol and silicone oil with identical radius = 1.4 mm and initial velocity. Contrary to Fig. 2B, changing the nature of the liquid affects the deceleration rate, which is significantly (30) lower for ethanol than for silicone oil. However, varying the drops’ freezing temperature or preheating them hardly influences the velocity amplitude (SI Appendix, Fig. S2). We now aim to understand and model the phenomena at the origin of the rich drop dynamics.
Origin of Self-Propulsion
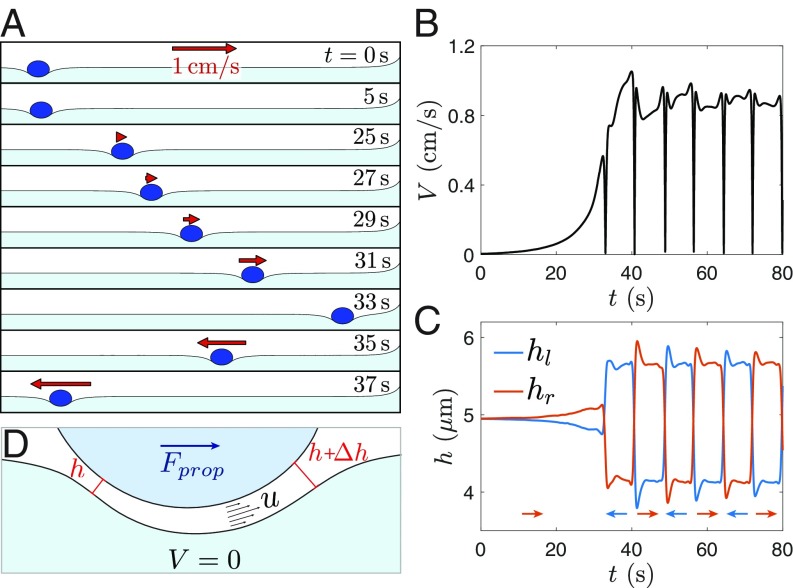
A first insight on the cause of self-propulsion is obtained through numerical simulation. Both vapor and liquid flows are calculated in a 2D model system, by using a sharp-interface finite element method. A drop is deposited at the center of a liquid-nitrogen bath, and the initial vapor film is symmetric. As is visible in Movie S5, the mesh is made very fine below the drop—to resolve the thin gas film—and coarser outside. For simplicity, thermal effects are neglected, and the bath evaporates at a constant rate of 2.15 g/. The motion of a drop with radius = 1 mm and viscosity = 16 mPas, as obtained numerically, is presented in Fig. 3A: Even if no preexisting asymmetry is imposed, the droplet spontaneously self-propels, as also is visible in Movie S6. In Fig. 3B, the drop velocity is plotted as a function of time: increases to finally reach a constant value 0.1 cm/s. Similarly to what is seen experimentally, the velocity amplitude is not significantly impacted by the repeated drop about-turns close to the edges of the bath.
Fig. 3.
(A) Successive images extracted from the 2D simulations. A drop with viscosity = 16 mPa.s and radius = 1 mm is deposited on an evaporating bath and spontaneously self-propels. The corresponding movie is Movie S6. (B) Drop velocity as a function of time . (C) Difference in film thickness between the left (, in blue) and right (, in red) parts of the drop where the film is thinner. (D) Model for the propulsion force: The film has a mean thickness , and vapor is continuously escaping with a characteristic velocity . The difference in the film thickness changes the vapor distribution, generating a viscous propelling force , directed toward the larger opening.
Beyond a mere reproduction of the self-propulsion, the simulation gives access to the details of the film thickness and its variation with time, information difficult to obtain experimentally. In Fig. 3C, the minimum film thicknesses (measured at the neck) on the left side of the drop (, in blue) and on the right side (, in red) are extracted from the simulation and plotted as a function of time. While initially, and are equal, they spontaneously diverge until a constant asymmetry m is reached. Thus, Fig. 3C gives essential indications on the origin of self-propulsion. First, comparison with Fig. 3B shows that the appearance of the asymmetry corresponds to the setting in motion of the drop. A geometrical asymmetry would indeed partially redirect the flow of vapor toward the larger opening and, thus, generate a propelling force. In addition, the film is systematically thicker at the front ( when the drop moves to the right; when it moves to the left). The drop follows the preferential direction of motion of the vapor, which indicates that the mechanism that causes self-propulsion is surely of viscous origin. Finally, it should be noted that the asymmetry spontaneously switches from left to right when the drop gets close to the liquid meniscus at the edge of the bath. While the direction of changes, its amplitude is not impacted: The same asymmetric state consistently reappears. This strongly suggests that symmetric film thickness is metastable and that self-propulsion is generated by a spontaneous and constant symmetry breaking within the vapor film.
Model
The main result of the simulation is now used to model drop dynamics. As observed in the numerics, we assume a constant asymmetry with amplitude in the film thickness (with ) between the front and the back of the drop. As illustrated in Fig. 3D, and similarly to what is seen in textured solids (7, 26), the asymmetry partially redirects the flow of vapor, which enables motion. The difference of viscous stresses between the front and the back generates a propelling force which can be estimated: is a fraction of the total viscous force exerted on the bottom of the drop, varying as (with the viscosity of nitrogen vapor and the typical velocity of the Poiseuille flow within the film), which gives . This expression is simplified by using lubrication theory: the pressure drop within the vapor film scales as , and the overpressure in the film sustains the drop, which implies for drops smaller than the capillary length (2, 16). These three expressions combined give the following propelling force:
| [1] |
which is similar to what is observed for uneven Leidenfrost solids (26). dominates at the first instants of motion, during acceleration, but as the drop velocity increases, the friction force gains importance. Our hypothesis is that its dominant contribution also comes from the film: While gliding (with velocity ), the drop entrains vapor, and its movement creates a secondary Couette flow within the film, with a mean velocity . This generates a viscous friction force that can be written as:
| [2] |
The full calculation (in a simplified situation) confirms this argument and is given in SI Appendix. The friction force (inversely proportional to the film thickness ) has the unusual property of increasing with time. Indeed, as the drop cools down, less and less nitrogen vapor is produced, and the film thins out. To fully determine , we then need to model . Calculations of the film thickness have been done with a different purpose for levitating drops on solids (2) or on hot baths (13) and in the inverse Leidenfrost state (16): We follow a similar line of arguments here.
For drops in an inverse Leidenfrost scenario, arises from two simultaneous processes: (i) vapor production and escape and (ii) drop-cooling dynamics. We give here the main physical ingredients (a detailed calculation can be found in SI Appendix). (i) Due to the temperature difference between the drop and the bath, heat diffuses through the film and vaporizes liquid nitrogen. The escaping vapor is then confined below the drop, and lubrication generates an overpressure that sustains the drop. For any given , these two elements give the following scaling law for :
| [3] |
where , , and , respectively, denote the viscosity, density, and conductivity of the vapor, the latent heat of vaporization of liquid nitrogen, drop density, and gravity. (ii) Simultaneously, due to heat diffusion through the film, the drop cools down. The rate of decrease of the drop internal energy (with the drop-specific heat) is equal to the rate at which energy diffuses through the vapor film . Combined with Eq. 3, integration of this differential equation finally gives , which is found to decrease linearly with time:
| [4] |
is the initial film thickness: For millimeter-sized drops and C, m. This is in good agreement with measurements done on solid substrates (2) or with the results of numerical calculations (13) for drops on a bath. The characteristic time arises from the drop-cooling dynamics: It is the time needed for drops to cool down from ambient temperature to liquid-nitrogen temperature. For millimeter-sized drops, this time is of the order of 20 s.
Using Eqs. 1, 2, and 4, we can finally model the dynamics of the droplet. Writing for the drop mass, Newton’s second law gives the following differential equation for the velocity amplitude :
| [5] |
with and numerical coefficients arising from geometrical factors (respectively, for and ) which are not considered in scaling laws. Since and both originate from the vapor flow within the film, we can assume that and are close. Thus, in the rest of the discussion, we will consider , and the two fitting parameters in Eq. 5 are and . The temporal dependence of the velocity amplitudes is simply deduced from Eq. 5 by using a separation of times scales. On the one hand, varies in a time 20 s, while, on the other hand, the characteristic time of the acceleration phase is 1 s. Therefore, during the acceleration phase, the film thickness remains roughly constant, and Eq. 5 can be approximated by a first-order linear differential equation. Denoting the initial drop velocity, , increases exponentially, with , until the drop reaches its terminal velocity , obtained by equalizing the propelling and friction forces (Eqs. 1 and 2):
| [6] |
On a longer time scale, film thinning affects that decreases linearly with time (as does, from Eq. 4) in a characteristic time . This model nicely reproduces the first two phases of the drop movement, as seen in Fig. 2 B and C: The darker lines are the numerical solution of Eq. 5, with the same fitting parameters = 15 and = 1.45 m. In Fig. 2B, the collapse of the plots during the deceleration phase is due to the drops reaching their terminal velocity, identical for all five experiments. However, the nature of the liquid affects the deceleration rate, as illustrated in Fig. 2C. The difference in deceleration rate between ethanol and silicone oil is mainly due to a difference in the liquid-specific heats . Ethanol drops with = 2,400 J/kg cool down in 43 s, where silicone-oil drops (with = 1,600 J/kg) cool down faster ( 30 s), which directly impacts the slope of .
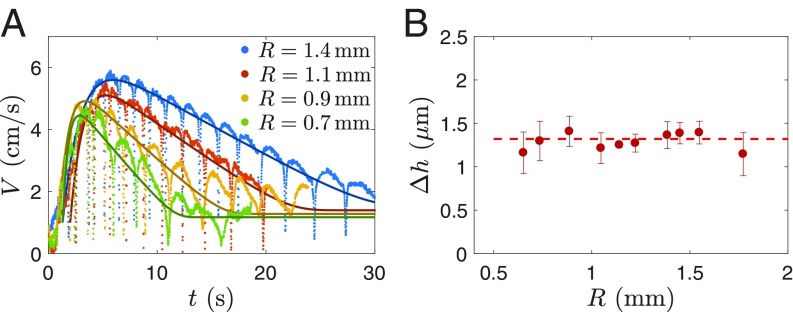
In addition, the amplitude of the asymmetry causing self-propulsion can be deduced from the velocity amplitude dynamics. Fig. 4A shows the best fit of obtained for varying drop radii : Smaller drops have lower internal energy and cool down faster, which is nicely reproduced by Eq. 5. By repeating systematically this experiment (with drop radius varied between 0.64 and 1.8 mm), we plot (in Fig. 4B) the value of giving the best fit as a function of . More specifically, we consider the second phase of drop dynamics, where drops decelerate at a constant rate (phase 2 in Fig. 2A). The experimental measurement of gives , which is expected to vary proportionally to , as calculated from Eq. 6. In Fig. 3C, is found to be of the order of 1 m, which is consistent with our initial hypothesis of a small film deformation (m) and with the result of the numerical simulation. Remarkably, remains constant over the range of drop radii we tested (0.64 mm 1.8 mm). This is quite different from what is seen for self-propelled uneven solids (26), where .
Fig. 4.
(A) Velocity of silicone-oil drops with varying drop radius . The darker lines are the solutions of Eq. 5 for each drop size. (B) Amplitude of the asymmetry deduced from the best fit of the drop deceleration.
Incidentally, Eq. 5 explains why the drop does not rotate in the stable asymmetric state: Reaching the terminal velocity, the propelling and friction forces balance, which implies—for an approximately spherical drop—that also the net torque on the drop balances, consistent with our observations.
Self-Propulsion of Pool Liquid and Frozen Drops
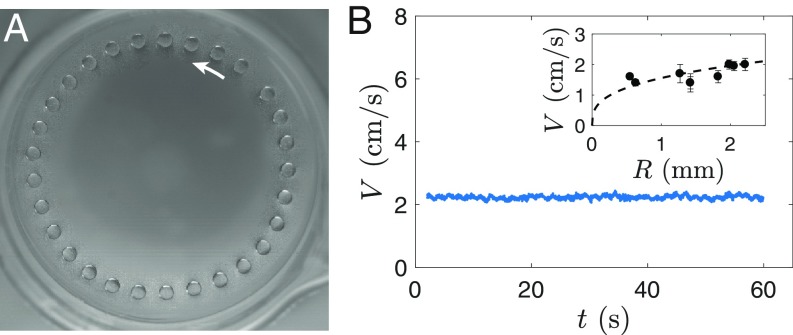
Interestingly, liquid-nitrogen drops deposited delicately on the liquid-nitrogen bath can also levitate (without coalescing) for long periods of time, even if their temperature is the same as that of the bath. Similarly to hot drops, these cryogenic drops are self-propelled: Fig. 5A shows successive positions (separated by 250 ms) of a liquid-nitrogen drop with radius mm 10 min after being deposited. As also seen in Movie S7, the drop has a regular circular trajectory. Such a trajectory is generated because the drop, released close to the edge of the beaker, is initially propelled almost tangentially to the wall. In Fig. 5B, the drop velocity is observed to remain constant, with 0.2 cm/s.
Fig. 5.
(A) Successive positions of a drop of liquid nitrogen with radius = 1.8 mm deposited close to the edge of a liquid-nitrogen bath. The images are separated by 250 ms. The arrow indicates the direction of movement of the drop. The corresponding movie is Movie S7. (B) Velocity of the liquid-nitrogen drop. stays constant, with cm/s. In B, Inset, is plotted as a function of the drop radius . The dotted line is a fit with , with as defined in Eq. 7.
Levitation of such cold objects is made possible by residual bath evaporation, happening despite the presence of an insulating box and a sacrificial bath. A 300-mL beaker with surface 57 typically empties in 3 h, which corresponds to an evaporation rate kg/s. This value is in close agreement to what is expected from radiative heat transfer, where kg/s, with the beaker diameter, the Stefan–Boltzmann constant, and and , respectively, ambient and liquid-nitrogen temperatures.
The continuous vapor production maintains a constantly renewed vapor film under the liquid-nitrogen drops, even if the droplet itself does not transfer heat to the system. Levitating is then the same as floating above a perfectly porous substrate through which gas escapes. The film thickness generated by the bath evaporation can be estimated in that situation: The vapor flux generated under the surface of a (cold) drop with radius is . This vapor is redirected within the film, so that , with the mean velocity of vapor. The pressure in the film is estimated from lubrication theory and as the vapor film sustains the drop . These expressions combined finally give:
| [7] |
The measured evaporation rates yield 10 m, which is smaller than the film thickness expected for hot drops (m), but sufficient to enable levitation. Moreover, Eq. 7 predicts , which we verified: Indeed, Eq. 6, predicts that , proportional to here, should also vary as . The velocity of liquid-nitrogen drops was measured for varying radii (Fig. 5B, Inset): The dotted line shows our model with , which fits reasonably well with our data, with a prefactor close to 1.
The same mechanism that enables noncoalescence of liquid-nitrogen drops also causes the persistence of levitation of initially hot droplets, long after they freeze to liquid-nitrogen temperatures. Indeed, from Eqs. 6 and 4, one would expect the drops to sink at the end of the deceleration phase, when the film thickness diminishes to zero. However, the bath residual evaporation—as described earlier—generates a constant vapor flux that adds up to the Leidenfrost flux. Even if this additional vapor flux is negligible in the first seconds (it is initially 100 times smaller), it becomes of critical importance as the levitating drops cool down. Indeed, it generates a 10 m-thick vapor film (as estimated from Eq. 7), which is sufficient to maintain in levitation droplets sufficiently light and smooth.
Full Model and Discussion
To also model the dynamics of drops after they completely cool down (as in Fig. 2A), we now consider the influence of the residual vapor flux on the film thickness . The calculation is provided in SI Appendix: By adding the two fluxes, the film thickness is found to be the solution of a polynomial equation: , that can be solved for any time . We solve Eq. 5 numerically by taking this last element into account: The continuous lines plotted in Figs. 2 and 4 show the velocity profiles predicted by the model, with fitting parameters = = 15 and = 1.45 m; and are calculated from Eqs. 4 and 7, respectively. As seen in Fig. 2A, the model matches all three phases of the drop movement. It also nicely reproduces the drop dynamics for varying initial conditions (Fig. 2B), liquid nature (Fig. 2C), and drop radii (Fig. 4A) without any change in the fitting parameters. This model, although simplified (it does not consider the variation of liquid properties as the droplets cool down, as well as the freezing dynamics) accounts convincingly for the details of the evolution of velocity amplitudes.
Remarkably, both experiments and numerical simulations are consistent with a stable symmetry breaking , which remains constant during the drop’s lifetime (even if the vapor flux diminishes by a factor of 100), and which does not vary with the drop size. Even if we cannot fully explain the exceptional stability of the asymmetric state, we can provide clues to its origin. In particular, the consistent motion of nondeformable objects (frozen drops or polyethylene marbles, as in Movie S2) indicates that most certainly originates from an asymmetric deformation of the liquid-nitrogen interface. What could then cause the surface of the bath to deform? A hypothesis is that the symmetry breaking is generated by an instability of the morphology of the vapor film itself, which is very different from that of classical Leidenfrost drops over a flat, rigid substrate (13, 19). In particular, a recent theoretical study (27) shows that the film exhibits localized oscillations at the neck, which can develop within the whole film for drops smaller than the capillary length. We surmise that these oscillations may be unstable, which would trigger a symmetry breaking when they are very slightly disturbed.
Conclusion
We demonstrate that drops deposited on a cold bath are naturally self-propelled, without external forcing. The complexity of drop dynamics results from the combination of three elements: (i) a stable symmetry breaking (associated with a variation of the film thickness) which causes self-propulsion; (ii) the thinning of the vapor film under the drops—due to their cooling—that increases the friction and is responsible for their deceleration; and, finally, (iii) the residual evaporation of the bath, which can cause persistent levitation long after drops freeze to the bath temperature.
An interesting parallel can be drawn with the very recent paper of Bouillant et al. (28), who showed that small drops can also exhibit spontaneous self-propulsion on flat solids. While, on solids, motion is induced by a symmetry breaking in the internal flow of the droplets, on a bath, it is most surely generated by an instability happening at the liquid-nitrogen interface. This difference fundamentally affects the behavior of the levitating objects: First, solid marbles can self-propel here, and they glide without rotation. In addition, the propelling force switches direction and instantly reappears after the drops have been reflected from a wall. This can be used to control droplets’ trajectories with very fine precision, by confining them between two walls. We can finally note that spontaneous motion is not solely limited to cryogenic baths: Liquid-nitrogen drops can also self-propel on an ethanol bath (as in Movie S8). This might increase the scope of such a study to ambient-temperature situations.
Materials and Methods
Homemade Cryostat.
The cryostat is a box of expanded polystyrene, with dimensions 30 × 30 × 25 cm and 4-cm-thick walls. Inside is placed a sacrificial bath (a beaker with diameter of 19 cm filled with 5 cm of liquid nitrogen). At its center, another beaker, with diameter D = 7.6 cm, is placed on a copper disk and filled with 10 cm of liquid nitrogen. The cryostat is closed by a polystyrene lid, which is removed for each experiment and then replaced.
Drops Tracking.
A homemade Python algorithm is used: It automatically extracts the (x, y) position of the drop center from an initial frame with known drop position and size. Bilateral filtering and median-estimated background subtraction are first applied. Then, at each step, the drop position is estimated (from the previously tracked position and speed), and the image is cropped around it. A Gaussian-blurred circle is drawn separately, and its center and radius are optimized through brute-force search to minimize its mean-squared error with the cropped image. This gives the drop location and radius with pixel precision.
Numerical Method.
The numerical simulation is based on a finite element method of the incompressible 2D Cartesian Navier–Stokes equations with sharp interfaces aligned with the mesh (see SI Appendix for more details). The 2D simulation domain has a size of 77 × 45 mm. The liquid surface is placed at a height of 20 mm, with a contact angle of 20° with respect to the walls. The implementation is done by using the framework oomph-lib. (29).
Supplementary Material
Acknowledgments
We thank Dominic Vella for insightful comments on the model; Corentin Tregouet for his initial and fruitful theoretical input; and Guillaume Lajoinie for carefully reading the manuscript.
Footnotes
The authors declare no conflict of interest.
This article is a PNAS Direct Submission.
This article contains supporting information online at www.pnas.org/lookup/suppl/doi:10.1073/pnas.1812288116/-/DCSupplemental.
References
- 1.Leidenfrost J. De Aquae Communis Nonnullis Qualitatibus Tractatus. Ovenius; Duisburg, Germany: 1756. [Google Scholar]
- 2.Biance A-L, Clanet C, Quéré D. Leidenfrost drops. Phys Fluids. 2003;15:1632–1637. [Google Scholar]
- 3.Tran T, Staat H, Prosperetti A, Sun C, Lohse D. Drop impact on superheated surfaces. Phys Rev Lett. 2012;108:036101. doi: 10.1103/PhysRevLett.108.036101. [DOI] [PubMed] [Google Scholar]
- 4.Celestini F, Frisch T, Pomeau Y. Take-off of small Leidenfrost droplets. Phys Rev Lett. 2012;109:034501. doi: 10.1103/PhysRevLett.109.034501. [DOI] [PubMed] [Google Scholar]
- 5.Brunet P, Snoeijer J. Star-drops formed by periodic excitation of an air cushion: A short review. Eur Phys J Spec Top. 2011;192:207–226. [Google Scholar]
- 6.Quéré D. Leidenfrost dynamics. Annu Rev Fluid Mech. 2013;45:197–215. [Google Scholar]
- 7.Linke H, et al. Self-propelled Leidenfrost droplets. Phys Rev Lett. 2006;96:154502. doi: 10.1103/PhysRevLett.96.154502. [DOI] [PubMed] [Google Scholar]
- 8.Dupeux G, et al. Viscous mechanism for Leidenfrost propulsion on a ratchet. Europhys Lett. 2011;96:58001. [Google Scholar]
- 9.Cousins T, Goldstein R, Jaworski J, Pesci A. A ratchet trap for Leidenfrost drops. J Fluid Mech. 2012;696:215–227. [Google Scholar]
- 10.Marín ÁG, et al. Capillary droplets on Leidenfrost micro-ratchets. Phys Fluids. 2012;24:122001. [Google Scholar]
- 11.Soto D, Lagubeau G, Clanet C, Quéré D. Surfing on a herringbone. Phys Rev Fluids. 2016;1:013902. [Google Scholar]
- 12.Hashmi A, et al. Leidenfrost levitation: Beyond droplets. Sci Rep. 2012;2:797. doi: 10.1038/srep00797. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 13.Maquet L, et al. Leidenfrost drops on a heated liquid pool. Phys Rev Fluids. 2016;1:053902. [Google Scholar]
- 14.Snezhko A, Ben Jacob E, Aranson I. Pulsating-gliding transition in the dynamics of levitating liquid nitrogen droplets. New J Phys. 2008;10:043034. [Google Scholar]
- 15.Kim H, Lee Y, Cho H. Levitation time measurement of water drops on the surface of liquid nitrogen. J Korean Phys Soc. 2011;58:1628–1632. [Google Scholar]
- 16.Adda-Bedia M, et al. Inverse Leidenfrost effect: Levitating drops on liquid nitrogen. Langmuir. 2016;32:4179–4188. doi: 10.1021/acs.langmuir.6b00574. [DOI] [PubMed] [Google Scholar]
- 17.Janssens S, Koizumi S, Fried E. Behavior of self-propelled acetone droplets in a Leidenfrost state on liquid substrates. Phys Fluids. 2017;29:032103. [Google Scholar]
- 18.Vella D. Floating versus sinking. Annu Rev Fluid Mech. 2015;47:115–135. [Google Scholar]
- 19.Wong C, Adda-Bedia M, Vella D. Non-wetting drops at liquid interfaces: From liquid marbles to Leidenfrost drops. Soft Matter. 2017;13:5250–5260. doi: 10.1039/c7sm00990a. [DOI] [PubMed] [Google Scholar]
- 20.Vakarelski I, Marston J, Chan D, Thoroddsen S. Drag reduction by Leidenfrost vapor layers. Phys Rev Lett. 2011;106:214501. doi: 10.1103/PhysRevLett.106.214501. [DOI] [PubMed] [Google Scholar]
- 21.Le Merrer M, Clanet C, Quéré D, Raphaël É, Chevy F. Wave drag on floating bodies. Proc Natl Acad Sci USA. 2011;108:15064–15068. doi: 10.1073/pnas.1106662108. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 22.Song Y, et al. Vitrification and levitation of a liquid droplet on liquid nitrogen. Proc Natl Acad Sci USA. 2010;107:4596–4600. doi: 10.1073/pnas.0914059107. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 23.Feng H, Xu Y, Yang T. Study on Leidenfrost effect of cryoprotectant droplets on liquid nitrogen with IR imaging technology and non isothermal crystallization kinetics model. Int J Heat Mass Transfer. 2018;127:413–421. [Google Scholar]
- 24.Hall RS, et al. Inverse Leidenfrost phenomenon. Nature. 1969;224:266–267. [Google Scholar]
- 25.Wildeman S, Sterl S, Sun C, Lohse D. Fast dynamics of water droplets freezing from the outside in. Phys Rev Lett. 2017;118:084101. doi: 10.1103/PhysRevLett.118.084101. [DOI] [PubMed] [Google Scholar]
- 26.Dupeux G. Self-propelling uneven Leidenfrost solids. Phys Fluids. 2013;25:051704. [Google Scholar]
- 27.van Limbeek M, Sobac B, Rednikov A, Colinet P, Snoeijer J. 2018. Asymptotic theory for a Leidenfrost drop on a liquid pool. arXiv: 1805.12003.
- 28.Bouillant A, et al. Leidenfrost wheels. Nat Phys. 2018;14:1188–1192. [Google Scholar]
- 29.Heil M, Hazel AL. oomph-lib—an object-oriented multi-physics finite-element library. Fluid Structure Interaction. 2006;53:19–49. [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.