Common ivy ensures water supply of the crown by well-balanced within-plant adjustments of hydraulic efficiency and safety. Patterns correspond to variations in xylem anatomy and are similar to those of self-supporting trees.
Keywords: Climber, drought resistance, embolism, leaf dimorphism, osmotic adaptation, plant water relations, xylem anatomy
Abstract
Lianas form long and flexible but disproportionately narrow stems, and thus require particular strategies to maintain the integrity of xylem water transport and ensure supply to large crown areas. The hydraulic architecture of lianas and the respective within-plant coordination of transport efficiency and safety, and the underlying anatomical variations in xylem, are largely unexplored. We analysed Hedera helix, a liana widespread in European temperate forests, with respect to hydraulic and xylem anatomical variations between the main stem and branches, between juvenile and adult life phases, and along the vertical axis. Main stems were significantly less embolism resistant but exhibited a higher hydraulic conductivity than branches. In branches, the cell turgor loss point of leaves decreased, while the embolism resistance and conductivity of xylem, as well as conduit diameters, increased with height. High water-transport capacities allow ivy to compensate for the small cross-section of stems, while the limited resistance to drought-induced xylem dysfunction of the main stem is probably linked to conservative stomatal regulation. Pronounced differences in xylem anatomy, hydraulic efficiency, and safety between the main stem and branches and along the vertical axis are surprisingly similar to those of self-supporting plants, and indicate that the coordination of carbon and water economies requires similar internal adjustments in tall plants.
Introduction
Lianas and other climbing plants are characterized by large crown areas supported by long and flexible but disproportionately narrow main stems (Schnitzer and Bongers, 2002; Wyka et al., 2013; Rosell and Olson, 2014). The discrepancy between leaf area and supporting stem cross-sectional area has consequences for both static and hydraulic aspects (Ewers, 1985). On the one hand, the mechanical function of the stem, and thus resource allocation to the xylem, can be reduced, as climbers are not self-supporting but rely on neighbouring trees or other structures to support their weight. Water transport and regulation, on the other hand, have to be optimized with regard to capacity and safety by a hydraulic architecture adapted to this growth form (Ewers and Fisher, 1991).
Lianas apparently show strong selection for conductive efficiency and exhibit higher specific conductivities and higher sap flux compared with angiosperm and gymnosperm trees (Ewers, 1985; Chen et al., 2017; Ichihashi et al., 2017). Comparisons of western poison oak plants growing as vines or as shrubs (Gartner, 1991), and of Bauhinia species with different growth forms (Ewers and Fisher, 1991), indicate that individuals with narrow vine stems are able to achieve the same leaf specific conductivity as individuals growing as shrubs with wider stems. Higher hydraulic efficiency is thought to be based on the formation of wider vessels in the xylem. Extremely wide vessels can be found in lianas from tropical rainforests (Gartner et al., 1990; Ewers and Fisher, 1991), but also in woody climbers from temperate regions (Tibbetts and Ewers, 2000). However, Rosell and Olson (2014) pointed out that climbing plants exhibit a higher variance in vessel diameter, rather than a higher mean vessel diameter, and Olson et al. (2014) showed that, for a given stem length, non-self-supporting plants have only slightly higher mean hydraulic vessel diameters than self-supporting plants. Tall plants need to optimize biomass allocation and xylem investment to guarantee efficient and safe long-distance water transport (Petit et al., 2016). Hydraulic optimality models suggest that vessel diameters therefore increase from stem tips toward the stem base following a power law (Anfodillo et al., 2006; Olson et al., 2014). This axial conduit widening can substantially buffer the effect of increasing path length on the cumulative hydrodynamic resistance (West et al., 1999; Mencuccini et al., 2007).
In contrast to their increased hydraulic efficiency, lianas were found to be less tolerant to drought-induced cavitation than co-occurring trees (Van der Sande et al., 2013; Chen et al., 2017). However, the combination of wide and efficient vessels together with embolism-resistant narrow vessels and tracheids provides a small but sufficient transport capacity during dry periods. This pronounced vessel dimorphism is highly characteristic of lianas and can be interpreted as a strategy to combine high hydraulic efficiency with sufficient hydraulic safety (Carlquist, 1985). Trees, in contrast, rarely achieve both high efficiency and high safety (Gleason et al., 2016). Moreover, several hypotheses for the abundant occurrence of starch-rich parenchyma in woody climbers exist, especially with respect to producing positive pressure and providing water and osmotic agents to enable repair mechanisms (Cochard et al., 1994; Tibbetts and Ewers, 2000; Chen et al., 2017). Many lianas also show a very sensitive stomatal response to increasing vapour pressure deficits during the day (Leuzinger et al., 2011; Chen et al., 2017).
Woody climbers are a widespread growth form in tropical rainforests, but also influence the dynamics and function of temperate forests by affecting species composition, tree regeneration, and water dynamics (Schnitzer and Bongers, 2002; Castagneri et al., 2013; Ichihashi et al., 2017). One of the most widespread lianas in temperate European forests is common ivy (Hedera helix L., Araliaceae). Common ivy climbs with anchoring roots on the host tree, supported by stable, upright elements, forms foliated branches from the base to the top, and can reach a height of up to 30 m (Metcalfe, 2005). The species is tolerant to a wide variety of substrates, but grows most vigorously in shaded, moist sites. It is limited in its range by low winter temperatures (Andergassen and Bauer, 2002) and may profit in the future from rising temperatures (Walther, 2002; Heuzé et al., 2009; Rehm et al., 2014) and elevated CO2 concentrations (Zotz et al., 2006). Ivy is a classical example of a heterophyllous plant, forming a juvenile and an adult phenotype depending on the plant height and light conditions. For instance, ivy plants growing on sun-exposed sites produce juvenile shoots up to a height of ~1.2 m and adult shoots above this height. The juvenile stage has vertically flattened branches with three- or five-lobed leaves and faster shoot growth but fewer leaves; in the adult stage, shorter, flowering shoots with numerous spirally arranged entire leaves are generated (Miller and Goodin, 1976; Metcalfe, 2005). The two phenotypes also differ in their photosynthetic capacity, stomatal and residual conductance leaf thickness, and number of chloroplasts (Bauer and Bauer, 1980; Hoflacher and Bauer, 1982), as well as frost resistance and timing of frost hardening (Andergassen and Bauer, 2002; Rehm et al., 2014).
Studies on H. helix are interesting from a physiological point of view for a variety of reasons. First, it is a widespread representative of lianas and thus offers general insights into structure–function relationships of non-self-supporting plants. Being a temperate liana, it can also be compared with numerous comparably well-studied temperate host trees. Second, ivy is a prominent example of heterophylly by forming two different phenotypes with leaves adapted to low- and high-light conditions. Third, this structural parasite is known to affect temperate forests, as it intensively interacts and competes with host trees for light and water. The hydraulic architecture of ivy and other temperate lianas has received little attention. The only available study on H. helix (Leuzinger et al. 2011) examined water relations, and reported surprisingly constant leaf water potential and sap flow across different environmental conditions, coupled with sensitive stomatal regulation. The authors assumed that the conservative flow control may have evolved as an adaptation to the potentially high vulnerability of the xylem to drought-induced embolism, and that potentially low transport resistances ensure moderate water potentials under transpiration, for example, at low vapour pressure deficits; however, this has not yet been confirmed.
In the present study, we analysed water transport efficiency and safety, and the underlying xylem anatomy and leaf cell hydraulic parameters, in H. helix. We expected this liana to exhibit overall high hydraulic efficiency but low hydraulic safety. Furthermore, we hypothesized that within-plant hydraulic patterns are affected by the demands of the climbing habit and the reduced mechanical function of the xylem, and thus differ from the hydraulic architecture of self-supporting trees.
Materials and methods
Study site and plant material
The study was conducted in the Botanical Garden of the University of Innsbruck, Austria (47.267°N, 11.393°E; 574 m above sea level), with an average annual precipitation of 896.5 mm and an average air temperature of 8.5 °C in the 30-year mean (ZAMG, 2002). Ivy plants with a growth height of about 15 m and stem diameters up to 9 cm were selected; these plants were climbing on an oak (Quercus robur), a pine (Pinus sylvestris), and the institute building. Branches (0.7–1 m long) were sampled by the use of telescopic scissors and the help of tree climbers at heights of 0–1 m (juvenile branches) and 1–2 m, 7–8 m, and 12–13 m (adult branches). Samples were immediately re-cut several times under water and rehydrated for at least 12 hours (Beikircher and Mayr, 2016). After branch sampling, stem sections were sampled in a similar fashion with a motor saw at 1–2 m and 7–8 m height from the main stems and then re-cut under water with a sharp carving knife. All measurements were made during the summer months between April and November.
Pressure–volume analysis
Leaf hydraulic parameters, including osmotic potential at saturation (Ψo), turgor loss point (Ψtlp), and modulus of elasticity (ε), were analysed via pressure–volume analyses (Tyree and Hammel, 1972) on five fully developed leaves of different plants, from each sampling height. Leaves were saturated before dehydration was applied and repeated measurements of Ψ and weight were made. Ψ was measured with a pressure chamber (model 1000 ‘upgraded to 100 bar’ pressure chamber; PMS Instrument, Albany, OR, USA). The relative water content was calculated from saturated, fresh, and dry weights and plotted against the inverse leaf Ψ (1/Ψ). The turgescent section was fitted with a parabolic function and the osmotic section with a linear regression according to Boyle’s law using Fig.P 2006 (Fig.P Software Inc., Hamilton, ON, Canada) to determine Ψtlp (the intercept of linear and parabolic function), Ψo (the negative inverse of the linear graph portion’s intercept with the y-axis), and ε (the slope of the curve above the turgor loss point; Ganthaler and Mayr, 2015). Parameters were calculated individually per leaf and then averaged per sampling height.
Hydraulic conductivity
Branch sections (10 per sampling height, up to 12 cm long and 6 mm in diameter) were excised under water, at least 10 cm distant from the basal branch end. Sample ends were re-cut several times by ~5 mm with a sharp wood-carving knife, decorticated, and sealed in the hydraulic measuring system. Stem sections (five per sampling height, up to 35 cm long and 40 mm in diameter) were treated likewise with a carving knife and connected to the measuring system with a rubber adaptor.
The flow rate was determined with a flow meter (mini Cori-Flow 100 g h−1, Bronkhorst High Tech, Ruurlo, The Netherlands) at a pressure of 5 kPa using distilled, filtered (0.22 µm), and degassed water containing 0.005% (v/v) Micropur (Katadyn Products, Kemptthal, Switzerland) to prevent microbial growth. Samples were repeatedly flushed at 70 kPa for 15 min to ensure that native embolism was removed and measurements represented maximum conductance. The specific hydraulic conductivity (ks; m2 s−1 Pa−1) was calculated as
where Q is the volume flow rate (expressed in m3 s−1), l is the length of the sample (in m), Ac is the xylem cross-sectional area (in m2), and ΔP is the pressure difference between the segment ends (in Pa). Calculations were corrected to 20 °C to account for changes in fluid viscosity with temperature.
Vulnerability analysis
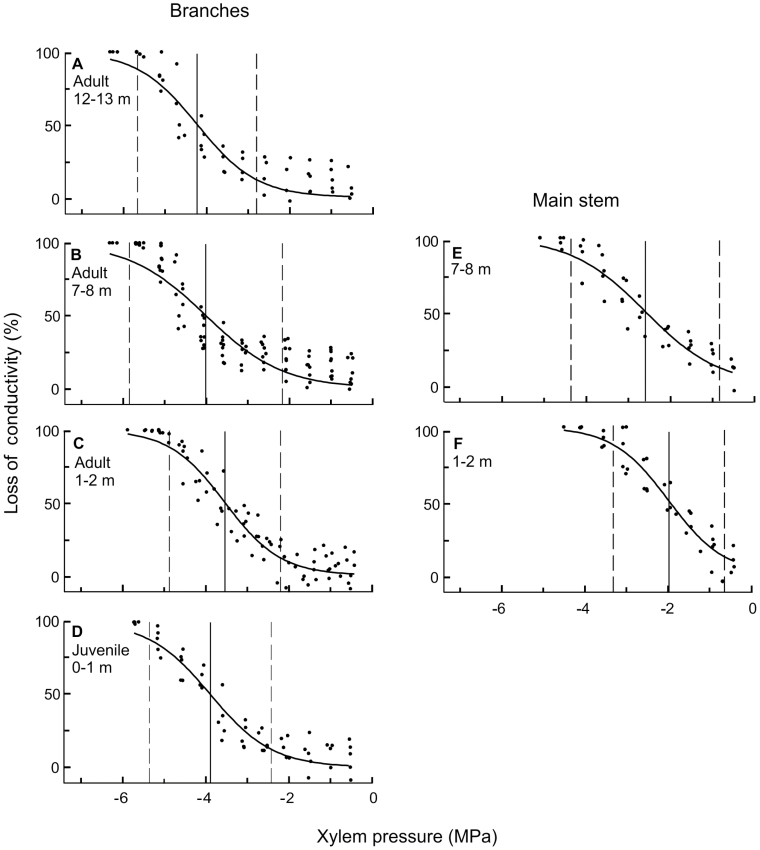
Vulnerability curves were analysed on five to nine samples of stems and branches from each height by using the cavitron technique (Cochard et al., 2005). This method uses centrifugal force to increase the water tension in a xylem segment while simultaneously measuring the loss of conductance. Fully hydrated branch and stem segments of 275 mm length were fixed in a custom-built 280 mm rotor in the centrifuge (Sorvall RC-5 Superspeed Refrigerated Centrifuge, Thermo Fisher Scientific, Waltham, MA, USA). Sample ends were positioned in upstream and downstream reservoirs, which were filled with distilled, filtered (0.22 μm), and degassed water containing 0.005% (v/v) Micropur. The temperature was set to 10 °C. Before the first measurement of hydraulic conductance, samples were equilibrated for 20 min at low rotating speed (inducing –0.25 MPa). Then, the rotational speed was increased stepwise to the next target pressure and hydraulic conductance was measured after an equilibration time of 1 min. The moving water meniscus in the upstream cuvette was observed using a high-resolution camera (Motic MC 2000, Motic China Group Co., Ltd) and the flow rate and hydraulic conductance were calculated. Percentage loss of conductivity (PLC) was calculated from the ratio of actual (after inducing a given Ψ) to the maximum (first measurement at −0.25 MPa) hydraulic conductance (Beikircher et al., 2010).
Curves of each sample were fitted with an exponential sigmoidal equation according to Pammenter and Vander Willigen (1998) as
where a is a constant related to the curve slope and ΨLC50 is the xylem Ψ at 50% loss of conductivity. Fitting of the curves was performed with Fig.P 2006 (Fig.P Software Inc., Hamilton, ON, Canada). We also calculated Ψ at 12% and 88% PLC (ΨLC12, ΨLC88) and averaged the results per sampling height.
Segments of the main stem had to be split lengthwise to fit into the cavitron rotor. For this, sticks of 2–3 cm diameter and 30 cm length were axially split from the main stem. This was performed under water with a carving knife, following the fibre structure of the wood and thus minimizing the number of open vessels on the lateral sites. We thus expected potential shifts in the vulnerability curve to be minor (open vessels would be emptied and thus not included in the analysis, potentially causing an underestimation of the vulnerability). An artefact due to the sample length can be excluded as the maximum conduit length was 73.2±3.3 mm in adult branches, 79.8±2.7 mm in juvenile branches, and 109.8±3.8 mm in the main stem (analysed by forcing compressed air through five branch and stem segments, respectively; Ewers and Fisher, 1989; Beikircher and Mayr, 2016). Vessels were thus distinctly shorter than the rotor diameter (280 mm) and could not be opened at both ends by sample preparation. Accordingly, the sigmoidal shape of the vulnerability curves from the stem and branches of H. helix also indicated that open vessel artefacts were not relevant.
Wood anatomical measurements
Branch and stem sections previously used for hydraulic conductivity measurements were soaked in ethanol/glycerol/water solution (1:1:1, v/v/v) for at least 2 weeks. Cross-sections (8 µm thickness) were cut with a microtome (Schlittenmikrotom G.S.L. 1, Schenkung Dapples, Zürich, Switzerland), stained with Etzold solution (fuchsin/chrysoidin/Astra blue), and analysed with a light microscope (Olympus BX41; Olympus Austria, Wien, Austria) interfaced with a digital camera (ProgRes CT3, Jenoptik, Jena, Germany). In randomly selected radial sectors, areas of all conduits (68–174 per sample) within the outermost two growth rings were analysed with the image analysis software ImageJ 1.45 (National Institutes of Health, MD, USA). The diameters were calculated from conduit areas, assuming a circular shape, averaged per sample, and the mean diameter (dmean) per species was calculated from these values. The average hydraulic conduit diameter (dh) was calculated from the diameter of all analysed conduits Kolb and Sperry (1999) as
To characterize conduit wall reinforcement, the wall thickness to span ratio (t/b)2 (Hacke and Sperry, 2001) was assessed. The thickness of tangential interconduit walls (t) and the conduit diameter (b) were measured for conduit pairs with average diameters within dh ±1 µm (five conduit pairs per sample). The values were averaged per sample, and the mean (t/b)2 per sampling point was calculated from these values. In addition, the percentage of xylem area covered by conduit lumen (arealumen) and the percentage of the cross-sectional area occupied by the central pith parenchyma (areapith) was measured.
Annual growth
On the main stem of three individual ivy plants, discs were taken at 1.5 m and 7.5 m above ground for determination of age and measurement of annual ring width. Measurements were made of each disc and results were averaged per sampling height.
Statistical analysis
Differences were tested with Student’s t-test after testing for homogeneity of variance (Levene test) and for Gaussian distribution (Kolmogorov–Smirnov test). Correlation analyses were carried out using Pearson product-moment correlation and were based on all individual measurement values (Fig. 1) or the mean values of branches and main stems from different sampling heights (Fig. 4). In the second case, the standard error was included in statistical tests as a weighting factor. All tests (two-tailed) were performed pairwise at a probability level of 5% using SPSS version 24 (IBM Corporation, Armonk, NY, USA). All values are reported as mean ±SE.
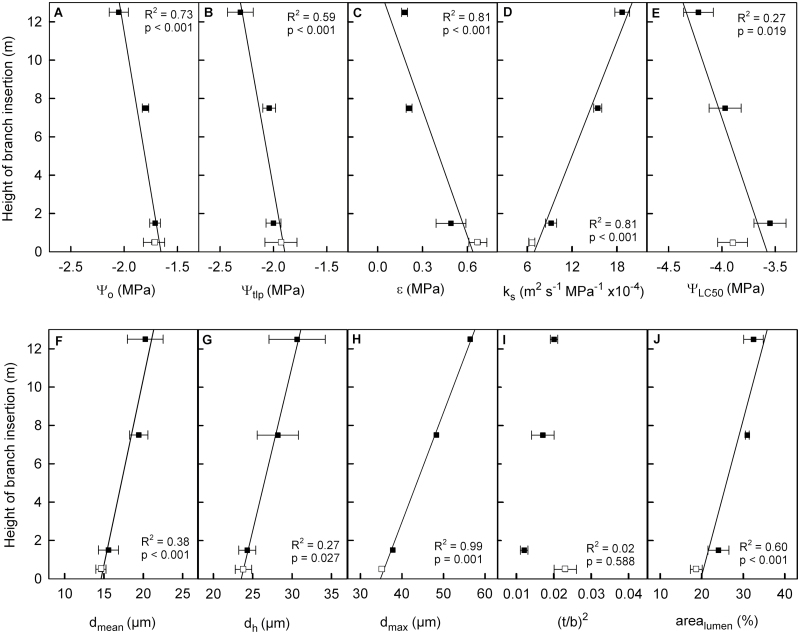
Fig. 1.
Correlation of hydraulic and anatomical parameters with sampling height. (A) Leaf osmotic potential at full turgor (Ψo), (B) cell turgor loss point (Ψtlp), (C) modulus of elasticity (ε), (D) specific hydraulic conductivity (ks), (E) water potential at 50% loss of conductivity (ΨLC50), (F) mean conduit diameter (dmean), (G) mean hydraulic diameter (dh), (H) maximum conduit diameter (dmax), (I) cell wall thickness to span ratio [(t/b)2], and (J) percentage of xylem area covered by conduit lumen (arealumen) of juvenile branches (empty squares) and adult branches (filled squares). Data presented are means ±SE.
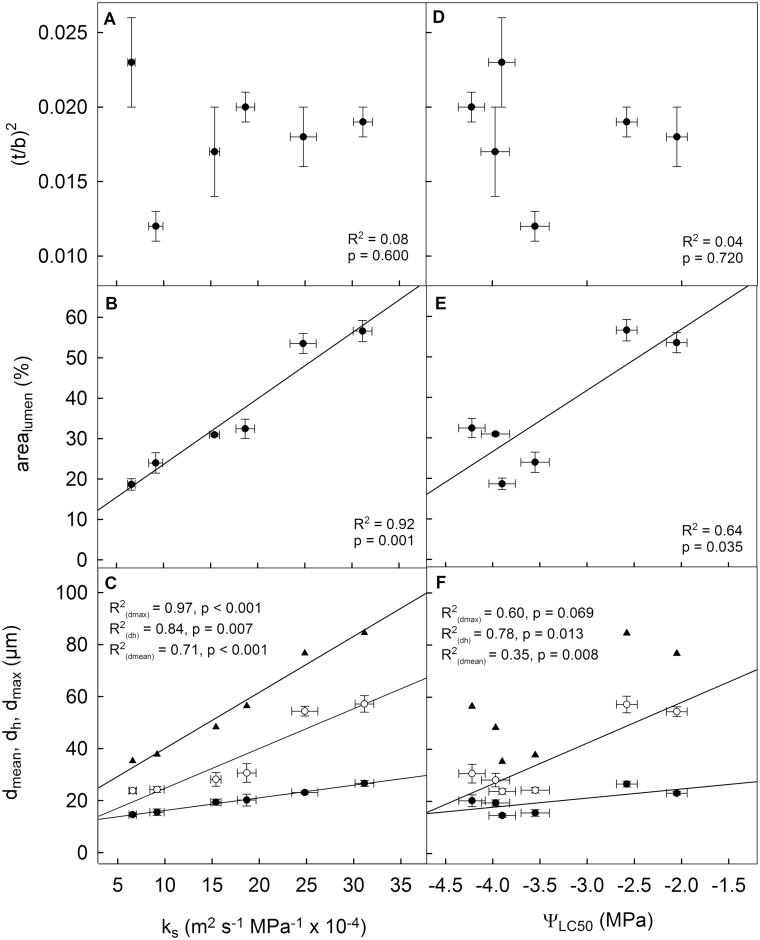
Fig. 4.
Correlation of wood anatomical parameters with specific hydraulic conductivity (ks) and vulnerability to cavitation (water potential at 50% loss of conductivity; ΨLC50). (A, D) Cell wall reinforcement [(t/b)2], (B, E) percentage of xylem area covered by conduit lumen (arealumen), and (C, F) mean conduit diameter (dmean; filled circles), mean hydraulic diameter (dh; open circles), and maximum diameter (dmax; triangles). Data presented are means ±SE of juvenile branches, adult branches, and the main stem from different sampling heights.
Results
Pressure–volume analysis
At the lowest sampling heights, Ψo of leaves was least negative (approximately –1.7 MPa) and similar in juvenile and adult leaves. It decreased with height to –2.05 MPa at 12–13 m (Table 1). Ψtlp followed a similar trend and decreased from –1.93 MPa in juvenile leaves to –2.31 MPa in adult leaves from the highest sampling point. Juvenile leaves had a higher ε than adult leaves, indicating less elastic cells in the juvenile foliage. Moreover, ε was significantly lower in adult leaves from the top than from the base of the plant. All three leaf hydraulic parameters correlated with sampling height (Fig. 1A–C), whether only adult branches or all branches were included in the analysis.
Table 1.
Pressure–volume analysis of Hedera helix
| Juvenile leaves | Adult leaves | |||
|---|---|---|---|---|
| Parameter | 0–1 m | 1–2 m | 7–8 m | 12–13 m |
| ψo (MPa) | –1.72±0.10a | –1.71±0.05a | –1.80±0.03a | –2.05±0.09b |
| ψtlp (MPa) | –1.93±0.15a | –2.00±0.07a | –2.04±0.06a | –2.31±0.12b |
| ε (MPa) | 0.67±0.06a | 0.49±0.10b | 0.21±0.02c | 0.18±0.02c |
Osmotic potential at saturation (Ψo), turgor loss point (Ψtlp), and modulus of elasticity (ε) of juvenile leaves and adult leaves from the lower, middle, and upper part of the plant. Data presented are means ±SE. Different letters indicate significant differences.
Hydraulic conductivity and vulnerability to drought-induced embolism
In stem and branch xylem, ks varied between 6.58 and 31.14 m2 s–1 MPa–1 ×10–4 along the hydraulic pathway. It increased significantly with height, both in the main stem and in branches (Table 2, Fig. 1D). Moreover, ks was more than twice as high in the main stem as in branches of the same sampling height. Juvenile branches showed a significantly lower ks compared with adult branches at the lowest sampling point (Table 2).
Table 2.
Xylem hydraulic and anatomical parameters of Hedera helix
| Juvenile branches | Adult branches | Main stem | ||||
|---|---|---|---|---|---|---|
| Parameter | 0–1 m | 1–2 m | 7–8 m | 12–13 m | 1–2 m | 7–8 m |
| k s (m2 s–1 MPa–1 × 10–4) | 6.58±0.40a | 9.16±0.75b | 15.40±0.54c | 18.67±0.99d | 24.82±1.96e | 31.14±1.41f |
| ψLC12 (MPa) | –2.43±0.22a | –2.22±0.23a | –2.13±0.27a | –2.76±0.28a | –0.75±0.13b | –0.88±0.12b |
| ψLC50 (MPa) | –3.90±0.14a | –3.55±0.15b | –3.97±0.15ac | –4.22±0.14c | –2.05±0.11d | –2.58±0.11e |
| ψLC88 (MPa) | –5.36±0.15a | –4.86±0.14b | –5.85±0.13c | –5.67±0.12ac | –3.32±0.18d | –4.32±0.12e |
| a | 1.38±0.10ab | 1.58±0.11a | 1.18±0.11b | 1.31±0.12ab | 1.60±0.14a | 1.19±0.05b |
| d mean (µm) | 14.60±0.64a | 15.55±1.27a | 19.40±1.16ab | 20.23±2.27b | 23.12±0.64bc | 26.63±1.13c |
| d max (µm) | 35.28 | 37.79 | 48.30 | 56.45 | 76.72 | 84.54 |
| d h (µm) | 23.81±1.05a | 24.29±1.08a | 28.17±2.62a | 30.63±3.58a | 54.41±1.87b | 57.19±3.17b |
| (t/b)2 | 0.023±0.003a | 0.012±0.001b | 0.017±0.003c | 0.020±0.001d | 0.018±0.002cd | 0.019±0.001cd |
| arealumen (%) | 18.65±1.42a | 23.97±2.52a | 30.93±0.45b | 32.42±2.37b | 53.51±2.47c | 56.61±2.65c |
| areapith (%) | 56.57±2.09a | 25.09±1.70b | 28.23±2.43b | 30.51±2.63b | 0.87±0.07c | 1.20±0.12c |
Specific hydraulic conductivity (ks), xylem water potential at 12%, 50%, and 88% loss of xylem conductivity (ΨLC12, ΨLC50, ΨLC88), slope of the vulnerability curve (a), mean conduit diameter (dmean), maximum conduit diameter (dmax), mean hydraulic diameter (dh), cell wall thickness to span ratio [(t/b)2], percentage of xylem area covered by conduit lumen (arealumen), and percentage of the cross-sectional area occupied by the central pith parenchyma (areapith). Data presented are means ±SE. Different letters indicate significant differences.
Ψ at 50% PLC (ΨLC50) of the main stem varied between –2.05 and –2.58 MPa, and ΨLC50 of branches varied between –3.55 and –4.22 MPa (Table 2). The higher vulnerability of the main stem was also characterized by less negative lower and upper vulnerability thresholds, that is, by significantly less negative ΨLC12 and ΨLC88 compared with respective values for the branches. In adult branches, ΨLC50 decreased with height (Fig. 1E, Fig. 2A–C), and the main stem also showed a more negative ΨLC50 at the higher section compared with the lower section (Table 2). Interestingly, the shift with increasing height was mainly caused by flatter vulnerability curves in both the main stem and branches at higher sampling points (Fig. 2; Table 2). Juvenile branches with a ΨLC50 of –3.90 MPa were slightly more resistant to drought-induced embolism formation than adult branches at 1–2 m height (–3.55 MPa; Table 2).
Fig. 2.
Drought-induced vulnerability (loss of conductivity versus water potential; pooled data of 5–9 curves) of (A–D) branches (adult from the upper, middle, and lower crown, and juvenile) and (E, F) the main stem (middle and lower crown) of Hedera helix. Vertical lines on each plot indicate the water potentials at (right to left) 12%, 50%, and 88% loss of conductivity.
Wood characteristics
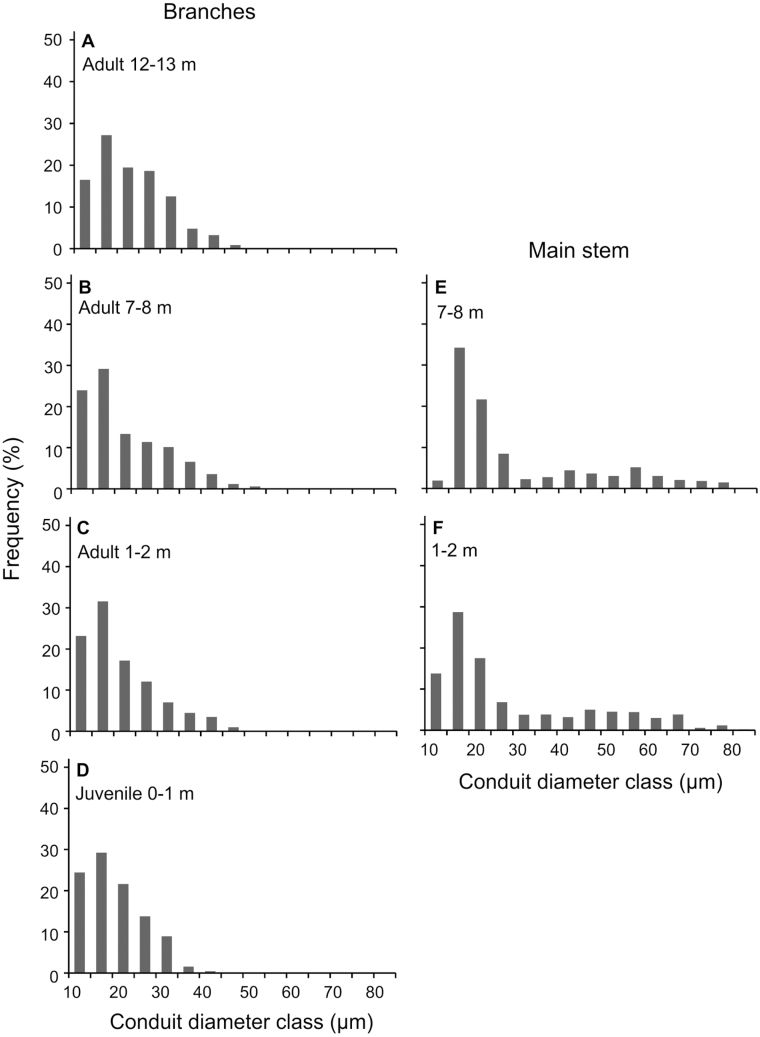
The main stem of H. helix was characterized by wider conduits compared with the branches, with a dmean up to 26.63 µm (branches 20.23 µm), dh up to 57.19 µm (branches 30.63 µm), and dmax up to 84.54 µm (branches 56.45 µm; Table 2). Conduit diameters (i.e. dmean, dh, and dmax) increased with height in both branches and the main stem (Table 2, Fig. 1F–H) and the frequency distribution showed a shift to wider conduits with increasing height (Fig. 3). The xylem of branches contained a higher proportion of fibres than was found in the stem (see Supplementary Fig. S1 at JXB online), resulting in a lower arealumen. This applied especially to juvenile branches, with an arealumen of 18.65% (compared with 56.61% in the upper stem; Table 2). Moreover, juvenile branches were characterized by extensive pith parenchyma in the centre and a narrow surrounding xylem area, resulting in a high areapith (56.6%; Table 2), while adult branches of the same diameter exhibited a smaller pith and thus a higher proportion of xylem area.
Fig. 3.
Mean distribution of conduit diameters (5 µm classes) in branches and main stems of Hedera helix. Data of five samples per sampling height were pooled.
Cell wall reinforcement was highest in juvenile branches, with a (t/b)2 of 0.023, and lowest in adult branches at the lowest sampling point (0.012). If only adult branches were considered, (t/b)2 increased significantly with height (R2=0.76, P<0.001), but due to the deviance of juvenile branches no overall correlation of branch cell wall reinforcement with height was detected (Fig. 1I). At 1–2 m height, (t/b)2 was significantly higher in the main stem than in adult branches (Table 2).
Correlation analysis between ks and wood anatomical parameters revealed a positive correlation of ks with dmean (R2=0.71), dh (R2=0.84), dmax (R2=0.97), and arealumen (R2=0.92), but not with (t/b)2 (R2=0.08, P=0.600; Fig. 4A–C). ΨLC50 was positively correlated with dmean (R2=0.35), dh (R2=0.78), and arealumen (R2=0.64), but not with dmax (R2=0.60, P=0.069) or (t/b)2 (R2=0.04, P=0.720; Fig. 4D–F).
Growth
Tree ring analysis revealed an age of 46–50 years at a minimum for the studied individual ivy plants (see Supplementary Fig. S2); unfortunately, the first rings near the pith could not be well distinguished in all discs. The annual rings of the main stem at 7.5 m height were significantly narrower than those at 1.5 m height for several years, but reached the same width during recent years, with a width of ~0.8 mm per year.
Discussion
The present study revealed clear differences between the main stem and branches, as well as pronounced vertical gradients in leaf osmotic parameters, hydraulic safety and efficiency, and xylem anatomical features in the liana H. helix. Results indicate that the coordination of carbon and water economies with respect to the tall growth form and a vertical light gradient forces non-self-supporting plants to follow within-plant hydraulic adjustments that are similar to those of trees.
General hydraulic strategy
Efficient and safe water transport from the base to the top is critical for plant growth and survival, especially if large leaf areas in the upper canopy are supported by long and narrow main stems. The present study indicates that ivy follows an efficient but risky hydraulic strategy in terms of a high ks in combination with a comparably high ΨLC50 in the main stem. This is consistent with previous research on tropical lianas (e.g. Van der Sande et al., 2013; Chen et al., 2017). The ks measured for H. helix (up to 18.67 m2 s–1 MPa–1 × 10–4 in branches and up to 31.14 m2 s–1 MPa–1 × 10–4 in the main stem; Table 2) was higher than mean values reported for temperate angiosperm trees, but lower than ks values in vines and most tropical lianas (Gartner et al., 1990; Carvalho et al., 2015; Chen et al., 2017). This is not surprising, as extremely wide vessels (>100 µm), which were found in many tropical lianas and vines (e.g. Gartner et al., 1990; Ewers and Fisher, 1991), were not present in ivy (Table 2, Fig. 3). However, the higher ks compared with those of trees can compensate for the smaller xylem cross-sectional area of the liana and enable high flow rates despite the relatively narrow main stem.
The strong down-regulation of stomatal conductance in ivy at vapour pressure deficits greater than 0.5 kPa (Leuzinger et al., 2011) implies that, despite its high ks in the main stem, ivy is not maximizing gas exchange during the middle of the day but avoids low Ψ. Accordingly, Ψ values were reported not to fall below –1.3 to –1.7 MPa at midday (at 23 m height). The derived assumption of Leuzinger et al. (2011) that this strategy originates from a high stem vulnerability to the formation of embolism can be confirmed by findings of the present study, as ΨLC50 was between –2.05 and –2.58 MPa in the main stem (Table 2). At approximately –1.5 MPa, a PLC of 20–30% can be expected in the main stem (Fig. 2E–F), which forces ivy to close stomata before more critical water potentials in the stem are reached. The vulnerability of the main stem of ivy is probably higher than of typical host trees such as Larix decidua, Picea abies, Quercus petraea, and Carpinus betulus. Unfortunately, available vulnerability data for these species (ΨLC50 between –3.50 and –3.98 MPa; Choat et al., 2012) and most other tree species are based on branch samples, while information on the vulnerability of main stems is scarce, due to methodological difficulties. Better knowledge of the hydraulics of mature plant trunks would be a prerequisite (see also Johnson et al., 2016) to better understand the hydraulic architecture of trees as well as lianas. Lower embolism resistance of lianas compared with trees has also been reported for tropical species and was based on comparisons of branches or seedling stems (Van der Sande et al., 2013; Chen et al., 2017). The investigated tropical lianas showed an overall higher vulnerability than that of ivy (ΨLC50 between –0.29 and –1.57 MPa), while vine-like bamboos adapted to drier habitats can reach ΨLC50 values below –4 MPa (Cochard et al., 1994). Further studies on temperate lianas would be needed to elucidate general trends in the variation in hydraulic efficiency and safety across climatic zones and precipitation gradients in climbing plants.
Hydraulics of the main stem versus branches
The water transport system in the main stem of ivy was distinctly more efficient than in branches, with a ks twice as high (Table 2). In contrast, the main stem was less embolism resistant, as its ΨLC50 was up to 1.5 MPa less negative compared with branches from the same height (Table 2). The higher hydraulic efficiency and lower safety in the main stem compared with branches corresponds to patterns reported for lianas from semi-arid regions (Carvalho et al., 2015) and several angiosperm and gymnosperm trees (Zimmermann, 1978; Domec et al., 2006; Beikircher and Mayr, 2008; Johnson et al., 2016). Due to the small cross-sectional area of the main stem, high ks is apparently essential for temperate lianas to prevent a strong drop of Ψ during transpiration, and limited embolism resistance is probably the consequence of a trade-off between safety and efficiency (i.e. based on pit characteristics and conduit size, grouping, and connection; Wheeler et al., 2005). Furthermore, comparably low hydraulic safety in the main stem might be sufficient for lianas growing under moist conditions, and even more so if they have conservative stomatal regulation (see above), as is the case in ivy. However, it remains unclear how temperate lianas exposed to frequent drought stress may balance hydraulic efficiency and safety.
The higher hydraulic safety observed in branches compared with the main stem in ivy can be related to the branches’ position at the distal end of the water transport pathway, where lower Ψ values occur during transpiration; a similar situation occurs in trees. Furthermore, branches of ivy showed a higher proportion of supporting tissue and smaller conduits in the xylem compared with the main stem (Supplementary Fig. S1), which may not only lead to lower ks but also correspond to lower ΨLC50 (Table 2). Rigid xylem structures in the branches are probably formed for mechanical reasons, which is especially relevant in adult crown parts of ivy, where shoots do not form adhesive rootlets (Bauer and Bauer, 1980) and thus cannot statically rely on the host tree.
Height-related hydraulic changes
With increasing height, Ψ decreases because of gravity and cumulative resistance in the hydraulic pathway (Tyree and Ewers, 1991). Distal parts of the plant thus require adaptations to maintain a favourable water status at lower Ψ. Accordingly, in H. helix, the Ψo, Ψtlp, and ε of leaves, as well as the ΨLC50 of stem and branches, decreased with increasing height (Fig. 1, Table 2), to counter potential problems such as wilting or embolism formation. Furthermore, ks increased in branches with increasing insertion height, and between 1 m and 7 m along the main stem (Fig. 1D, Table 2). Higher ks in upper branches has also been reported for several tall tree species (Clearwater and Meinzer, 2001; Burgess et al., 2006; Domec et al., 2006; Yoshimura 2011), indicating that hydraulic traits are coordinated in a similar way within both self-supporting and non-self-supporting tall plants to ensure water supply to a large number of leaves with high transpirational demands in the upper crown (Pfautsch et al., 2018) and to compensate for their height ‘disadvantage’ (Zimmermann, 1978; Bettiati et al., 2012). Higher xylem conductivity was the result of larger conduits as well as a higher arealumen and may be crucial to balance hydraulic resistances in the crown (see the section on xylem structure, below, for a detailed discussion). There was no trade-off between safety and efficiency in branches across different heights in ivy, as branches from the upper crown regions showed high hydraulic safety and efficiency.
Hydraulics of juvenile versus adult branches
Juvenile leaves of H. helix did not differ in Ψo and Ψtlp compared with adult leaves near the ground (Table 1). Although this may seem unexpected, because juvenile leaves are characterized as shade leaves and adult leaves as sun leaves (Bauer and Bauer, 1980), it has to be borne in mind that those leaves grew under similar low-light conditions. We chose large ivy plants, in which the lowest adult crown parts were situated in the shadow of the upper (host and ivy) crown. The differences between the two life stages were more pronounced with respect to adult leaves at higher levels, where the liana reached the canopy of the host tree, where there are higher light levels and consequently higher photosynthetic and transpiration rates (Leuzinger et al., 2011). Adult leaves from the upper crown showed a better osmotic adaptation to low water potentials (Ψo –2.05 MPa and Ψtlp –2.31 MPa; see also Burghardt and Riederer, 2003 for comparison). Cell walls were more elastic in adult than in juvenile leaves, which corresponds to the fact that juvenile leaves are more rigid and morphologically different from adult leaves (Bauer and Bauer, 1980). Moreover, the juvenile and adult life phases differed in ks and ΨLC50, with juvenile branches showing a lower xylem efficiency but higher embolism resistance (Table 2). Low ks is probably sufficient in juvenile branches because the cumulative area and transpiration rates of juvenile leaves are lower than in adult leaves (Miller and Goodin, 1976, Bauer and Bauer, 1980). In this case, high embolism resistance (Fig. 2D, Table 2) is advantageous as it guarantees permanent, although small, transport capacity. As soon as the plant starts to form a large crown with adult branches, probably in combination with an extended root system, transport capacity may become more important and hydraulic traits characteristic for tall plants more pronounced.
Xylem structure
Tree ring analyses revealed increasing growth rates during the first 25 years of the life span of the studied ivy plants (Supplementary Fig. S2), when the liana was progressively extending its upper canopy. Mean annual xylem growth during the most recent 5 years settled at 0.82±0.06 mm, comparable to values reported for ivy in alluvial environments by Castagneri et al. (2013) (1.05±0.54 mm) and Heuzé et al. (2009) (0.77–1.89 mm).
Xylem anatomical parameters differed significantly between the main stem and branches, across heights, and between juvenile and adult branches of H. helix (Table 2, Fig. 1F–J) and explained most of the variation in hydraulic efficiency and partly also the variation in hydraulic safety. In accordance with the Hagen–Poiseuille equation, ks was highly correlated with dh, but also with dmean, dmax, and arealumen (Fig. 4B, C). For several lianas and vines, studies revealed a stronger linkage between hydraulic conductance and conduit size than in trees (Ewers et al., 1989; Gartner et al., 1990). This can be explained by the long vessels and thus comparably minor pit effects (Tyree and Zimmermann, 2002), and by the reduced mechanical function of the wood in non-self-supporting plants (Tyree and Ewers, 1991). Internal xylem anatomical adjustments are crucial for tall plants, as gravitational pressure and path length resistances increase with plant size. Scaling conduit dimensions along the vertical axis can minimize the height effect, maximize the hydraulic conductance for a given carbon investment, and improve equal water supply to all parts of the crown (Mencuccini et al., 2007; Bettiati et al., 2012). Accordingly, conduit widening was empirically observed for trees in several studies (e.g. Anfodillo et al., 2006; Olson et al., 2014; Prendin et al., 2018); it was also reported for non-self-supporting plants by comparing species with varying stem length (interspecific tapering; Olson et al., 2014), but had not previously been analysed within a liana. Although the present study does not allow us to draw detailed conclusions about the axial widening within ivy because of the limited number of sampling points along the stem, it seems likely that this liana behaves like self-supporting trees. According to conduit widening allometry, the diameter varies sharply near the apex but very little near the stem base (Anfodillo et al., 2006), and the small increase in conduit diameter at the trunk base found in ivy (Table 2) is also a frequently observed phenomenon in trees (Bettiati et al., 2012; Pfautsch et al., 2018). The clear increase of conduit dimensions with branch insertion height (Fig. 1F–H) was similarly found in several tree species (Clearwater and Meinzer, 2001; Burgess et al., 2006; Bettiati et al., 2012) and can equalize the hydraulic resistance of all root-to-leaf pathways within the crown. As the water transport distance from the trunk base is longer in distal leaves, they would be hydraulically disadvantaged compared with leaves on lower branches unless larger conduits reduced the hydraulic resistance.
Interrelations between embolism resistance and xylem anatomy were not pronounced in ivy, as ΨLC50 was correlated with dh, dmean, and arealumen, but not with dmax and (t/b)2 (Fig. 4D–F), although the higher vulnerability of adult versus juvenile branches, and of lower versus upper branches, was associated with lower (t/b)2 (Table 2). A relationship between embolism resistance and (t/b)2 could be expected, as lower ΨLC50 values are commonly associated with higher wall reinforcement to reduce wall bending and the risk of conduit collapse under negative pressure (Hacke and Sperry, 2001; Wheeler et al., 2005). In contrast, the relationship with dh might be indirect via pit properties (the ‘pit area hypothesis’). For instance, microtomographic observations on Laurus nobilis showed that at moderate water stress the fraction of embolized vessels was highest in the upper vessel diameter classes (Nardini et al., 2017). Vulnerability to freeze–thaw-induced embolism, which is more directly linked to conduit diameter, was not considered in our study. Nevertheless, this aspect has been suggested to be relevant for temperate lianas (Ewers, 1985; Tibbetts and Ewers, 2000) and should be investigated in further studies.
Overall, the close linkage between wood anatomical traits and ks indicates a strong selective pressure for hydraulic efficiency in temperate lianas. Balance of hydraulic efficiency with hydraulic safety is achieved in H. helix, as in other liana species (Carlquist, 1985), by a characteristic combination of wide and efficient vessels with narrow and probably embolism-resistant vessels in the main stem (Fig. 3).
Conclusions
The temperate liana H. helix shows a hydraulic architecture adapted to its climbing habit, tall growth form, and occurrence in rather moist forests, although its hydraulic characteristics are less pronounced than in better-studied tropical lianas. Anatomical features of xylem compensate for the limited cross-sectional area of the main stem, ensuring high hydraulic efficiency and water supply of a large leaf area. Low transport resistances, together with the reported sensitive stomatal response, can prevent a strong drop in Ψ upon transpiration and thus relatively high ΨLC50 in the stem may not be critical for performance and survival.
Within-plant hydraulic variations were pronounced between branches and the main stem and with increasing branch insertion height, but small between the juvenile and adult life phases. Patterns of xylem anatomy and hydraulic efficiency and safety are in accordance with the pattern reported for trees, suggesting that lianas apply the same effective architectural design as self-supporting plants to reduce the path length effect on the total hydraulic resistance and guarantee efficient and safe water supply to all parts of the crown. This study highlights the necessity of studying whole-plant hydraulic architecture, including varying growth forms, to better understand the hydraulics of tall plants and underlying functional trade-offs.
Author contributions
SM and AG led the study; all authors contributed to the experimental design and methodical developments, conducted field and laboratory measurements, and performed data analysis; AG prepared the article with contributions from all the authors; SM supervised and complemented the writing.
Supplementary data
Supplementary data are available at JXB online.
Fig. S1. Cross-sections of a Hedera helix branch and main stem.
Fig. S2. Growth (annual ring width) of Hedera helix stems from the years 1965 to 2015.
Acknowledgements
The study was supported by the Austrian Science Fund (FWF): P29896-B22 and T667-B16. We thank the Botanical Garden of the Department of Botany, University of Innsbruck, and the involved gardeners and tree climbers for help with sampling, as well as Birgit Dämon for excellent assistance in the laboratory and Walter Oberhuber for help with tree ring analysis. We are grateful to anonymous reviewers for constructive comments that helped to improve the manuscript.
References
- Andergassen S, Bauer H. 2002. Frost hardiness in the juvenile and adult life phase of ivy (Hedera helix L.). Plant Ecology 161, 207–213. [Google Scholar]
- Anfodillo T, Carraro V, Carrer M, Fior C, Rossi S. 2006. Convergent tapering of xylem conduits in different woody species. New Phytologist 169, 279–290. [DOI] [PubMed] [Google Scholar]
- Bauer H, Bauer U. 1980. Photosynthesis in leaves of the juvenile and adult life phase of ivy (Hedera helix). Physiologia Plantarum 49, 366–372. [Google Scholar]
- Bettiati D, Petit G, Anfodillo T. 2012. Testing the equi-resistance principle of the xylem transport system in a small ash tree: empirical support from anatomical analyses. Tree Physiology 32, 171–177. [DOI] [PubMed] [Google Scholar]
- Beikircher B, Ameglio T, Cochard H, Mayr S. 2010. Limitation of the Cavitron technique by conifer pit aspiration. Journal of Experimental Botany 61, 3385–3393. [DOI] [PubMed] [Google Scholar]
- Beikircher B, Mayr S. 2008. The hydraulic architecture of Juniperus communis L. ssp. communis: shrubs and trees compared. Plant, Cell & Environment 31, 1545–1556. [DOI] [PubMed] [Google Scholar]
- Beikircher B, Mayr S. 2016. Avoidance of harvesting and sampling artefacts in hydraulic analyses: a protocol tested on Malus domestica. Tree Physiology 36, 797–803. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Burgess SS, Pittermann J, Dawson TE. 2006. Hydraulic efficiency and safety of branch xylem increases with height in Sequoia sempervirens (D. Don) crowns. Plant, Cell & Environment 29, 229–239. [DOI] [PubMed] [Google Scholar]
- Burghardt M, Riederer M. 2003. Ecophysiological relevance of cuticular transpiration of deciduous and evergreen plants in relation to stomatal closure and leaf water potential. Journal of Experimental Botany 54, 1941–1949. [DOI] [PubMed] [Google Scholar]
- Carlquist S. 1985. Observations on functional wood histology of vines and lianas: vessel dimorphism, tracheids, vasicentric tracheids, narrow vessels, and parenchyma. Aliso 11, 139–157. [Google Scholar]
- Carvalho ECD, Martins FR, Soares AA, Oliveira RS, Muniz CR, Araújo FS. 2015. Hydraulic architecture of lianas in a semiarid climate: efficiency or safety? Acta Botanica Brasilica 29, 198–206. [Google Scholar]
- Castagneri D, Garbarino M, Nola P. 2013. Host preference and growth patterns of ivy (Hedera helix L.) in a temperate alluvial forest. Plant Ecology 214, 1–9. [Google Scholar]
- Chen YJ, Schnitzer SA, Zhang YJ, Fan ZX, Goldstein G, Tomlinson KW, Lin H, Zhang JL, Cao KF. 2017. Physiological regulation and efficient xylem water transport regulate diurnal water and carbon balances of tropical lianas. Functional Ecology 31, 306–317. [Google Scholar]
- Choat B, Jansen S, Brodribb TJ, et al. 2012. Global convergence in the vulnerability of forests to drought. Nature 491, 752–755. [DOI] [PubMed] [Google Scholar]
- Clearwater MJ, Meinzer FC. 2001. Relationships between hydraulic architecture and leaf photosynthetic capacity in nitrogen-fertilized Eucalyptus grandis trees. Tree Physiology 21, 683–690. [DOI] [PubMed] [Google Scholar]
- Cochard H, Damour G, Bodet C, Tharwat I, Poirier M, Améglio T. 2005. Evaluation of a new centrifuge technique for rapid generation of xylem vulnerability curves. Physiologia Plantarum 124, 410–418. [Google Scholar]
- Cochard H, Ewers FW, Tyree MT. 1994. Water relations of a tropical vine-like bamboo (Rhipidocladum racemiflorum): root pressures, vulnerability to cavitation and seasonal changes in embolism. Journal of Experimental Botany 45, 1085–1089. [Google Scholar]
- Domec JC, Lachenbruch B, Meinzer FC. 2006. Bordered pit structure and function determine spatial patterns of air-seeding thresholds in xylem of Douglas-fir (Pseudotsuga menziesii; Pinaceae) trees. American Journal of Botany 93, 1588–1600. [DOI] [PubMed] [Google Scholar]
- Ewers FW. 1985. Xylem structure and water conduction in conifer trees, dicot trees, and lianas. IAWA Journal 6, 309–317. [Google Scholar]
- Ewers FW, Fisher JB. 1989. Techniques for measuring vessel lengths and diameters in stems of woody plants. American Journal of Botany 76, 645–656. [Google Scholar]
- Ewers FW, Fisher JB. 1991. Why vines have narrow stems: Histological trends in Bauhinia (Fabaceae). Oecologia 88, 233–237. [DOI] [PubMed] [Google Scholar]
- Ewers FW, Fisher JB, Chiu ST. 1989. Water transport in the liana Bauhinia fassoglensis (Fabaceae). Plant Physiology 91, 1625–1631. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Ganthaler A, Mayr S. 2015. Dwarf shrub hydraulics: two Vaccinium species (Vaccinium myrtillus, Vaccinium vitis-idaea) of the European Alps compared. Physiologia Plantarum 155, 424–434. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gartner BL. 1991. Stem hydraulic properties of vines vs. shrubs of western poison oak, Toxicodendron diversilobum. Oecologia 87, 180–189. [DOI] [PubMed] [Google Scholar]
- Gartner BL, Bullock SH, Mooney HA, By Brown V, Whitbeck JL. 1990. Water transport properties of vine and tree stems in a tropical deciduous forest. American Journal of Botany 77, 742–749. [Google Scholar]
- Gleason SM, Westoby M, Jansen S, et al. 2016. Weak tradeoff between xylem safety and xylem-specific hydraulic efficiency across the world’s woody plant species. New Phytologist 209, 123–136. [DOI] [PubMed] [Google Scholar]
- Hacke UG, Sperry JS. 2001. Functional and ecological xylem anatomy. Perspectives in Plant Ecology, Evolution and Systematics 4, 97–115. [Google Scholar]
- Heuzé P, Dupouey JL, Schnitzler A. 2009. Radial growth response of Hedera helix to hydrological changes and climatic variability in the Rhine floodplain. River Research and Applications 25, 393–404. [Google Scholar]
- Hoflacher H, Bauer H. 1982. Light acclimation in leaves of the juvenile and adult life phases of ivy (Hedera helix). Physiologia Plantarum 56, 177–182. [Google Scholar]
- Ichihashi R, Chiu CW, Komatsu H, Kume T, Shinohara Y, Tateishi M, Tsuruta K, Otsuki K. 2017. Contribution of lianas to community-level canopy transpiration in a warm-temperate forest. Functional Ecology 31, 1690–1699. [Google Scholar]
- Johnson DM, Wortemann R, McCulloh KA, Jordan-Meille L, Ward E, Warren JM, Palmroth S, Domec JC. 2016. A test of the hydraulic vulnerability segmentation hypothesis in angiosperm and conifer tree species. Tree Physiology 36, 983–993. [DOI] [PubMed] [Google Scholar]
- Kolb KJ, Sperry JS. 1999. Differences in drought adaptation between subspecies of sagebrush (Artemisia tridentata). Ecology 80, 2373–2384. [Google Scholar]
- Leuzinger S, Hartmann A, Körner C. 2011. Water relations of climbing ivy in a temperate forest. Planta 233, 1087–1096. [DOI] [PubMed] [Google Scholar]
- Mencuccini M, Hölttä T, Petit G, Magnani F. 2007. Sanio’s laws revisited. Size-dependent changes in the xylem architecture of trees. Ecology Letters 10, 1084–1093. [DOI] [PubMed] [Google Scholar]
- Metcalfe DJ. 2005. Hedera helix L. Journal of Ecology 93, 632–648. [Google Scholar]
- Miller DR, Goodin JR. 1976. Cellular growth rates of juvenile and adult Hedera helix L. Plant Science Letters 7, 397–401. [Google Scholar]
- Nardini A, Savi T, Losso A, Petit G, Pacilè S, Tromba G, Mayr S, Trifilò P, Lo Gullo MA, Salleo S. 2017. X-ray microtomography observations of xylem embolism in stems of Laurus nobilis are consistent with hydraulic measurements of percentage loss of conductance. New Phytologist 213, 1068–1075. [DOI] [PubMed] [Google Scholar]
- Olson ME, Anfodillo T, Rosell JA, Petit G, Crivellaro A, Isnard S, León-Gómez C, Alvarado-Cárdenas LO, Castorena M. 2014. Universal hydraulics of the flowering plants: vessel diameter scales with stem length across angiosperm lineages, habits and climates. Ecology Letters 17, 988–997. [DOI] [PubMed] [Google Scholar]
- Pammenter NW, Vander Willigen C. 1998. A mathematical and statistical analysis of the curves illustrating vulnerability of xylem to cavitation. Tree Physiology 18, 589–593. [DOI] [PubMed] [Google Scholar]
- Petit G, Savi T, Consolini M, Anfodillo T, Nardini A. 2016. Interplay of growth rate and xylem plasticity for optimal coordination of carbon and hydraulic economies in Fraxinus ornus trees. Tree Physiology 36, 1310–1319. [DOI] [PubMed] [Google Scholar]
- Pfautsch S, Aspinwall MJ, Drake JE, Chacon-Doria L, Langelaan RJA, Tissue DT, Tjoelker MG, Lens F. 2018. Traits and trade-offs in whole-tree hydraulic architecture along the vertical axis of Eucalyptus grandis. Annals of Botany 121, 129–141. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Prendin AL, Mayr S, Beikircher B, von Arx G, Petit G. 2018. Xylem anatomical adjustments prioritize hydraulic efficiency over safety as Norway spruce trees grow taller. Tree Physiology 38, 1088–1097. [DOI] [PubMed] [Google Scholar]
- Rehm EM, Lenz A, Hoch G, Körner C. 2014. Spring patterns of freezing resistance and photosynthesis of two leaf phenotypes of Hedera helix. Basic and Applied Ecology 15, 543–550. [Google Scholar]
- Rosell JA, Olson ME. 2014. Do lianas really have wide vessels? Vessel diameter–stem length scaling in non-self-supporting plants. Perspectives in Plant Ecology, Evolution and Systematics 16, 288–295. [Google Scholar]
- Schnitzer SA, Bongers F. 2002. The ecology of lianas and their role in forests. Trends in Ecology and Evolution 17, 223–230. [Google Scholar]
- Tibbetts TJ, Ewers FW. 2000. Root pressure and specific conductivity in temperate lianas: exotic Celastrus orbiculatus (Celastraceae) vs. native Vitis riparia (Vitaceae). American Journal of Botany 87, 1272–1278. [PubMed] [Google Scholar]
- Tyree MT, Ewers FW. 1991. The hydraulic architecture of trees and other woody plants. New Phytologist 119, 345–360. [Google Scholar]
- Tyree MT, Hammel HT. 1972. The measurement of the turgor pressure and the water relations of plants by the pressure-bomb technique. Journal of Experimental Botany 23, 267–282. [Google Scholar]
- Tyree MT, Zimmermann MH. 2002. Xylem structure and the ascent of sap. Berlin: Springer. [Google Scholar]
- van der Sande MT, Poorter L, Schnitzer SA, Markesteijn L. 2013. Are lianas more drought-tolerant than trees? A test for the role of hydraulic architecture and other stem and leaf traits. Oecologia 172, 961–972. [DOI] [PubMed] [Google Scholar]
- Walther GR. 2002. Weakening of climate constraints with global warming and its consequences for evergreen broad-leaved species. Folia Geobotanica 37, 129–139. [Google Scholar]
- West GB, Brown JH, Enquist BJ. 1999. A general model for the structure and allometry of plant vascular systems. Nature 400, 664–667. [Google Scholar]
- Wheeler JK, Sperry JS, Hacke UG, Hoang N. 2005. Inter-vessel pitting and cavitation in woody Rosaceae and other vesselled plants: a basis for a safety versus efficiency trade-off in xylem transport. Plant Cell and Environment 28, 800–812. [Google Scholar]
- Wyka TP, Oleksyn J, Karolewski P, Schnitzer SA. 2013. Phenotypic correlates of the lianescent growth form: a review. Annals of Botany 112, 1667–1681. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Yoshimura K. 2011. Hydraulic function contributes to the variation in shoot morphology within the crown in Quercus crispula. Tree Physiology 31, 774–781. [DOI] [PubMed] [Google Scholar]
- ZAMG Zentralanstalt für Meteorologie und Geodynamik. 2002. Klimadaten von Österreich 1971-2000 http://www.zamg.ac.at/fix/klima/oe71-00/klima2000/klimadaten_oesterreich _1971_frame1.htm. Accessed December 18, 2017.
- Zimmermann MH. 1978. Hydraulic architecture of some diffuse-porous trees. Canadian Journal of Botany 56, 2286–2295. [Google Scholar]
- Zotz G, Cueni N, Körner C. 2006. In situ growth stimulation of a temperate zone liana (Hedera helix) in elevated CO2. Functional Ecology 20, 763–769. [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.