Molecular Mechanism for Folding Cooperativity of Functional RNAs in Living Organisms

Abstract

A diverse set of organisms has adapted to live under extreme conditions. The molecular origin of the stability is unclear, however. It is not known whether the adaptation of functional RNAs, which have intricate tertiary structures, arises from strengthening of tertiary or secondary structure. Herein we evaluate effects of sequence changes on the thermostability of tRNA^phe using experimental and computational approaches. To separate out effects of secondary and tertiary structure, we modify base pairing strength in the acceptor stem, which does not participate in tertiary structure. In dilute solution conditions, strengthening secondary structure leads to non-two-state thermal denaturation curves and has small effects on thermostability, or the temperature at which tertiary structure and function is lost. In contrast, under cellular conditions with crowding and Mg²⁺-chelated amino acids, where two-state cooperative unfolding is maintained, strengthening secondary structure enhances thermostability. Investigation of stabilities of each tRNA stem across 44 organisms with a range of optimal growing temperatures revealed that organisms that grow in warmer environments have more stable stems. We also used Shannon entropies to identify positions of higher and lower information content, or sequence conservation, in tRNA^phe and found that secondary structures have modest information content allowing them to drive thermal adaptation, while tertiary structures have maximal information content preventing them from participating in thermal adaptation. Base paired regions with no tertiary structure and modest information content thus offer a facile evolutionary route to enhancing the thermostability of functional RNA by simple molecular rules of base pairing.

Life exists at temperatures ranging from freezing to boiling water, raising questions as to the molecular mechanisms for thermal adaptation. Extensive studies have been conducted on protein folding under extreme conditions,^1–3 but relatively little is known about molecular mechanisms for adaption of RNA sequence to demanding environments. Elucidation of such mechanisms can help establish how extant life has flourished on Earth, as well as provide plausible pathways for the evolution of RNA sequences on early Earth in an RNA World scenario.

Of special interest are so-called ‘functional RNAs’, which require precise tertiary structures for function. These naturally occurring RNAs include ribozymes, riboswitches, rRNA, and tRNA and are key components of an RNA World.^4,5 Crystal structures reveal that tertiary contacts in functional RNAs are diverse and complex and include base triples, ribose zippers, and U-turns.⁶ Underlying these tertiary structures are relatively simple base paired regions that provide the structural framework of the RNA. This leads to hierarchical folding of RNA in which secondary structure formation precedes tertiary structure formation.^{7, 8}

Given the need for functional RNAs to adapt to diverse conditions, the question arises as to whether thermostability—the temperature at which tertiary structure is lost— comes from strengthening tertiary structures or secondary structures. When tertiary structure unfolds, RNA function is lost. Therefore, it might seem that thermostability would increase by strengthening tertiary interactions rather than secondary structure. However, another mechanism is possible. Sosnick and Pan introduced the concept of functional stability, which is the difference in free energy between the fully functional state and the penultimately stable state.⁹ If that penultimately stable state is not secondary structure but rather unfolded RNA, then folding would be cooperative and secondary structure strength could increase thermostability.

To investigate this notion, we study the folding of a series of tRNA^phe constructs with variable strength acceptor stems under diverse solution conditions including dilute buffer, crowders, and Mg²⁺-chelated amino acids, which we and others have found induce cooperative RNA folding.^10–13 The strength of the acceptor stem was tuned by switching between G^•U / AU pairs and GC pairs. We observe that strengthened tRNA mutants fold cooperatively under the most biological-like conditions of crowding in Mg²⁺-chelated amino acids and that such strengthening of base pairing increases thermostability. Moreover, computational analysis of a set of tRNA^phe sequences from organisms that live in a wide range of temperatures reveals that base paired regions strengthen in thermophiles while tertiary structure-participating nucleotides are invariant. This suggests that in nature, tRNA adapts to harsh conditions not by strengthening tertiary interactions but by strengthening base pairing. Given the simple nature of base pairing and the large energetic effects of single nucleotide changes, this offers a simple route to adaptation of RNA and emphasizes the importance of cooperative RNA folding conditions to RNA evolution.

Materials and Methods

Chemicals.

PEG8000, HEPES, MgCl₂, and sodium cacodylate were purchased from Sigma. KCl was purchased from J. T. Baker. Calf intestinal phosphatase and polynucleotide kinase were purchased from NEB.

RNA Constructs and Preparation.

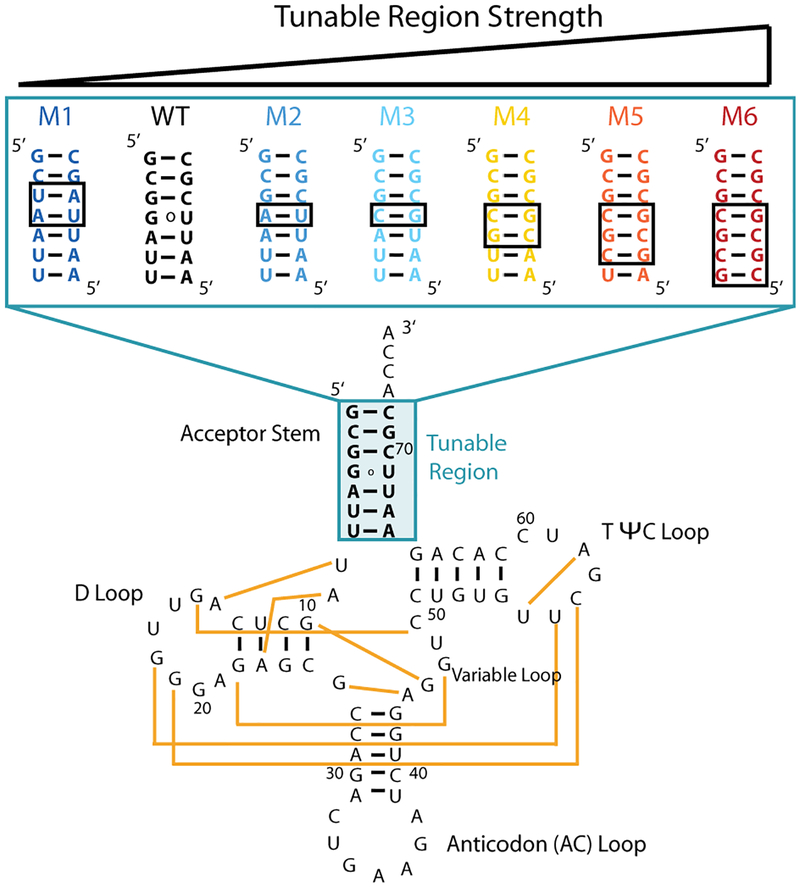
Wild-type (WT) tRNA^phe and mutants (M) were transcribed with a hemi-duplex DNA template purchased from IDT that was used without further purification, as previously described¹¹. The RNA was buffer exchanged into 10 mM sodium cacdoylate using an Amicon ultracentrifugal filter (3 kDa molecular weight cutoff). The RNA sequences are below, and nucleotides mutated from WT are in bold.

FL tRNA^phe:

5ʹGCGGAUUUAGCUCAGUUGGGAGAGCGCCAGACUGAAGAUCUGGAGGUCCUGUGUUCGAUCCACAGAAUUCGCACCA

Mutant 1 (M1):

5’GCUAAUUUAGCUCAGUUGGGAGAGCGCCAGACUGAAGAUCUGGAGGUCCUGUGUUCGAUCCACAGAAUUAGCACCA 3’

Mutant 2 (M2):

5’GCGAAUUUAGCUCAGUUGGGAGAGCGCCAGACUGAAGAUCUGGAGGUCCUGUGUUCGAUCCACAGAAUUCGCACCA 3’

Mutant 3 (M3):

5’GCGCAUUUAGCUCAGUUGGGAGAGCGCCAGACUGAAGAUCUGGAGGUCCUGUGUUCGAUCCACAGAAUGCGCACCA 3’

Mutant 4 (M4):

5’GCGCGUUUAGCUCAGUUGGGAGAGCGCCAGACUGAAGAUCUGGAGGUCCUGUGUUCGAUCCACAGAACGCGCACCA 3’

Mutant 5 (M5):

5’GCGCGCUUAGCUCAGUUGGGAGAGCGCCAGACUGAAGAUCUGGAGGUCCUGUGUUCGAUCCACAGAGCGCGCACCA 3’

Mutant 6 (M6):

5’GCGCGCGUAGCUCAGUUGGGAGAGCGCCAGACUGAAGAUCUGGAGGUCCUGUGUUCGAUCCACAGCGCGCGCACCA 3’

Thermal Denaturation.

RNA was renatured by denaturing at 95°C for 3 min and annealing at room temperature for 10 min in the presence of KCl and sodium cacodylate (pH 7.0). After cooling, MgCl₂, polyethylene glycol (PEG) 8000, and amino acids were added to the RNA solution. The sample was then heated at 55 °C for 3 min and cooled at room temperature for 10 min. Samples were spun down at 14,000 rpm for 5 min at 4 °C to remove air bubbles and particulates. Samples were final concentrations of 0.5 μM RNA in 10 mM sodium cacodylate (pH 7.0), 140 mM KCl, 0.5–2.0 mM MgCl₂, and 0–20% (w/v) PEG8000, and 0–106. mM amino acids, as indicated¹³. Further explanation of Mg²⁺-chelated samples is in Table S4. Thermal denaturation experiments were performed on an HP 8452 diode-array refurbished by OLIS, Inc with a data point collected every 0.5 °C and a data point collected ~0.5 °C/min with absorbance detection from 230–330 nm. This method is referred to as “optical melting”.

Thermal Denaturation Data Analysis

Thermal denaturation data on WT and mutant RNAs was truncated, as in Fig. 2 and Fig. S1, to remove excess baselines and fit globally from 250–290 nm using a two-state model with sloping baselines and a nonlinear Marquartdt algorithm in IgorPro¹⁴ using equation 1, where m_u and m_f are the slopes of the unfolded and folded baselines, b_u and b_f are the y-intercepts of the unfolded and folded baselines, ΔH is the enthalpy of folding, and T_M is the melting temperature in Kelvin. R is the gas constant of 0.001987 kcal⁻¹ K⁻¹ mol⁻¹. In global fitting the slopes and y-intercepts were allowed to vary at each wavelength, but the ΔH and T_M were held constant. The traces at 260 nm were normalized for fraction unfolded using equation 2 which uses the sloping baselines from the global fits to normalize for fraction unfolded, where f is the fraction unfolded and A_T is the raw absorbance at a temperature, T. There is noise in some of the polyethylene glycol data in mutant with high stem stability because the absorbance change in these transitions was low.

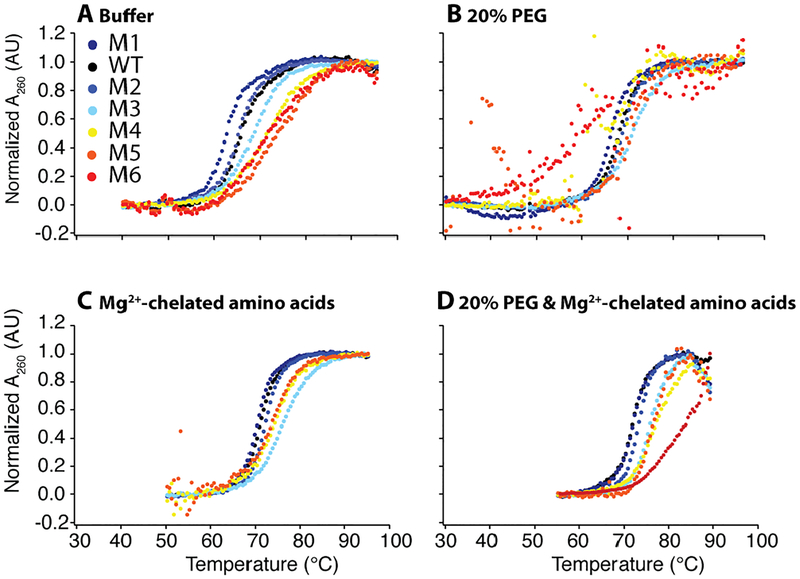
Figure 2.

WT and mutant thermal denaturation under in vivo-like solutions in the background of 2.0 mM free Mg²⁺. Each construct was globally fit every 2 nm between 250 and 290 nm. Thermal denaturation scans at 260 nm normalized using global fitting parameters in (A) buffer, (B) 20% PEG8000, (C) additional 14.0 mM Mg²⁺ weakly chelated to amino acids, and (D) 20% PEG8000 and additional 14.0 mM Mg²⁺ weakly chelated to amino acids. All four panels are in the background of 2.0 mM Mg²⁺ and 140 mM K⁺. Low temperature data was truncated in the fitting to avoid excess baselines, as is plotted above.

$$f(T)=\frac{\left(m_{u}T+b_u\right)+\left(m_{f}T+b_f\right)exp\left(\left[\frac{\Delta H}{R}\right]\left[\frac{1}{T_M+273.15}-\frac{1}{T+273.15}\right]\right)}{1+exp\left(\left[\frac{\Delta H}{R}\right]\left[\frac{1}{T_M+273.15}-\frac{1}{T+273.15}\right]\right)}$$ (eq. 1)

$$f_{unfolded}=\frac{A_T-\left(m_{f}T+b_f\right)}{\left(m_{u}T+b_u\right)-\left(m_{f}T+b_f\right)}$$ (eq. 2)

The theoretical non- and fully cooperative enthalpies of folding were calculated to compare with the experimental ΔH. The non-cooperative ΔH is the ΔH_folding derived using nearest neighbor parameters of the WT and mutant acceptor stems¹⁵. The fully cooperative ΔH is the experimentally derived ΔH_folding of WT plus or minus the nearest neighbor model ΔΔH of the mutant acceptor stems.

Small Angle X-ray Scattering Data Collection and Analysis

WT and MT tRNA constructs were transcribed, purified, and buffer exchanged as described previously¹¹. RNA was renatured in 1× SAXS buffer by denaturing at 95 °C for 3 min and annealing at room temperature for 10 min. After cooling, MgCl₂ was added to the sample which was heated at 55 °C for 3 min then cooled at room temperature for 10 min. Samples were centrifuged at 14k rpm for 10 min to minimize aggregation¹⁶.

SAXS data were collected on G1 station at MacCHESS-the solution scattering beamline at the Cornell High Energy Synchrotron Source (CHESS)¹⁷ using in-line size-exclusion chromatography (SEC) to separate monomers from aggregates, as previously described¹¹. Samples were at a final concentration of 0.2 mg/mL in buffer with 2.0 mM Mg²⁺. SAXS data were analyzed as previously described using the linear Gunier region to obtain structural data¹¹. The scattering curves were compared with simulated tRNA scattering curves using the FoXS webserver^{18, 19}.

In-line Probing

The triphosphate on the 5’ end of T7 transcripts was removed by incubation with calf intestinal phosphatase (CIP) at 37 °C for 20 min, followed by a phenol/chloroform extraction and an ethanol precipitation. This RNA was labeled on the 5’ end with [γ−³²P]ATP by incubation with polynucleotide kinase at 37 °C for 30 min. The labeled RNA was purified by 10% PAGE followed by a crush and soak and ethanol precipitation procedure. 500k cpm of labeled RNA with 140 mM KCl and 20 mM Tris (pH 8.3) was renatured by denaturing at 95 °C for 1 min and annealing at room temperature for 5 min. After annealing, PEG8000, MgCl₂, and amino acids were added as indicated, and the solution was heated at 55 °C for 1 min then cooled at room temperature for 5 min. The samples were incubated at 37 °C and aliquots removed at 12, 24, 36, and 48 hours, which were quenched with 50 mM Tris (pH 7.0) and 20 mM EDTA. RNA was fractionated on a 10% PAGE gel and visualized with a PhosphorImager. Lanes containing different amounts of salts and amino acids were separated on the gel to avoid running contamination. Gel data was analyzed using semi-automated footprinting analysis (SAFA) software²⁰ to obtain reactivities of each individual band. The reactivity of each band was normalized to nucleotides 34–36 in the anticodon loop, which were single-stranded in all solution conditions. This corrects for percent reacted and loading differences.

Optimal Growth Temperature Analysis

We analyzed the strength of tRNA stems, ribosomal RNA GC percent, and genome GC percent against optimal growth temperature of organisms. The base pairs in the acceptor, D, anticodon, and TYC stem for RNA sequences were found using the Transfer RNA database²¹. The portions of the sequence that compose the 5’ and 3’ ends of each stem were input to the RNACofold webserver, part of the Vienna Package, to determine the ΔG of each stem. The growing temperatures of the organisms was found in the DSMZ German Collection of Microorganisms²². Genome and rRNA GC content vs. OGT was fit for a linear correlation in Igor Pro. The sequences from the organisms were binned into six temperature ranges (20–29 °C with 14 sequences, 30–40 °C with 11 sequences, 50–69 °C with 5 sequences, 70–79 °C with 4 sequences, 80–89 °C with 5 sequences, and 90–99 °C with 4 sequences), the temperature in each bin was averaged, and the five weakest ΔG_folding in each bin were averaged together. A linear fit for threshold ΔG_folding was calculated using the averaged values in Igor Pro.

Calculating Information Content

Information content was calculated for each position in tRNA to determine conservation. Nucleotides that are highly conserved have 2 bits of information, nucleotides that are not conserved have 0 bits of information, while two nucleotides that co-vary with Watson-Crick base pairing share 2 bits of information. Information content was calculated separately for the loop and stem positions, as previously described²³. For the nucleotides in loops, information content was calculated by finding the Shannon uncertainty (H) from the sequence alignment using equation 4, where P_i is the probability of finding a particular base, (A, C, G, U) at position i in the sequence. The Shannon uncertainty for genomes is approximately 2, and the information content (IC) is H_genome minus H_position, according to equation 5.

$$H_{position}=-\sum P_i{log}_2{P}_i$$ (eq. 4)

$$IC=H_{genome}-H_{position}$$ (eq. 5)

For stem regions, covariation of base pairs was accounted for when finding information content. Equations 4 and 5 were used to find the Shannon uncertainty and the information content.

Results

Dilute Solution Conditions Lead to Non-Cooperative Folding with Stronger Base Pairing.

Folding of WT and mutant tRNAs (Fig. 1, Tables S1, S2) were first examined under dilute solution conditions using a background of 140 mM K^{+ 24} and either 0.5 or 2.0 mM free Mg²⁺, characteristic of eukaryotic and prokaryotic divalent concentrations, respectively.^25–29 Data were fit according to a two-state model to calculate thermodynamic parameters and test for level of cooperativity.¹⁰ In this approach, the transition with the major absorbance change dominates the fit in a multi-transition system. The quality of the global fits can be found in Table S3.

Figure 1.

Wild-type and mutant constructs of tRNA^phe. Tertiary interactions are depicted on the WT construct with yellow lines, based on PDB 1ehz. Mutant constructs (M1–M6) vary sequence and base pairing strength in the tunable region of the acceptor stem (shaded blue) and have ‘ACCA’ at the 3’ end. Base-pairing regions that are different between mutants and WT are in black boxes. Colors of WT and mutant are maintained in all display items.

In 2.0 mM Mg²⁺, WT tRNA unfolds in a single sharp transition and was well fit to a two-state model suggesting cooperativity, wherein secondary structure unfolds concomitant with tertiary structure (Fig. 2A). The six acceptor stem mutants (M1–M6) were fit to the same two-state model. Their apparent melting temperatures (T_Ms) increase monotonically over a range of 11.4 °C, reflecting increased acceptor stem base pairing strength. Folding of low stability mutants, WT and M1, in dilute buffer is cooperative as confirmed through their highly negative ΔH_folding values of ~–80 kcal/mol (Table S1). However, the three highest stability mutants, M4–M6, display a broad unfolding transition and a smaller ΔH_folding, suggesting that secondary and tertiary structure do not unfold together (Fig. 2A). In particular, these mutants have ΔH_folding values of only ~–65 to ~–40 kcal/mol, reflecting fewer bonds broken in the major unfolding transition and thus multi-state folding. Loss of cooperativity is also mirrored in relative enthalpy values between mutant and WT (ΔH_M/ΔH_WT), which reveals fractional values as low as 0.5 as acceptor stem base pairing strength increases (Table S1). Similar effects are found in 0.5 mM Mg²⁺ (Table S2, Fig. S1). As such, the apparent T_M does not accurately reflect tertiary structure unfolding for the more stable mutants in dilute buffer conditions.

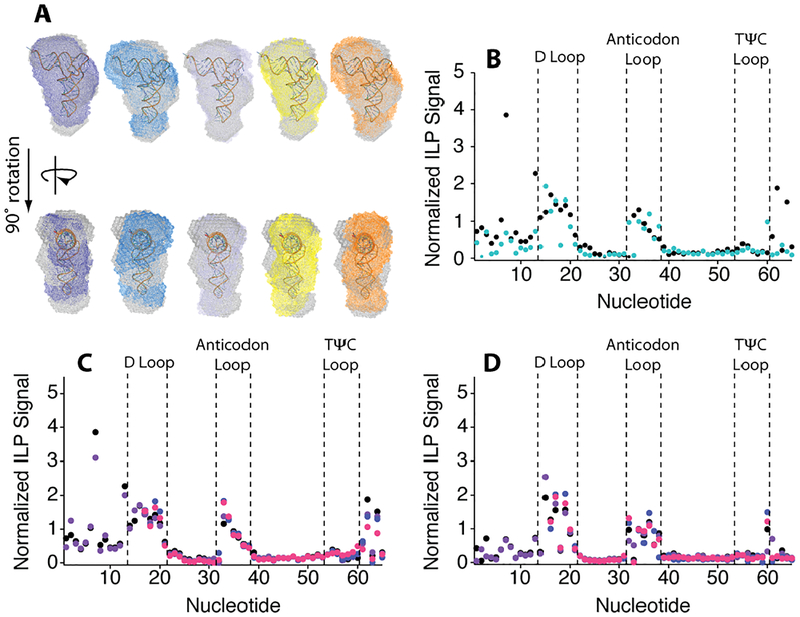
We verified that loss of cooperativity was not correlated with lack of native folding using small angle X-ray scattering (SAXS) to judge globular structure³⁰ and in-line probing (ILP) to assess local structure³¹. Overlay of WT and mutant SAXS scattering envelope is excellent, supporting retention of native structure (Fig. 3A). The SAXS structural parameters of P(r), R_g, and D_max, as well as excluded volume are similar for all constructs (Table S4, Fig. S2). Experimental scattering curves align well with the simulated scattering curve of tRNA^phe (PDB 1ehz) again supporting WT-like overall structure for all constructs (Fig. S3). Moreover, the ILP profiles for WT and M5, representing low and high stability constructs, were virtually identical and consistent with native secondary structure including the critical 5’ end of the RNA where the mutations reside, supporting the same native base pairing patterns (Figs. 3B, S4). Thus, base pair changes in the acceptor stem modulate tRNA stability and folding pathway without affecting the final structure.

Figure 3.

Small angle X-ray scattering bead models of M1–M5 aligned with the WT bead model and the tRNA crystal structure in buffer with 2.0 mM Mg²⁺. (A) Alignment of WT (grey) and M1 (purple), M2 (blue), M3 (light blue), M4 (yellow), and M5 (orange) DAMAVER envelopes. (B) Normalized ILP signal comparing WT (black) with M5 (teal) in buffer. Normalized ILP Signal of (C) WT and (D) M5 normalized ILP signal in (black) buffer, (purple) 20% PEG8000, (blue) Mg²⁺-chelated amino acids and (pink) 20% PEG8000 with Mg²⁺-chelated amino acids in the background of 2.0 mM free Mg²⁺. Nucleotides 1–15 were not analyzed in samples containing Mg²⁺-chelated amino acids due to salt contamination.

In Vivo-Like Conditions Favor Cooperative Folding and Thermostability with Stronger Base Pairing.

We tested effects of cellular crowding in 0.5 or 2.0 mM Mg²⁺, using 20% w/v PEG8000, and/or cellular levels of amino acids with weakly chelated Mg²⁺ (aaCM).¹³ Previous studies from our lab revealed that the cooperativity of WT tRNA folding is enhanced equally in diverse crowders, including 20% PEG4000, PEG8000, Dextran10, Dextran70, and Ficoll70;¹⁰ 20% PEG8000 is thus a representative choice. In the presence of 20% PEG8000 and 2.0 mM Mg²⁺, WT tRNA again unfolds in a single sharp transition, with a T_M of 68.0 °C, supporting cooperative folding (Fig. 2B). Indeed, the ΔH_folding of WT tRNA is slightly larger than in dilute buffer, by almost −10 kcal/mol (Table S1). The unfolding of mutant tRNAs was sensitive to crowding agent as well (Fig. 2B). Notably, the ΔH_folding values for the WT and the mutant tRNAs are similar in crowded conditions, indicating cooperative unfolding for WT and mutants. For example, WT and M5 have ΔH_folding values of −80.4 and −62.7 kcal/mol, respectively, and ΔH_M/ΔH_WT range from 1.1 to 0.8. Similar effects were found in 0.5 mM Mg²⁺ (Table S2). However, the T_Ms of the mutant tRNAs are relatively unchanged in crowder. For instance, the range in T_M is only 4.1 °C in crowder compared to 11.4 °C found without crowder (Table S1).

Next, we tested the stability of the tRNAs in amino acid-chelated Mg²⁺, which contains 2.0 mM free Mg²⁺ and 14.0 mM Mg²⁺ that is weakly chelated to a mixture of 96.0 mM L-glutamate, 4.3 mM L-aspartate, 3.8 mM L-glutamine, and 2.6 mM L-alanine (described in detail in the Materials and Methods)—the four most abundant amino acids in E. coli. This system mimics the one that is found naturally in bacteria.¹³ In aaCM, the observed T_M and ΔH are higher in magnitude than PEG8000, by ~3 °C and ~–70 kcal/mol, respectively (Fig. 2C). Although the T_Ms are generally higher and range over 6 °C, as secondary strength stability increases cooperativity is lost, with ΔH_mut/ΔH_WT as low as 0.6. In summary, neither crowded nor aaCM solutions alone have optimal properties for thermostability: crowding has modest affects on thermostability, while aaCM loses cooperativity.

Finally, we studied the combination of aaCM and crowding to test if the favorable effects of each were additive and to more closely mimic in vivo conditions. In this combination, we observed that RNAs maintain two-state folding over a wide range of thermostability (Fig. 4A). Strikingly, large gains in thermostability and relatively high folding cooperativity are observed for all but M6, the most stable mutant (Fig. 2D). We were interested in quantifying the extent of cooperativity change for each mutant. In a cooperative system, as secondary strength increases, ΔH_folding should become larger in magnitude since more hydrogen bonds are made. We constructed theoretical fully cooperative and non-cooperative ΔH_folding limits for the most biological condition of 20% PEG8000 and aaCM (Fig. 4B). The non-cooperative limit was calculated using nearest neighbor parameters for the acceptor stems of WT and mutant tRNA³², while the fully cooperative limit was calculated as the experimentally derived ΔH of WT (Table S1) plus the nearest neighbor model ΔΔH of the mutant acceptor stems, as described in the Materials and Methods.

Figure 4.

Melting temperature and enthalpy of folding of WT and mutant tRNAs in 2.0 mM free Mg²⁺ with 20% PEG8000 with Mg²⁺-chelated amino acids. (A) Melting temperature and (B) ΔH_folding of tRNA and mutants in 20% PEG8000 and additional Mg²⁺-chelated amino acids. See Materials and Methods for the calculation of (pink) non-cooperative ΔH and (purple) fully cooperative ΔH limits.

As depicted in Figure 4, constructs M1, WT, and M2 fit to a ΔH_folding very close to the fully cooperative limit. However, as acceptor stem strength increases the ΔH becomes somewhat less cooperative (Fig. 4). For M3–M5, maintain partial cooperative behavior. However, the strongest mutant, M6, unfolds in an almost fully non-cooperative manner, presumably because its all-GC acceptor stem unfolds subsequent to tertiary structure. Nonetheless, it is clear that strengthening base pairing in the acceptor stem leads to greater thermal stability for WT and M1–M5. When we look at less cellular solution conditions, cooperativity is lost at much lower secondary structure strength (Fig. S5). Finally, we note that the various crowded and aaCM conditions did not affect the global tRNA structure, as the ILP profiles for WT and M5 were unaffected by buffer, 20% PEG8000, aaCM, or a combination 20%PEG8000 and aaCM (Fig. 3C, D).

Evidence that Nature Selects for Thermostable tRNAs Through Strengthening Secondary Structure not Tertiary Structure.

Given that our above results show that increased secondary structure strength drives increased functional stability under biological conditions, we hypothesized that strong secondary structures may be found in functional RNAs from thermophiles owing to natural selection. We analyzed the following characteristics in a series of organisms with a wide range of optimal growth temperatures (OGT): genomic and rRNA GC percentage, ΔG of each stem in tRNA^phe, and average ΔG (ΔG_avg) of the four stems in tRNA^phe.

No observable trend was found between OGT and genome GC percent, where the R² of a linear fit is 0.063 (plotted as 100–GC percentage in Fig. 5A). This indicates that there is no underlying GC bias to the genome of thermophilic organisms. However, a strong positive linear correlation, with an R² of 0.73, exists between rRNA GC percent and OGT (plotted as 100–GC percentage in Fig 5B); clearly, organisms that grow optimally at higher temperatures have higher GC content in their rRNA, similar to previous reports.^33–35 Strikingly, linear trends also exist between OGT and a threshold ΔG_folding for tRNA acceptor, anticodon, and TΨC stems, as well as ΔG_avg; the R² for linear fits for these four plots are all at or above 0.85 (Fig. 5D–F, Fig. S6). In these plots, the term “threshold ΔG_folding” means that we average the five weakest ΔG_folding values within a given temperature bin, as defined in the Materials and Methods, which represent a minimal stability needed to maintain function (see Discussion). For the D stem, no correlation of ΔG and OGT was found; rather, we observed two sequence clusters, each of which is fairly weak (Fig. 5C). Notably, the D stem is the only stem in tRNA^phe that participates in tertiary interactions (Fig. 1), with three of its four base pairs engaged in such interactions. We hypothesize that the highly conserved tertiary contacts preclude variation of these nucleotides.

Figure 5.

Analysis of tRNA sequences from organisms with a large range of optimal growing temperatures. A comparison between optimal growth temperature and (A) whole genome GC percent, (B) ribosomal RNA GC percent, and the stability of the (C) D stem, (D) acceptor stem, (E) anticodon stem, (F) TψC stem. In panels B, D-F there are sequence bins based on OGT (pink dots) and threshold DGfolding lines (pink lines) for stem stability as a function of growth temperature, see Materials and Methods for further details.

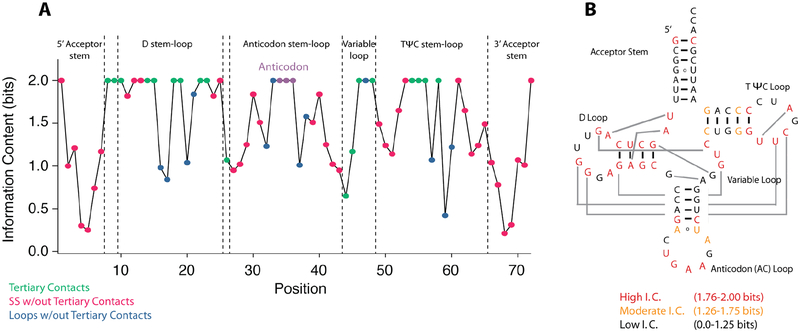
To look for variable residues, tRNA^phe sequences from 44 organisms were aligned and the information content, a measure of conservation, of each position in the RNA was calculated according to Shannon entropies.^{6, 36, 37} As described in the Materials and Methods, nucleotides that are highly conserved have 2 bits of information, nucleotides that are not conserved have 0 bits of information, while two nucleotides that co-vary with Watson-Crick base pairing share 2 bits of information. Strikingly, nucleotides that are involved in tertiary contacts have an information content close to 2 (Fig. 6A). There are eighteen nucleotides that participate in tertiary contacts and fifteen of these have 2 bits of information each. This suggests that strengthening of tertiary structure is not a route to thermal stability in tRNA.

Figure 6.

Information content of each position in tRNA^phe. (A) The information bits for each position of tRNA^phe. Positions are color coded as follows: tertiary contacts (green), secondary structure without tertiary contacts (pink), loops without tertiary contacts (blue), and the anticodon stem (purple). (B) Information content mapped onto the secondary structure of tRNA. Regions of high information content (1.76–2.00 bits), moderate information content (1.26–1.75 bits) and low information content (0.00–1.25 bits) are colored in red, orange, and black respectively. Joining lines between the D and TYC loops are passing behind the AC stem.

In contrast, nucleotides involved in secondary structure have moderate (1.26–1.75 bits) or low (0.00–1.25 bits) information content. This suggests that secondary structure base pairs tend to co-vary to maintain base pairing. When mapped onto the tRNA secondary structure, positions of lower information content and conservation cluster in the acceptor, anticodon, and TYC stems, with lowest information content in the acceptor stem where we made the mutants that were tested experimentally (Fig. 6B). In summary, bases with lower information content are found in the stems and tend towards higher GC content in thermophiles, while bases with higher information content are found in tertiary interactions and are not routes to thermal stability.

Discussion

RNA can have diverse functions including catalysis in ribozymes and small molecule binding in riboswitches to regulate gene expression. In addition, certain RNAs such as tRNA and rRNA have extensive tertiary structures and mediate protein expression. The pathways for adaption of these functional RNA to extreme temperatures has remained elusive. Since loss of tertiary structure leads to loss of function, one route to thermal stability might be strengthening of tertiary interactions. This could occur, for example, through enhanced metal ion interactions and long-range interactions. Such a means for stability was found in a SELEX experiment on a group I intron,³⁸ but the question remains as to how thermal stability is obtained during natural selection.

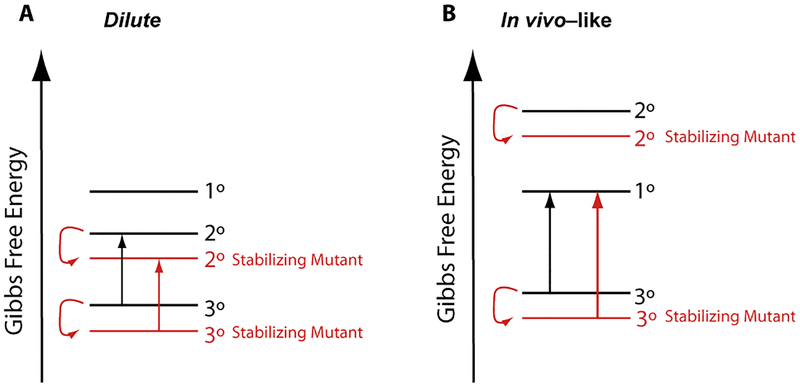
Proteins are known to fold cooperatively in nature, especially small compact proteins.^39,40 Less is known about whether RNAs fold cooperatively. Crothers and co-workers showed that tRNA can fold cooperatively in high Mg²⁺ conditions,⁴¹ and we found that yeast tRNA^phe folds cooperatively in crowded conditions that arises by both stabilization of tertiary structure and destabilization of secondary structure.^{10, 11} When model RNAs unfold cooperatively, strengthening of base pairing, even in positions without tertiary interactions, can contribute to thermostability.⁴² We thus entertained the notion that variations in stems apart from tertiary interactions could tune the temperature at which tertiary interactions in natural RNAs melt. Indeed, we found this to be the case. We found that strengthening the acceptor stem by changing G^•U and AU pairs to GC pairs led to greater thermal stability, but only when folding was cooperative, i.e. under cellular crowded conditions containing Mg²⁺-chelated amino acids. Figure 7 provides a conceptual framework for this observation using the Pan and Sosnick concept of functional free energy.⁹ Under dilute conditions, where folding is non-cooperative, strengthening of secondary structure leads to no change in the functional free energy since secondary structure is the penultimately stable state and also contained in the fully folded RNA. However, under in vivo-like conditions, where folding is cooperative, strengthening of secondary structure enhances the functional free energy since the unfolded RNA is the penultimately stable state.

Figure 7.

Conceptual free energy diagram of WT and stabilizing mutants under dilute and in vivo-like conditions. (A) In dilute solution conditions folding is non-cooperative, and stabilization of secondary structure does not increase cooperativity because the penultimate state is also stabilized. (B) In more in vivo-like conditions folding is cooperative, and stabilization of secondary structure leads to an increase in cooperativity because the penultimate state is primary structure, which does not change in stability.

These findings were corroborated by analysis of a database we constructed of tRNA^phe from 44 organisms that live at a broad range of temperatures. This analysis revealed that long-range tertiary interacting nucleotides have maximal information content and thus cannot change. With the exception of a single GC pair fixed toward the end of each helix, presumably present to stabilize the helix ends,⁴³ the regions of moderate information content were largely found to be in the stems (Fig. 6). Indeed, in nature the stems strengthen with growing temperature, suggesting that they provide a route to stability. Such base pairing changes can lead to several kcal/mol gain in free energy from just a single base change.⁴⁴ Thus, strengthening secondary structure of a cooperatively folding natural RNA provides a facile route to large effects in stability, albeit the sequence may be subject to other selection pressures such as serving as an identity element for aminoacylation. Moreover, observation that secondary structure increases with (OGT) in nature supports that tRNAs fold cooperatively in vivo in a diverse set of organisms.

Changing the tertiary structure of the RNA, rather than secondary structure, could be detrimental, given the generally greater molecular complexity of tertiary structure. For instance, new tertiary interactions in tRNA might interfere with the translation machinery. Additionally, observation that nucleotides involved in tertiary structure do not change with elevated growth temperature suggests that population of tertiary structure is relatively temperature independent or even endothermic; this contrasts sharply with secondary structure formation, which is strongly exothermic.⁶ Given that formation of tertiary structure is generally accompanied by water release,^{45, 46} tertiary structure formation may be entropically driven. Indeed, early studies show that metal binding to ATP is endothermic, presumably driven by the entropy gain from water release.⁴⁷ In addition, docking of the P1 helix into the catalytic core of the Tetrahymena ribozyme, which is mediated by 2’ hydroxyl tertiary interactions, is entropically favored with a ΔS ranging from +37 to +62 eu and a ΔH ranging from +8.5 to +19 kcal/mol.^{48, 49} The origin of this effect has been attributed to water release. The Woodson lab reported that the entropy of the unfolded state of Azoarcus ribozyme is decreased in the presence of crowding agents, which decreases the entropy loss upon tertiary structure docking.^{12, 50} In other cases, folding of other RNA tertiary interactions have been reported to be modestly enthalpically favored, indicating that the actual results will depend on the details of number and type of tertiary interactions.^51,52

The effects observed herein were lost with the most stable stem of all GC base pairs in M6. However, our experiments were conducted on tRNAs without the natural modifications. It is well established that modifications strengthen tertiary structure,^{53, 54} thus it is possible that even this mutant would fold cooperatively in this background. Hyperthermophiles tend to have more modified bases than mesophiles. These modifications are found in positions that participate in both secondary and tertiary structure, often strengthening contacts or increasing structure rigidity, suggesting that nature has multiple methods of selecting for RNA thermostability.^{55, 56} Thermostable proteins use several methods to improve stability, including more charged interactions, more disulfide bonds, and increasing rigidity.⁵⁷

In this study, we introduced the notion of threshold free energy, which we define as the minimal free energy needed to keep a helix folded. In other words, it is possible to have a helix with greater stability than the threshold but not less. A similar notion was used by Szostak and co-workers in describing the threshold information content needed to attain an RNA with a given function, such as catalytic rate.²³ Inspection of Figure 5 suggests that the threshold is approached by most sequences in all but the lowest growth temperatures presumably because of the entropy gain from lower information content. It remains to be explored whether similar principles apply to other functional RNAs. Our results suggest a simple mechanism for functional RNAs to adapt to higher temperatures by increasing the stability of secondary structure regions with low to moderate information content while maintaining tertiary contacts, structure, and stability.