DFT Fe_a3-O/O-O Vibrational Frequency Calculations over Catalytic Reaction Cycle States in the Dinuclear Center of Cytochrome c Oxidase

Abstract

Density functional vibrational frequency calculations have been performed on eight geometry optimized cytochrome c oxidase (CcO) dinuclear center (DNC) reaction cycle intermediates and on the oxymyoglobin (oxyMb) active site. The calculated Fe-O and O-O stretching modes and their frequency shifts along the reaction cycle have been compared with the available resonance Raman (rR) measurements. The calculations support the proposal that in state A[Fe_a3³⁺-O₂^−•⋯Cu_B⁺] of CcO, O₂ binds with Fe_a3²⁺ in a similar bent end-on geometry to that in oxyMb. The calculations show that the observed 20 cm⁻¹ shift of the Fe_a3-O stretching mode from state P_R to F is caused by the protonation of the OH⁻ ligand on Cu_B²⁺ (P_R[Fe_a3⁴⁺=O²⁻⋯HO⁻-Cu_B²⁺] → F[Fe_a3⁴⁺=O²⁻⋯H₂O-Cu_B²⁺]), and that the H₂O ligand is still on the Cu_B²⁺ site in the rR identified F[Fe_a3⁴⁺=O²⁻⋯H₂O-Cu_B²⁺] state. Further, the observed rR band at 356 cm⁻¹ between states P_R and F is likely an O-Fe_a3-porphyrin bending mode. The observed 450 cm⁻¹ low Fe_a3-O frequency mode for the O_H active oxidized state has been reproduced by our calculations on a nearly symmetrically bridged Fe_a3³⁺-OH-Cu_B²⁺ structure with a relatively long Fe_a3-O distance near 2 Å. Based on Badger’s rule, the calculated Fe_a3-O distances correlate well with the calculated ν_Fe-O^−2/3 (ν_Fe-O is the Fe_a3-O stretching frequency) with correlation coefficient R = 0.973.

1. Introduction

As the terminal oxidase of cell respiration, cytochrome c oxidase (CcO) reduces O₂ to H₂O and pumps protons across the membrane to create the chemiosmotic proton gradient used by ATP synthase to synthesize ATP.^1–4 Because of its important biological function, CcO has been extensively studied, and the overall structure of the molecule and several important intermediate states of the reaction cycle are established.⁵ X-ray crystallographic studies are critical in locating the redox-active sites and possible transport pathways for protons, molecular oxygen, and electrons in CcO.^6–16 The catalytic site of CcO which binds and reduces O₂ by 4e⁻/4H⁺ transfer contains a heme a₃ (Fe_a3) and a Cu ion (Cu_B). Fe_a3 and Cu_B are close to each other (~5 Å). This Fe_a3-Cu_B active site is usually called the dinuclear (or binuclear) center/complex (DNC or BNC). Two other redox centers are also present in CcO. One is a homodinuclear Cu dimer (2Cu_A) which serves as the initial site of electron entry to CcO,^17,18 and the other is also a heme, which is heme A (Fe_a) in the case of aa₃ type of CcO, or heme B (Fe_b) in ba₃ type of CcO. Electrons transfer from cytochrome c to Cu_A, then on to heme A/B, and from there to the DNC Fe_a3-Cu_B.^19,20 The DNC structures of aa₃ and ba₃ oxidases are nearly identical. Because of their considerable structural and functional similarities, these two types of enzymes probably have a common mechanism of O₂ reduction.⁴

Briefly, the iron in the Fe_a3 site is coordinated to heme and an axial histidine ligand (His384, residue numbers in this paper are by default for ba₃ CcO from Thermus thermophilus (Tt)), while the copper in the Cu_B site is coordinated to three histidine ligands: His233, His282, and His283. His233 covalently links with the Tyr237 side chain. This linkage is common to all CcO’s but otherwise unknown in metalloenzymes. This unique cross-linked tyrosine residue takes an important role in the processes of electron/proton transfer in CcO. The oxidation, spin, and ligation states of the Fe_a3 and Cu_B sites change during the catalytic cycle.

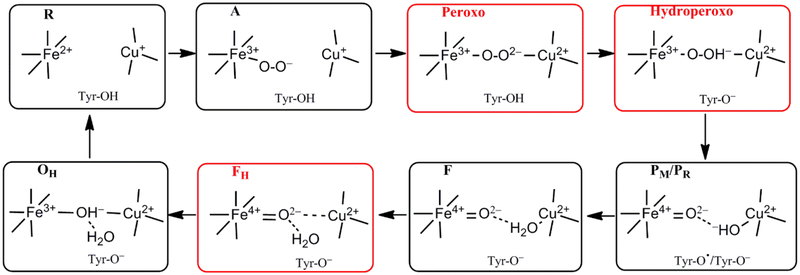
Starting from the binding of O₂ with the reduced (R) DNC, several catalytic intermediates have been well characterized by resonance Raman (rR) studies^21–25 (see Figure 1):

Figure 1.

Feasible intermediate states of the DNC in the catalytic cycle, in which A, P_M/P_R, F, and O_H were identified by resonance Raman (RR) experiments, and their DNC’s are likely in the forms presented above. Although the Peroxo, Hydroperoxo, and F_H states (drawn in red frames) were not observed experimentally, they may exist for a short time and will also be studied in the current paper.

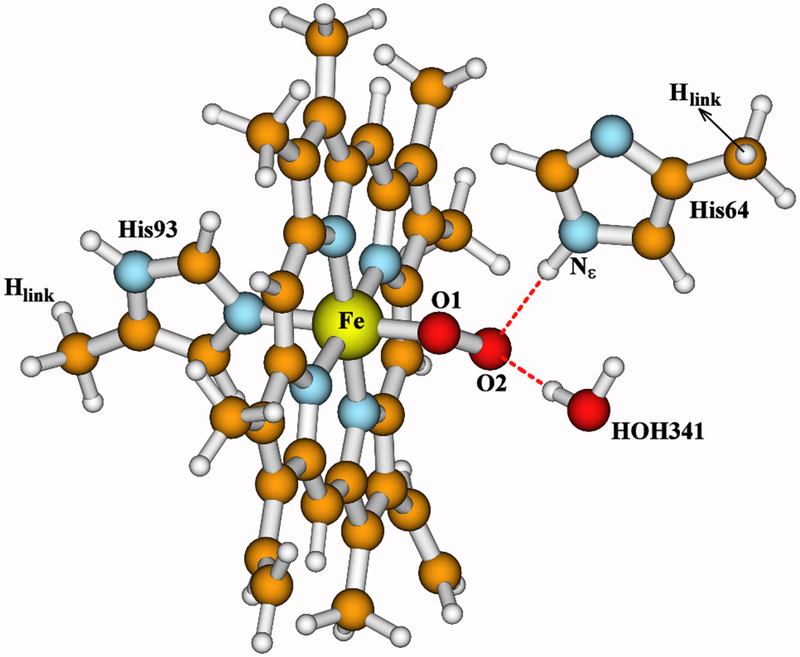
(1) State A. State A is formed when molecular O₂ binds with Fe_a3²⁺, and the DNC is formally in the Fe_a3³⁺-O₂^−•⋯Cu_B⁺ state.^5,22,26 This A-intermediate was identified by an Fe_a3-O₂ stretching mode at ~571 cm⁻¹ in rR measurements.^5,21–25,27 Since this band is located at nearly the same wavenumber position of oxymyoglobin (oxyMb) (569-572 cm⁻¹),^28–30 and since O₂ binds with Fe²⁺ in a bent end-on position in the X-ray crystal structures of oxyMb (see Figure 2),^29,31,32 it has been proposed that O₂ also binds with Fe_a3²⁺ in a similar bent end-on geometry in CcO (see Figure 3 for our DNC model of state A).

Figure 2.

Active site model of oxymyoglobin (oxyMb), built from the X-ray crystal structure 1MBO and geometry optimized using OLYP-D3-BJ functional within COSMO solvation model.³¹

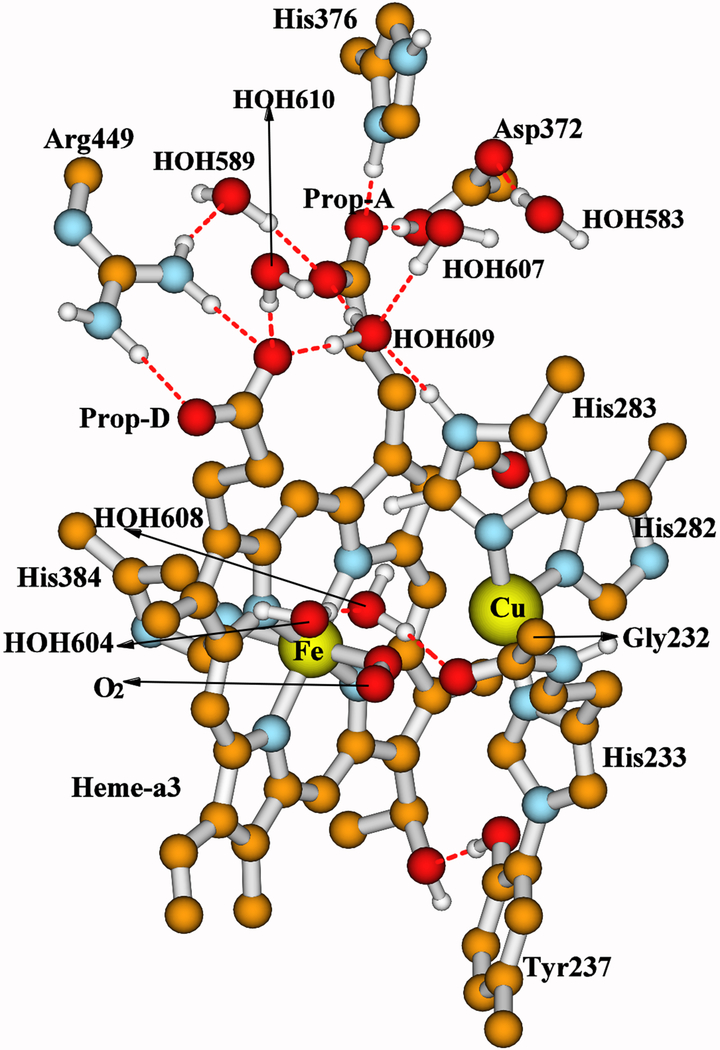
Figure 3.

Our whole quantum cluster model for state A of the DNC, built from the X-ray crystal structure 3S8G of ba₃ CcO from Thermus thermophilus (Tt).¹⁶ For more visibility, some apolar or link H atoms are not shown on the picture. A full picture (Figure S1) with all the H atoms is given in the Supporting Information. A clearer view for both the central Fe_a3³⁺−O₂^−•⋯Cu_B⁺ portion and the top cluster of the model are given in Figure 4.

(2) State P. This state is not a peroxide-containing compound (as implied in the notation), but one in which the dioxygen O-O bond has already been cleaved.^33–37 Four electrons need to transfer to O₂ for the O-O bond cleavage. It is well established that, among the four electrons, two are from the Fe_a3 site (Fe_a3²⁺ → Fe_a3⁴⁺) and one is from Cu_B (Cu_B⁺ → Cu_B²⁺). Experiments starting from the mixed valence state (2Cu_A^1.5+,Fe_a,b³⁺,Fe_a3²⁺,Cu_B⁺)^38–40 show that the 4^th electron originates from the unique cross-linked tyrosine (Tyr-O⁻ → Tyr-O^• radical).^41,42 The P state obtained this way is called P_M, which can be represented as [Fe_a3⁴⁺=O²⁻, OH⁻-Cu_B²⁺, Tyr-O^•]. When the O₂ binding starts from the fully reduced enzyme (2Cu_A⁺,Fe_a,b²⁺,Fe_a3²⁺,Cu_B⁺), an electron would be transferred from heme A/B, and the P-intermediate is called P_R[Fe_a3⁴⁺=O²⁻⋯HO⁻-Cu_B²⁺, Tyr-O⁻].⁴⁰ The Fe⁴⁺=O²⁻ stretching mode of the P_M intermediate was identified at ~804 cm⁻¹ by rR measurements.^{5,27,36,43–45} The optical spectrum of P_R was found similar or identical to the spectrum of the P_M intermediate.⁴⁰

Although no stable intermediates between A and P are observed, it is generally believed that the bridging ferric-peroxo [Fe_a3³⁺-O₂²⁻-Cu_B²⁺, Tyr-OH] and ferric-hydroperoxo [Fe_a3³⁺-(O₂H)⁻-Cu_B²⁺, Tyr-O⁻] states have to be formed before the O-O bond cleavage, and the proton in the bridging OOH⁻ originates from the unique cross-linked tyrosine.^2,46 Our recent broken-symmetry^47–49 density functional theory (DFT) calculations have shown that the O-O bond breaking energy barrier in the transition of [Fe_a3³⁺-(O-OH)⁻-Cu_B²⁺, Tyr-O⁻] → [Fe_a3⁴⁺=O²⁻⋯HO⁻-Cu_B²⁺, Tyr-O^•] is very small (less than 3.0/2.0 kcal mol⁻¹ in PW91-D3/OLYP-D3^50–53 calculations).⁵⁴

(3) State F is the next identified intermediate with the Fe_a3⁴⁺=O²⁻ stretching mode at ~785 cm⁻¹.^{5,27,36,44,55–58} F differs from P_R by an additional proton.^2,40,59,60 The observed EPR spectra for P_R and F are very different.⁴⁰ Hansson et al.³⁸ showed that, the copper hyperfine lines of the unique EPR signal of the P_R intermediate were broadened when ¹⁷O² was used as oxidant, and the broadening was consistent with bonding of one of the two oxygen atoms as an OH⁻ ligand of Cu_B²⁺. Morgan et al.⁴⁰ supported this proposal and further suggested that, in F, the copper OH⁻ ligand may have been protonated to water, allowing for strong hydrogen bonding and exchange coupling between the Fe_a3⁴⁺ and Cu_B²⁺ sites. Therefore, it is usually modeled that the Cu_B site has an OH⁻ ligand in P_M/P_R[Fe_a3⁴⁺=O²⁻⋯HO⁻-Cu_B²⁺, Tyr-O^•/Tyr-O⁻], and an H₂O ligand in F[Fe_a3⁴⁺=O²⁻⋯H₂O-Cu_B²⁺, Tyr-O⁻].^27,59

Sharma et al. have proposed that the water ligand in F likely dissociates from the copper (they call the state F_H).⁶⁰ However, the F_H state they obtained from their DFT calculations has higher energy than the prior state with the H₂O ligand on Cu_B.⁶⁰ Very recently, we have also found in our DFT calculations that, the DNC structure of F[Fe_a3⁴⁺=O²⁻⋯H₂O-Cu_B²⁺, Tyr-O⁻] (labeled as Fe_a3⁴⁺=O²⁻⋯H₂O-Cu_B²⁺-Y237⁻-H376⁺-M2in Ref. 61) has more Cu_B⁺-Tyr-O^• character and therefore has a relatively long Cu_B-O distance (2.26 Å). When purposely moving the H₂O ligand away from the Cu_B site (the H₂O still H-bonds with O²⁻), we have found a lower-energy (by 6 kcal mol⁻¹) structure (Fe_a3⁴⁺=O²⁻⋯Cu_B²⁺-Y237⁻-H376⁺-M3 in Ref. 61), in which the Cu_B²⁺⋯O²⁻ (ferryl-oxygen) distance is only around 2.4 Å. We therefore have also proposed that the H₂O ligand has dissociated from Cu_B in state F.⁶¹ However, anticipating later results, in Section 3.2 we will show from our vibrational frequency calculations that the H₂O ligand still binds with Cu_B²⁺ in the rR identified F state. The H₂O-dissociated F structure will also be called “F_H” hereafter (see Figure 1).

(4) A rR band at 356 cm⁻¹ was observed and was suggested to be the His-Fe_a3⁴⁺=O²⁻ bending mode for a DNC structure between states P_R and F.^5,62,63

(5) Next, a very important activated oxidized Fe_a3³⁺⋯Cu_B²⁺ state beyond state F, which is called O_H, has been identified with a high-spin Fe_a3³⁺ and with the Fe_a3³⁺-OH⁻ stretching mode at 450 cm⁻¹.⁵⁶ Such a stretching frequency is very low with respect to other hydroxide bound heme species.²⁷ Therefore, it has been suggested that there is a strong H-bond to the oxygen atom of the heme-bound hydroxide, thereby weakening the Fe_a3-O bond and generating a high-spin configuration.²⁷ However, a feasible alternative is that the O_H state has a bridging OH⁻ in the Fe_a3³⁺-OH⁻-Cu_B²⁺ form.^60,61,64 And since the O²⁻ ligand already bridges the two metal sites in F_H, once the F_H state is formed, the O_H state with bridging OH⁻ is readily formed upon one electron reduction and proton transfer to F_H.

Although the intermediate states A, P_M/P_R, F, and O_H mentioned above were identified well by rR measurements, the detailed DNC structures of these states are still not certain. In the current paper, we apply vibrational frequency calculations on our geometry optimized DNC structures for the intermediate states and compare the calculated Fe_a3-O₂/Fe_a3-O vibrational frequencies and related frequency shifts with the available observed data from rR experiments.

2. Calculation Methods

The starting geometries of the model clusters for the DNC intermediate states are established based on the ba₃ CcO X-ray crystal structure 3S8G.¹⁶ The calculation method for geometry optimizations using ADF^65–67 is the same (broken-symmetry^47–49/OLYP-D3-BJ⁶⁸/TZP/DZP plus COSMO^69–72 solvation model) as in our recent publication,⁶¹ and the geometry optimized structures of states P_R(Fe_a3⁴⁺=O²⁻⋯HO⁻-Cu_B²⁺, Tyr-O⁻), F(Fe_a3⁴⁺=O²⁻⋯H₂O-Cu_B²⁺, Tyr-O⁻), F_H(Fe_a3⁴⁺=O²⁻⋯Cu_B²⁺, Tyr-O⁻), and O_H[Fe_a3³⁺-OH⁻-Cu_B²⁺, Tyr-O⁻] are directly taken from Ref. 61 for vibrational frequency calculations in the current paper. More details of our geometry optimization and vibrational frequency calculation methods can be found in Supporting Information.

For comparison, both analytical and numerical vibrational frequency calculations are then performed at the optimized geometries. For each DNC intermediate structure, we compute a partial Hessian (~110 atoms) in analytical frequency calculations, in which all the methyl groups and the atoms in the upper cluster (Arg449, His376, Asp372, HOH583, HOH589, HOH607, HOH610, and the shifted waters HOH604 and HOH608 in states F, F_H, and O_H) are excluded from Hessian calculations. In numerical frequency calculations, we applied the mobile block Hessian (MBH) approach built in ADF,^73,74 in which the following groups were treated as individual mobile blocks: each methyl group in the model cluster, each residue side chain of Arg449, His376, and Asp372, and each of the water molecules which are also excluded from analytical Hessian calculations mentioned above. In the MBH method, each mobile block (for example, a methyl group or a water molecule) is treated as a rigid block, which moves as a whole and has only six frequencies related to its rigid motions. We will first apply the analytical full/partial Hessian and numerical full/MB Hessian vibrational frequency calculations at the fully/partially optimized geometries of the oxyMb active site model (Figure 2) to establish the accuracy of these different approaches for the Fe-O/O-O stretching modes.

3. Results and Discussion

3.1. Calculations on the OxyMb Active Site

Our active site model of oxyMb is taken from the X-ray crystal structure 1MBO (1.6 Å resolution).³¹ The molecular oxygen (O1-O2) H-bonds with the −N_εH group of the distal His64 side chain.³² A water molecule HOH341 found near the O2 atom in 1MBO is also included in our model. Overall, our model includes the heme Fe atom, the molecular oxygen (O1-O2), the porphyrin with truncated propionate groups, the water molecule HOH341, and the side chains of His93 and His64 in which each C_α atom is replaced by an H_link atom. To compare different geometry optimization and vibrational frequency calculation methods, we have performed the following calculations at the oxyMb model: 1) Similar to the DNC model calculations, the geometry of the oxyMb active site model is partially optimized with the positions of the H_link atoms on His93 and His64 fixed (H_link-Fixed). 2) The geometry of this model is fully optimized (Full-Opt) without any constraint. 3) Analytical full-Hessian (Anal. Full-H) vibrational frequency calculations are performed at the two optimized geometries. 4) Analytical partial-Hessian (Anal. Partial-H) vibrational frequency calculations are performed at the two optimized geometries, in which the atoms in all the methyl groups are excluded from the Hessian calculations. 5) Numerical full-Hessian (Num. Full-H) frequency calculations are performed. And 6) numerical MBH calculations are applied at the two optimized geometries, where each methyl group is treated as a rigid mobile block (MB).

The calculated broken-symmetry (BS) state relative energies, the main geometric and Mulliken net spin population properties of the two optimized geometries are given in Table 1. Whether the heme-oxygen complex in oxyMb or oxyhemoglobin is in the Fe²⁺-O₂, or in the Fe³⁺-O₂^−• form, or in the mixture of both forms has a long history of discussion and investigation,^75–78 and an extended discussion is not within the scope of the current paper. Briefly, the net spin results of our calculations show that the oxyMb complex is better described as in the Fe³⁺-O₂^−• state, in which the low-spin (LS) Fe³⁺ site antiferromagnetically (AF) coupled with the superoxide ion O₂^−•. The distance between O2 and the oxygen atom of HOH341 is only 1.47 Å in the X-ray crystal structure 1MBO, which is too short for H-bonding interaction. After DFT geometry optimizations, this distance is increased to 2.84-2.85 Å. The Full-geometry optimization yields a little lower energy (by 1.1 kcal mol⁻¹) than the H_link-Fixed geometry. Yet, the main bond lengths and angles in the Fe-O₂ center of the two optimized geometries are nearly the same.

Table 1 .

DFT OLYP-D3-BJ Calculated Geometric and Mulliken Net Spin Properties of the Active Site of OxyMb (Figure 2).^a

	Geometry (Å, degree)						ΔEBS b	Net Spin c
	Fe-N(H93)	Fe-O1	O1-O2	∠FeO1O2	O2⋯Nε(H64)	O2⋯O341		Fe	O1	O2
	Hlink-Fixed d	2.01	1.79	1.32	117.7	2.85		2.85	0.0	0.71	−0.32	−0.40
Full-Opt. e	2.02	1.80	1.32	117.3	2.87	2.84	−1.1	0.73	−0.33	−0.41
Exp.	2.07	1.83	1.21	115.6	2.77	1.47

^a.The initial geometry of the oxymyoglobin (oxyMb) active site model cluster is taken from the X-ray crystal structure 1MBO,³¹ which is also given here as the experimental (Exp.) data for comparison.

^b.Relative calculated broken-symmetry state energies in kcal mol⁻¹.

^c.The Mulliken net spin populations (number of unpaired electrons) on Fe, O1, and O2.

^d.During geometry optimization, the positions of the H_link atoms (replacing the C_α atoms) on His93 and His64 side chains are fixed.

^e.The geometry of the model cluster is fully optimized without constraint.

Our calculated and experimentally observed Fe-O and O-O stretching modes are compared in Table 2. Overall, for this small oxyMb active site model, different vibrational frequency calculation methods yield very similar ν(Fe-O) (523 – 533 cm⁻¹) and ν(O-O) (1083 – 1090 cm⁻¹) frequencies. When focusing on the ν(Fe-O) calculated results at the two optimized geometries (H_link-Fixed vs. Full-Opt), one can see that each calculation method yields almost the same frequencies (529 vs. 528 cm⁻¹, 524 vs. 523 cm⁻¹, 533 vs. 530 cm⁻¹, and 531 vs. 529 cm⁻¹ from the methods Anal. Full-H, Anal. Partial-H, Num. Full-H, and Num. MBH, respectively). Therefore, fixing the two H_link atom positions during geometry optimization has little effect on the Fe-O bond length and the Fe-O stretching mode. Further, The ν(Fe-O) obtained from Anal. Partial-H calculation is different from the Anal. Full-H calculation by only 5 cm⁻¹ for both geometries. And the Num. MBH calculated ν(Fe-O) (531 cm⁻¹ for H_link-Fixed geometry and 529 cm⁻¹ for Full-Opt geometry) is almost the same as the corresponding Num. Full-H result (533 cm⁻¹ for H_link-Fixed geometry and 530 cm⁻¹ for Full-Opt geometry). Therefore, excluding all the methyl groups or treating each methyl group as a mobile block in Hessian calculations in analytical or numerical vibrational frequency calculations also has little effect on the calculated Fe-O stretching mode in our oxyMb model. Similar conclusions can also be made for the O-O stretching mode calculations here.

Table 2.

DFT OLYP-D3-BJ Calculated and Experimentally (Exp.) Observed Fe-O and O-O Vibration Frequencies in the Active Site of OxyMb.^a

	ν(Fe-O) (cm−1) d				ν(O-O) (cm−1) d
	Anal. Full-H	Anal. Partial-H	Num. Full-H	Num. MBH	Anal. Full-H	Anal. Partial-H	Num. Full-H	Num. MBH
	Hlink-Fixed b	529	524	533	531	1083	1084	1087	1085
Full-Opt. c	528	523	530	529	1090	1089	1090	1088
Exp.	569,28 570,28 571,29 57230				1103 95

^a.See Table 1 for geometrical properties.

^b.During geometry optimization, the positions of the H_link atoms (replacing the C_α atoms) on His93 and His64 side chains are fixed.

^c.The geometry of the model cluster is fully optimized without constraint.

^d.Frequency calculations are performed with four methods: (1) the exact full Hessian matrix is calculated analytically (Anal. Full-H); (2) the exact full Hessian matrix is calculated numerically (Num. Full-H); (3) a partial Hessian matrix excluding all methyl groups is calculated analytically (Anal. Partial-H); and (4) a Hessian matrix in which each methyl group is treated as a mobile block is calculated numerically (Num. MBH).

Comparing with the experimental data, our calculations underestimate the ν(Fe-O) frequency in average by ~43 cm⁻¹, and underestimate the ν(O-O) mode by only ~17 cm⁻¹. Other DFT functionals like BP86 may increase the calculation accuracy for the ν(Fe-O) mode by ~20 cm⁻¹.⁷⁹ However, it overestimates the ν(O-O) mode by ~100 cm⁻¹.⁷⁹ Therefore, when we compare our calculated Fe-O/O-O frequencies with the observed values, we will focus more on the relative calculated frequencies or frequency shifts than on the absolute frequencies. Next, we move on to the CcO DNC model calculations and will first compare if the calculated ν(Fe-O) frequency in state A is similar to the calculated results in oxyMb.

3.2. Calculations on the CcO DNC Models

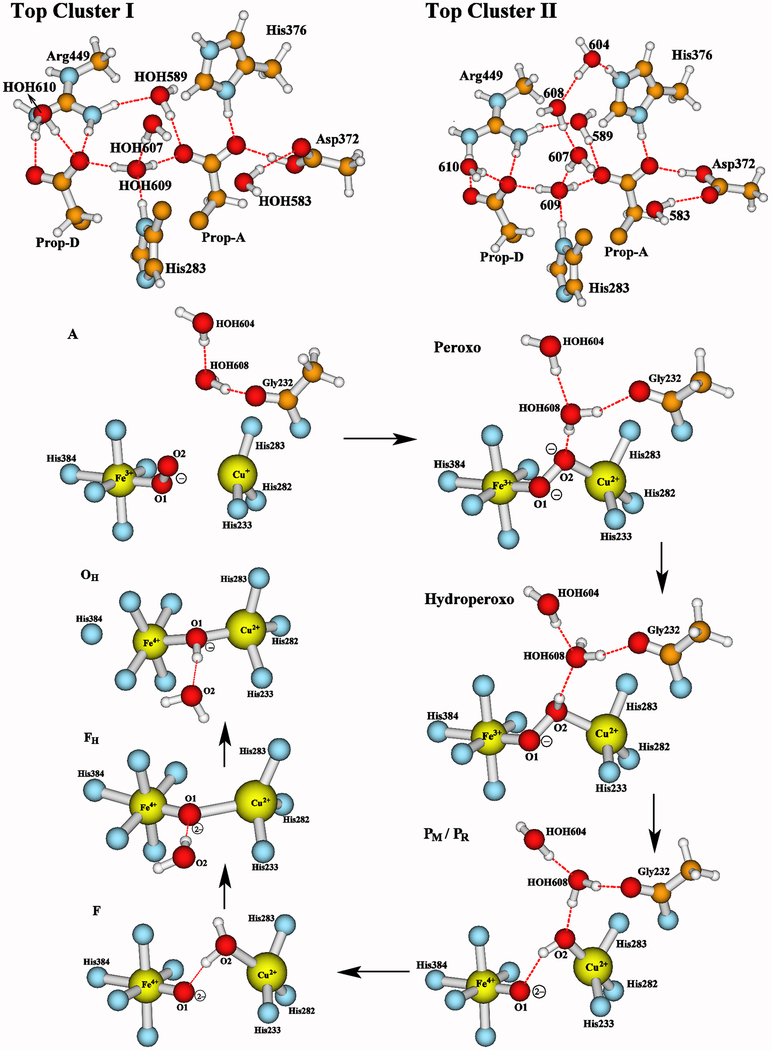
The detailed structures of the Fe_a3⋯Cu_B center and the locations of the two water molecules HOH604 and HOH608 (see the caption of Figure 4) change during the reaction cycle. However, the overall structure of each DNC cluster studied here is similar to the whole structure of state A shown in Figure 3. Therefore, for clarity, only the central and the top portions of the DNC cluster for each state are shown in Figure 4.

Figure 4.

A closer look at the central and the top portions of the DNC model clusters studied here. Our recent calculations⁶¹ have shown that the two water molecules HOH604 and HOH608 found near the DNC in the X-ray crystal structure 3S8G are likely to shift to the top of the water cluster (see Top Cluster II) during the P_R → F transition. Therefore, in A, Peroxo, Hydroperoxo, P_M and P_R states, these two water molecules are within the DNC and the top portion of each cluster with the H-bonding interactions is shown in “Top Cluster I”. In the following states F, F_H, and O_H, these two water molecules are shifted to the top of the cluster as shown in “Top Cluster II”. Our previous pK_a calculations^54,61 have indicated that the His376 side chain is likely in the cationic protonated state in these DNC clusters, and the Tyr237 side chain is in neutral protonated state in A and Peroxo intermediates, and in ionic deprotonated state in Hydroperoxo, P_R, F, F_H, and O_H intermediates (the proton on the heme-a₃ farnesyl hydroxyl group is rotated to have H-bonding interaction with the Tyr237⁻ side chain). In P_M, the Tyr237 side chain is in the Tyr237-O^• radical state. The rest of each model is similar to what was shown in Figure 3.

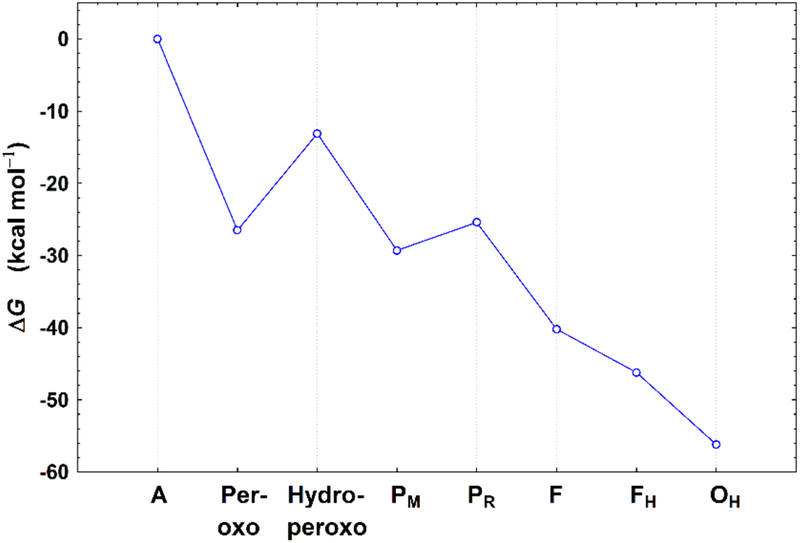
The calculated bond distances, the estimated relative free energies, and Mulliken net spin population properties of the optimized geometries for the A, Peroxo, Hydroperoxo, P_M, P_R, F, F_H, and O_H states are given in Table 3. The negative sign of the net spin on Cu_B indicates the spins of the Fe_a3 and Cu_B sites are antiferromagnetically (AF) coupled. Since the broken-symmetry state is a mixture of pure spin states,^47–49 we have calculated the spin projection energy corrections^80–82 for each state, and have estimated the free energy changes at pH = 7 with the corrections of the zero point energy differences (ΔZPE) and with the reference to a typical cytochrome c redox potential of E⁰ = +0.22 eV.⁶¹ The calculated spin projection corrections (ΔE_corr) range from −2.1 to 0.1 kcal mol⁻¹ for the eight DNC states (see Table S1). The ΔG change from A → O_H is shown in Figure 5. A detailed description of the spin-projection correction calculations and the analysis of the ΔG profile are given in the Supporting Information.

Table 3.

OLYP-D3-BJ Calculated Geometrical, Energetic, and net Spin Properties of the Optimized DNC Geometries in the Following Sates: A, Peroxo, Hydroperoxo, P_M, P_R, F, F_H, and O_H.

State	Structure a	Geometry (Å)					ΔG c	Q d	Net Spin e
		Fe-N(H384)	Fe-O1	O1-O2 (O1⋯O2)	Cu-O b	Fe⋯Cu			Fea3	O1	O2	CuB	Y237
		A	Fea33+,LS-O2−•⋯-CuB+,Y-OH	2.15	1.82	1.30			2.95	4.55	0.0	1	0.96	−0.30	−0.51	−0.06	0.0
Peroxo	Fea33+,LS-O22−-CuB2+,Y-OH	2.12	1.80	1.37	1.94	3.88	−26.5	1	0.74	−0.02	−0.21	−0.34	0.0
Hydroperoxo	Fea33+,LS-−OOH-CuB2+,Y-O−	2.07	1.78	1.50	2.11	4.31	−13.1	1	0.88	0.13	−0.06	−0.34	−0.39
PM	Fea34+,IS=O2−⋯HO−-CuB2+,Y-O•	2.10	1.65	2.68	1.90	4.28	−29.3	1	1.29	0.75	−0.21	−0.50	−0.90
PR	Fea34+,IS=O2−⋯HO−-CuB2+,Y-O−	2.09	1.65	2.67	1.91	4.42	−25.4	0	1.28	0.77	−0.21	−0.50	0.0
F	Fea34+,IS=O2−⋯H2O-CuB2+,Y-O−	2.10	1.66	2.52	2.26	4.58	−40.2	1	1.37	0.69	−0.01	−0.36	−0.41
FH	Fea34+,IS=O2−⋯CuB2+,Y-O−	2.15	1.68		2.40	3.81	−46.2	1	1.37	0.67		−0.22	−0.59
OH	Fea33+,HS-OH−-CuB2+,Y-O−	2.43	1.98		1.99	3.58	−56.2	1	4.02	0.13		−0.37	−0.25

^a.LS stands for low-spin, IS is for intermediate-spin, and HS is for high-spin. In state A, the LS Fe_a3³⁺ site is AF-coupled to the O₂^−• radical, and in other states given here, the Fe_a3 site AF-couples to the Cu_B site.

^b.In states A, F_H, and O_H, this is the distance between Cu_B and O1 (see Figure 4). In other states, this is the Cu_B-O2 distance.

^c.Calculated relative free energies at pH = 7 (kcal mol⁻¹), including the spin-projection corrections to the broken-symmetry state energies, the zero-point energy differences, and with the reference to a typical cytochrome c redox potential of E⁰ = +0.22 eV (see details in Supporting Information).

^d.The net charge of the model cluster.

^e.The Mulliken net spin populations (number of unpaired electrons) on Fe_a3, O1, O2, and Cu_B, and on the heavy atoms of the Tyr237 (Y) side chain (the sum total).

Figure 5.

ΔG change from A → O_H (see Supporting Information for detailed analysis).

The calculated vibrational Fe_a3-O and O-O stretching modes and the O-Fe_a3-Porphyrin bending modes in both Analytical partial-Hessian (Anal. Partial-H) and numerical mobile block Hessian (Num. MBH) methods are given in Table 4 and are compared with the available experimental data observed from rR frequency measurements.

Table 4.

Calculated Fe_a3-O and O-O Mayer Bond Orders (MBO) and Comparison of the Calculated and Observed (Exp) Vibrational Frequencies (cm⁻¹) of the Fe_a3-O and O-O Stretching Modes and the O-Fe_a3-Porphyrin Bending Modes in CcO DNC Sates A, Peroxo, Hydroperoxo, P_M, P_R, F, F_H, and O_H.^a

State	Structure	Fe-O MBO	ν(Fe-O)			O-O MBO	ν(O-O) or ν(O-Fe-Por) c
			Anal. Partial-H	Num. MBH	Exp b		Anal. Partial-H	Num. MBH	Exp
A	Fea33+,LS-O2−•⋯-CuB+,Y-OH	0.480	518	520	57121−24	1.282	1142	1145	1159,d 1148e
Peroxo	Fea33+,LS-O22−-CuB2+,Y-OH	0.544	577	584		1.063	906	907	870f
Hydroperoxo	Fea33+,LS-−OOH-CuB2+,Y-O−	0.586	666	672		0.911	664	673
PM	Fea34+,IS=O2−⋯HO−-CuB2+,Y-O•	1.191	838	830	80443−45		357	367
PR	Fea34+,IS=O2−⋯HO−-CuB2+,Y-O−	1.196	844	842	80440		378	372	356g
F	Fea34+,IS=O2−⋯H2O-CuB2+,Y-O−	1.144	823	822	78536,44,55–58		374	381	356g
FH	Fea34+,IS=O2−⋯CuB2+,Y-O−	1.118	775	774			375	396
OH	Fea33+,HS-OH−-CuB2+,Y-O−	0.518	452/458	471/476	45056

^a.Other calculated properties of these structures are given in Table 3. The vibrational frequencies were calculated with two methods: analytical frequency calculations with partial Hessian (Anal. Partial-H) and numerical frequency calculations with mobile block Hessian (Num. MBH).

^b.Different groups^21–24 reported a little different values of the Fe_a3-O stretch modes (cm⁻¹) observed from resonance Raman (rR) experiments for a certain state. For simplicity, we only cite the commonly agreed values used in the review article of Ref. 5.

^c.These are the O-O stretch modes for states A, Peroxo, and Hydroperoxo. For other states, these are the O-Fe-Porphyrin bending modes.

^d.O-O stretching mode observed in a picket-fence oxyporphyrin.⁸³

^e.O-O stretching mode observed in a cobalt-superoxide complex CoO₂/Cu[NMePr]⁺.^84,85

^f.O-O stretching mode observed in a synthetic Fe³⁺-O₂²⁻-Cu²⁺ complex, which is structurally analogous to the Peroxo state of the DNC.⁸⁶

^g.A rR band that was observed and was suggested to be the His-Fe_a3⁴⁺=O²⁻ bending mode for a DNC structure between states P_R and F.^5,62,63 Our calculations show that this is likely an O-Fe_a3-Porphyrin bending mode.

For a certain structure, there can be multiple vibrational modes containing Fe_a3-O displacements. These modes are normally next to each other and are of very similar frequencies. The Fe_a3-O stretching frequencies reported here are those having the largest Fe_a3-O stretching displacements. Overall, the two calculation methods predicted similar frequencies for a certain vibrational mode. The largest difference yielded by the two calculation methods is ~20 cm⁻¹ for the O-Fe_a3-Porphyrin bending mode in F_H and the Fe_a3-O stretching mode in O_H. For the rest of the vibrational modes given in Table 4, the differences between the two calculation methods are less than 10 cm⁻¹. We will therefore focus on the data obtained from the Analytical partial-Hessian calculations in the following discussions.

Our calculated A state is best described as Fe_a3³⁺-O₂^−•, in which the low-spin Fe_a3³⁺ site AF-coupled with the superoxide ion O₂^−•, similar to the oxygen adduct in oxyMb. In state A, the calculated Fe-O distance is by 0.02/0.03 Å longer and the O-O distance is by 0.02 Å shorter than the corresponding calculated distances in oxyMb, likely because there are H-bonding interactions between O₂^−• and His64/HOH341 in oxyMb. As a result, the calculated ν(Fe-O) stretching mode in A (518 cm⁻¹) is by ~10 cm⁻¹ lower than the average (528 cm⁻¹) of the calculated ν(Fe-O) in oxyMb. However, considering 10 cm⁻¹ is only 2% of 528 cm⁻¹, and different calculation methods on the oxyMb active site can yield up to 10 cm⁻¹ difference for the ν(Fe-O) mode, we conclude that the calculated ν(Fe-O) in state A of CcO and in oxyMb are nearly the same. Further, the calculated ν(Fe-O) mode in the Peroxo state (577 cm⁻¹) is considerably larger (by ~50 cm⁻¹) than the ν(Fe-O) stretch frequency in oxyMb. Therefore, our calculations confirm that the experimentally observed state A of CcO is not in the peroxo state, but has a similar O₂ end-on structure as in oxyMb.

There are no experimental data for the O-O stretching modes available for the DNC of CcO. However, our calculated ν(O-O) for the superoxide in state A (1142 cm⁻¹) is very close to the observed O-O stretching mode 1159 cm⁻¹ observed in a picket-fence oxyporphyrin⁸³ and to the 1148 cm⁻¹ observed in a cobalt-superoxide complex CoO₂/Cu[NMePr]⁺.^84,85 The O-O stretching mode in a synthetic Fe³⁺-O₂²⁻-Cu²⁺ complex that is structurally analogous to the Peroxo state of the DNC was reported by Adam et al. at 870 cm⁻¹,⁸⁶ which is reasonably close to our calculated ν(O-O) (906 cm⁻¹) for the Peroxo state.

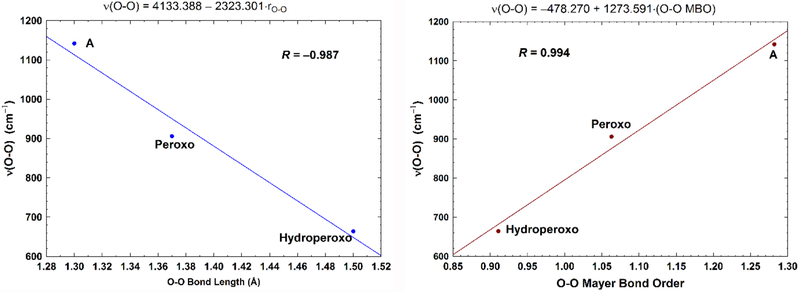
Further, we have calculated the Fe_a3-O and O-O Mayer bond orders (MBO)⁸⁷ and have given the results in Table 4. Our calculated Mayer O-O bond orders for the superoxide in state A, the Peroxo state, and the Hydroperoxo state are 1.28, 1.06, and 0.91, respectively, which agree with the calculations by Cramer et al⁸⁸ (on side-on bound metal-O₂ complexes) very well, and are consistent with the increase of the corresponding Fe_a3-O bond orders (0.48, 0.54, and 0.59, respectively). Strong correlation has been shown between the O-O bond lengths/orders and the O-O stretching frequencies.⁸⁸ We therefore also performed the linear regression between both the O-O bond length and bond orders vs. the O-O stretching frequencies, although for only three points. The correlation coefficients are near unity (in absolute value) in both fittings with R = −0.987 and R = 0.994, respectively (see Figure 6). Very recently, Dey’s group has also shown the linear correlation between Fe-O and O-O stretching frequencies in different superoxide adducts of porphyrins, heme enzymes, and in several model systems.⁸⁹

Figure 6.

Left: Correlation between the calculated (using analytical partial-Hessian method) O-O stretching frequencies (ν(O-O), cm⁻¹) and the O-O bond lengths (r_O-O, Å). Very similar fitting is obtained with R = −0.985, when using the calculated frequencies obtained from the numerical-MBH method. Right: Correlation between the calculated (using analytical partial-Hessian method) O-O stretching frequencies (cm⁻¹) and the Mayer O-O bond orders (MBO). Very similar fitting is also obtained with R = 0.995, when using the calculated frequencies obtained from the numerical-MBH method.

Experimentally it has been observed that the optical spectrum of P_R is similar to or identical to the spectrum of the P_M intermediate.⁴⁰ Our calculations indeed yield very similar Fe_a3-O stretching frequencies in P_R and P_M (844 vs. 838 cm⁻¹). From P_R to F, rR measurements show that the ν(Fe-O) shifts from 804 cm⁻¹ to 785 cm⁻¹.^{5,27,36,44,55–58} This ~20 cm⁻¹ shift was suggested being caused by the protonation of the copper OH⁻ ligand in the transition of P_R[Fe_a3⁴⁺=O²⁻⋯HO⁻-Cu_B²⁺] → F [Fe_a3⁴⁺=O²⁻⋯H₂O-Cu_B²⁺].⁴⁰ Our recent calculations have shown that the DNC of F_H (see Figure 4) with the H₂O ligand dissociating from the Cu_B²⁺ site yields lower-energy (by 6 kcal mol⁻¹) than the F state with H₂O ligand on Cu_B²⁺.⁶¹ Therefore we have proposed that the H₂O ligand might have dissociated from Cu_B in state F.⁶¹ However, our current calculated ν(Fe-O) shift from P_R (844 cm⁻¹) to F_H (775 cm⁻¹) is ~70 cm⁻¹, which is much larger than the observed value of ~20 cm⁻¹. On the other hand, from P_R to F (where the H₂O ligand is still on Cu_B²⁺), our calculations yield 21 cm⁻¹ ν(Fe-O) shift (844 cm⁻¹ → 823 cm⁻¹), which is in excellent agreement with the observed value. Therefore, our current vibrational frequency calculations support that state F is in the [Fe_a3⁴⁺=O²⁻⋯H₂O-Cu_B²⁺] structure, where the H₂O ligand is still on the Cu_B²⁺ site. This does not exclude the existence of state F_H. F_H is possibly the precursor of O_H, but with a very short life time. Experimentally, a low Fe_a3-O stretching frequency of 450 cm⁻¹ was observed for state O_H.⁵⁶ Our calculations on the O_H[Fe_a3^3+,HS-OH⁻-Cu_B²⁺, Tyr-O⁻] DNC structure reproduce this ν(Fe-O) frequency (452 cm⁻¹) very well.

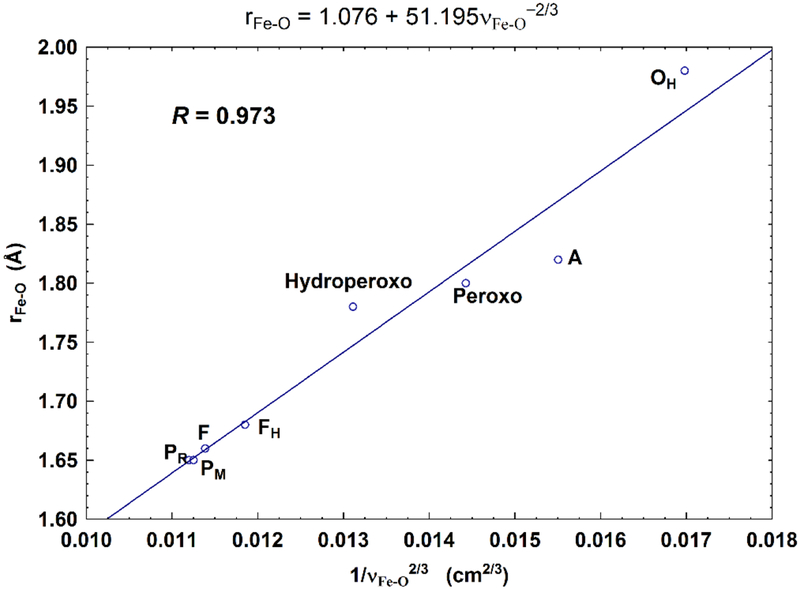
According to Badger’s rule (an empirical formula),⁹⁰ the equilibrium internuclear distance r has linear correlation with 1/ν^2/3, where ν is the vibrational frequency. To see how well this rule applies to our calculated unscaled Fe_a3-O bond lengths (r_Fe-O) and the ν(Fe-O) (or ν_Fe-O) stretch frequencies, we performed linear regression of r_Fe-O vs. ν_Fe-O^−2/3 for the eight DNC models given in Table 3 and 4. The linear fitting is shown in Figure 7 with the correlation coefficient R = 0.973. Although some of the calculated ν(Fe-O) mode frequencies are off from the experimental data (overestimate for state A and underestimate for states P_M/P_R and F), overall our calculated Fe_a3-O stretching frequencies are reasonable, and are correlated with the Fe_a3-O bond length very well based on Badger’s rule.

Figure 7.

Correlation between the calculated Fe_a3-O bond lengths (r_Fe-O, Å) and the corresponding Fe_a3-O stretch frequencies (1/ν_Fe-O^2/3, based on Badger’s rule) for the eight DNC state structures studied in the current paper. The Fe_a3-O stretching frequencies (cm⁻¹) are calculated using the analytical partial-Hessian method. Very similar fitting is obtained with R = 0.963, when using the calculated frequencies obtained from the numerical-MBH method.

Further, a rR band at 356 cm⁻¹ was observed between states P_R and F in the reaction between fully reduced CcO and O₂.^5,62,63 It has been proposed that this band is an His-Fe_a3⁴⁺=O²⁻ bending mode, caused by the distortion of the Fe_a3⁴⁺=O²⁻ bond from its ordinary upright structure (nearly perpendicular to the mean porphyrin plan).⁶² Our calculations show very similar vibrational frequencies (378 vs. 374 cm⁻¹) in this region for P_R and F, which involve mainly the displacement of O, Fe_a3, and the N atoms of the porphyrin ring. Therefore, the observed rR band at 356 cm⁻¹ is likely an O-Fe_a3-porphyrin bending mode.

For iron-porphyrin complexes, several symmetric ligand vibrations have been identified especially in the ν₄(~1330–1375 cm⁻¹) and ν₂(~1540–1575 cm⁻¹) regions, which are correlated with the Fe oxidation and spin states (see Table 1 of Ref. 91).^92–94 In particular, the ν₄/ν₂ marker bands for the Fe²⁺-OH₂, L-Fe³⁺-OOH⁻, L-Fe⁴⁺=O²⁻, and the Fe³⁺-OH⁻ intermediates produced during the oxygen-reducing process by an iron porphyrin are given as 1350/1540, 1349/1545, 1377/1574, and 1364/1555 cm⁻¹, respectively.⁹¹ In our calculations, we found two major symmetric heme-a₃ ligand vibrational bands in each of the ν₄ and ν₂ regions for each of the DNC intermediates. These ν₄/ν₂ calculated frequencies are A(1358,1381 cm⁻¹)/(1532,1574 cm⁻¹), Peroxo(1361,1380 cm⁻¹)/(1536,1572 cm⁻¹), Hydroperoxo(1368,1380 cm⁻¹)/(1534,1571 cm⁻¹), P_M(1365,1385 cm⁻¹)/(1532,1569 cm⁻¹), P_R(1367,1384 cm⁻¹)/(1531,1571 cm⁻¹), F(1367,1379 cm⁻¹)/(1533,1570 cm⁻¹), F_H(1368,1380 cm⁻¹)/(1536,1575 cm⁻¹), and O_H(1361,1376 cm⁻¹)/(1521,1576 cm⁻¹). However, we do not know which combinations of the ν₄/ν₂ bands can be observed without calculating the rR intensities.

4. Conclusions

Resonance Raman (rR) analyses have been extensively applied to the studies of the reaction between CcO and O₂.⁵ Although the detailed dinuclear center (DNC) intermediate structures during the reaction cycle may not be drawn directly from these experiments, the isotope-sensitive rR bands, especially the Fe_a3-O stretching bands and their changes obtained during the O₂-reduction process have provided very insightful information about the structures and the oxidation states of the DNC of CcO. In the current paper, we have performed vibrational frequency calculations at eight DNC intermediate model clusters, and have compared the calculated Fe_a3-O and O-O stretching modes and their shifts with the available rR measurements. Similar calculations have also been performed on the active site model of oxymyoglobin (oxyMb), which has been well characterized experimentally, in order to establish the accuracy of the different calculation methods.

The eight DNC states we have studied here are: A[Fe_a3³⁺-O₂^−•⋯Cu_B⁺, Tyr-OH], Peroxo[Fe_a3³⁺-O₂²⁻-Cu_B²⁺, Tyr-OH], Hydroperoxo[Fe_a3³⁺-(O₂H)⁻-Cu_B²⁺, Tyr-O⁻], P_M[Fe_a3⁴⁺=O²⁻⋯OH⁻-Cu_B²⁺, Tyr-O^•], P_R[Fe_a3⁴⁺=O²⁻⋯OH⁻-Cu_B²⁺, Tyr-O⁻], F[Fe_a3⁴⁺=O²⁻⋯H₂O-Cu_B²⁺, Tyr-O⁻], F_H[Fe_a3⁴⁺=O²⁻⋯Cu_B²⁺, Tyr-O⁻], and O_H[Fe_a3³⁺-OH⁻-Cu_B²⁺, Tyr-O⁻].

The following conclusions have been drawn from our current calculations: 1) Our calculated Fe-O stretching frequencies in state A[Fe_a3³⁺-O₂^−•⋯Cu_B⁺] (with an end-on O₂ binding geometry) of the CcO DNC and in the active site model of oxyMb are very close to each other, which is consistent with what was observed from the rR experiments, and which supports that O₂ binds with Fe_a3²⁺ in CcO in a similar bent end-on geometry as in oxyMb. 2) The calculated O-O bond lengths and bond orders correlate well with the O-O stretching frequencies. 3) Our calculated Fe_a3-O distances (r_Fe-O) also correlate very well with ν_Fe-O^−2/3 (ν_Fe-O are the calculated Fe_a3-O stretching frequencies) for the eight intermediate structures studied here. Therefore, Badger’s rule can be applied to the correlation between r_Fe-O and ν_Fe-O. 4) Our calculations yield ~20 cm⁻¹ shift for the Fe_a3-O stretching mode from state P_R[Fe_a3⁴⁺=O²⁻⋯OH⁻-Cu_B²⁺] to F[Fe_a3⁴⁺=O²⁻⋯H₂O-Cu_B²⁺]. This predicted frequency shift is in excellent agreement with rR experiment. Therefore, it is highly likely that the H₂O ligand is still on the Cu_B²⁺ site in state F. The F_H state (previously we have proposed it to be the F state),⁶¹ where the H₂O ligand has dissociated from the Cu_B²⁺ site, is likely not the state F identified by rR experiment. However, our calculations do not exclude the existence of the F_H state. The F_H intermediate is possibly formed before the O_H state with a very short lifetime. 5) Our calculations show that the observed rR band at 356 cm⁻¹ between states P_R and F is likely an O-Fe_a3-porphyrin bending mode. And 6) the low Fe_a3-O frequency near 450 cm⁻¹, both observed by rR and calculated by DFT for state O_H (the active oxidized state), is as expected for a nearly symmetrically bridged Fe_a3³⁺-OH-Cu_B²⁺ structure with a relatively long Fe_a3-O distance near 2 Å. Finally, our calculations can aid future experiments in identifying the intermediate states peroxo, hydroperoxo, and F_H.