Transcranial magnetic stimulation and the challenge of coil placement: A comparison of conventional and stereotaxic neuronavigational strategies

Abstract

The combination of transcranial magnetic stimulation (TMS) with functional neuroimaging has expanded the potential of TMS for human brain mapping. The precise and reliable positioning of the TMS coil is not a simple task, however. Modern frameless stereotaxic systems allow investigators to base navigation either on the subject's structural magnetic resonance imaging (MRI), functional MRI data, or the use of functional neuroimaging data from the literature, so‐called “probabilistic approach.” The latter assumes consistency across individuals in the location of task‐related “activations” in standardized stereotaxic space. Conventional nonstereotaxic localization of brain areas is also a common method for defining the coil position. Our aim was to evaluate the accuracy of five different localization strategies in one single study. The left primary motor cortex (left M1‐Hand) was used as target region. Three approaches were based on real‐time frameless stereotaxy using information based on either anatomical or functional MRI. The remaining two strategies relied either on standard cranial landmarks (i.e., the International 10–20 EEG system) or a standardized function‐guided procedure (i.e., the spatial relationship between the left and right M1‐Hand). The results were compared to a TMS‐based mapping of the primary motor cortex; center of gravity of motor‐evoked potentials (MEP‐CoG) was calculated for each subject (n = 10). Our findings suggest that highest precision can be achieved with fMRI‐guided stimulation, which was accurate within the range of millimeters. Very consistent results were also obtained with the “probabilistic” approach. In view of these findings, we discuss the methods and special characteristics of each localization strategy. Hum Brain Mapp, 2008. © 2007 Wiley‐Liss, Inc.

INTRODUCTION

Transcranial magnetic stimulation (TMS) can modulate neural processes in the human brain at high temporal and spatial resolution. In cognitive neuroscience, its noninvasive nature has made it attractive as a tool for assessing causality in structure‐function relationships revealed with functional neuroimaging [Paus, 2005; Walsh and Cowey, 2000]. TMS has also a potential as a clinical diagnostic tool [Kobayashi and Pascual‐Leone, 2003], and as a therapy for several neuropsychiatric disorders, most notably depression [George et al., 2003; Lisanby et al., 2002]. If, however, magnetic stimulation does not result in an overt response such as muscle twitches or phosphenes due to stimulation of the motor or visual cortex, respectively, appropriate placement of the coil can be challenging [Paus, 2005]. Although various methods of positioning the coil have been described, there is no consensus on a TMS targeting strategy. Moreover, researchers have suggested that inconsistency in the placement of the TMS coil over the intended area (i.e., the dorsolateral prefrontal cortex, DLPFC) could explain the diverging results of some treatment studies in depression [Herwig et al., 2001a; Lisanby et al., 2002]. In general, optically tracked frameless stereotaxic neuronavigation systems, which combine anatomical magnetic resonance imaging (MRI) data with TMS, have been developed to tackle this problem by guiding the coil to regions selected on the individual's magnetic resonance (MR) images [Boroojerdi et al., 1999; Ettinger et al., 1998; Gugino et al., 2001; Herwig et al., 2001b, 2002; Krings et al., 1997a; Neggers et al., 2004; Paus, 1999]. These systems achieve the virtual linkage between MR images and real anatomy, and allow three‐dimensional (3D) orientation by interactive visual navigation. In principle, stereotaxic neuronavigation can be based on the subject's structural (anatomical) MRI or the functional MRI obtained in the same subject. Another approach represents the use of functional neuroimaging data from the literature [“probabilistic approach”; Paus et al., 1997]. Thus, one has not only to decide whether or not the study design would benefit from the use of stereotaxic neuronavigation but also which strategy to choose in order to locate the desired region of interest with reasonable accuracy. On the other hand, conventional nonstereotaxically navigated localization of brain areas is still a common method to define coil position. In particular, researchers often use the International 10–20 EEG system for the coil positioning [e.g., Herwig et al., 2003; Okamoto et al., 2004] or standardized function‐guided procedures [e.g., Herwig et al., 2001a]. The latter include, for instance, localization of the primary motor or visual cortex by recording, respectively, TMS‐induced motor‐evoked potentials (MEP), or phosphenes [Cowey and Walsh, 2000].

The aim of the current study was to evaluate the efficiency and accuracy of five different TMS localization strategies. The representation of the right hand in the left primary motor cortex (left M1‐Hand) was used as target site. Three approaches were based on real‐time frameless stereotaxic neuronavigation using either anatomical or functional MRI information. The remaining two approaches relied either on standard cranial landmarks (i.e., the International 10–20 EEG system) or used a standardized function‐guided procedure, namely the localization of the homotopic motor area of the contralateral hemisphere. To achieve our goal, we compared the results to a TMS‐based mapping of the primary motor cortex. Such TMS maps have high spatial accuracy, as evaluated using PET [Classen et al., 1998; Wassermann et al., 1996], fMRI [Bastings et al., 1998; Foltys et al., 2000; Lotze et al., 2003; Terao et al., 1998], MEG [Ruohonen et al., 1996], and direct electrical stimulation of the exposed human motor cortex [Krings et al., 1997b, 1998]. The center of gravity of motor‐evoked potentials (MEP‐CoG) was calculated for each subject as described by others [Boroojerdi et al., 1999; Classen et al., 1998]. We then defined two parameters as measures of accuracy of the five test conditions: (1) spatial distance to the center of gravity of the motor mapping (MEP‐CoG) and (2) amplitude‐weighted MEP size relative to that obtained at the center of gravity. The choice of M1‐Hand enabled us to compare directly five different strategies and to compare the results to a TMS‐based mapping providing the aforementioned measures of quality. Nonetheless, we believe that this comparison highlights general methodological issues faced by investigators targeting other cortical regions.

MATERIALS AND METHODS

Subjects

Ten healthy subjects (6 male, 4 female, mean age 28 ± 3.7 years, all right handed) participated in the study. The study was approved by the local ethics committee and all subjects gave informed consent. Exclusion criteria used in the selection of subjects conformed to the current guidelines for TMS research [Belmaker et al., 2003; Wassermann, 1998].

Transcranial Magnetic Stimulation

A custom‐made forehead and chin rest was used to minimize head movements. This position was maintained stable throughout the study. Focal TMS was delivered using a Magstim 200 stimulator (Magstim, Dyfed, UK) equipped with a 9.0 cm figure‐of‐eight shaped coil. Every stimulation was carried out throughout the entire experiment with the handle pointing backwards and approximately 45° lateral from the mid‐line. EMG recordings were made from the relaxed first dorsal interosseus muscle (FDI) of the right hand with Ag/AgCl surface electrodes in tendon‐belly arrangement. A provisional point of optimal excitability was determined at suprathreshold intensity (approximately 60% of maximal stimulator output) and resting motor threshold (minimum stimulation intensity necessary to induce a response of at least 50 μV in 5 of 10 consecutive trials) was measured [Rossini et al., 1994]. The frequency of stimulation was less than 0.2 Hz (i.e., more than 5 s between consecutive stimuli). Motor thresholds ranged between 38 and 50% (SD 5%) maximum stimulator output. The stimulator output intensity was then adjusted, until stable responses with amplitudes of 0.5–1.0 mV could be recorded (stimulator output intensity ranging from 42 to 57%, SD 6%). Subjects were instructed to keep their hands still and as relaxed as possible. The level of muscle relaxation was monitored continuously by means of audio‐visual feedback. MEPs were amplified and digitized using a PowerLab 3T module (AD Instruments, Colorado Springs, CO) with a band‐pass of 20–1,000 Hz at a sampling rate of 4 kHz, and stored for off‐line analysis. The measurement of MEP amplitudes is known to be associated with high intra‐ and interindividual variability. Independent fluctuations in excitability of motor cortex neurons and of cortical and spinal interneurons at the time of stimulation may play a role [Kiers et al., 1993]. Thickbroom et al. [1999] showed that accurate mapping studies can nevertheless be carried out in the presence of such intrinsic variability. Interindividual differences result from many different factors (e.g., size of the target muscle, skin conductance, position of recording electrodes). To decrease further interindividual variability, we therefore normalized MEP amplitudes to the supramaximal peripheral M‐response. Such a procedure has been proved useful in previous mapping studies [Boroojerdi et al., 1999]. A Viking IV EMG device (Viasys, Conshohocken, PA) was used to determine the size of the supramaximal peripheral M‐response (i.e., to apply electrical stimuli with increasing intensities to the ulnar nerve at the wrist until an increase of the M‐response could no longer be observed).

Motor Mapping and Calculation of the Center of Gravity

Mapping the motor cortex with TMS is based on the relationship between the averaged amplitude of the MEP and the density of cortical motoneurons in the area of stimulation [Cohen et al., 1991; Wassermann et al., 1992]. TMS motor maps are known to have very high intraindividual reproducibility [Miranda et al., 1997]. A tightly fitting white Lycra swimming cap was placed over the subject's head. We marked a grid of 6 × 5 points with each point 15 mm apart (i.e., grid extension 7.5 cm in anterior–posterior and 6 cm in mediolateral direction) on the left side, beginning 2 cm lateral from Cz towards the ear (Fig. 1A). Six stimuli were applied in a random sequence over each point of the grid. Classen et al. [1998] showed that the accuracy of determining the amplitude‐weighted center of gravity (MEP‐CoG) corresponds to the number of stimuli at one point. The deviation of the CoG determined after six stimuli was within 2 mm of the optimal CoG estimate [Classen et al., 1998]. The CoG of the MEPs was calculated using the formula X _CoG = Σ_ia_iX_i/Σa_i with a_i being the mean MEP amplitude at the site with coordinate X_i related to the Cartesian coordinate system. The MEP‐CoG of a map of MEPs is known to yield a more accurate estimate of the location on the scalp directly overlying the region of maximum excitability than simply taking the location from the matrix where the MEP is maximal [Boroojerdi et al., 1999; Neggers et al., 2004]. The calculated position of the MEP‐CoG was marked on the subject's head.

Figure 1.

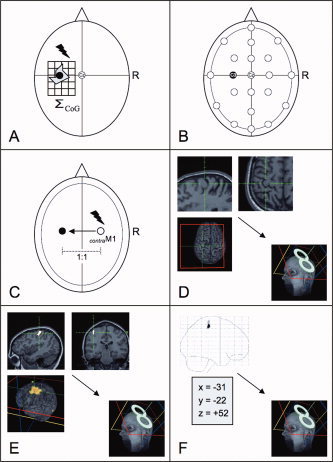
Schematic illustration of experimental conditions: (A) A TMS‐based motor mapping and calculation of the center of gravity (MEP‐CoG) served as referential condition. Localization of left M1‐Hand (black dot) using (B) the International 10–20 EEG system (P _C3), (C) standardized function‐guided procedure (P _cM1), (D) structural (i.e., anatomical) MR image (P _ana), (E) functional MRI data (single subject) (P _fMRI), and (F) functional MRI data (group data/“probabilistic approach”) (P _probab). Note that the selected anatomical MR images show the “omega” and “hook sign.” For details, see text.

After the completion of the motor mapping we tested five different approaches to localize the target region, i.e., the left M1‐Hand. Each position was also marked on the subject's head to determine its spatial distance to the MEP‐CoG. Six MEPs were recorded at each position in the same manner as described earlier. The order of approaches was counterbalanced across subjects.

Conventional Nonnavigated Strategies

Localization of left M1‐Hand using the International 10–20 EEG system (P_C3)

The 10–20 International electrode system [Jasper, 1958] is a commonly used method for positioning of the TMS coil. It locates the electrodes on the scalp using standard cranial landmarks (i.e., nasion, inion, and preauricular points). A main assumption of the system is that there is a consistent correlation between scalp locations and underlying brain structures across subjects. Several studies have examined the validity of this correlation using cadavers [Blume et al., 1974; Jasper, 1958], X‐ray [Hellstrom et al., 1963; Morris et al., 1986], computerized tomography [Homan et al., 1987; Myslobodsky and Bar‐Ziv, 1989; Myslobodsky et al., 1990], and MRI [Gevins et al., 1990; Grzeszczuk et al., 1993; Jack et al., 1990; Lagerlund et al., 1993; Okamoto et al., 2004; Steinmetz et al., 1989; Towle et al., 1993; Van den Elsen et al., 1991; Vitali et al., 2002]. We chose the position of the electrode C3 to target left M1‐Hand (Fig. 1B). Previous studies suggest that the C3/C4 pair overlays either the post‐ or precentral cortex, depending on the individual's brain anatomy [Homan et al., 1987; Jasper, 1958; Okamoto et al., 2004; Steinmetz et al., 1989; Towle et al., 1993].

Localization using a standardized function‐guided procedure (P_cM1)

Another common method to locate a brain area uses its spatial relationship to a reasonably certain position. In particular, TMS researchers rely upon functional criteria such as motor responses or phosphenes obtained from stimulation of the primary motor or visual cortex, respectively. For instance, Pascual‐Leone et al. [1996] proposed the following procedure for locating the dorsolateral prefrontal cortex. First, the location of M1‐Hand was determined as a reference point by means of TMS. Then the coil was positioned 5 cm anterior to M1‐Hand on a line parallel to the midsagittal line using information derived from the Talairach atlas [Talairach and Tournoux, 1988]. Similar procedures were subsequently developed by others (e.g., in studies of the prefrontal cortex [George et al., 1997; Mottaghy et al., 2002], the premotor cortex [Lee and van Donkelaar, 2006; Schluter et al., 1998], or the somatosensory cortex [Koch et al., 2006]). To validate such function‐guided strategies, we incorporated an example of this approach into our study design: On the opposite hemisphere, we first determined the optimal scalp site (i.e., right M1‐Hand) from which TMS induced MEPs of maximal amplitude in the contralateral FDI. Since previous mapping studies of motor cortex demonstrated that the interhemispheric position of TMS maps is very similar in the mediolateral axis [within the millimeters range, Byrnes et al., 1998], we then “mirrored” the coil position to the left hemisphere using the nasion‐inion line as mirror axis to derive the homotopic brain area (i.e., left M1‐Hand) (Fig. 1C).

Strategies Using Frameless Stereotaxic Neuronavigation

Localization using the structural (anatomical) MR image (P_ana)

Here we used the subject's structural brain image together with frameless stereotaxic neuronavigation to identify the left M1‐Hand using common anatomical landmarks. Prior to every neuronavigated session, a T1‐weighted, high‐resolution MR scan of the subject's brain was acquired (Philips Gyroscan 1.5 T scanner, 160 contiguous 1 mm thick sagittal slices). The subject's head and the individual MR scan were carefully coregistered in a common reference frame with an infrared optical‐tracking neuronavigational system (Polaris System, Northern Digital, Waterloo, Ontario, Canada, and Localite software, Localite GmbH, Sankt Augustin – Bonn, Germany). The anatomical landmarks used were the tip and the alar wings of the nose, the nibs of the tragus of both ears, and the internal angles of both eyes. Following the coregistration, the left M1‐Hand was localized by anatomical landmarks on the MR scan (Fig. 1D). The methods used to identify the precentral gyrus were (1) the identification of the typical shape of the motor hand area, i.e., the “hand knob” (omega (90%) or epsilon (10%) configuration in the axial plane, hook configuration in the sagittal plane [Yousry et al., 1997], (2) the detection of the typical course of the superior frontal sulcus [i.e., “axial method,” Kido et al., 1980], and (3) the recognition of the course of the anterior horizontal and ascending branches of the sylvian fissure and precentral sulcus [i.e., the “lateral sagittal method,” Naidich et al., 2001]. If possible, the central sulcal vein was also used as a landmark on 3D rendered images [Yousry et al., 1996]. In agreement with Penfield's homunculus, previous fMRI studies of primary motor cortex showed that activations related to thumb movements tend toward the lateral aspect of the hand knob [Denslow et al., 2004, 2005; Lotze et al., 2000, 2003]. Accordingly, the lateral portion of the hand knob was selected as a target. We assumed that the peak electrical field induced by TMS is generated along an axis that passes through the coil center and is perpendicular to the plane defined by the figure‐of‐eight coil. Hence, the navigation software was used to define a trajectory through the target and the scalp point closest to the cortical target (i.e., entry position) on the basis of each subject's anatomical MRI scan. The navigation system allows us to align the trajectory with the axis of the TMS coil by tracking the 3D‐orientation of the coil in real time on a computer screen.

Localization using individual functional MRI data (P_fMRI)

A modern strategy to locate a certain brain area is to base its identification in the same subject using fMRI. We used fMRI to identify first in each individual the left M1‐Hand area in a simple motor paradigm. Each task consisted of eight 30‐s blocks alternating between rest and activation, the latter involving a repetitive finger to thumb opposition of the right hand at a rate of approximately 1 Hz. Blood oxygenation level‐dependent (BOLD) images were obtained with a gradient‐echo EPI sequence (TR, 2.6 s; TE, 50 ms; flip angle, 90°; slice thickness, 4.2 mm; image matrix, 64 × 64 voxels) using a Philips 1.5 T MRI scanner. The voxel size was 3 × 3 × 3 mm³; 28 axial slices covering the whole brain were acquired. The fMRI data from each subject were processed using Statistical Parametric Mapping Software (SPM2; http://www.fil.ion.ac.uk). First, dummy scans were discarded. The remaining scans were then realigned and the EPI images were smoothed using a Gaussian kernel (9 × 9 × 9 mm³), in order to improve the signal‐to‐noise ratio. For the following parameter estimation, an appropriate design matrix using a box‐car function as reference waveform was specified. The voxel‐by‐voxel parameter estimation for the smoothed data using a statistical threshold of P = 0.05 (corrected, FDR) was done according to the general linear model. The resulting SPM(t)‐maps were then transformed into Talairach space and coregistered with the individual T1 anatomical MRI images using the T1 template (ICBM152) supplied with SPM2. The TMS coil was positioned with frameless stereotaxy as described earlier over the site in the left primary motor cortex that showed the fMRI activation clusters with the highest t values (Fig. 1E).

Localization using group functional MRI data (P_probab)

Identification of the target region can also be based on group averages of PET/fMRI data [Paus, 1999; Paus et al., 1997]. This so‐called “probabilistic” approach may be important in cases when it is not possible to obtain reliable cortical activations on the single‐subject level, e.g. due to insufficient statistical power. This approach aims at taking advantage of a relatively high consistency in the location of task‐related “activations” across individuals [Paus et al., 1997]. It uses x, y, and z coordinates of activation peaks identified in prior group‐based PET or fMRI studies and reported in standardized stereotaxic space.

Although the probabilistic approach has been successfully applied in a number of combined PET/TMS studies [Paus, 1999; Paus et al., 1997, 1998; Strafella et al., 2003], this approach has never been systematically evaluated in comparison to other strategies. The normalization procedure was performed by normalizing each individual (native) head image to the standard ICBM152 brain template by means of an iterative algorithm that searches for the optimal projection of a given brain onto the MNI brain using nonlinear‐basis functions of SPM2. The average probabilistic location of the left M1‐Hand in this stereotaxic space (i.e., x = −31, y = −22, and z = 52) was derived from a meta‐analysis of previous blood flow activation studies that involved finger movements of the right hand [for details, see Paus et al., 1998]. This location was transformed to the individual subject's brain coordinate “native” space using a reverse native‐to‐stereotaxic transformation [Paus et al., 1997]. This procedure allows determining the target region in a given subject (for further details, see Fig. 2). The final step required us to position the coil over the location, now marked on the MR images, which we achieved using frameless stereotaxy as described above (Figs. 1F and 2).

Figure 2.

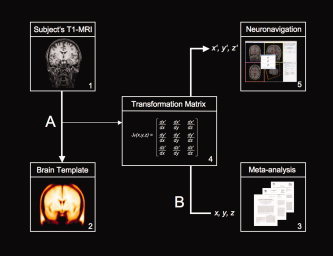
Schematic illustration of the “probabilistic approach”. (A) The subject's anatomical MRI scan (1) is registered with standard stereotaxic space (2) using common tools to analyze functional brain images (e.g., SPM; http://www.fil.ion.ac.uk or MINC; http://www.bic.mni.mcgill.ca/software/minc/). The latest version of SPM (SPM5) uses, for instance, a modified version of the ICBM Tissue Probabilistic Atlases (http://www.loni.ucla.edu/ICBM/) as standard brain template (i.e., tissue probability map). In this case, the original data are derived from 452 T1‐weighted scans, which were aligned with an atlas space, corrected for scan inhomogeneities, and classified into grey matter, white matter, and cerebrospinal fluid. The operation produces transformation matrices (i.e., vector fields) where three values are associated with each location in the field (4). (B) These transformation parameters can then be used for inverse transformation. The field maps coordinates in the standardized image (x, y, z) back to co‐coordinates in the original (native) image (x′, y′, z′). In this way, average probabilistic locations in stereotaxic space (e.g., MNI‐space) derived from meta‐analysis (3) can be transformed into the corresponding “probabilistic” coordinate in the original volume. Finally, this location in “native” space is process by the neuronavigation software to determine the position of the coil stereotaxically (5).

Statistical Analysis

The peak‐to‐peak amplitudes of MEPs were averaged for each point of stimulation (P _C3, P _cM1, P _ana, P _fMRI, and P _probab). The amplitude‐weighted center of gravity (MEP‐CoG) was calculated as described earlier. Then the mean MEP amplitudes were expressed as values relative to the MEP‐CoG (mean MEP/MEP‐CoG). The spatial differences of the locations of the five different approaches were measured with respect to the location of the MEP‐CoG. The distances were determined in the anterior‐posterior (i.e., y‐axis) and the mediolateral direction (i.e., x‐axis). Finally, the mean Cartesian distances in two‐dimensional space (x–y) were calculated from the x and y coordinates. Data were analyzed with repeated measure analysis of variance (ANOVA). We applied Fisher's LSD test to compute post hoc comparisons. Pearson's correlation coefficient was used to examine whether there was a correlation between changes in MEP size and changes in mean distance. Differences were considered significant at a level of P < 0.05.

RESULTS

In all but one subjects, we detected hand knob‐like structures on the structural MRI as an inverted omega protruding into the central sulcus. In the remaining subject, the hand knob was epsilon shaped. Functional MRI revealed a localized significant (P < 0.05, corrected) increase in BOLD signal intensity in each individual. The fMRI activity was consistently located in the region of the hand knob of the left precentral gyrus. Each location was projected onto the horizontal plane as viewed from above (Fig. 3).

Figure 3.

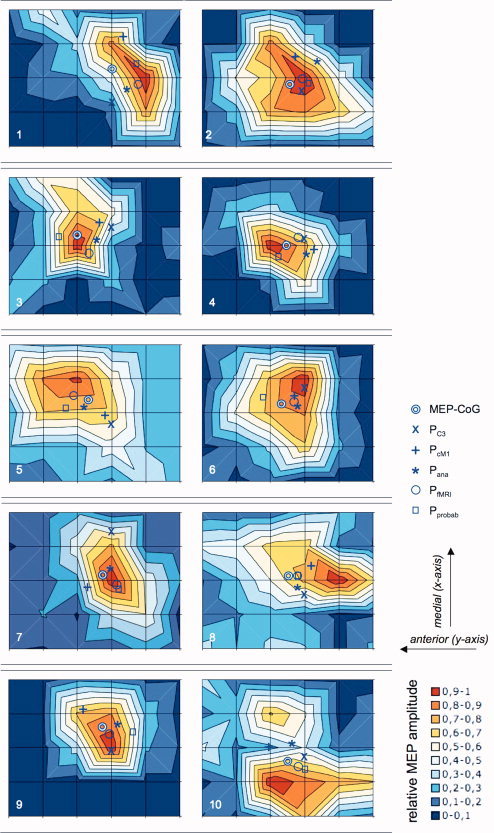
Illustration of contour maps derived from averaged responses to 6 trials of TMS at scalp sites 15 mm apart in subjects 1–10. Contours represent 10 percentiles of the maximal averaged response as viewed from above. Double circles indicate amplitude‐weighted centers of gravity. The letters X indicate position of EEG electrode C3. Plus signs refer to positions derived from the contralateral motor hand area. Asterixis indicate “anatomical”, single circles “functional” and boxes “probabilistic” image‐guided locations, respectively (for details, see text). Please note that there could be small errors due to the graphical conversion and reproduction of the original data. For correct values, refer to Table I.

In 9 out of 10 subjects, the MEP‐CoGs were located anterior to both the P _fMRI (absolute range 3–10 mm, SD 3.8 mm) and P _ana (range 2–13 mm, SD 4.2 mm) (Table I). P _fMRI and P _ana showed the smallest variation in the anterior–posterior direction. P _C3 was also located posterior to the MEP‐CoG in most cases (range 1–14 mm, SD 4.3). In the mediolateral direction, P _fMRI and P _probab were associated with the smallest deviations (SD 3.7 and 3.1 mm, respectively). P _cM1 (range 1–12 mm, SD 6.3 mm) and P _C3 (range 2–21 mm, SD 10.6 mm) showed the largest variation in the mediolateral direction. The mean distances calculated from the Cartesian x–y distances relative to the MEP‐CoG were largest for P _C3 (12.3 ± 4.4 mm) and P _cM1 (10.2 ± 2.2 mm) and smallest for P _fMRI (6.3 ± 2.5 mm). The mean distances of P _probab (8.6 ± 2.2 mm) and P _ana (7.8 ± 3.4 mm) were in the same range (Fig. 4, Table I).

Table I.

Mean spatial distances

Subject number	Distance (mm)
	P C3			P cM1			P ana			P fMRI			P probab
	x	y	x–y	x	y	x–y	x	y	x–y	x	y	x–y	x	y	x–y
1	−14	−1	14.0	12	4	12.6	−10	5	11.2	−6	10	11.7	1	8	8.1
2	−3	6	6.7	10	4	10.8	7	13	14.8	1	6	6.1	0	10	10.0
3	2	14	14.1	3	10	10.4	−4	9	9.8	−8	5	9.4	−2	−8	8.2
4	2	9	9.2	−1	12	12.0	−3	9	9.5	3	6	6.7	−6	−3	6.7
5	−11	12	16.3	−7	9	11.4	−4	−2	4.5	2	−5	5.4	−4	−9	9.8
6	8	9	12.0	3	4	5.0	−1	5	5.1	1	4	4.1	3	−9	9.5
7	21	4	21.4	−6	−6	8.5	3	3	4.2	−3	5	5.8	−6	6	8.5
8	−8	7	10.6	5	9	10.3	−4	4	5.7	1	4	4.1	1	4	4.1
9	−10	4	10.8	7	−9	11.4	1	7	7.1	−3	3	4.2	−3	12	12.4
10	3	7	7.6	6	−8	10.0	6	2	6.3	−3	4	5.0	−4	8	8.9
Mean	−1.0	7.1	12.3	3.2	2.9	10.2	−0.9	5.5	7.8	−1.5	4.2	6.3	−2.0	1.9	8.6
SD	10.6	4.3	4.4	6.3	7.8	2.2	5.2	4.2	3.4	3.7	3.8	2.5	3.1	8.3	2.2

Individual data in mm of the spatial distances between the MEP‐CoG and P _C3, P _cM1, P _ana, P _fMRI, and P _probab, respectively (for details, see text). The distances were determined in the anterior–posterior (y‐axis) and the mediolateral direction (x‐axis). Positive x and y values correspond to spatial variance between P _C3, P _cM1, P _ana, P _fMRI, or P _probab and the MEP‐CoG in the medial (x‐axis) and posterior (y‐axis) direction, respectively. Negative x and y values indicate vice versa deviation in the lateral and anterior direction. The mean distances in two‐dimensional space (x–y) were additionally calculated from the x, y coordinates.

Figure 4.

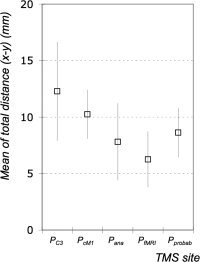
Total mean distances (x–y) and standard deviations (SD) in mm between the MEP‐CoG and the positions localized using the International 10–20 EEG system (P _C3), a standardized function‐guided procedure (P _cM1), structural (i.e., anatomical) MR image (P _ana), functional MRI data (single subject) (P _fMRI), and functional MRI data (group data/“prohabilistic approach”) (P _Probab). Data were averaged from all subjects.

One‐way repeated measures ANOVA with site of stimulation (5 levels) showed a significant main effect (F = 5.96; P = 0.001). Post hoc comparison revealed that P _C3 was associated with a significantly larger mean distance to the MEP‐CoG compared to the mean distances of the three neuronavigated approaches (i.e., P _ana, P _fMRI, or P _probab; P < 0.01). Likewise, the mean distance between P _cM1 and the MEP‐CoG was significantly higher compared to P _fMRI (P < 0.006). However, the comparison of the mean distances of P _cM1 and P _ana showed a nonsignificant trend only (P < 0.08).

TMS elicited motor responses in the FDI at each determined location in all subjects. Further analysis indicated considerable differences of the amplitudes of the mean MEPs normalized to the MEP‐CoG: P _C3 48.6%, P _cM1 56.0%, P _probab 73.6%, P _ana 76.0%, and P _fMRI 87.6% (Fig. 5). One‐way ANOVA with site of stimulation (5 levels) revealed a significant main effect (F = 8.14; P < 0.001). Post hoc comparisons showed that significant lower MEP sizes were obtained at the sites determined by nonnavigated strategies (i.e., P _C3 and P _cM1) in comparison to the three neuronavigated approaches (i.e., P _ana, P _fMRI, or P _probab; P < 0.04). No significant differences were noted between P _C3 and P _cM1 or between P _ana, P _fMRI, and P _probab, respectively. The correlation analysis revealed that changes in mean MEP size were highly negatively correlated with changes in mean distance (r = −0.98, P = 0.002).

Figure 5.

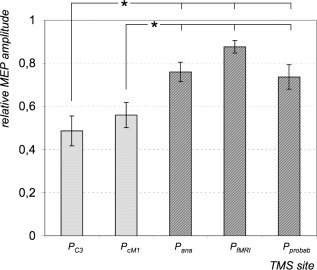
Mean MEP size (relative to MEP‐CoG) obtained at the positions localized using the International 10–20 EEG system (P _C3), a standardized function‐guided procedure (P _cM1), structural (anatomical) MR image (P _ana), functional MRI data (single subject) (P _fMRI), and functional MRI data (group data/“prohabilistic approach”) (P _probab). Post hoc comparison showed that significant lower MEP sizes were obtained at the sites determined by nonnavigated strategies (P _C3 and P _cM1) in comparison to the three neuronavigated approaches (P _ana, P _fMRI, or P _probab). Data were averaged from all subjects. Asterixis indicate significance (P < 0.05). Bars indicate standard errors.

DISCUSSION

In this study, we focused on the accuracy and efficacy of five different strategies in localizing the primary motor cortex. Three strategies applied frameless stereotaxic neuronavigation, whereas the remaining two either relied on standard cranial landmarks or used a standardized function‐guided procedure. First, we found that MEPs could be obtained at each determined site of stimulation, independently of the localization strategy used. Furthermore, a correlation was found between both outcome measures (i.e., between MEP amplitude and the mean distance relative to the MEP‐CoG). Overall, the MEP‐CoG was consistently located slightly anterior to the five test positions in the anterior–posterior direction (means ranging from 2 to 7 mm). This finding has also been reported by others. Herwig et al. [2002] found a spatial divergence of the centers of gravity from fMRI and MEP with a mean distance of about 10 mm, with the MEP‐CoG located consistently anterior to the motor task‐related activation (mean derivation 7.5 mm). Using a similar technique, [Lotze et al., 2003] observed MEP‐CoGs 10 mm anterior to fMRI activation induced by thumb movements. It was speculated that this might be related to the physical properties of the figure‐of‐eight coil used in the aforementioned studies since an iron core TMS coil produced slightly diverging results [Neggers et al., 2004]. The calculated MEP size of the CoG was consistently higher compared to mean MEP obtained at the five testing sites. Although spatial deviations were relatively small compared to the size of the induced electric field in the range of several square centimeters [Fox et al., 2004; Thielscher and Kammer, 2002, 2004; Wagner et al., 2004], there were considerable differences among the test conditions.

Conventional Nonnavigated Strategies

Localization of left M1‐Hand using the International 10–20 EEG system (P_C3)

In our study, the localization of the left M1‐Hand using the 10–20 electrode system produced the least accurate results of all tested approaches. Although MEPs could be obtained in each single subject, the MEP amplitudes and spatial accuracy were significantly lower compared to other conditions, which confirms the findings of Herwig et al. [2003]. This is not surprising given the fact that the 10–20 system relies on cranial landmarks (e.g., nasion, inion, and preauricular points). It is well known that frequent difficulties in defining these landmarks or asymmetries and deformities of the skull and brain, respectively, are sources of inaccuracy of electrode positioning [Binnie et al., 1982; Habib et al., 1984; Myslobodsky and Bar‐Ziv, 1989; Myslobodsky et al., 1990; Towle et al., 1993]. Furthermore, a placement error up to 5 mm also has to be considered even in trained EEG technicians. Some electrode positions can, therefore, be located more reliably than others. For instance, midline electrode locations show the least variability across sessions [e.g., Towle et al., 1993]. Some authors reported the greatest measurement variability for electrodes Fp1, Fp2, F3, F4, T5, and T6 [Lagerlund et al., 1993; Towle et al., 1993]. However, others reported the greatest variability for F3, F4, O1, and O2 [Myslobodsky et al., 1990; Okamoto et al., 2004]. These discrepancies most likely result from different measuring methods. Herwig et al. [2003] evaluated the electrode positions (F3, F4, T3, TP3, and P3) and found interindividual variations in the different axes concerning one electrode position below the range of 20 mm.

Apart from the technical errors, interindividual variability in the position of cerebral sulci represents another source of variation. In particular, the position of the C3–C4 pair in the anterior–posterior direction is quite controversial. Jasper [1958] found C3–C4 within 10 mm of the central sulcus. However, his marking of C3–C4 on the postcentral region in a figure led to some confusion, since he mentioned the position as precentral in the text. Homan et al. [1987] found the pair to be located above the precentral sulcus, caudal to the middle frontal gyrus. In a small MRI study of epileptic patients, Vitali et al. [2002] reported that anterior–posterior position of the C3–C4 pair had the largest standard deviation (13 mm) depending on the variable location of the central sulcus. Overall, it has been suggested that the pair overlays either the post‐ or precentral cortex, depending on the individual's anatomy [Okamoto et al., 2004; Steinmetz et al., 1989; Towle et al., 1993]. However, a small number of TMS studies on somatosensory function suggested that C3–C4 are located slightly posterior to the primary motor cortex [Harris et al., 2002; Knecht et al., 2003]. To tackle this issue of uncertainty, Okamoto et al. [2004] established recently a correspondence between the 10–20 electrode system and standard stereotaxic space (MNI space) [Jurzak et al., 2005; Okamoto et al., 2004]. They computed y = −16.4 as position on the y‐axis (e.g., anterior‐posterior direction) in MNI space for electrode C3 projected onto the cortical surface. This location was only slightly anterior to our “probabilistic” (intracortical) location of M1‐Hand (y = −22), which we used for stereotaxic neuronavigation (refer to later section). However, it has to be considered that all subjects were Japanese who generally have shorter and wider hemispheres than Caucasians [Zilles et al., 2001].

In conclusion, although stereotaxic positioning is more accurate, the 10–20 electrode system may be an acceptable compromise under certain circumstances [Herwig et al., 2003]. It is important to notice that the accuracy differs between certain electrode positions, e.g. a much larger variability is associated with C3, C4 than with P3, P4. Nevertheless, the 10–20 electrode system has considerable advantages: it is easily applicable in its practicable use, and from an economical point of view at low costs compared to neuroimaging methods, which may not be available in every situation and/or environment. Moreover, the recent attempt of Okamoto et al. [2004] to transfer the 10–20 electrode system to standard stereotaxic space represents a very promising attempt to reduce further the error associated with the correlation between scalp locations and their underlying cerebral structures [Jurzak et al., 2005; Okamoto et al., 2004].

Localization using a standardized function‐guided procedure (P_cM1)

A common method to locate a brain area is to define the coil position based upon a reference point at which TMS can elicit objective responses. This is relatively easy to achieve for the primary motor (muscle twitch) and visual cortex (phosphene). Other brain areas can be subsequently targeted by drawing upon established spatial relationships, derived from anatomic atlases, to the reasonably certain positions. The precision of such an approach has been evaluated in one study only, which targeted at the dorsolateral prefrontal cortex (DLPFC) [Herwig et al., 2001a]. Using stereotaxy for evaluation, the final location of the coil relative to the underlying cortical structures was found to be quite variable (the coil was not placed over the DLPFC as intended in 7 out of 22 subjects). On the other hand, Rektorova and Paus used anatomical MR scans obtained in 152 healthy volunteers and calculated a probabilistic location of a “prefrontal” region located 5 cm in front of the left probabilistic primary motor cortex, thus mimicking procedure often use when targeting prefrontal cortex for treatment of depression; the standardized stereotaxic coordinates are x = −40, y = 32, and z = 30 [Paus and Barrett, 2004] and, as such, fall within the “prefrontal” cortex as defined by functional imaging.

In our study, we used an analogous procedure by employing the contralateral M1‐Hand as a reference for the placement of the coil above the homotopic brain area (i.e., left M1‐Hand). In principle, this procedure yielded the same precision as the approach based on the 10–20 electrode system. Precision was significantly lower compared to the strategies applying frameless stereotaxic neuronavigation. Errors may result, in particular, from movements of the coil in the mediolateral direction in conjunction with hemispheric asymmetries of the subject's brain [Habib et al., 1984; Kim et al., 1993]. It is also known that the dominant motor cortex exhibits a larger representation for small hand muscles [Krings et al., 1997a]. On the contrary, previous mapping studies demonstrated that the interhemispheric position of TMS maps is very similar in the mediolateral axis [Byrnes et al., 1998]. This finding suggests that inaccuracy may primarily be related to imprecise measurements due to technical limitations, since these are usually carried out manually on the subject's head with a tape measure and marker pen. In conclusion, our results emphasize that function‐guided procedures, which use functional criteria together with spatial information derived from anatomical atlases, do not sufficiently account for individual variations in the distance between the reference and target sites. These standardized procedures are without doubt easily applicable and should therefore be applied in situations, which do not demand high spatial precision.

Strategies Using Frameless Stereotaxic Neuronavigation

In our study, the use of real time image‐based frameless neuronavigation was associated with significantly higher motor responses and lower spatial deviances compared to nonnavigated strategies. No statistically significant differences were found across the three approaches. Their outcome almost reached the level of the referential motor mapping. Nevertheless, image‐guided neuronavigational procedures may be influenced by system‐made and/or man‐made errors, respectively [Spetzger et al., 2002]. On one side, the accuracy of the system depends on its technical limitations as well as on the quality of the neuroradiological investigation (i.e., the particular sequence and its parameters such as slice thickness etc.), on the other it relies upon an exact subject‐image registration (i.e., sufficient user training) [Schonfeldt‐Lecuona et al., 2005; Steinmeier et al., 2000]. Nevertheless, frameless stereotaxic coil positioning provides good reliability. For instance, Schonfeldt‐Lecuona et al. [2005] demonstrated a sufficient within‐session stability and intersession repeatability (i.e., mean Euclidean distance of 1.6 and 2.5 mm, respectively).

In contrast to the high technical accuracy of neuronavigation with fiducial reference frames in surgical settings (ranging within 0.55 ± 0.29 mm) [Kaus et al., 1997], frameless stereotaxy is generally associated with higher spatial errors [from 4 to 8 mm, according to Zinreich et al., 1993]. Thus, minimization of all controllable errors is the prerequisite for the successful use of stereotaxic navigation systems. In particular, precisely centered and pin‐point touching of the skin fiducials is essential. Plausibility checks should be additionally performed during the application, especially if the software interface does not provide feedback about the registration error. The main advantage of frameless stereotaxy is that it allows investigators to align the center of the figure‐of‐eight coil with the target site and to monitor all degrees of freedom of the coil, including the angle of the coil on the scalp. In this way frameless stereotaxy can also assure a constant coil position over time, which is of crucial importance in studies applying repetitive TMS (rTMS) over a few minutes or more.

Localization using the structural (anatomical) MR image (P_ana)

The gross anatomy of the cerebral cortex can serve as a reference system when conducting a TMS study. However, the correlation between macroscopic anatomical features of the cortex (e.g., gyri, sulci, etc.) and the functional subdivisions of the cortex (e.g., Brodmann areas) is imperfect and varies considerably between regions. Further disadvantages of this strategy are individual differences of gyral folding and cortical layering [Rademacher et al., 2001] as well as the fact that reliable anatomical landmarks do not exist for every target site. The anatomical location of M1‐Hand can be identified using reliable and evaluated landmarks as described by Yousry et al. [1997]. We were able to identify the “hand knob” of the motor cortex in each single subject. Our findings are in line with the results of recent studies, which evaluated anatomical MR image‐guided positioning [Denslow et al., 2004, 2005]. Similar to the location of the MEP‐CoG in our study, it was found that positions located by the method of “trial and error” were located slightly more anterior than the anatomical MR‐guided positions. The placement based solely on anatomical image data proved that it is as reliable as that resulting from functional guidance. Stereotaxic neuronavigation on the basis of anatomical landmarks can thus be recommended if an adequately trained investigator can reliably identify the target region by well‐defined landmarks. However, this may certainly not be always the case. Then the supplementary use of fMRI may be considered a good alternative (see below).

Localization using individual functional MRI data (P_fMRI)

Previous studies have proven that precise anatomical location of M1‐Hand can be achieved with fMRI [Boroojerdi et al., 1999; Kim et al., 1993; Krings et al., 1997a; Lotze et al., 2000, 2003]. In the present study, the fMRI‐guided approach led to the highest motor responses, which may be explained by a relative close anterior–posterior distance to the MEP‐CoG together with the smallest variation (4.2 ± 3.7 mm) compared to the remaining four test conditions. Our results match those of previous studies, which reported spatial correspondence of TMS and fMRI within a millimeter range [Boroojerdi et al., 1999; Bastings et al., 1998; Denslow et al., 2004; Krings et al., 1997a; Neggers et al., 2004]. Some authors could achieve even higher precision (e.g., a distance of 2.3 ± 0.8 mm reported by Terao et al. [1998]). The MEP‐CoG was consistently located anterior to the motor task‐related activation. This shift could result from a posterior displacement of the BOLD signal conditioned by simultaneous movement‐related somatosensory activation. On the other hand, it was found that the proprioceptive input to the motor areas, if any, is small [Mima et al., 1999], and that the activation of M1‐Hand resulting from active index finger movements does not differ between proprioceptive deafferented patients and healthy controls [Reddy et al., 2001].

In conclusion, frameless stereotaxy in combination with fMRI reduces the influence of interindividual anatomical variability and no longer assumes a relationship between anatomical landmarks of the cerebral cortex and task‐related functional activations. Although it seems that this concept provides best accuracy, it may be associated with some sources of error. First, the validity and quality of the fMRI investigation has to be considered. For instance, it is well known that some imaging techniques are more sensitive to signal change caused by veins, which can shift the BOLD contrast away from the site of real neural activation [Krings et al., 1999; Ramsey et al., 1998]. Furthermore, the neuronal populations that cause the BOLD signal and the neurons activated by TMS may not be identical [Attwell and Iadecola, 2002] and the correspondence between cytoarchitecture and functional representation may also be inconsistent [Brett et al., 2002]. Finally, the method requires that (1) the same individual participates in both stages of the study (fMRI and TMS) and (2) reliable fMRI activations of the desired cortical region can be obtained on the single‐subject level.

Localization using the group functional MRI data (P_probab)

To target the same cortical region in all subjects, Paus et al. [1997, 1999] developed a “probabilistic” approach, which takes advantage of both standardized stereotaxic space and frameless stereotaxic neuronavigation. This approach uses coordinates of the target site as reported, in standardized stereotaxic space (e.g., ICBM[MNI]152 or MNI305), in a number of previous functional imaging studies. In the first step, a high‐resolution MRI of the subject's brain is transformed into standardized stereotaxic space. Coordinates of the target (i.e., x, y, and z) are then projected back into each subject's “native” MRI space [for details, see Paus et al., 1997]. Although this concept has been used in many recent TMS studies, it has not been consistently evaluated so far. In the present study, we applied this strategy to identify the left M1‐Hand. The probabilistic coordinates were derived from a previous rTMS/PET study [Paus et al., 1998]. Surprisingly, we did not find significant differences in the precision in comparison to both the anatomical and fMRI‐guided approaches. Compared with the fMRI‐guided strategy, however, the probabilistic approach was associated with higher variability, especially in the anterior–posterior direction (probabilistic: range 3–12 mm, SD 8 mm; fMRI: 3–10 mm, SD 4 mm). This finding implies that probabilistic approaches can be used for reliably targeting cortical regions of interest. One should note, however, that the factors influencing the quality of this strategy are manifold. Errors can certainly arise from individual differences in the gross anatomy of the brain and skull. To express brains of different sizes in the same coordinates, they are spatially normalized to a standard template brain. Since such a procedure is imperfect per se, the choice of templates, coordinate systems, and normalization algorithms should be performed with great care. In principle, the probabilistic strategy is influenced by all factors relevant to the use of single subject fMRI data (refer to earlier section). However, its high accuracy may be explained by a “trade off” situation. On one hand, the use of probabilistic coordinates may be more error prone to individual anatomical variations, and on the other it may profit from the fact that task‐induced activations obtained in the single subject may be less reliable in studies of some cognitive tasks.

Limitations of the Study

One shortcoming of our mapping protocol is that we related the motor maps not to the individual, 3D skull anatomy but instead to a two‐dimensional (2D) grid (i.e., a Cartesian coordinate system). We assume, however, that the distortion, which occurs certainly when a 3D map is represented on a 2D coordinate system, is tolerable in the case of the motor cortex. Previous mapping experiments showed that cortical motor representation can be investigated despite the use of a 2D coordinate system [Boroojerdi et al., 1999; Cohen et al., 1991; Foltys et al., 2000; Macdonell et al., 1999; Metman et al., 1993; Wilson et al., 1993]. Furthermore, this technical restriction did not obviously affect the measure of MEP amplitudes, which was highly negatively correlated with the measure of spatial distances. Finally, our results of the fMRI‐guided navigation were in the same range as those of Herwig et al. [2002], who used a very precise 3D motor mapping. At first glance, the relatively poor spatial resolution of the TMS map and the fact that the area of stimulation with a figure‐of‐eight coil is also relatively large at 1–2 cm² [Bohning et al., 2001] may also represent potential limitations of our study. However, mapping studies have demonstrated that the CoG can be determined with a very high spatial resolution within a few millimeters [Boroojerdi et al., 1999; Classen et al., 1998; Miranda et al., 1997; Thickbroom et al., 1999; Wilson et al., 1993], which is due to averaging across the number of sites and stimuli applied at each site. In our study we applied 7.5 cm × 6 cm grids with 30 points. Although grids of this size have been used previously to map motor areas [Boroojerdi et al., 1999; Foltys et al., 2000], their actual resolution has not been evaluated systematically. Classen et al. [1998] compared grids with various specifications and estimated that the MEP‐CoG obtained with a smaller but finer grid (5 × 5 cm², 25 points) differs approximately 2–4 mm from the optimal CoG estimate. However, their results also showed that increasing the map size by extending the stimulated field to positions where no responses could be elicited results in a substantial improvement in accuracy of CoG compared to calculations based to a fixed area.

Although we intended to clarify the problem of coil positioning for areas outside the motor cortex related to higher cognitive functions, we have chosen the motor cortex as the primary target site. Therefore, another potential drawback of our study may be that our results may not extend into just any cortical brain area. For instance, we discussed that the 10–20 electrode system may locate other brain areas more reliably than the motor cortex due to high interindividual anterior‐posterior shift of the central sulcus [Luders et al., 2003]. The exemplary choice of M1‐Hand only enabled us (1) to compare directly five different strategies and (2) to contrast the results to a TMS‐based mapping providing two measures of quality (distance and MEP amplitude). It has to be noted that TMS target sites could also be located by strategies different to the investigated procedures in our study. Some researchers verified the coil position by reproduction of a known TMS‐induced effect on a second behavioral task prior to the main task of the study. For instance, Gobel et al. [2001] identified the angular gyrus site in their study on number comparison by testing first the previously proven effect of rTMS on a visual search task [Walsh et al., 1998, 1999]. Similarly, Grosbras and Paus [2002, 2003] used a functional probe to verify placement of the coil over the frontal eye field. The placing of fiducial markers (i.e., vitamin E capsules) visible in imaging is also a common method to verify the position of the TMS coil after the experiment [Bastings et al., 1998; Terao et al., 1998].

Further Methodological Aspects Relevant to the Positioning of the TMS Coil

In the past, researchers have often neglected the problem of coil orientation in studies on cognitive function. Although the importance of coil orientation on the motor [Brasil‐Neto et al., 1992] and visual cortex [Kammer et al., 2001] has been demonstrated, there have been only a few studies so far, which investigated the relevance of coil orientation for nonprimary motor and visual areas, respectively [e.g., frontal cortex, Hill et al., 2000]. Fox et al. [2004] recently hypothesized that cortical responses to TMS are probably highest when the induced electrical field is oriented parallel to cortical columns. In the future, the development of more focal TMS coils [e.g., Kim et al., 2006] and mechanical positioning aids may increase the accuracy and reproducibility of stereotaxic coil positioning [e.g., Fox et al., 1997; Krings et al., 1997b; Paus et al., 1997]. For instance, Lancaster et al. [2004] reported that they were able to achieve an overall accuracy in positioning of about 2 mm by means of an image‐guided robotically positioned TMS system, which incorporated also the aforementioned theory of Fox et al. [2004]. Recently, Knecht et al. [2005] investigated another frequently unrecognized, but critical issue. For identical TMS intensities they found that regional differences in scalp‐to‐cortex distance (there is usually large lateral to medial gradient) can translate into differences in electric field strength in the underlying superficial cortex of up to a factor of two. Further software developments may also contribute to higher accuracy. For instance, Noirhomme et al. [2004] created an automated method, associated with a remarkable precision, to visualize TMS responses (i.e., motor responses) by projecting them in real‐time onto the segmented subject's brain. Significant advances in precise coil positioning may also result from the recent development of MR‐compatible TMS systems. Because of the nature of these systems, MR image‐guided TMS application can be performed inside the MR scanner without an error‐prone coregistration procedure that uses fiducial markers and marks on the subject's head [Bestmann et al., 2003; Bohning et al., 2003; Denslow et al., 2005].

CONCLUSION

The results of this study demonstrate that the highest precision can be achieved with stereotaxic guided coil placement. Accuracy of frameless‐stereotaxy is on the order of several millimeters even under ideal circumstances. Surprisingly, very consistent results were also obtained with the “probabilistic” approach based on standard stereotaxic space. Overall, there are probably manifold factors that influence the final choice of the appropriate methodological approach in a given setup. Because of the variety of different localization strategies, researchers should be aware of the associated advantages and disadvantages, respectively. In particular, one should pay attention to the special characteristics linked with the desired brain area (e.g., presence or absence of anatomical landmarks or validity of fMRI activations). It seems reasonable that further methodological refinements will result in an even more precise, reliable, and comfortable positioning and monitoring of the TMS coil. Until then, exact coil positioning remains, however, a sophisticated challenge in the studies of human cognition.