R1ρ Sensitivity to pH and Other Compounds at Clinically Accessible Spin-Lock Fields in the Presence of Proteins

Abstract

Numerous human diseases involve abnormal metabolism, and proton exchange is an effective source of magnetic resonance imaging (MRI) contrast for assessing metabolism. One MRI technique that capitalizes on proton exchange is R₁ relaxation in the rotating frame (R_1ρ). Here we investigated the sensitivity of R_1ρ to various proton-exchange mechanisms at spin-lock pulses within FDA safety guidelines for radiofrequency-induced heating. We systematically varied pH known to change the rate of proton exchange as well as the glucose and lysine concentrations, thus changing the number of amide, hydroxyl, and amine exchangeable sites in a series of egg-white albumin phantoms. The resulting effects on quantitative relaxation time measurements of R_1ρ, R₁, and R₂ were observed at 3T. Using spin-lock amplitudes available for human imaging (less than 23.5μT) at near physiologic temperatures, we found R_1ρ was more sensitive to physiologic changes in pH than to changes in glucose and lysine concentrations. In addition, R_1ρ was more sensitive to pH changes than R₁ and R₂. Models of proton exchange fit to the relaxation measurements suggest that amide groups were the primary source of pH sensitivity. Together, these experiments suggest an optimal spin-lock amplitude for measuring pH changes while not exceeding FDA subject heating limitations.

INTRODUCTION

Proton exchange is being increasingly utilized as a source of intrinsic contrast in magnetic resonance imaging (MRI). In tissue, water protons are continuously exchanged with protons on amide (O=C-NH-), hydroxyl (-OH) and amine (-NH₂) groups abundant in macromolecules such as proteins. The magnetization of protons in water and macromolecules is sensitive to this exchange. The rate of exchange (k_ex) depends on the forward (k₁) and reverse (k_-1) rate constants and can range from 10–500s⁻¹ for amide protons to 500–10,000s⁻¹ for hydroxyl and amine protons¹.

$${\text{k}}_{\text{ex}}={\text{k}}_1+{\text{k}}_{-1}.$$ (1)

The two most commonly used MRI techniques sensitive to proton exchange are R₁ relaxation in the rotating frame (R_1ρ) and chemical exchange saturation transfer (CEST). These techniques have been used to study several psychiatric and neurological disorders including Alzheimer’s Disease^2–4, Parkinson’s Disease ⁵, Huntington’s Disease ⁶, panic disorder ⁷, and bipolar disorder ^8,9. Both static^8,9 and functional⁷ changes in R_1ρ relaxation rates have been observed, which have been interpreted to be driven at least in part by abnormalities in brain pH. However, solvent concentrations or other macromolecules could also contribute to the observed changes. Relatively little work has been done to characterize R_1ρ relaxation rates using commonly employed clinical MRI field strengths (e.g. 3T) and spin-lock amplitudes that are within the Food and Drug Administration (FDA) radiofrequency heating limits.

MRI pulse sequences for acquiring R_1ρ typically involve a spin-lock block comprised of the following: 1) a 90° radiofrequency (RF) pulse tipping the magnetization into the transverse plane; 2) a spin-lock RF pulse of constant amplitude; and 3) a second 90° RF pulse restoring the magnetization to the longitudinal direction¹⁰. To help minimize B₁ and B₀ sensitivity of the spin-lock block, adiabatic 90° RF pulses can be used¹¹, and the phase of the spin-lock pulse can be alternated for each half of its duration¹². R_1ρ relaxation rate can be calculated from two or more images with different spin-lock pulse durations (TSL) using Equation 2:

$$R_{1\rho }=\frac{1}{T_{1\rho }}=-\ln (\frac{S_{TSL}}{S_0})/TSL,$$ (2)

where S_TSL is the signal collected after applying the spin-lock block and S₀ is the signal without applying the spin-lock pulse. Previous work has attributed the sensitivity at lower spin-lock amplitudes to amide exchange and other low frequency processes^13,14 while work at ultra-high magnetic fields and large spin-lock amplitudes (greater than 23.5μT) have found that spin-lock pulses are sensitive to hydroxyl and amine protons^15,16.

While a number of studies have aimed to discern factors affecting R_1ρ relaxation rates, these studies have almost exclusively been conducted with high spin-lock amplitudes greater than 23.5μT (equivalent to a frequency of more than 1000 Hz) and/or using ultrahigh-field (7T or higher) small bore scanners where specimen heating was not a concern or was not monitored^17–19. Such studies have identified a number of important factors²⁰ contributing to R_1ρ relaxation rates including solute concentration (glucose²¹ and glutamate¹⁷), pH²², temperature²³ and molecular rotational rate²⁴. Other contributions to R_1ρ relaxation rates have been identified including interactions between large macromolecules and water via dipolar and diffusion relaxation pathways^25,26. Moreover, outcomes from R_1ρ relaxation rate studies depended on both field strength²⁷ and the amplitude of the applied spin-lock pulse²⁸. To better understand the contributions from several sources including proton exchange, diffusion, temperature, and pH Spear and Gore recently undertook an extensive study to explore their effect on R_1ρ relaxation rates²⁹. They showed that temperature and pH were two key factors that influenced the rate of proton exchange. In addition, they showed that the multiple pools of exchanging protons behave independently and that multiple R1ρ measurements can be used to derive an exchange rate constant.

The goal of this work was to better understand the role of proton exchange with compounds abundant in brain tissue and thought to potentially contribute to R_1ρ changes by building upon the fundamental framework of Spear and Gore. These studies may be helpful in understanding the findings previously observed in prior human studies at 3T that have identified differences in R_1ρ relaxation times between individuals with psychiatric and neurological disorders and controls. Using well-controlled phantoms, three experiments were performed to investigate the effects of changing the chemical environment in the presence of proteins on R_1ρ relaxation rates. This was done by adjusting pH (known to alter rate of amide proton transfer) within the phantom as well as changing the number of amide, hydroxyl, and amine exchangeable sites by adding glucose and lysine in the physiological range of concentration. These studies were performed in a 3T MRI scanner across a range of temperatures (9–34°C) while maintaining the specific absorption rate (SAR) levels within allowed FDA levels. Based on our previous R_1ρ imaging work in phantoms and animals³⁰, we hypothesized that at 3T R_1ρ would exhibit a greater sensitivity to pH as compared to the other metabolites studied.

MATERIALS & METHODS

Phantom Preparation

Phantoms were created that allowed for the systematic variation of pH, glucose, and lysine concentrations while controlling temperature. The spherical shell of a Magphan® EMR051 phantom (The Phantom Laboratory; Greenwich, NY) was used for this study. The interior of each phantom housed three fiberglass plates that were printed to hold seven 50mL Falcon tubes inside the sphere. The plugs at either end of the phantom were replaced with nylon barbs used to connect tygon tubing to the phantom providing a constant temperature water bath to the tubes inside the phantom. The other end of the hoses was connected to a water recirculating system (Endocal RTE-110, NESLAB) housed outside of the scan room (Supplementary Materials, Figure S1).

Experiments were performed using four separate phantoms, which contained various concentrations of pH, glucose, lysine, and water (control) added to 45 mL liquid egg-white albumin (HyVee generic brand, 0.124mM of proteins). The first phantom tested the effects of pH on R_1ρ. Varying amounts of hydrochloric acid (HCl, 1N) were added to different Falcon tubes to produce pH values across a physiologically relevant range (pH 6.01, 6.51, 7.02, 7.25, 7.51, 7.79 and 8.0). pH was confirmed using an Accumet AB15 pH meter (Fisher Scientific; Pittsburg, PA). The second phantom tested the effects of changing the concentration of hydroxyl exchange sites by changing glucose concentration. Glucose concentration was varied from 0, 5, 10, 15, 20, 30, and 40 mM (pH 9.03 for all vials). The third phantom tested the effects of changing the number of amine exchange sites. Lysine concentration was varied from 0, 0.5, 1.0, 1.5, 2.0, 2.5, and 3.0 mM (pH 9.03 for all vials). The pH, glucose, and lysine concentrations were selected to encompass the physiological range expected in the in vivo brain. The first three phantoms allowed for the exploration of proton exchange mediated by changing the rate of exchange versus changes in the number of exchangeable sites. The fourth phantom was a water control phantom that contained two sets of three vials diluted with either water or HCl (1N) (1, 2, and 3mL). The seventh vial contained only liquid egg whites. pH was then measured in the tubes, and the pH for the water-containing vials was 9.03, 9.04, and 9.05 while the HCl-containing vials had pH values of 7.42, 6.46 and 5.93, respectively. The tube containing only egg whites had a pH of 9.00.

MR Imaging

All MR imaging was performed on a Siemens 3T TIM Trio MRI scanner (Erlangen, Germany). The spherical phantom was placed in a circularly polarized head coil. The thermostatic bath was then set to the appropriate temperature (9, 22, 34°C) for the imaging experiment and allowed to circulate for 30 minutes before initiating imaging. Temperature within the phantom was measured at the start and end of each imaging experiment. Imaging included a three-plane localizer, B₀ mapping, B₁ mapping, and R_1ρ, R₂, and R₁ relaxation rate mapping. R_1ρ mapping was collected in the coronal plane using a continuous-wave spin-lock preparation pulse and adiabatic excitation in a turbo spin-echo sequence with the following parameters: TR = 5000 ms, TE = 12 ms, slice thickness = 5.0 mm, FOV = 22.0×22.0 cm, matrix = 256×256, bandwidth = 130 Hz/pixel, time of spin-lock (TSL) = 0 and 100 ms, turbo factor = 4. Johnson et al. have shown that optimal SNR can be achieved with two spin-lock times³¹, thus the spin-lock times here were chosen while maintaining SAR within FDA limits. At room temperature, the egg-white medium had an R_1ρ of 3.92. R₂ mapping was acquired using Malcom-Levitt (MLEV) preparation with four refocusing hard pulses, which provided effective echo times of 0 and 100 ms. All other imaging parameters were the same as the R_1ρ based turbo spin-echo sequence. R₁ mapping was collected using a coronal inversion recovery (IR) sequence with the following parameters: TR = 5000 ms, TE = 12 ms, slice thickness = 5.0 mm, FOV = 22.0×22.0 cm, matrix = 256×256, Bandwidth = 130 Hz/pixel. Sequential acquisitions were performed with TI = 50, 150, 250, 450, 750, and 1150 ms. Three separate measurements were obtained for R_1ρ, R₂ and R₁ in each phantom preparation and temperature with different vial placements for each measurement.

For the pH, glucose, and lysine phantoms, R_1ρ imaging was performed at four spin-lock field strengths (4.7, 9.4, 14.1, and 18.8μT) corresponding to frequencies of 200, 400, 600 and 800 Hz to assess sensitivity across a variety of spin-lock amplitudes that may be applied in vivo. These phantoms were imaged at 9, 22, and 34°C. The water control phantom was assessed using only the 9.4μT spin-lock pulse at 22°C.

MR Image Analysis

To generate the relaxation maps, voxel-by-voxel calculation of the relaxation rates was performed using MATLAB (MathWorks; Natick, MA). R_1ρ and R₂ were calculated by fitting the respective TSL or TE to a single exponential decay model (Eq. 2), and R₁ was calculated using a single exponential recovery model as specified in Equation 3.

$$R_1=\frac{1}{T_1}=-\ln \left(\raisebox{1ex}{$(S_0-S_{TI})$}\!\left/ \!\raisebox{-1ex}{$2S_0$}\right.\right)/TI$$ (3)

Once the relaxation maps were generated, regions of interest (ROI) were manually defined for each tube in MATLAB (Fig. 3). The resulting ROIs were placed in regions where the B₀ field map was between 0.5–1.2Hz and the B₁ map was between ±10°. The median value and standard deviation were obtained from each ROI. This was done independently for each of the repeated measurements, relaxation property, and phantom preparations. This allowed us to evaluate both the sensitivity as well as the reliability of the measurements. To visualize the results, the plots were normalized to one of the metabolite concentrations. The relaxation parameters were normalized to the results obtained at 7.02 (pH), 15mM (glucose), and 1.5mM (lysine), respectively. The change in the relaxation rates relative to these reference concentrations were plotted as percent changes. Error propagation was used to translate the standard deviation at each vial onto the percentage change plots.

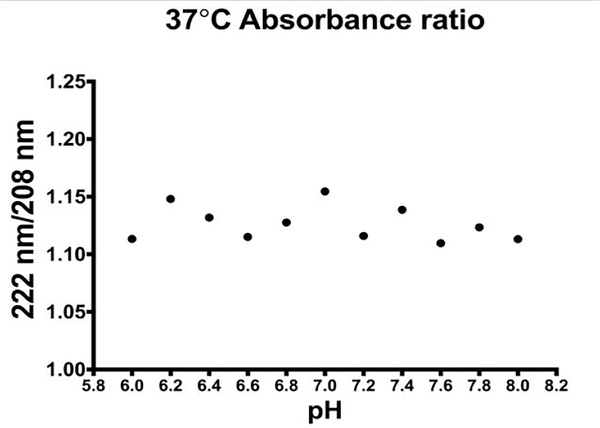
Figure 3.

Results from the circular dichroism experiment showing the ratio of absorbance between 222 and 208 nm wavelengths. The results show no change in the protein folding across the pH range studied.

Circular Dichroism Experiment

To determine if the proteins in the pH phantom were denatured as result of adding HCl to the egg white albumin, circular dichroism measurements were obtained. Eleven vials (50mL) were produced with egg white albumin, phosphate buffered saline solutions at pH ranging from 6 to 8 with steps of 0.2 by doping the solutions with HCl and sodium hydroxide (NaOH). At temperatures similar to those used in the imaging experiments (4, 22, 37°C), circular dichroism spectra were obtained in the samples at wavelengths 190–260. These measurements were repeated 5 times. A ratio of absorbance at 222 and 208nm was used to assess if changes in protein folding were observed³².

Two-Pool Model Fit to Relaxation Measurements

To estimate the type of solute protons responsible for the pH sensitivity of the R_1ρ measurements, a two-pool proton exchange model, based on the work of Chopra et al.²⁷ and adapted by Cobb et al.^33,34, was fit to the R_1ρ relaxation measurements. This model describes R_1ρ for protons undergoing two-site exchange as follows ^29,35:

$$R_{1\rho }=\frac{1}{T_{1\rho }}=\frac{R_2{S}_p^2+R_{1\rho }^{\mathrm{\infty }}{\omega }_1^2}{S_p^2+{\omega }_1^2};S_p^2=\frac{R_{1b}+r_b}{R_{2b}+r_b}({(R_{2b}+r_b)}^2+\mathrm{\Delta }{\omega }_b^2).$$ (4)

In Equation 4, R₂ is the transverse relaxation rate for the system and R_1ρ^∞ is the value of R_1ρ when then spin lock amplitude approaches infinity. R_1b (=1/T_1b) and R_2b (=1/T_2b) are the longitudinal and transverse relaxation rates of bound protons in the laboratory frame. The proton exchange rate is r_b and Δω_b is the chemical-shift of the solute protons (from the water frequency) while ω₁ is the applied spin-lock amplitude.

For each pH value, Equation 4 was fit to the experimental R_1ρ measurements as a function of the applied spin-lock amplitude, with Δω_b and r_b set to values appropriate for exchange with amide protons (Δω_b =3.35ppm, r_b =20s⁻¹)³⁶, hydroxyl groups (Δω_b =1.25ppm, r_b =1500s⁻¹)³⁶, and amines (Δω_b =3ppm, r_b =4000s⁻¹)³⁶. The relaxation rates of amide protons (R_1b = 0.51 s⁻¹ and R_2b = 4.19 s⁻¹), glucose (R_1b = 0.20 s⁻¹ and R_2b= 0.50 s⁻¹), and lysine (R_1b = 0.50 s⁻¹ and R_2b = 1.00 s⁻¹) as measured by Sykes et al. for ovalbumin at B₀=100MHz (2.35T)³⁷ were used. The fitnlm function in MATLAB was then used to generate a non-linear fit of Equation 4 to the R1ρ dispersion measurements at each pH, solving for $R_{1\rho }^{\infty }$, R₂, and r_b. The values specified above for r_b was used as an initial condition for the fitting procedure while initial values were assigned to R₂ and $R_{1\rho }^{\infty }$ based on the R₂ and R₁ relaxation rates observed in the MR imaging experiments. These values were just initial conditions and the parameters were then allowed to float during model fitting. The resulting estimates for the relaxation rates were compared to those obtained experimentally.

RESULTS

Example R_1ρ relaxation rate maps obtained in the pH, glucose, and lysine phantoms are shown in Figure S2 of the Supplementary Materials. ROI based measurements obtained from these maps for R_1ρ, R₂, and R₁ relaxation rates are summarized below. Examples of the B₀ maps are shown in Figure S3 of the Supplementary Materials.

pH Phantom

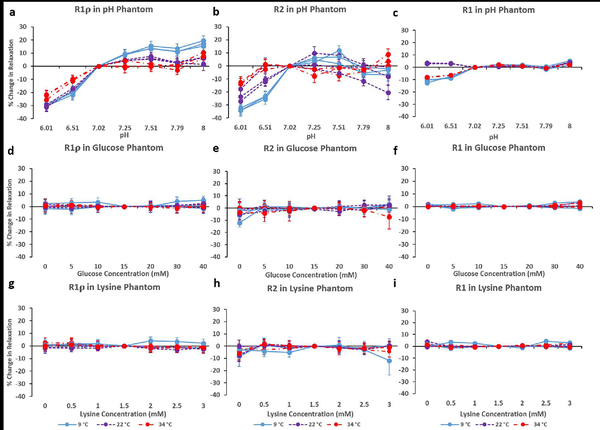
R_1ρ mapping of the pH phantom showed the expected sensitivity to pH. Relaxation rate percent change plots for R_1ρ with 400Hz spin-lock amplitude are shown in Figure 1a. The sensitivity to pH was observed across the entire experimental temperature range and at 34°C R_1ρ showed the greatest sensitivity to pH changes as compared to R₂ and R₁ relaxation. Changes in R_1ρ relaxation rates did show a subtle dependence on temperature with a larger decrease observed at 9°C (approx. −30%) as compared to 34°C (approx. −25%) across the pH range studied (6.0–8.0). The R_1ρ relaxation rates ranged from 2.18 to 3.7 s⁻¹ at 9°C and from 2.41 to 3.4 s⁻¹ at 34°C. Little difference was observed between the R_1ρ relaxation rates at 34°C and 22°C. In the water control phantom, small changes in the water concentration did not change the R_1ρ relaxation rates (Supplementary Materials Figure S4).

Figure 1.

Along the columns, change in R_1ρ and R₂ and R₁ values are shown for the pH (a,b,c), glucose (d,e,f), and lysine (g,h,i) phantoms respectively, Measurements were obtained at three temperatures (9, 22, and 34 °C) and three independent measurements were obtained for each condition and relaxation parameter. Data for 9 °C is shown as a solid line (light blue), 22 °C is a dashed line (purple), and 34 °C is a dot/dashed line (red).

R₂ relaxation rates were sensitive to pH in the phantom similar to those observed for R_1ρ relaxation rates (Figure 1b). However, the R₂ experiments showed a greater temperature dependence. A 35% decrease in R₂ relaxation rates at pH 6.0 relative to pH 7.02 was observed at 9°C, which as reduced to 12% at 34°C. Only small variations (approx. 5%) in the R₂ relaxation rates were observed above pH 7.0. Figure S5b in the Supplementary Materials shows the temperature sensitivity for the R₂ measurements.

The R₁ relaxation rates showed very little sensitivity to pH with decreases of less than 10% between pH 6.0 and 7.0 (Figure 1c). In addition, the pH sensitivity did not change with temperature.

Glucose Phantom

By adding varying concentrations of glucose to liquid egg-white solutions, the number of hydroxyl exchangeable sites was increased. Figure 1 shows the relative change plots of the relaxation rates acquired with respect to temperature. R_1ρ relaxation rates showed very little change in response to glucose concentration at three temperatures studied (Figure 1d). Across the glucose concentration range studied in this experiment, R_1ρ relaxation rates showed changes of 6%, 4%, and 5% at 9, 22, and 34°C respectively. Similar small changes were observed for R₂ (Figure 1e) and R₁ (Figure 1f) relaxation rates in the phantom at 34°C. At lower temperatures, variation in the R₂ relaxation rates were observed between the tubes containing 0 and 5mM concentrations of glucose, but little change was observed between 5 and 40 mM.

Lysine Phantom

Fast exchanging amine protons were added to liquid egg-white solutions by adding lysine at varying concentrations. All three of the quantitative relaxation measures (R_1ρ, R₂, and R₁) showed little sensitivity to the lysine concentrations added to the various tubes in the phantom (Figure 1, g–i). R_1ρ showed had 4% change across the full concentration range used in this study at 34°C.

Dispersion Data

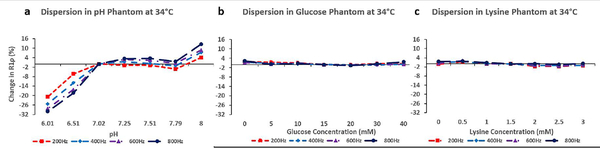
The impact of the R_1ρ spin-lock pulse amplitude was also explored in this study. The overall RF energy deposited in the body is limited by the FDA and practically, this limits the maximum amplitude of the spin-lock pulse to be 1000Hz or less to keep the RF levels below the FDA limit in a clinically acceptable acquisition time. The sensitivity of R_1ρ to changes in pH, glucose, and lysine concentrations was evaluated for spin-lock amplitudes ranging from 4.7 to 18.8 μT (200–800Hz) to determine if the sequence was sensitive to different exchange rates based on the amplitude of the spin-lock pulse. In Figure 2 the relative changes in R1ρ relaxation rates are shown for each phantom at 34°C. For R_1ρ, the 18.8 μT (800Hz) spin-lock produced the greatest change (approx. 43%) in R_1ρ from pH 7.01 to 6.01. The other spin-lock amplitudes also showed pH sensitivity but with a smaller change of 40% (14.2 μT), 35% (9.4 μT) and 20% (4.7 μT). R_1ρ relaxation rates showed no sensitivity to glucose and lysine in the phantoms across all the spin-lock amplitudes used in this study.

Figure 2.

Plots of relative change in R_1ρ at 34 °C with varying spin-lock frequency (dispersion). R1ρ dispersion is shown for the pH (a), glucose (b) and lysine (c) phantoms.

Circular Dichroism Measurements

To discern the mechanism underlying the sensitivity of R_1ρ imaging to pH, circular dichroism measurements were obtained. Figure 3 shows the absorbance ratio 222nm/208nm as a function of pH at 37°C. Across the pH range, there was only a slight variance in the absorption at 222nm when compared with the 208 nm reference wavelength. This means the absorption by the helical structures remained constant throughout the experiments. Thus, there was little or no denaturation of the proteins, suggesting the pH sensitivity of R_1ρ is not likely due to changes in protein conformation.

Two-Pool Model Fit to Relaxation Measurements

To evaluate the type of exchange processes contributing to the observed R_1ρ changes, the two-pool model described in Equation 4 was fit using parameters for amide protons, hydroxyl groups, and amines. The model behavior was only reasonable for the parameters associated with amide exchange. The model for amide exchange generated similar trends for the R₂ measurements as observed in the phantom (Table 1). Table 1 also includes the R₂ and R₁ relaxation rates measured from the imaging experiments for comparison. An error of approximately 8% was observed for the R₂ values generated from the model as compared to the experimental results. Measured R_1ρ values were consistently between measured R₂ and calculated $R_{1\rho }^{\infty }$ for the same conditions. The model fit for the $R_{1\rho }^{\infty }$ measurements were approximately a factor of 4 shorter than the observed R₁ values in the phantom. The model behavior for parameters suitable for amines and hydroxyl groups generated unrealistic values for the fitted parameters (i.e. $R_{1\rho }^{\infty }$ < 0, or extremely large r_b) and thus the corresponding models were considered to be invalid.

Table 1:

Two-Pool model estimates for exchange rate (r_b), transverse relaxation rate (R₂), and $R_{1\rho }^{\infty }$. R_1ρ, R₁, and R₂ relaxation rates measured from the imaging experiments at 34°C are shown as well.

pH	Phantom R1ρ (s−1)* 800 Hz SL	Phantom R1 (s−1)*	Model Fit ${\mathit{R}}_{\mathbf{1}\mathit{\rho }}^{\mathit{\infty }}$ (s−1)*	Phantom R2 (s−1)*	Model Fit R2(s−1)*	Exchange Rate rb (s−1)*
6.01	1.8±0.03	0.45±0.01	1.4±0.17	3.5±0.03	3.1±0.12	225±67
6.51	2.1 ±0.09	0.46±0.01	1.6±0.24	4.1±0.06	3.7±0.17	220±76
7.02	2.6±0.06	0.5±0.01	2.1±0.31	4.1±0.03	3.8±0.16	281±62
7.25	2.7±0.06	0.51±0.01	2.2±0.34	3.9±0.14	3.7±0.14	331±148
7.51	2.7±0.09	0.51±0.02	2.3±0.23	3.9±0.11	3.8±0.13	261±105
7.79	2.6±0.05	0.49±0.01	2.2±0.37	3.9±0.005	3.6±0.16	310±83
8	2.9±0.16	0.52±0.02	2.5±0.65	4.2±0.20	3.9±0.33	276±304

^*Mean ± Standard Deviation

DISCUSSION

We have previously observed R_1ρ relaxation rate changes in studies of psychiatric^7–9 and neurological disorders^2,5,6, which were interpreted as likely due to changes in brain pH and metabolism. This study was undertaken to better understand factors that may influence R_1ρ relaxation rates including pH, other solutes (glucose and lysine), as well as temperature. Since the manipulation of a single compound in vivo is challenging, this study used a physical phantom to systematically vary compound concentrations. Imaging was performed on a 3T MRI scanner while using spin-lock amplitudes within FDA SAR limits. The key findings from the experiments showed that R_1ρ relaxation was only sensitive to changes in pH while showing little to no sensitivity to glucose and lysine concentrations within the physiological range. Furthermore, the two-pool proton exchange modeling showed that this pH sensitivity was likely the result of amide proton exchange rates and not due to amine and hydroxyl exchange. R_1ρ relaxation showed the greatest sensitivity to pH near body temperature as compared to R₂ and R₁ relaxation rates. Finally, the results of this work were in good agreement with the work by Spear and Gore, which also found that pH and temperature were two of the primary contributing factors to changes in proton exchange²⁹. This work did extend the findings of Spear and Gore by using a more heterogenous protein media for the phantoms, a lower MR field strength, and a broader temperature range.

In the present study, a direct relationship between R_1ρ relaxation rates and pH was observed between 6.0–7.0 at all spin-lock amplitudes (4.7 to 18.8 μT) used. This relationship was stronger as the spin-lock amplitude increased and appeared to be reaching a plateau at 18.8μT. The higher spin-lock amplitudes (14.1 and 18.8μT) showed sensitivity to pH up to 7.5, which were not evident at the lower spin-lock amplitudes. We restricted the maximum spin-lock amplitude to value consistent with clinical SAR limitations while maintaining maximum spin-lock duration of 100ms. These findings are in good agreement with previous studies reported in the literature (Table 2)^{17,22,30,38–40}. A similar relationship between R_1ρ relaxation rates and pH has been observed with spin-lock amplitudes greater than 25μT (1065Hz)³⁹, but the greatest pH sensitivity were observed when the spin-lock amplitude was less than 23.5μT (1000Hz)⁴⁰ by Kettunen et al. as well as Spear and Gore²⁹. Magnotta et al. previously reported a similar relationship between R_1ρ and pH in agar phantoms, which contained potassium phosphate buffer (KPB) and bovine serum albumin (BSA) at 3T³⁰. The only difference between the results presented here and by Magnotta et al.³⁰ was the wider range of the R_1ρ sensitivity to pH likely due to differences in the proteins used for the phantom construction (BSA versus egg white ovalbumin). Finally, Willumsen observed exchange rate changes in the pH range 6.5–7.5 in aqueous ovalbumin media using deuterium experiments⁴¹.

Table 2:

Relationship between amine, hydroxyl, and amine exchange and relaxation times from the literature spin-lock amplitudes (B₁) and static (B₀) field used in this study are included. The correlations are summarized in the table as positive (+), negative (−), or no correlation (↔). n/r signifies values not reported while empty cells were not measured in the study

Reference	Relaxation Trend with pH
	R1ρ	B1 (μT)	R2	R1	pH Range	B0 (T)
Kettunen et al.22	+	56.4	+		7.27–6.81 a	4.7
Magnotta et al.30	+	23.5			8.0–6.0b	4.7
Jokivarsi et al.38	+	40	+		7.31-n/r a	4.7
Mäkelä et al.39	+	25.1–27.7	+	-	8.0–5.5	4.7
Jin et al.17	+	2.9–94			7.5–6.0	9.4
Koenig et al.45				-	5.2–4.0	0.1
				-	7.4–6.5	0.1
				+	10–8.4	0.1
Meiboom et al.43			+	↔	8–6	0.7
			-	↔	10–8	0.7
Schilling et al.44			+	↔	7–6	2.4
			-	↔	7–8	2.4
Owusu et al.	+	4.7–18.8	+	+	8.0–6.0	3.0
	Relaxation Trend with Hydroxyl Protons				Concentration
Schuenke et al.42	+	5.0			5–20 mM	7.0
Jin et al.17	+	2.9–93.9			0–30 mM	9.4
Spear et al.29	+	1.2–235			0–200 mM	4.7,7.0
Owusu et al.	↔	4.7–18.8	↔	↔	0–40 mM	3.0
	Relaxation Trend with Amine Protons
Jin et al.17	-	2.9–93.9			0–20 mM b	9.4
Owusu et al.	↔	4.7–18.8	↔	↔	0–3.0 mM	3.0

^ain vivo data

^bagarose phantom.

R_1ρ showed little sensitivity to glucose and lysine in the presence of protein in the current study. In addition, no significant changes were observed in R₂ and R₁ relaxation rates. These findings contrast with previous work by Schuenke et al.⁴² and Jin and Kim¹⁷ conducted at ultra-high field. Both studies found a relationship between R_1ρ relaxation rates and hydroxyl concentrations by adjusting glucose levels while the work by Jin and Kim also found a relationship with amine group concentrations by adjusting glutamate levels. A possible explanation for the insensitivity to hydroxyl concentration changes in the current study may be due to the different field strengths employed across the studies. The strength of the main magnetic field affects the chemical shift of the solute protons. The chemical shift for the hydroxyl group hydrogen is close to water (approximately 100Hz at 3T) and higher field strengths will increase the chemical shift and thus the ability to differentiate these two pools. Thus, the sensitivity to proton exchange is dependent on the strength of the static field; the current study employed a 3T scanner, which is more than 2.5 times lower than the previous field strengths used. The effect of spin-lock amplitude on sensitivity to hydroxyl concentration changes was studied by Spear and Gore²⁹. Between spin-lock amplitudes of 200 to 800Hz, the authors showed a 40% increase in R_1ρ when the concentration of glucose was increased from 0 to 50mM. We were unable to detect this much change in R_1ρ signal. The decrease in SNR from spin-lock amplitude may have contributed to the lack of detection. Furthermore, a likely explanation for the R_1ρ insensitivity to changes in amine group concentrations in the current study is the low amplitude spin-lock pulses. Amine protons exchange at rates faster than that of amide protons and require higher spin-lock amplitudes for sensitivity than were achievable at 3T while adhering to FDA limits for subject heating.

The Two-Pool model based on Cobb et al.³³ for proton exchange suggests that the sensitivity of R_1ρ in these experiments to pH at spin-lock amplitudes available when performing human imaging studies (less than 23.5μT) is likely due to changes in amide exchange. The resulting model fit to the dispersion data was statistically significant and the resulting estimated parameters for R₂ exhibited similar trends as observed in our imaging experiments. Furthermore, the model fit using hydroxyl, and amine exchange parameters did not generate reasonable estimates for the relaxation measurements. It should be noted that the resulting model fit did not change significantly based on the initial values assigned to r_b, R₂, and $R_{1\rho }^{\infty }$. The calculated values for $R_{1\rho }^{\infty }$ were significantly shorter than the observed R₁ values. This indicates that additional factors may need to be added to the two-pool model to fully characterize the relaxation behavior. Finally, no changes in protein structure were observed based on the circular dichroism experiments.

In addition to R_1ρ sensitivity to pH, R₂ and R₁ relaxation also showed sensitivity to pH as summarized in Table 2. The R₂ relaxation measurements conducted in this study showed sensitivity to pH. This work agrees with prior studies, which have shown similar R₂ sensitivity to pH^35,43,44. Meiboom et al. attributed the pH sensitivity of R₂ relaxation rates to the breaking and forming of hydrogen bonds⁴³. In addition, we observed the greatest R2 sensitivity to pH at 9 °C which agrees with work by Chopra et al.³⁵ R₁ relaxation rates also exhibited a dependence on pH at 9°C and 34°C with little change at 22°C in the range of pH 6.0–7.0. The literature has reported mixed results related to R₁ sensitivity to pH with some prior studies reporting pH sensitivity^39,45 while others finding no pH sensitivity^43,45. These discrepancies may be related to the protein used, the ionic strength of the solutions in these experiments, the temperature, or pH range over which the measurements were made.

The results of this study suggest that changes in R_1ρ relaxation rates that have been previously observed in various psychiatric and neurological disorders using spin-lock amplitudes of less than 23.5μT (1000Hz) may be due to changes in pH. Furthermore, we have previously shown a 500s⁻¹ increase in the R_1ρ relaxation rate in bipolar disorder as compared to controls⁸. Based on the results obtained at 34°C in this study, this magnitude of a R_1ρ relaxation time change cannot be explained by hydroxyl and amine proton exchange based on the phantoms and modeling used in this study.

The pH, glucose and lysine concentration ranges tested here are broader than those occurring in brain tissue. However, there are limitations that need to be considered when extrapolating results from this phantom study to imaging of the brain in vivo. In egg white, there are two other proteins (ovotransferrin and ovomucoid) in solution apart from ovalbumin that exist in relatively large concentrations. The average acid dissociation constants (i.e. isoelectric point) of these proteins do not vary greatly (between 4–6). However, the brain expresses on the order of 15,000 proteins⁴⁶, with a range of isoelectric points. Different isoelectric points are likely to alter the relative pH sensitivity R_1ρ relaxation rate. Differences in temperature before and after scanning were minimized due to the re-circulator; however, temperature did drift slightly during the experiment. While this temperature change was limited to approximately 2° C, it may have added to some of the variations in the relaxation measurements observed in the phantoms. In biological tissue, however, proton exchange is not the only contributor to R1ρ change. Diffusion of protons through regions where magnetic susceptibility change have also been shown to affect R_1ρ considerably independent of proton exchange^47,48. Though this work was conducted at 7T, the effects of magnetic susceptibility are relevant at all static field strengths. Recently, Ali et al. have concluded that the sensitivity of R_1ρ to macromolecular change is significant and thus must be considered ⁴⁹. Due to pathophysiology, cellular density and metabolism will lead to changes in macromolecular concentration, which may also influence R1ρ relaxation times.

In conclusion, R_1ρ is more sensitive to physiological changes in pH than glucose or lysine concentration in a protein at 3T. Near physiologic temperature, R_1ρ is more sensitive to pH changes in the physiologic range than R₂ and R₁, although R₂ and R₁ also show some sensitivity to pH changes. R_1ρ results also reflected the pH dependence of exchange in proteins. This suggests that changes in acidity in the presence of proteins may be an underlying physiologic response driving the changes in R_1ρ observed in applications to neurologic or psychiatric disorders at 3T. Additionally, we show that spin-lock pulses at low amplitudes produce adequate contrast to see pH related changes in tissue, well below SAR limits.

Supplementary Material

FigureS1

Figure S1 a) Diagram of experimental setup for imaging with temperature control. The water circulation system is placed in the scanner control room and the phantom in the transmit-receive coil with tubing attached. Both phantom and coil are moved into the scanner bore before imaging. The directional arrows indicate the flow of water to and from the phantom. b) A cross-sectional view of the phantom with Falcon tubes inserted.

FigureS4

Figure S4 Relative change plot of R_1ρ relaxation rate in separate egg-white phantom containing HCl and water. The relative change data were at 22 °C.

FigureS3

Figure S3 Example B₀ maps of the (a) pH, (b) glucose and (c) lysine phantoms at 22 °C. The color scale represents the homogeneity of spins as the result of a 90-degree hard pulse. 0° represents a rightly tipped spin and ±40° represents over or under tipped spins according to the sign.

FigureS5

Figure S5 R_1ρ (a) and R₂ (b) relaxation rates in the pH phantom showing temperature dependence. Differences based on temperature are much more pronounced in the R₂ relaxation rates as compared to R_1ρ. The values plotted are averages of three repetitions at each temperature. Error bars here are based on standard deviations of the relaxation rates from the three repetitions at each pH.

FigureS2

Figure S2 Example of image quality obtained in the phantom for R_1ρ imaging in the a) pH, b) glucose and c) lysine phantoms. An example ROI used for the measurements is shown in the vial with the pH measured as 7.25. The median value within the ROI was used for the relaxation measurements.

ABBREVIATIONS

R₁

Longitudinal relaxation rate in the laboratory frame

R₂

Transverse relaxation rate in the laboratory frame

R_1ρ(ω)

Longitudinal relaxation rate in the rotating frame

T_1ρ

1/R_1ρ

TSL

Duration of the spin-lock pulse block

CEST

Chemical exchange saturation transfer

B₀

Static magnetic field

B₁

Magnetic component of the radiofrequency field

SAR

Specific absorption

Glc

Glucose

Glu

Glutamate

HCl

Hydrochloric acid

NaOH

Sodium hydroxide

BSA

Bovine serum albumin

k_ex

Proton exchange rate