Abstract
Magnetic Weyl semimetals have novel transport phenomena related to pairs of Weyl nodes in the band structure. Although the existence of Weyl fermions is expected in various oxides, the evidence of Weyl fermions in oxide materials remains elusive. Here we show direct quantum transport evidence of Weyl fermions in an epitaxial 4d ferromagnetic oxide SrRuO3. We employ machine-learning-assisted molecular beam epitaxy to synthesize SrRuO3 films whose quality is sufficiently high to probe their intrinsic transport properties. Experimental observation of the five transport signatures of Weyl fermions—the linear positive magnetoresistance, chiral-anomaly-induced negative magnetoresistance, π phase shift in a quantum oscillation, light cyclotron mass, and high quantum mobility of about 10,000 cm2V−1s−1—combined with first-principles electronic structure calculations establishes SrRuO3 as a magnetic Weyl semimetal. We also clarify the disorder dependence of the transport of the Weyl fermions, which gives a clear guideline for accessing the topologically nontrivial transport phenomena.
Subject terms: Electronic properties and materials, Magnetic properties and materials, Topological insulators
Despite various predictions, the evidence of Weyl fermions in oxide materials remains elusive. Here, the authors show evidence of Weyl fermions in quantum transport measurements in an epitaxial ferromagnetic oxide SrRuO3.
Introduction
Weyl fermions in a magnetic material have novel transport phenomena related to pairs of Weyl nodes1–5, and they are of both scientific and technological interest, with the potential for use in high-performance electronics, spintronics, and quantum computing. Although Weyl fermions have been predicted to exist in various oxides6–8, evidence for their existence in oxide materials remains elusive9–11. SrRuO3, a 4d ferromagnetic metal often used as an epitaxial conducting layer in oxide heterostructures12–15, provides a promising opportunity to seek the existence of Weyl fermions in a magnetic material. State-of-the-art oxide thin film growth technologies, augmented by machine learning techniques, may allow access to such topological matter. Here, we show direct quantum transport evidence of Weyl fermions in an epitaxial ferromagnetic oxide SrRuO3: unsaturated linear positive magnetoresistance (MR)16–20, chiral-anomaly induced negative MR1,16,21, π Berry phase accumulated along cyclotron orbits16,18–20, light cyclotron masses16–20,22–24 and high quantum mobility of about 10,000 cm2 V−1 s−1 16,17,22–27. We employed machine-learning-assisted molecular beam epitaxy (MBE)28 to synthesize SrRuO3 films whose quality is sufficiently high to probe their intrinsic quantum transport properties. We also clarified the disorder dependence of the transport of the Weyl fermions, and provided a brand-new diagram for the Weyl transport, which gives a clear guideline for accessing the topologically nontrivial transport phenomena. Our results establish SrRuO3 as a magnetic Weyl semimetal and topological oxide electronics as a new research field.
Weyl semimetals, which host Weyl fermions described by the Weyl Hamiltonian, have intriguing and fascinating transport phenomena based on the chiral anomaly and linear band dispersion with spin-momentum locking1–4,29, such as chiral-anomaly induced negative MR and high mobility16,17,21. Compared with space-inversion-symmetry-breaking Weyl semimetals30, time-reversal-symmetry (TRS)-breaking ones are thought to be more suitable for spintronic applications3,31,32. For example, since the distribution of Weyl nodes in magnets is determined by the spin texture1, this distribution is expected to be controlled by the magnetization switching technique33,34. Recent angle-resolved photoemission spectroscopy (ARPES) studies have found experimental evidence for the electronic structure of magnetic Weyl semimetals Co3Sn2S22,3 and Co2MnGa4, such as the presence of bulk Weyl points with linear dispersions and surface Fermi arcs. Demonstrating the relevance of Weyl fermions in a magnetic material to spintronic and electronic applications requires information on quantum oscillations, which allows us to characterize transport properties of individual orbits in a magnetic Weyl semimetal. However, systematic and comprehensive measurements of quantum transport, including the quantum oscillations, have been hampered in magnetic Weyl semimetals because of the difficulty in achieving a quantum lifetime long enough to observe them in metallic systems. Since specimens in the form of epitaxial films are advantageous for future device applications of magnetic Weyl semimetals, it is urgently required to prepare single-crystalline thin films35 of magnetic Weyl semimetals whose quality is sufficiently high to probe quantum transport properties.
Theoretically, the presence of Weyl fermions has been predicted for SrRuO3, a 4d ferromagnetic material7. SrRuO3 is widely used as an epitaxial conducting layer in oxide electronics and spintronics owing to the unique nature of ferromagnetic metal, compatibility with other perovskite-structured oxides, and chemical stability12–15,34. Theoretical studies predicted that the electronic structure of SrRuO3 may include a large number of Weyl nodes caused by the TRS breaking and spin–orbit coupling (SOC) (Fig. 1a)7, and suggested that the Berry phase from the Weyl nodes gives rise to an anomalous Hall effect (AHE) in it.7,10,36 However, a definitive conclusion on the presence of Weyl fermions near the Fermi energy (EF) cannot be drawn from observations of the AHE alone7,9,10, because the AHE reported so far for SrRuO3 can be well reproduced using a function composed of both intrinsic (Karplus–Luttinger (KL) mechanism) and extrinsic (side-jump scattering) terms37,38.
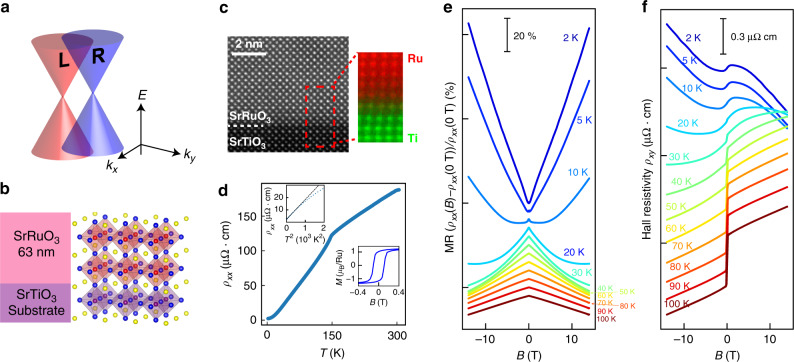
Fig. 1. Sample characteristics and temperature dependence of the magnetoresistance and Hall resistivity of SrRuO3.
a Schematic image of a pair of Weyl nodes with opposite chiralities (L and R). In ferromagnetic SrRuO3, the TRS breaking lifts the spin degeneracy and leads to linear band crossing at many k points, resulting in a pair of Weyl nodes with opposite chiralities. b Schematic of the sample and crystal structures of the SrRuO3 films (63-nm thick) on a SrTiO3 substrate. In the schematic crystal image, yellow, blue, red, and purple spheres indicate strontium, oxygen, ruthenium, and titanium, respectively. c Cross-sectional high-angle annular dark field scanning transmission electron microscopy (HAADF-STEM) image of the SrRuO3 film with the RRR of 71 taken along the [100] axis of the SrTiO3 substrate. The inset in c shows a color overlay of the electron energy loss spectroscopy (EELS)-STEM images for the Ti-L2,3- (green) and Ru-M4,5-edge (red). Epitaxial growth of the high-quality single-crystalline SrRuO3 film with an abrupt substrate/film interface is seen in the images. d ρxx–T curves for the SrRuO3 film with the RRR of 84.3. The left inset in d shows the ρxx vs. T2 plot with the linear fitting (black dashed line). We defined the Fermi liquid region as the temperature range where the experimental ρxx and the fitting line are close enough to each other (<0.1 μΩ cm). The right inset in d shows the magnetization M vs. B curve at 10 K with B applied in the out-of-plane [001] direction of the SrTiO3 substrate. e, f MR (ρxx(B) − ρxx(0 T))/ρxx(0 T) and Hall resistivity ρxy(B) curves at 2–100 K for the SrRuO3 film with the RRR of 84.3 with B applied in the out-of-plane [001] direction of the SrTiO3 substrate. In e and f, the MR and the Hall resistivity at each temperature have been offset by 7% and 0.22 μΩ cm, respectively, for easy viewing.
In this study, we conducted comprehensive high field magnetotransport measurements (see Methods section “Magnetotransport measurements”) including quantum oscillations of resistivity (i.e., Shubnikov-de Haas (SdH) oscillations), on an extraordinarily high-quality SrRuO3 film (63-nm thick) epitaxially grown on SrTiO3 (Fig. 1b, c and Supplementary Fig. 1a). Our first-principles electronic structure calculations predicted the presence of Weyl fermions within an energy range of −0.2 to 0.2 eV around the Fermi level in SrRuO3. To probe the contribution of the Weyl fermions to the transport properties, it is necessary to identify the following five signatures from the magnetotransport data: (1) unsaturated linear positive MR16–20 (2) chiral-anomaly induced negative MR1,16,21, (3) π Berry phase accumulated along the cyclotron orbits16,18–20, (4) light cyclotron mass16,18–20,22–24, and (5) high quantum mobility16,17,22–27. Although the light cyclotron mass, high mobility, and linear positive MR also exist in semiconductors with parabolic bands39–41, we confirmed the existence of the Weyl fermions in SrRuO3 by observing all of the five signatures.
Results
Temperature dependence of resistivity
The residual resistivity ratio (RRR), which is defined as the ratio of the longitudinal resistivity ρxx at 300 K [ρxx(300 K)] and T0 K [ρxx(T0 K)] (T: temperature), is a good measure to gauge the purity of a metallic system, that is, the quality of single-crystalline SrRuO3 thin films (see Methods section “Determination of the RRR in SrRuO3”). In fact, high RRR values are indispensable for exploring intrinsic electronic states. More specifically, RRR values above 40 and 60 have enabled observations of sharp, dispersive quasiparticle peaks near the Fermi level by ARPES42 and quantum oscillations via electrical resistivity43, respectively. To form SrRuO3 with quality exceeding current levels, we employed our recently developed machine-learning-assisted MBE (see Methods section “Machine-learning-assisted MBE”)28.
The resistivity ρxx vs. T curve of the SrRuO3 thin film shows a clear kink at the Curie temperature (TC) of ∼152 K (Fig. 1d)12, while the magnetization measurement at T = 10 K shows a typical ferromagnetic hysteresis loop (Fig. 1d, right inset). With a residual resistivity ρxx (T0 K) of 2.23 μΩ cm and an RRR of 84.3, SrRuO3 thin films grown by machine-learning-assisted MBE are superior to those prepared by any other method12,28,43,44. Below approximately 20 K, the T2 scattering rate () expected in a Fermi liquid is observed (Fig. 1d, left inset)12,42, indicating that the intrinsic transport phenomenon is seen below this temperature (hereafter called TF).
Temperature dependence of magnetoresistance and Hall resistivity
In this Fermi liquid region [T < TF (20 K)], a semimetallic behavior is seen in the MR (ρxx(B) − ρxx(0 T))/ρxx(0 T) (Fig. 1e) and Hall resistivity ρxy(B) (Fig. 1f) curves with the magnetic field B applied in the out-of-plane [001] direction of the SrTiO3 substrate. As shown in Fig. 1e, ρxx above TF shows the negative MR because of the suppression of magnetic scattering12,45,46, and the MR changes its sign below TF. Importantly, the positive MR at 2 K shows no signature of saturation even up to 14 T, which is typical of a semimetal16,47 and also commonly seen in Weyl semimetals1,16–20. Especially in the case of Weyl semimetals, linear energy dispersion of Weyl nodes is considered to be one of the most plausible origins of unsaturated linear positive MR48,49 (see Methods section “Excluding other possible origins of the positive MR in SrRuO3”). In addition, as shown in Fig. 1f, the ρxy(B) curves below TF are nonlinear, indicating the coexistence of multiple types of carriers (electrons and holes). We note that, below TF, the AHE, which stems from the extrinsic side-jump scattering and intrinsic KL mechanisms in SrRuO3,37,38 is well suppressed due to the small residual resistivity of the SrRuO3 film with the RRR of 84.3, and thus the ρxy(B) curves below TF are dominated by the ordinary Hall effect (see Methods section “Temperature dependence of the AHE in the SrRuO3 film with the RRR = 84.3”). Below 10 K, where the AHE is negligible, both the ρxy(B) values and the slopes of ρxy(B) change their signs in the high-B region, signaling the possibility of the coexistence of high-mobility electrons with low-mobility holes16. Importantly, both the unsaturated linear positive MR and nonlinear Hall resistivity features start to appear simultaneously when the measurement temperature is decreased to the Fermi liquid range [T < TF (20 K)]. This indicates that the unsaturated linear positive MR stems from the electron- and hole-like Weyl fermions.
Angle dependence of magnetoresistance
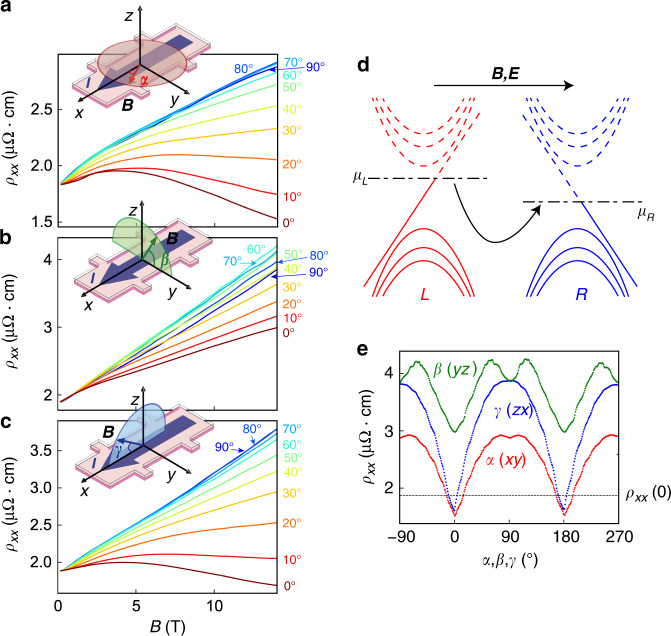
Next, we observed the chiral-anomaly induced negative MR, which is an important signature of Weyl fermions1,16,21,50. To clarify the anisotropic character of the chiral-anomaly induced negative MR thoroughly and systematically, we measured ρxx(B) at B angles α, β, and γ in the xy, yz, and zx planes, respectively (Fig. 2a–c). The rotation angles α, β, and γ are defined in the insets of Fig. 2a–c. When B is applied perpendicular to the current I (B⊥I, α = 90° or β = 0-90° or γ = 90°), the unsaturated linear positive MR is observed. The unsaturated linear positive MR seen here is expected owing to the presence of the Weyl fermions, and those states are supposedly anisotropic because of the 0.5% compressive strain of SrRuO3 induced by the SrTiO3 substrates28. This anisotropy is confirmed by varying β (Fig. 2b). In contrast, when B is rotated parallel to the current (B//I, α = 0° or γ = 0°), the MR turns negative and becomes linear above 8 T (Fig. 2a, c and Supplementary Fig. 4c). Theoretical calculations based on the semiclassical Boltzmann kinetic equation predict that TRS-breaking Weyl semimetals show a negative MR that is linear in B1,50, in comparison with the quadratic dependence expected for space-inversion-symmetry-breaking Weyl semimetals16,21. Thus, the observed linear increase of the negative MR is consistent with a chiral anomaly in magnetic Weyl semimetals. The chiral-anomaly induced negative MR can be understood from the violation of the conservation rules of chiral charges as shown in Fig. 2d50, and it should be maximum when B is exactly parallel to I (α = 0°, 180°, or γ = 0°, 180°). As expected, this anisotropic feature of the negative MR is observed at α = 0°, 180° or γ = 0°, 180° under 14 T (Fig. 2e). In addition, the peak structures in the angle dependence of the negative MR at B//I (α = 0°, 180° or γ = 0°, 180°) are similar to those in previous observations of the chiral anomaly in other Weyl semimetals1,16,21,51,52. These results confirm that this linear negative MR is induced by the chiral anomaly (see Methods section “Excluding other possible origins of the negative MR in SrRuO3”). As a consequence of the contributions from the positive MR and negative MR, ρxx(B) is lower than the zero field resistivity ρ0 = ρxx(0 T) when α and γ are near 0° or 180°.
Fig. 2. Chiral anomaly in the transport of SrRuO3.
a–c, MR ρxx(B) at B angles α (a), β (b), and γ (c) at 2 K for the SrRuO3 film with the RRR of 84.3. Angles α, β, and γ are defined in the insets of (a–c). d Schematic image of the chiral-anomaly induced negative MR. Red and blue bands represent Landau levels of a pair of Weyl nodes with opposite chiralities L and R, respectively. Non-orthogonal electric and magnetic fields (E·B ≠ 0) lead to the chiral charge transfer between the two Weyl nodes with opposite chiralities (L and R). The currents of the chiral charges are observed as a form of negative MR. Here, μL and μR indicate chemical potentials in the Weyl points with opposite chiralities. e α-, β-, and γ-angular dependences of the MR ρxx with B = 14 T at 2 K for the SrRuO3 film with the RRR of 84.3. The black dashed line indicates the ρxx (0 T) value. The region below the black dashed line shows negative MR.
Quantum oscillations
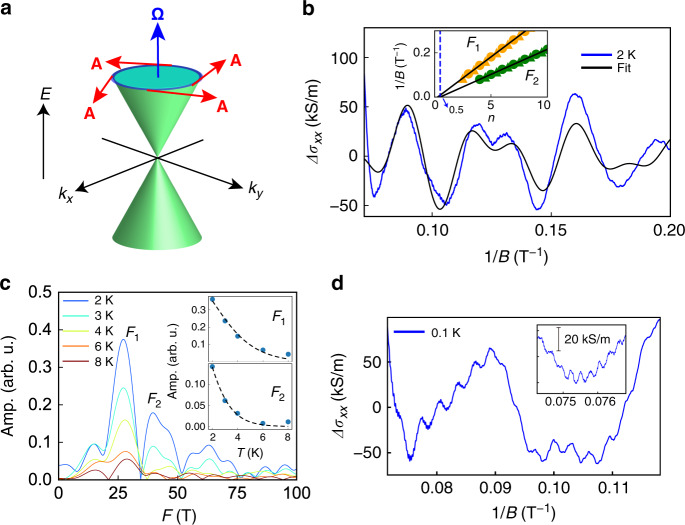
The Berry phase has become an important concept in condensed matter physics over the past three decades, since it represents a topological classification of the system and it plays a fundamental role in various phenomena, such as electric polarization, orbital magnetism, etc.53. However, revealing the π Berry phase, originating from a band touching point of the Weyl node16, has been challenging in magnetic Weyl semimetals. Here, we detect the π Berry phase accumulation along the cyclotron orbits, for the first time in magnetic Weyl semimetals, by measurement techniques sensitive to the quantized energy levels, i.e., SdH oscillation. The nontrivial π Berry phase, which is acquired by a surface integral of the Berry curvature Ω over a closed surface containing a Weyl point in the k-space (Fig. 3a)16,18–20, causes a phase shift of quantum oscillations. According to the Lifshitz–Kosevich (LK) theory, the magnitude of the SdH oscillation is described as16,18–20,54
| 1 |
where Δσxx is the oscillation component of the longitudinal conductivity, Ai is the normalization factor, Xi = 2π2kBT/ħωci, kB is the Boltzmann constant, ħ is the reduced Planck constant, ωci is the cyclotron frequency defined as eB/mi*, mi* is the cyclotron mass, TDi is the Dingle temperature, Fi is the frequency of the SdH oscillation, and 2πβBi is the phase shift caused by the Berry phase as mentioned above. The subscript i is the label of an orbit of carriers. Note that, even when a large number of carriers in 3D multiband systems pin the chemical potential, the linear dispersion of the Weyl fermions leads to an unconventional phase shift in the SdH oscillation (see Methods section “Effect of the Fermi-level pinning on the phase shift in the SdH oscillation”). To extract the Berry phases in SrRuO3, we used the LK formula [Eq. (1)] for two frequencies to fit the SdH oscillation data. Figure 3b shows the SdH oscillation data at 2 K and the fitting results by Eq. (1). (see Methods section “Data pretreatment for quantum oscillations”). The oscillation spectrum is considerably complex because of the contribution from several subbands7. To reduce the fitting parameters, we first carried out fast Fourier transform of the SdH oscillation (Fig. 3c), and extracted the F1 (26 T), F2 (44 T), m1* (0.35m0), and m2* (0.58m0) (m0, electron rest mass) values from the peak positions and the temperature dependence of F1 and F2 based on the LK theory (Fig. 3c, insets). Small masses are expected for Weyl fermions, which would fulfill the light cyclotron mass signature16,18–20,22–24. From the fitting to the data at 0.07 T−1 < 1/B < 0.2 T−1 shown in Fig. 3b, we obtained TD1 = 0.63 K, TD2 = 0.34 K, βB1 = 0.27, and βB2 = 0.48. The 2πβB2 value of 0.96π indicates the presence of the nontrivial π Berry phase arising from the mass-less dispersion of the Weyl fermions (see Methods section “Exclusion of other possible mechanisms of the phase shift in SrRuO3”). Although the interpretations of phase shifts between 0 and π in topological materials are still controversial, the 2πβB1 value of 0.54π implies that the energy dispersion of the F1 orbit has both quadratic (trivial) and linear (nontrivial) features55,56. These results cannot be reproduced by fixed zero Berry phases, confirming the existence of a nonzero Berry phase (Supplementary Fig. 6a).
Fig. 3. Quantum oscillations of SrRuO3.
a Schematic image of energy dispersion near a Weyl point. Blue and red arrows represent the Berry curvature Ω and the Berry connection A, respectively. The π Berry phase is accumulated along the cyclotron orbits (blue circle). b SdH oscillation measured at 2 K with B (5 T < B < 14 T) applied in the out-of-plane [001] direction of the SrTiO3 substrate for the SrRuO3 film with the RRR of 84.3. Black curve is the fitting curve of Eq. (1). The fitting was carried out by a nonlinear least squares method with the fitting parameters A1, β1, TD1, A2, β2, and TD2. The inset shows fan diagrams of two oscillation components of F1 (=26 T) and F2 (=44 T), which are shown as orange and green symbols, respectively. Here, the circles and triangles indicate integer and half-integer indexes of the oscillation components. c Fourier transform spectra of the SdH oscillations at 2–8 K. Insets in c show the mass estimations of the F1 and F2 orbits according to the LK theory. Black dashed curves are the fitting curves. d SdH oscillation observed at 0.1 K for the SrRuO3 film with the RRR of 84.3. The inset in d shows a close-up at around 0.075 T−1. The oscillation holds four kinds of other trivial orbits with higher frequencies.
The SdH oscillations not only give an insight into the topological nature, but also provide evidence of very high mobility of the Weyl fermions enclosing the Weyl points. We quantitatively determine the mobility of the charge carriers of the F1 and F2 orbits by calculating the quantum mobility, µqi = eħ/(2πkBmi*TDi). The obtained µq1 and µq2 values are 9.6 × 103 and 1.1 × 104 cm2 V−1 s−1, respectively. In addition, assuming isotropic Weyl nodes, we can estimate the carrier concentrations ni = (n1 = 3.81017 and n2 = 8.31017 ) and the chemical potentials µi = eħFi/mi* (µ1 = 8.5 meV and µ2 = 8.8 meV).57 These results mean that F1 and F2 come from the high-mobility and low-concentration Weyl fermions enclosing the Weyl points.
In addition to the Weyl fermions as evidenced by the five signatures described above, there are trivial Ru 4d bands crossing the EF in SrRuO37,12,42,43. SdH experiments performed at 0.1 K (Fig. 3d) confirmed some of these trivial Fermi pockets with F3 = 300 T, F4 = 500 T, F5 = 3500 T, and F6 = 3850 T (see Methods section “SdH oscillations of trivial orbits”). These four orbits have heavier masses (>2.8m0) than those of F1 and F2, which are consistent with the reported values for SrRuO312,43. The Fermi pocket areas of F3 (0.029 Å−2) and F4 (0.048 Å−2) are close to those of the 364 T (0.035 Å−2) orbit reported in earlier de Haas–van Alphen measurements58, and the Fermi pocket areas of F5 (0.334 Å−2), and F6 (0.368 Å−2) correspond to the 1 band (0.33–0.37 Å−2) observed by the ARPES and in earlier SdH measurements42,43. Noteworthy is that the Fermi pocket areas of F1 (0.0025 Å−2) and F2 (0.0042 Å−2) are more than ten times smaller than those of the trivial orbits, indicating that F1 and F2 stem from the small Fermi pockets as a feature of the Weyl fermions.
Disorder dependence of transport properties
Observing the intrinsic transport signatures of the Weyl fermions requires a high-quality SrRuO3 sample, since it is easily hindered by disorders such as defects and impurities. To show the disorder dependence of the transport phenomena in SrRuO3, we investigated the RRR dependence of TC, TF, and the highest temperature where the linear positive MR, one of the clear features of Weyl fermion transport in SrRuO3, remains (hereafter called TW) (Fig. 4a–c) (see Methods section “RRR dependence of the ferromagnetism, Fermi liquid behavior, and Weyl behavior”). As shown in Fig. 4c, the ferromagnetism becomes weaker (TC < 150 K) below RRR = 8.93, the Fermi liquid behavior remains regardless of the RRR values even with low RRR = 6.61, and the positive MR is observed over the RRR = 19.4. It is remarkable that the positive MR ratio at 9 T increases with increasing RRR (Fig. 4a, b), which indicates that the Weyl fermions become more dominant in the transport. In the RRR dependence of the Hall resistivity, ρxy(B), the nonlinear B dependence becomes more prominent with a sign change in dρxy/dB with increasing RRR (see details in Methods section “RRR dependence of the Hall resistivity”). Thus, a high-quality SrRuO3 sample is essential for observing the intrinsic transport of the Weyl fermions, and the diagram presented in Fig. 4c will be an effective guideline for realizing topologically nontrivial transport phenomena of the Weyl fermions to connect magnetic Weyl semimetals to spintronic devices31,32.
Fig. 4. RRR dependence of the transport phenomena in SrRuO3.
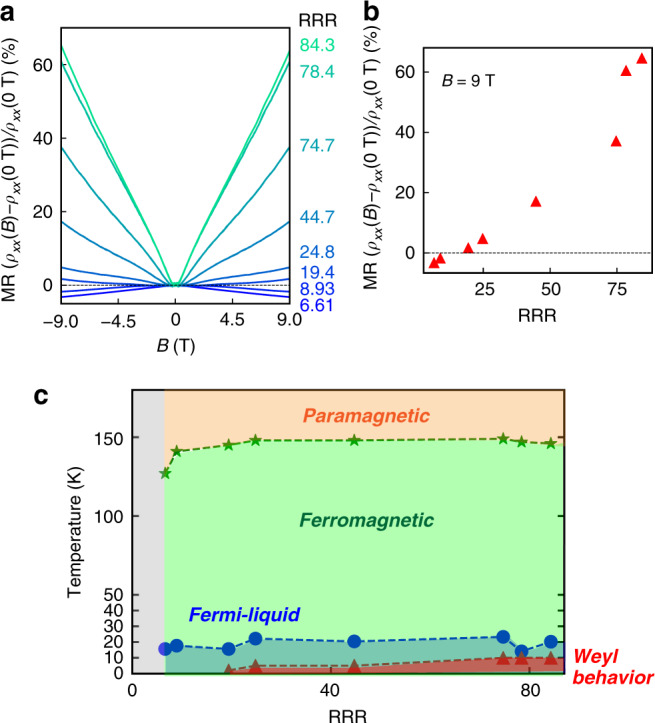
a RRR dependence of the MR (ρxx(B) − ρxx(0 T))/ρxx(0 T) measured at 2 or 2.3 K. When RRR > 19.4, the positive MR, which is one piece of experimental evidence of the Weyl fermions in SrRuO3, clearly emerges. b RRR dependence of the MR (ρxx(B) − ρxx (0 T))/ρxx (0 T) at B = 9 T and T = 2 or 2.3 K. c Diagram of the RRR dependence of TC, TF, and TW, which are shown as green stars, blue circles, and red triangles, respectively.
Electronic structure calculations
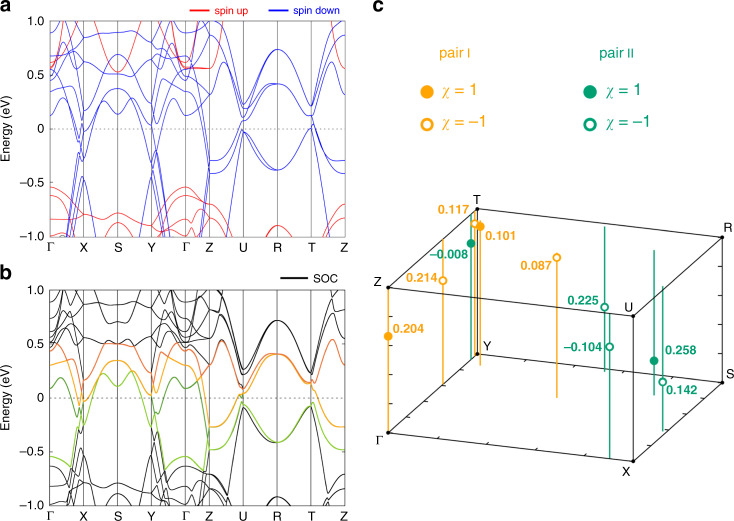
Thus far, the magnetotransport data of a high-quality SrRuO3 shows all of the expected marks of a magnetic Weyl semimetal. For certainty and theoretical rigor, we performed first-principles electronic structure calculations (see Methods section “Computational details”) to analyze the energy dispersion of SrRuO3. The calculated electronic structure for the orthorhombic Pbnm phase of SrRuO3 for the ferromagnetic ground state shows a half-metallic behavior (Fig. 5a) which agrees with previous electronic structure calculations59. The bands near the Fermi level are formed by the t2g Ru states hybridized with the O 2p orbitals (Supplementary Fig. 8a). The calculated magnetic moment per Ru ion (~1.4 , as tabulated in Supplementary Table 2) is close to the experimental saturation magnetic moment of 1.25 /Ru of our SrRuO3 films.28 We observe that in the ferromagnetic phase the Ru spins tend to align along the crystallographic b axis reducing the symmetry of the system from D2h to C2h. To identify the existence of the Weyl points (the band crossing points that carry chiral charge) by evaluating the outward Berry flux enclosed in a small sphere, we examined each band crossing of two pairs of bands I and II, shown in Fig. 5b, near the Fermi level in the presence of SOC with the magnetization along the orthorhombic c-axis. The resulting Weyl points are shown and listed in Fig. 5c and Supplementary Tables 3 and 4, respectively. We identified a total of 29 pairs of Weyl points with opposite chirality in the full Brillouin zone (BZ). An earlier theoretical study also made similar predictions of the existence of Weyl points in the case of a cubic structure of SrRuO3.7 Most of these Weyl points are found to exist within an energy range of −0.2 to 0.2 eV around the Fermi level. Among them, for WPz61–4 (8–16 meV) in Supplementary Table 4 is very close to the experimental chemical potentials of the Weyl fermions estimated from the SdH oscillations (µ1 = 8.5 meV and µ2 = 8.8 meV for the F1 and F2 orbitals, respectively). The Weyl points near the Fermi level are expected to contribute to all the observed quantum transport phenomena reported in this study. It is important to note that a small monoclinic distortion induced by the substrate does not significantly change the band structure of SrRuO3 and that Weyl points are robust with the distortion as long as the inversion symmetry is present (see Method section “First-principles calculations of Weyl points”), leading to a congruence of the experimental findings with theoretical predictions.
Fig. 5. Predicted Weyl points in the orthorhombic Pbnm (D2h) phase of SrRuO3.
a Band structure for the ferromagnetic ground state without spin-orbit coupling as obtained from GGA + U calculations with U = 2.6 eV and J = 0.6 eV. b Band structure for the ferromagnetic state with spin–orbit coupling and the magnetization along the orthorhombic c-axis obtained from GGA + U + SOC calculations with U = 2.6 eV and J = 0.6 eV. Two pairs of bands I (orange and dark orange bands) and II (green and dark green bands) are selected for calculating the corresponding chirality at each band crossing point. c Positions of the Weyl points in the irreducible part of the Brillouin zone calculated for two pairs of bands I (orange filled and open circles for = 1 and −1, respectively) and II (green filled and open circles for = 1 and −1, respectively). Numbers next to the points indicate their energy distance from the Fermi level (E − EF, where EF is the Fermi energy) in eV unit. Vertical orange and green lines indicate the in-plane (Γ–X–S–Y plane) positions of the Weyl points for easy viewing. Note that the orange and dark green bands are degenerate at the border of the Brillouin zone (X–S–Y and Z–U–R–T–Z).
In conclusion, we have observed the emergence of Weyl fermions in epitaxial SrRuO3 film with the best crystal quality ever reported12, which was grown by machine-learning-assisted MBE. Experimental observation of the five important transport signatures of Weyl fermions—the linear positive MR, chiral-anomaly induced negative MR, π phase shift in a quantum oscillation, light cyclotron mass, and high quantum mobility of about 10,000 cm2 V−1 s−1—combined with first-principles electronic structure calculations establishes SrRuO3 as a magnetic Weyl semimetal. In addition, the RRR dependences of ferromagnetism, Fermi liquid behavior, and positive MR serve as a road map to merge two emerging fields: topology in condensed matter and oxide electronics. Our results will pave the way for topological oxide electronics.
Methods
Magnetotransport measurements
We first deposited the Ag electrodes on a SrRuO3 surface. Then, we patterned the samples into 200 × 350 μm2 Hall bar structures by photolithography and Ar ion milling. Resistivity was measured using the four-probe method at 100 μA in a Physical Property Measurement System (PPMS) DynaCool sample chamber equipped with a rotating sample stage. Low-noise measurements below 1 K were performed by an AC analog lock-in technique, and the sample was cooled down in a 3He–4He dilution refrigerator.
Determination of the RRR in SrRuO3
The RRR was determined as the ratio of the longitudinal resistivity ρxx at 300 K [ρxx(300 K)] and T0 [ρxx(T0 K)]. SrRuO3 exhibits Fermi liquid behavior at a low temperature, which is characterized by a linear relationship between ρxx and T2.12,42 Based on this relationship, we estimated ρxx (T0 K) by extrapolating the ρxx vs. T2 fitting line below 10 K (Supplementary Fig. 1b).
Machine-learning-assisted MBE
We grew the high-quality SrRuO3 films (63-nm thick) on (001) SrTiO3 substrates in a custom-designed MBE setup equipped with multiple e-beam evaporators for Sr and Ru (Supplementary Fig. 2a). We precisely controlled the elemental fluxes, even those of elements with high melting points, e.g., Ru (2250 °C), by monitoring the flux rates with an electron-impact-emission-spectroscopy sensor and feeding the results back to the power supplies for the e-beam evaporators. The oxidation during growth was carried out with ozone (O3) gas. O3 gas (15% O3 + 85% O2) was introduced through an alumina nozzle pointed at the substrate. Further information about the MBE setup and preparation of the substrates is described elsewhere60. The surface morphology of our SrRuO3 films is composed of atomically flat terraces and steps, as observed by atomic force microscopy28. Together with Laue fringes in the θ–2θ X-ray diffraction patterns28, this indicates the high crystalline order and large coherent volume of our SrRuO3 films.
Fine-tuning of growth conditions is essential but challenging for high-RRR SrRuO3 growth. Therefore, only a few papers have reported SrRuO3 films with RRRs over 50.28,43,44 While a conventional trial-and-error approach may be a way to optimize the growth conditions, this is time-consuming as well as costly, and the optimization efficiency largely depends on the skill and experience of individual researchers. To avoid such a time-consuming approach and reduce experimental time and cost, we employed machine-learning-assisted MBE, which we developed in previous research28. Here, three important growth parameters [Ru flux rate, growth temperature, and nozzle-to-substrate distance (Supplementary Fig. 2a)] were optimized by a Bayesian optimization (BO) algorithm, which is a sample-efficient approach for global optimization61. This algorithm sequentially produces the three parameter values at which a high RRR value is predicted given past trials.
Supplementary Figure 2b shows the procedure for machine-learning-assisted MBE growth of the SrRuO3 thin films based on the BO algorithm. Here, we optimized each parameter in turn using BO. We first chose one of the growth parameters to update and fixed the rest, ran the BO algorithm to search the growth parameter, and then switched to another growth parameter. This is because BO can be inefficient in large dimensions due to the difficulty of predicting the outcome value for unseen parameters. Here, RRR = S(x) is the target function specific to our SrRuO3 films, and x is the growth parameter (Ru flux rate, growth temperature, or nozzle-to-substrate distance). BO constructs a model to predict the value of S(x) for unseen x using the result of past M trials , where xm is the mth growth parameter and RRRm is the corresponding RRR value. Specifically, we use the Gaussian process regression (GPR) as a prediction model28,62. GPR predicts S(x) as a Gaussian random variable following N (, ). This means and are calculated from x and the M data points. Subsequently, we choose the growth parameter x in the next run such that the expected improvement (EI)63 is maximized. EI balances the exploitation and exploration by using the predicted and at x. This measures the expectation of improvement over the best RRR observed so far. This routine is iterated until further improvement is no longer expected. In practice, we terminate the iteration when the number of trials reaches the predetermined budget. Here, we stopped the routine at 11 samplings per parameter. After completing 11 samplings for a certain parameter, we chose the value that gave the highest RRR and started the optimization of another parameter. In this optimization procedure, we used RRR (T = 4 K) instead of RRR (T0 K) for easy estimation. Further details about the implementation of machine-learning-assisted MBE are described elsewhere28.
In our previous study28, we carried out the optimization of the Ru flux rate while keeping the other parameters unaltered. Subsequently, we tuned the growth temperature and the nozzle-to-substrate distance. As a result, we obtained a high-RRR (51.8) SrRuO3 film in only 24 MBE growth runs (Supplementary Fig 2c). Since the RRR was still lower than the highest value reported (80) in the literature12,44, we further carried out the re-optimization of the Ru flux rate and growth temperature with previously optimized parameters (the Ru flux = 0.42 Å s−1, growth temperature = 721 °C, and nozzle-to-substrate distance = 15 mm) as a starting point to find the global-best point in the three-dimensional parameter space. Supplementary Figure 2c shows the highest experimental RRR values plotted as a function of the total number of MBE growth runs. With the re-optimization of the Ru flux rate and growth temperature, the highest RRR (T = 4 K) value increased and reached 81 in only 44 MBE growth runs. The highest experimental RRR (T0 K), 84.3, was achieved at the Ru flux = 0.365 Å s−1, growth temperature = 781 °C, and nozzle-to-substrate distance = 15 mm. The availability of such high-quality film allowed us to probe the intrinsic transport properties of SrRuO3.
Excluding other possible origins of the positive MR in SrRuO3
Since SrRuO3 has complex Fermi surfaces with both topologically trivial and nontrivial bands, the contribution from the trivial bands to the MR, such as orbital MR (∝B2)64, anisotropic MR (AMR) (∝ relative angle between the electric current and the magnetization), and weak anti-localization (WAL) (∝B−1/2)65, will appear in the MR in our SrRuO3 films. In fact, the AMR feature is clearly observed in the near-zero field region for the SrRuO3 film with the RRR of 84.3 as shown in Supplementary Fig. 3a. While it is difficult to distinguish each contribution on the MR in a low-field region (<0.5 T), the MR in a high field region (>0.5 T) is clearly dominated by the unsaturated linear positive MR. The MR curve at 2 K for the SrRuO3 film with the RRR of 84.3 with B applied in the out-of-plane [001] direction of the SrTiO3 substrate (Supplementary Fig. 3b) is linear above 0.5 T. This means that the magnetic field dependences of the WAL (∝B−1/2) and of the orbital MR (∝B2) provide only a very limited contribution to the net MR response and that the WAL and the orbital MR are negligibly small above 0.5 T. In addition, the contribution of the AMR above 0.5 T is also negligible since the magnetization of the SrRuO3 films saturates at about 0.5 T (see Supplementary Fig. 3c).
Next, the linear positive MR caused by carrier fluctuations39,48,66–70, originating from disorders and/or non-uniformity of dopants, can be excluded in the SrRuO3 film with the RRR of 84.3, since its Hall resistivity is not a linear function of B as shown in Supplementary Fig. 3d. The carrier fluctuations can cause the linear positive MR by an admixture of a component of the Hall resistivity to the longitudinal resistivity since the mobility fluctuation makes the Hall voltage contribute to the longitudinal voltage. In ref. 39, the admixture of a component of the Hall resistivity to the longitudinal resistivity ρxx due to the carrier fluctuations is expressed as
| 2 |
where ρxy is the Hall resistivity, and B is the external magnetic field. Therefore, ρxx is proportional to B when dρxy/dB is constant. For example, the GaAs 2DEG system shows clear linear MR reflecting its high-linearity of ρxy in the whole measurement range (0–33 T)39. In our case, however, the Hall resistivity shows nonlinear behavior reflecting its semi-metallic feature (Supplementary Fig. 3d). In fact, the curve calculated by the ρxy curve (Supplementary Fig. 3e) does not agree with the measured MR (ρxx(B)−ρxx(0 T))/ρxx(0 T) in the SrRuO3 film, especially in the low-magnetic-field range (0 T < B < 4 T), where the nonlinearity of the Hall resistivity (Supplementary Fig. 3d) is prominent. This means that carrier fluctuations are not the origin of the linear positive MR in our SrRuO3 films.
The admixture of a component of the Hall resistivity to the longitudinal resistivity is also ruled out by taking the small Hall resistivity of SrRuO3 into consideration. For the admixture of the Hall voltage, to cause the large positive MR over 100%, a large Hall voltage due to a small carrier density is necessary; only semiconducting materials can show this type of large MR. For example, the GaAs 2DEG system with the small sheet carrier density of 3 × 1011 cm−2 shows by far larger linear MR (~105%)39 than that of Bi2Se3 (~1%) with the large sheet carrier density of 1.7 × 1016 cm−2.68 In contrast, since the SrRuO3 film with the RRR of 84.3 is metallic, the Hall resistivity (=0.36 μΩ cm) at 2 K and 14 T is more than ten times smaller than the longitudinal resistivity (=3.79 μΩ cm) at 2 K and 14 T. This means that the longitudinal voltage in SrRuO3 is less affected by the Hall voltage.
Therefore, we concluded that the observed unsaturated linear positive MR above 0.5 T originates from the linear energy dispersion around the Weyl nodes and that the contribution from the conventional bands on the MR is negligible in the SrRuO3 film with the RRR of 84.3.
Temperature dependence of the AHE in the SrRuO3 film with the RRR = 84.3
It is known that the AHE in SrRuO3 is caused by an extrinsic factor (side-jump scattering) in addition to an intrinsic factor (KL mechanism)12,37,38. To determine the contributions of the intrinsic and extrinsic factors to the AHE, we investigated the temperature-dependent scaling of the AHE in the SrRuO3 film with the RRR = 84.3.
The Hall resistivity ρxy(B) in SrRuO3 is described as the summation of the ordinary and anomalous components of Hall effects12,37,38
| 3 |
is proportional to the perpendicular magnetization component M⊥: = cρsM⊥(c; constant). Here, the proportional coefficient ρs differs depending on the origin of the AHE, which can be of intrinsic (KL mechanism) and extrinsic (side jump scattering) origin12,37,38. As shown in Supplementary Fig. 4a, above TF (~20 K), clear AHE, which is proportional to the magnetization hysteresis curve (e.g., Fig. 1d, right inset), is discernible, and it dominates Hall resistivity in a near-zero magnetic field. On the other hand, below TF, the AHE components are negligibly small.
The temperature dependence of the AHE in SrRuO3 has been well reproduced by a model where both the intrinsic KL mechanism and the extrinsic side-jump scattering terms are taken into account38. In this model, the relationship between ρs and ρxx is described as
| 4 |
where a1–a3, and Δ are the fitting parameters, which are associated with the band structure38. The first term describes a contribution from the off-diagonal matrix elements of the velocity operators, called the KL term in the model38, and the second term is the contribution from the side-jump scattering. In this model, the KL term is obtained by incorporating the finite scattering rate, which is inversely proportional to , into Kubo’s formula71, and the constant a1 in Eq. (4) is expressed as
| 5 |
where b is a constant and is expressed as
| 6 |
Here, can be associated with a Berry phase72. The vy and vx are the velocity operators in the direction of y and x axes, respectively, is a set of quasi-momentum of the states producing the dominant contribution to , and . Here, “1” and “2” are indices to denote two individual bands: the former crosses the Fermi level, while the latter is fully occupied. When the system has multiple bands crossing the EF, the value is expressed by the sum of the values for each band. Therefore, the relationship between and (Eq. (4)) does not even change in the system with multiple bands. Accordingly, this model can be applied to analyze the temperature dependence of the AHE of SrRuO3, although detailed information about the electronic bands near EF in SrRuO3 is required to assign the contribution of each band to the intrinsic AHE.
Supplementary Figure 4b shows the ρs vs. ρxx plot of the SrRuO3 film with the RRR = 84.3 and the fitting result of Eq. (4). The fitting curve reproduces the AHE sufficiently, indicating that the AHE in the SrRuO3 film arises from the intrinsic KL mechanisms along with the extrinsic side-jump scattering. The important point in Eq. (4) is that the AHE is asymptotic to zero when ρxx0. Accordingly, for the SrRuO3 film with such a very high RRR (84.3), equivalently with a very small residual ρxx, AHE becomes negligibly small at low temperatures. Therefore, ρxy(B) curves below TF in our data are dominated by the ordinary Hall effect.
Excluding other possible origins of the negative MR in SrRuO3
Here, we exclude other possible mechanisms as the origin of the anisotropic linear negative MR at 2 K for the SrRuO3 film with the RRR of 84.3. As described in the Methods section “Excluding other possible origins of the positive MR in SrRuO3”, the contribution from the trivial bands to the MR, such as orbital MR64, AMR, and WAL65, are negligible above 0.5 T.
The electron-magnon scattering73 is also excluded as an origin of the linear negative MR (Fig. 2). The measurement temperature (2 K) of the anisotropic linear negative MR is too low to excite magnons in SrRuO3. A recent inelastic neutron scattering study on single-crystalline SrRuO374 reveals that the magnon gap of SrRuO3 is equal to 11.5 K. Therefore, the contribution from magnon scattering to the negative MR is negligible.
Finally, the boundary scattering effect is also excluded. The values of mean free paths lm for the Weyl fermions (F1 (=26 T) and F2 (=44 T) orbits), estimated from the SdH oscillation in Fig. 3b, are 89 and 132 nm, respectively. Here, the values are estimated by (where vF is Fermi velocity, kB is Boltzmann’s constant, and TD is the Dingle temperature). The mean free paths of other orbits (F3–F6) are smaller than these two orbits (F1 and F2). Since the mean free paths of the Weyl fermions are larger than the thickness of the SrRuO3 films ( = 63 nm), the boundary scattering may contribute to the negative MR. However, we can exclude its contribution as described as follows. The boundary-scattering-induced MR should saturate in a high-magnetic-field region75, since boundary scattering does not occur when cyclotron diameter dc (=ℏkF/(πeB); kF, Fermi wave number) is smaller than the film thickness ( = 63 nm). If the MR had been induced by boundary scattering, it would have saturated when B is larger than 0.93 and 1.2 T for the F1 and F2 orbits, respectively, at which dc becomes comparable to . On the contrary, the observed negative MR (Fig. 2a, c) does not show saturation behavior with B. Note that the coherent lengths of the other orbits (F3–F6) are not long enough to travel a full cyclotron trajectory at the measurement temperature (=2 K), where only the F1 and F2 SdH oscillation peaks are detected; as shown in Fig. 3d, the SdH oscillation peaks of F3–F6 are observed at 0.1 K. Therefore, the contribution of the boundary scattering to the negative MR (Fig. 2a, c) is negligibly small.
Effect of the Fermi-level pinning on the phase shift in the SdH oscillation
A large number of carriers in 3D multiband systems pin the chemical potential. In 2018, Kuntsevich et al. phenomenologically explained that, even when a large number of carriers in normal bands pin the chemical potential, the SdH oscillation of the Dirac (linear) dispersion should show the π phase shift76. Here, we will explain why the chemical potential pinning does not affect the phase shift in the SdH oscillations, along with ref. 76.
We will begin with the general explanation of SdH oscillations without the Fermi-level (chemical potential) pinning. In the SdH oscillation, the minimal conductivity is obtained when the chemical potential μc is located in the mid-gap between the Landau levels (Supplementary Fig. 5a). For this μc, the Nth Landau level beneath the chemical potential is fully occupied and the density of states shows the minimal value. Reflecting this situation, in the LK theory77, the magnetic field at which the magnitude of the SdH oscillation takes a minimal value is expressed as
| 7 |
Here, Fi is the frequency of the SdH oscillation, is the magnetic field giving the minimal value of the conductivity at the Nth Landau level, and 2πβBi is the phase shift. The important point of Eq. (7) is that the can be expressed as a linear function of N. This ensures the validity of using Eq. (7) for the determination of the phase shift caused by the Berry phase as we did in the main text.
Next, we consider the situation where a large number of carriers in normal bands pin the chemical potential, i.e. The Nth Landau levels of the quadratic energy band and the linear energy band are expressed as76,78
| 8 |
and
| 9 |
respectively, where m is an effective mass, v is the velocity of electrons in the linear band, and N is the Landau level index. The conductivity becomes maximum at with which the Landau level crosses μc, since the density at μc becomes maximum when μc is located at the center of the Landau level (Supplementary Fig. 5b). Therefore, by solving the equation , we can obtain the relationships between N and the magnetic field at which the conductivity becomes maximum as
| 10 |
and
| 11 |
for the quadratic and linear bands, respectively. Since is located roughly at the middle point between and , we can obtain the relationship between and N by shifting N in Eqs. (10) and (11) by a half-integer as
| 12 |
and
| 13 |
respectively. Then, by using the relationship between the and the Fermi wave number kF for the quadratic and linear band, and , respectively, and the relationship between the Fermi surface area S () and Fi, , Eqs. (12) and (13) can be simply expressed as
| 14 |
and
| 15 |
Equations (14) and (15) mean that the trivial (quadratic) and nontrivial (linear) dispersion provides 0 and π (=2π × 1/2) phase shifts, respectively, even when a large number of carriers pin the chemical potential. Therefore, whether the chemical potential is pinned or not in our SrRuO3 film, we can estimate the phase shift by using the LK theory [Eq. (1)]. Physically, the difference in the phase shifts in Eqs. (14) and (15) comes from the difference in the Berry phases of quadratic and linear dispersions76.
In a 3D system with B applied in the kz direction, a two-dimensional cyclotron motion occurs in the kx–ky plane in 3D k-space at the kz position where the area of the Fermi surface takes an extremal value79. This cyclotron orbit is called an “extremal orbit”. As in the case of 2DEG Rashba systems, graphene, and topological surface states, observation of the π Berry phase, which originates from a band touching point of the Weyl node and accumulates along the extremal orbit, is one of the important signatures of Weyl fermions20,23,24. Therefore, we think that the observed π Berry phase in the SdH oscillations is acquired by a surface integral of the Berry curvature Ω over a closed surface containing a Weyl point in k-space (Fig. 3a).
Data pretreatment for quantum oscillations
Pretreatments of the SdH oscillation data are crucial for deconvoluting quantum oscillation spectra, since magnetoconductivity data generally contain not only oscillation components but also other magnetoresistive components as background signals43. In particular, SdH oscillations in SrRuO3 are subject to being masked by large non-saturated positive MR (Supplementary Fig. 5c)43. Here, we subtracted the background using a polynomial function up to the fifth order and extracted the oscillation components as shown in Supplementary Fig. 5d. Then, we carried out the well-established pretreatment procedure for Fourier transform of quantum oscillations80,81. First, we interpolated the background-subtracted data to prepare an equally spaced data set as a function of 1/B. Then, we multiplied the Hanning window function to obtain the periodicity of the experimental data. Finally, we performed fast Fourier transform on the treated data set.
Exclusion of other possible mechanisms of the phase shift in SrRuO3
Here, we exclude other possible non-topological effects that could cause the phase shift in SdH oscillations, which are the mosaic effect, magnetic breakdown, and Zeeman splitting.
The phase shift and deviation of the SdH oscillations from the conventional LK theory, which occur at crystal grains and magnetic domains, are collectively called the mosaic effect79,82. This effect may occur in samples having multiple crystal domains, such as polycrystals, or in ferromagnets having magnetic domain structures. However, it should be negligible in our samples, because they are high-quality single-crystalline thin films as shown by a STEM image (Fig. 1c, Supplementary Fig. 1a, and Supplementary Fig. 5e) and they are free from magnetic domain structures as shown in Supplementary Fig. 3c where magnetization is saturated at about 0.5 T.
Next, the possibility of magnetic breakdown is also ruled out since there is no magnetic breakdown orbits in the SdH oscillations for the SrRuO3 film with the RRR of 84.3 (Fig. 3). Magnetic breakdown occurs when different orbits approach each other closely in k-space under the presence of large magnetic fields, resulting in a new orbit (a magnetic breakdown orbit) whose frequency is given by the sum of the frequencies of the original orbits83. If magnetic breakdown had occurred in our measurement field range (|B| < 14 T), we would have observed magnetic breakdown orbits whose frequencies are F1 + F2 (=70 T), F1 + F3 (=326 T), and so on. However, we did not find such frequencies in our quantum oscillation analysis as shown in Supplementary Table 1. In particular, F1 and F2, which are responsible for the non-trivial phase shifts, cannot be produced by the sum of the other orbitals’ frequencies. Therefore, magnetic breakdown is not the origin of the phase shift.
Finally, we focus on the effect of Zeeman splitting on the phase shift in a magnetic Weyl semimetal. The condition where the quantum oscillation takes minimal or maximum values is expressed as78
| 16 |
Here, F is a frequency, H is a magnetic field, n is an integer value, γ indicates the phase shift caused by the Berry phase, and S is the spin-splitting parameter from Zeeman effect. The ± sign indicates the up/down spins in each Landau level. Due to the Zeeman effect, every Landau level is split-off by the magnetic field, and finally it affects the phase shift γ through the change of the S value. In fact, this Zeeman splitting of Landau levels changes SdH spectra in Weyl semimetals and magnetic Dirac materials20,84, in which Landau levels are degenerate, and this effect has to be taken into account to deduce the phase shift γ from the experimental data. By contrast, in SrRuO3, all Landau levels are not degenerate since the ferromagnetic exchange coupling lifts the spin degeneracy of all the electronic bands crossing the EF12,42,43,85. Altogether, since the S value of Eq. (16) is zero in SrRuO3, we can simply estimate the phase shift γ by the LK theory77, in which S is not taken into account, and assign the phase shift in the SdH oscillations to the Berry phase accumulation along the cyclotron orbits.
SdH oscillations of trivial orbits
Together with SdH oscillations from the nontrivial orbits having low frequencies (F1 and F2) (Fig. 3b, c), we observed SdH oscillations from the trivial orbits having high frequencies (F3–F6) at 0.07 K < T < 0.75 K and 12.5 T < B < 14 T (Fig. 3d and Supplementary Fig. 6b). Since the carriers in the trivial orbits in SrRuO3 are expected to have larger effective masses than those in the F1 and F2 orbits12,43,58, measurements of the former oscillations should be carried out in relatively low-T and high-B regions. We estimated the cyclotron masses of the carriers in F3–F6 orbits from the temperature dependences of the respective peaks based on the LK theory (Supplementary Fig. 6c–f). In the LK theory for the mass estimation, B is determined as the interval value in the magnetic field range. Supplementary Table 1 shows the estimated cyclotron masses for F1–F6. The cyclotron masses in the F3–F6 orbits are relatively high (>2.8m0), reflecting the trivial band structure (energy dispersions) as their origin.
RRR dependence of the ferromagnetism, Fermi liquid behavior, and Weyl behavior
In Fig. 4a–c, we investigated the RRR dependence of the ferromagnetism, Fermi liquid behavior, and Weyl behavior in SrRuO3. For the ferromagnetism, TC values are estimated as the position of the kinks in ρxx vs. T curves as shown in Supplementary Fig. 7a. For the Fermi liquid behavior, we defined the Fermi liquid region (T < TF) as the temperature range where the experimental ρxx and the linear fitting line in ρxx vs. T2 are close enough to each other (<0.1 μΩ cm) as shown in Supplementary Fig. 7b. The upper limit temperature for measuring Weyl behavior in SrRuO3 (TW) is defined as the highest temperature at which the resistivity at zero field is lower than that at 9 T (ρxx(0 T) < ρxx(9 T)).
RRR dependence of the Hall resistivity
Supplementary Figure 7c shows the Hall resistivity ρxy(B) curves of the different RRR samples at 2 or 2.3 K with B applied in the out-of-plane [001] direction of the SrTiO3 substrate. As we explained in the main paper, the ρxy(B) curves of the SrRuO3 film with the RRR of 84.3 at 2 K is nonlinear, indicating the coexistence of multiple types of the Weyl fermions from which the unsaturated linear positive MR stems. Notably, as shown in Supplementary Fig. 7c, dρxy/dB changes its sign from negative to positive with decreasing RRR. In the SrRuO3 film with the RRR of 8.93, clear AHE is observed near zero magnetic field due to its large residual resistivity of 20.2 μΩ cm, and the ρxy(B) curve shows the linear dependence on B above 5 T as highlighted in Supplementary Fig. 7c. The carrier concentration and the mobility of the holes of the SrRuO3 film with the RRR of 8.93, which are estimated from the slope of the ρxy(B) above 5 T, are 4.04 × 1022 cm−3 and 7.65 cm2 V−1 s−1, respectively. The carrier concentration and the mobility are consistent with the reported values for the trivial Ru 4d bands crossing the EF in SrRuO344,86. These results mean that the Weyl fermions become more dominant in the transport properties with increasing RRR and that the contribution of the Weyl fermions on the Hall resistivity is negligibly small when the RRR is 8.93.
As described in the main text, the unsaturated linear positive MR also becomes more prominent with increasing RRR and decreasing temperature below TF, indicating again that the nonlinear ρxy(B) is a hallmark of the existence of the Weyl fermions in SrRuO3 and that the Weyl fermions become dominant in the transport when scatterings from impurities and phonons are sufficiently suppressed.
Computational details
Electronic structure calculations were performed within the density functional theory and generalized gradient approximation (GGA, Perdew–Burke–Ernzerhof)87 for the exchange correlation functional in the projector-augmented plane wave (PAW) formalism88 as implemented in the Vienna ab-initio Simulation package89. The energy cutoff was set to 500 eV, the Brillouin zone was sampled by an 8 × 8 × 6 Monkhorst–Pack mesh90, and the convergence criterion for the electronic density was defined as 10−8 eV. The effect of electronic correlations in the Ru d shell (4d4 for Ru4+) was taken into account by using the rotationally invariant GGA + U scheme91 with U = 2.6 eV and J = 0.6 eV. The choice of parameters is justified by early estimations92 and is in agreement with other studies93,94.
First-principles calculations of Weyl points
The orthorhombic phase of SrRuO3 has the Pbnm (#62) space group, which corresponds to the D2h point group with symmetries of inversion (I), three mirror planes (mx, my, mz), and 180° rotations around the orthorhombic axes (Cx, Cy, Cz). The crystal structure parameters considered in the present study are a = 5.5670 Å, b = 5.5304 Å, c = 7.8446 Å,12 and the atomic Wyckoff positions in fractional coordinates are given as 4c (0.5027, 0.5157, 0.25) for Sr, 4b (0.25, 0, 0) for Ru, and 8d (0.7248, 0.2764, 0.0278) for O.
The results of electronic structure calculations with and without SOC are shown in Supplementary Fig. 8. One can see that the bands close to the Fermi level are formed by the Ru 4d states hybridized with the O 2p states and the electronic spectrum reveals half-metallicity (Supplementary Fig. 8a) in agreement with previous electronic structure calculations59. The ferromagnetic alignment was found to be the ground state configuration with the easy axis along the orthorhombic b axis (−2.17 meV/f.u. and −0.35 meV/f.u.), and the calculated magnetic moments per Ru ion (Supplementary Table 2) are close to the experimental saturation magnetic moment of 1.25 /Ru.28 The ferromagnetic state reduces the symmetry to the C2h point group with one mirror plane and one rotation axis symmetry, perpendicular and parallel to the magnetization direction, respectively.
For numerical identification of the Weyl points, one needs to have a band structure with high resolution in the reciprocal space. To interpolate the resulting electronic spectrum, we employed maximally localized Wannier functions as implemented in the wannier90 package95. The wannierization was carried out by projecting the bands corresponding to the Ru eg and t2g states onto the atomic d orbitals in the local coordinate frame at each Ru site.
To locate the points of degeneracy between the bands in the reciprocal space, we performed a steepest-descent minimization of the gap function on a uniform grid of up to 31 × 31 × 31 covering the Brillouin zone, where the bands are considered degenerate when the gap is below the threshold of 10−5 eV96. To eliminate accidental crossings, the corresponding chirality at each identified point is determined by evaluating the outward Berry flux enclosed in a small sphere. The calculated chiralities should obey the following symmetry properties: does not change its sign under 180° rotations around the magnetization axis and changes its sign under mirror reflection and inversion. In this study, we only consider the points with .
We have selected two pairs of bands I and II for the cases when the magnetization is along the orthorhombic c and b axes, as shown in Supplementary Fig. 9 and Supplementary Fig. 10, respectively. The corresponding gap function eV is calculated to demonstrate the proximity of the selected bands. From the number of the calculated band crossings, the Weyl points were identified as the ones that have close energy positions and k-point coordinates and whose chiralities obey the symmetry properties in the full Brillouin zone. The resulting Weyl points are listed in Supplementary Tables 3–6. Numerical differences in the coordinates of the Weyl points can be attributed to small spin canting (see Supplementary Table 2), which slightly breaks inversion symmetry (the reflection and rotation symmetries along to the magnetization are intact). From Supplementary Tables 3–6, most of the Weyl points are found to exist within an energy range of −0.2 to 0.2 eV around the Fermi level. In particular, for WPz61–4 (8–16 meV), which are located near the Y–T line in the Z–Γ–Y–T plane as shown in Fig. 5c, is very close to the experimental chemical potentials of the Weyl fermions estimated from the SdH oscillations (µ1 = 8.5 meV and µ2 = 8.8 meV for the F1 and F2 orbitals, respectively).
From the obtained results, one can clearly see the presence of the Weyl fermions below and above the Fermi level coexisting with trivial half-metallic bands. However, it is worth commenting on another scenario. According to previous theoretical studies93,94, there is no clear consensus on whether the electronic spectrum of orthorhombic SrRuO3 in the ferromagnetic state is half-metallic or not, and the result turns out to depend on the details of electronic structure calculations. While our theoretical results are in good agreement with the present experiments, we do not rule out the possibility of a non-half-metallic behavior with both spin channels crossing close to the Fermi level. Assuming that the spin-up states can also lie at the Fermi level, there will be an extra set of band crossings in addition to the Weyl points already defined in a half-metallic scenario.
Finally, it is worth noting that a small monoclinic distortion induced by the SrTiO3 substrate breaks the orthorhombic D2h symmetry. The reported crystal structure parameters of epitaxial SrRuO3 on SrTiO3 are a = 5.5290 Å, b = 5.5770 Å, c = 7.8100 Å, = 89.41°.12 According to our electronic structure calculations within GGA + U with U = 2.6 eV and J = 0.6 eV for the monoclinic SrRuO3 on SrTiO3 (Supplementary Fig. 11), the electronic spectrum does not reveal any qualitative changes from that for the orthorhombic D2h symmetry, while additional band crossings may occur due to the lowered crystal symmetry.
Supplementary information
Acknowledgements
We thank Yuichi Onuma for valuable discussions. A part of this work was conducted at Advanced Characterization Nanotechnology Platform of the University of Tokyo, supported by “Nanotechnology Platform” of the Ministry of Education, Culture, Sports, Science and Technology (MEXT), Japan. K.T. acknowledges the support from the Japan Society for the Promotion of Science (JSPS) Fellowships for Young Scientists and the Material Education program for the future leaders in Research, Industry, and Technology (MERIT).
Author contributions
Y.K.W. conceived the idea, designed the experiments, and led the project. Y.K.W. and Y.K. designed and built the MBE system. Y.K.W., T.O., and H.S. implemented the Bayesian optimization algorithm for the sample growth. Y.K.W. grew the samples. Y.K.W. and K.T. carried out the sample characterizations. K.T., H.I., and Y.K.W. fabricated the Hall bar structures and carried out transport measurements. K.T. and Y.K.W. analyzed and interpreted the data. S.A.N. and H.D. carried out the electronic-structure calculations. K.T., Y.K.W., H.I., Y.K., T.O., H.S., S.A.N., H.D., M.T., Y.T., and H.Y. contributed to the discussion of the data. K.T. and Y.K.W. co-wrote the paper with input from all authors.
Data availability
The data that support the findings of this study are available from the corresponding author upon reasonable request.
Competing interests
The authors declare no competing interests.
Footnotes
Peer review information Nature Communications thanks the anonymous reviewer(s) for their contribution to the peer review of this work.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
These authors contributed equally: Kosuke Takiguchi, Yuki K. Wakabayashi.
Supplementary information
Supplementary information is available for this paper at 10.1038/s41467-020-18646-8.
References
- 1.Kuroda K, et al. Evidence for magnetic Weyl fermions in a correlated metal. Nat. Mater. 2017;16:1090. doi: 10.1038/nmat4987. [DOI] [PubMed] [Google Scholar]
- 2.Morali N, et al. Fermi-arc diversity on surface terminations of the magnetic Weyl semimetal Co3Sn2S2. Science. 2019;365:1286. doi: 10.1126/science.aav2334. [DOI] [PubMed] [Google Scholar]
- 3.Liu DF, et al. Magnetic Weyl semimetal phase in a Kagomé crystal. Science. 2019;365:1282. doi: 10.1126/science.aav2873. [DOI] [PubMed] [Google Scholar]
- 4.Belopolski I, et al. Discovery of topological Weyl fermion lines and drumhead surface states in a room temperature magnet. Science. 2019;365:1278. doi: 10.1126/science.aav2327. [DOI] [PubMed] [Google Scholar]
- 5.Soh JR, et al. Ideal Weyl semimetal induced by magnetic exchange. Phys. Rev. B. 2019;100:201102(R). doi: 10.1103/PhysRevB.100.201102. [DOI] [Google Scholar]
- 6.Wan X, Turner AM, Vishwanath A, Savrasov SY. Topological semimetal and Fermi-arc surface states in the electronic structure of pyrochlore iridates. Phys. Rev. B. 2011;83:205101. doi: 10.1103/PhysRevB.83.205101. [DOI] [Google Scholar]
- 7.Chen Y, Bergman DL, Burkov AA. Weyl fermions and the anomalous Hall effect in metallic ferromagnets. Phys. Rev. B. 2013;88:125110. doi: 10.1103/PhysRevB.88.125110. [DOI] [Google Scholar]
- 8.Watanabe H, Po HC, Vishwanath A. Structure and topology of band structures in the 1651 magnetic space groups. Sci. Adv. 2018;4:eaat8685. doi: 10.1126/sciadv.aat8685. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 9.Shimano H, et al. Terahertz Faraday rotation induced by an anomalous Hall effect in the itinerant ferromagnet SrRuO3. Eur. Phys. Lett. 2011;95:17002. doi: 10.1209/0295-5075/95/17002. [DOI] [Google Scholar]
- 10.Itoh S, et al. Weyl fermions and spin dynamics of metallic ferromagnet SrRuO3. Nat. Commun. 2016;7:11788. doi: 10.1038/ncomms11788. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 11.Ohtsuki T, et al. Strain-induced spontaneous Hall effect in an epitaxial thin film of a Luttinger semimetal. Proc. Natl Acad. Sci. USA. 2019;116:8803. doi: 10.1073/pnas.1819489116. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 12.Koster G, et al. Structure, physical properties, and applications of SrRuO3 thin films. Rev. Mod. Phys. 2012;84:253. doi: 10.1103/RevModPhys.84.253. [DOI] [Google Scholar]
- 13.Worledge DC, Geballe TH. Negative spin-polarization of SrRuO3. Phys. Rev. Lett. 2000;85:5182. doi: 10.1103/PhysRevLett.85.5182. [DOI] [PubMed] [Google Scholar]
- 14.Boschker H, et al. Ferromagnetism and conductivity in atomically thin SrRuO3. Phys. Rev. X. 2019;9:011027. [Google Scholar]
- 15.Matsuno J, et al. Interface-driven topological Hall effect in SrRuO3-SrIrO3 bilayer. Sci. Adv. 2016;2:e1600304. doi: 10.1126/sciadv.1600304. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 16.Huang X, et al. Observation of the chiral-anomaly-induced negative magnetoresistance: in 3D Weyl semimetal TaAs. Phys. Rev. X. 2015;5:031023. [Google Scholar]
- 17.Singha R, Pariari AK, Satpati B, Mandal P. Large nonsaturating magnetoresistance and signature of nondegenerate Dirac nodes in ZrSiS. Proc. Natl Acad. Sci. USA. 2017;114:2468. doi: 10.1073/pnas.1618004114. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 18.He LP, et al. Quantum transport evidence for the three-dimensional dirac semimetal phase in Cd3As2. Phys. Rev. Lett. 2014;113:246402. doi: 10.1103/PhysRevLett.113.246402. [DOI] [PubMed] [Google Scholar]
- 19.Xiang ZJ, et al. Angular-dependent phase factor of Shubnikov-de Haas oscillations in the dirac semimetal Cd3As2. Phys. Rev. Lett. 2015;115:226401. doi: 10.1103/PhysRevLett.115.226401. [DOI] [PubMed] [Google Scholar]
- 20.Hu J, et al. π Berry phase and Zeeman splitting of Weyl semimetal TaP. Sci. Rep. 2016;6:18674. doi: 10.1038/srep18674. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 21.Xiong J, et al. Evidence for the chiral anomaly in the Dirac semimetal Na3Bi. Science. 2015;350:413. doi: 10.1126/science.aac6089. [DOI] [PubMed] [Google Scholar]
- 22.Zhang CL, et al. Magnetic-tunnelling-induced Weyl node annihilation in TaP. Nat. Phys. 2017;13:979. doi: 10.1038/nphys4183. [DOI] [Google Scholar]
- 23.Arnold F, et al. Chiral Weyl pockets and Fermi surface topology of the Weyl semimetal TaAs. Phys. Rev. Lett. 2016;117:146401. doi: 10.1103/PhysRevLett.117.146401. [DOI] [PubMed] [Google Scholar]
- 24.Sergelius P, et al. Berry phase and band structure analysis of the Weyl semimetal NbP. Sci. Rep. 2016;6:33859. doi: 10.1038/srep33859. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 25.Li P, et al. Evidence for topological type-II Weyl semimetal WTe2. Nat. Commun. 2017;8:2150. doi: 10.1038/s41467-017-02237-1. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 26.Orbanić F, et al. Three-dimensional Dirac semimetal and magnetic quantum oscillations in Cd3As2. J. Phys. Conf. Ser. 2017;903:012038. doi: 10.1088/1742-6596/903/1/012038. [DOI] [Google Scholar]
- 27.Takahashi KS, et al. Anomalous Hall effect derived from multiple Weyl nodes in high-mobility EuTiO3 films. Sci. Adv. 2018;4:eaar7880. doi: 10.1126/sciadv.aar7880. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 28.Wakabayashi YK, et al. Machine-learning-assisted thin-film growth: Bayesian optimization in molecular beam epitaxy of SrRuO3 thin films. APL Mater. 2019;7:101114. doi: 10.1063/1.5123019. [DOI] [Google Scholar]
- 29.Fujioka J, et al. Strong-correlation induced high-mobility electrons in Dirac semimetal of perovskite oxide. Nat. Commun. 2019;10:362. doi: 10.1038/s41467-018-08149-y. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 30.Xu SY, et al. Discovery of a Weyl fermion semimetal and topological Fermi arcs. Science. 2015;349:613. doi: 10.1126/science.aaa9297. [DOI] [PubMed] [Google Scholar]
- 31.Kurebayashi D, Nomura K. Voltage-driven magnetization switching and spin pumping in Weyl semimetals. Phys. Rev. Appl. 2016;6:044013. doi: 10.1103/PhysRevApplied.6.044013. [DOI] [Google Scholar]
- 32.Araki Y, Nomura K. Charge pumping induced by magnetic texture dynamics in Weyl semimetals. Phys. Rev. Appl. 2018;10:014007. doi: 10.1103/PhysRevApplied.10.014007. [DOI] [Google Scholar]
- 33.Shiota Y, et al. Induction of coherent magnetization switching in a few atomic layers of FeCo using voltage pulses. Nat. Mater. 2012;11:39. doi: 10.1038/nmat3172. [DOI] [PubMed] [Google Scholar]
- 34.Liu L, et al. Current-induced magnetization switching in all-oxide heterostructures. Nat. Nanotechnol. 2019;14:939. doi: 10.1038/s41565-019-0534-7. [DOI] [PubMed] [Google Scholar]
- 35.Ma, Y. et al. Realization of epitaxial thin films of the topological crystalline insulator Sr3SnO. Adv. Mat.32, 2000809 (2020). [DOI] [PubMed]
- 36.Fang Z, et al. The anomalous Hall Effect and magnetic monopoles in momentum space. Science. 2003;302:92. doi: 10.1126/science.1089408. [DOI] [PubMed] [Google Scholar]
- 37.Karplus R, Luttinger J. Hall effect in ferromagnetics. Phys. Rev. 1954;95:1154. doi: 10.1103/PhysRev.95.1154. [DOI] [Google Scholar]
- 38.Haham N, et al. Scaling of the anomalous Hall effect in SrRuO3. Phys. Rev. B. 2011;84:174439. doi: 10.1103/PhysRevB.84.174439. [DOI] [Google Scholar]
- 39.Khouri K, et al. Linear magnetoresistance in a quasifree two-dimensional electron gas in an ultrahigh mobility GaAs quantum well. Phys. Rev. Lett. 2016;117:256601. doi: 10.1103/PhysRevLett.117.256601. [DOI] [PubMed] [Google Scholar]
- 40.Sammon M, Zudov MA, Shklovskii BI. Mobility and quantum mobility of modern GaAs/AlGaAs heterostructures. Phys. Rev. Mater. 2018;2:064604. doi: 10.1103/PhysRevMaterials.2.064604. [DOI] [Google Scholar]
- 41.Madelung, O. Semiconductors: Data Handbook (Springer, Berlin, Germany, 2013).
- 42.Shai DE, et al. Quasiparticle mass enhancement and temperature dependence of the electronic structure of ferromagnetic SrRuO3 thin films. Phys. Rev. Lett. 2013;110:087004. doi: 10.1103/PhysRevLett.110.087004. [DOI] [PubMed] [Google Scholar]
- 43.Mackenzie A, Reiner J. Observation of quantum oscillations in the electrical resistivity. Phys. Rev. B. 1998;58:R13318(R). doi: 10.1103/PhysRevB.58.R13318. [DOI] [Google Scholar]
- 44.Izumi M, et al. Magnetotransport of SrRuO3 thin film on SrTiO3 (001) J. Phys. Soc. Jpn. 1997;66:3893. doi: 10.1143/JPSJ.66.3893. [DOI] [Google Scholar]
- 45.Coey LMD, Venkatesan M. Half-metallic ferromagnetism: example of CrO2. J. Appl. Phys. 2012;91:8345. doi: 10.1063/1.1447879. [DOI] [Google Scholar]
- 46.Wang KY, Edmonds KW, Campion RP, Zhao LX, Foxon CT, Gallagher BL. Anisotropic magnetoresistance and magnetic anisotropy in high-quality (Ga,Mn)As films. Phys. Rev. B. 2005;72:085201. doi: 10.1103/PhysRevB.72.085201. [DOI] [Google Scholar]
- 47.Ali MN, et al. Large, non-saturating magnetoresistance in WTe2. Nature. 2014;514:205. doi: 10.1038/nature13763. [DOI] [PubMed] [Google Scholar]
- 48.Abrikosov A. Quantum magnetoresistance. Phys. Rev. B. 1998;58:2788. doi: 10.1103/PhysRevB.58.2788. [DOI] [Google Scholar]
- 49.Shekhar C, et al. Extremely large magnetoresistance and ultrahigh mobility in the topological Weyl semimetal candidate NbP. Nat. Phys. 2015;11:645. doi: 10.1038/nphys3372. [DOI] [Google Scholar]
- 50.Son DT, Spivak BZ. Chiral anomaly and classical negative magnetoresistance of Weyl metals. Phys. Rev. B. 2013;88:104412. doi: 10.1103/PhysRevB.88.104412. [DOI] [Google Scholar]
- 51.Zhang C-L, et al. Signatures of the Adler-Bell-Jackiw chiral anomaly in a Weyl fermion semimetal. Nat. Commun. 2016;7:10735. doi: 10.1038/ncomms10735. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 52.Hirschberger M, et al. The chiral anomaly and thermopower of Weyl fermions in the half-Heusler GdPtBi. Nat. Mater. 2016;15:1161. doi: 10.1038/nmat4684. [DOI] [PubMed] [Google Scholar]
- 53.Xiao D, Chang MC, Niu Q. Berry phase effects on electronic properties. Rev. Mod. Phys. 2010;82:1959. doi: 10.1103/RevModPhys.82.1959. [DOI] [Google Scholar]
- 54.Murakawa H, et al. Detection of Berry’s phase in a bulk Rashba semiconductor. Science. 2013;342:1490. doi: 10.1126/science.1242247. [DOI] [PubMed] [Google Scholar]
- 55.Wright AR, McKenzie RH. Quantum oscillations and Berry’s phase in topological insulator surface states with broken particle-hole symmetry. Phys. Rev. B. 2013;87:085411. doi: 10.1103/PhysRevB.87.085411. [DOI] [Google Scholar]
- 56.LuK’Yanchuk IA, Kopelevich Y. Phase analysis of quantum oscillations in graphite. Phys. Rev. Lett. 2004;93:166402. doi: 10.1103/PhysRevLett.93.166402. [DOI] [PubMed] [Google Scholar]
- 57.Eto K, Ren Z, Taskin AA, Segawa K, Ando Y. Angular-dependent oscillations of the magnetoresistance in Bi2Se3 due to the three-dimensional bulk Fermi surface. Phys. Rev. B. 2005;81:195309. doi: 10.1103/PhysRevB.81.195309. [DOI] [Google Scholar]
- 58.Alexander CS, McCall S, Schlottmann P, Crow JE, Cao G. Angle-resolved de Haas-van Alphen study of SrRuO3. Phys. Rev. B. 2005;72:024415. doi: 10.1103/PhysRevB.72.024415. [DOI] [Google Scholar]
- 59.Ryee S, Jang SW, Kino H, Kotani T, Han MJ. Quasiparticle self-consistent GW calculation of Sr2RuO4 and SrRuO3. Phys. Rev. B. 2016;93:075125. doi: 10.1103/PhysRevB.93.075125. [DOI] [Google Scholar]
- 60.Wakabayashi YK, et al. Ferromagnetism above 1000 K in a highly cation-ordered double-perovskite insulator Sr3OsO6. Nat. Commun. 2019;7:101114. doi: 10.1038/s41467-019-08440-6. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 61.Snoek, J., Larochelle, H. & Adams, paper presented at Advances in Neural Information Processing Systems 25, 2012, see also http://papers.nips.cc/paper/4522-practical-bayesian-optimization.
- 62.Wakabayashi YK, et al. Improved adaptive sampling method utilizing Gaussian process regression for prediction of spectral peak structures. Appl. Phys. Express. 2018;11:112401. doi: 10.7567/APEX.11.112401. [DOI] [Google Scholar]
- 63.Mockus J, Tiesis V, Zilinskas A. The application of Bayesian methods for seeking the extremum. Towards Glob. Optim. 1978;2:117. [Google Scholar]
- 64.Collaudin A, et al. Angle dependence of the orbital magnetoresistance in bismuth. Phys. Rev. X. 2015;5:021022. [Google Scholar]
- 65.Kim H, et al. Dirac versus Weyl fermions in topological insulators: Adler-Bell-Jackiw anomaly in transport phenomena. Phys. Rev. Lett. 2013;111:246603. doi: 10.1103/PhysRevLett.111.246603. [DOI] [PubMed] [Google Scholar]
- 66.Rötger T, et al. Relation between low-temperature quantum and high-temperature classical magnetotransport in a two-dimensional electron gas. Phys. Rev. Lett. 1989;62:90. doi: 10.1103/PhysRevLett.62.90. [DOI] [PubMed] [Google Scholar]
- 67.Parish MM, Littlewood PB. Non-saturating magnetoresistance in heavily disordered semiconductors. Nature. 2003;426:162. doi: 10.1038/nature02073. [DOI] [PubMed] [Google Scholar]
- 68.Wang WJ, et al. Disorder-dominated linear magnetoresistance in topological insulator Bi2Se3 thin films. Appl. Phys. Lett. 2017;111:232105. doi: 10.1063/1.5000880. [DOI] [Google Scholar]
- 69.Abrikosov A. Quantum linear magnetoresistance. Eur. Phys. Lett. 2000;49:789. doi: 10.1209/epl/i2000-00220-2. [DOI] [Google Scholar]
- 70.Hu J, Rosenbaum T. Classical and quantum routes to linear magnetoresistance. Nat. Mater. 2008;7:697. doi: 10.1038/nmat2259. [DOI] [PubMed] [Google Scholar]
- 71.Murayama, Y. Mesoscopic Systems: Fundamentals and Applications (Wiley-VCH,Weinheim, Germany, 2001), pp. 213.
- 72.Nagaosa N, et al. Anomalous Hall effect. Rev. Mod. Phys. 2010;82:1539. doi: 10.1103/RevModPhys.82.1539. [DOI] [Google Scholar]
- 73.Raquet B, et al. Electron-magnon scattering and magnetic resistivity in 3d ferromagnets. Phys. Rev. B. 2002;66:024433. doi: 10.1103/PhysRevB.66.024433. [DOI] [Google Scholar]
- 74.Jenni K, et al. Interplay of electronic and spin degrees in ferromagnetic SrRuO3: anomalous softening of the magnon gap and stiffness. Phys. Rev. Lett. 2019;123:017202. doi: 10.1103/PhysRevLett.123.017202. [DOI] [PubMed] [Google Scholar]
- 75.Nikolaeva A, et al. Diameter-dependent thermopower of bismuth nanowires. Phys. Rev. B. 2008;77:035422. doi: 10.1103/PhysRevB.77.035422. [DOI] [Google Scholar]
- 76.Kuntsevich A, Yu, Shupletsov AV, Minkov GM. Simple mechanisms that impede the Berry phase identification from magneto-oscillations. Phys. Rev. B. 2018;97:195431. doi: 10.1103/PhysRevB.97.195431. [DOI] [Google Scholar]
- 77.Lifshitz IM, Kosevich AM. Theory of magnetic susceptibility in metals at low temperatures. Sov. Phys. JETP. 1956;2:636. [Google Scholar]
- 78.Shoenberg, D. Magnetic Oscillations in Metals (Cambridge University Press, Cambridge, UK, 1984).
- 79.Shoenberg D. Magnetic interaction and phase smearing. J. Low. Temp. Phys. 1976;25:755. doi: 10.1007/BF00657297. [DOI] [Google Scholar]
- 80.Beukman A, et al. Spin-orbit interaction in a dual gated InAs/GaSb quantum well. Phys. Rev. B. 2017;96:241401(R). doi: 10.1103/PhysRevB.96.241401. [DOI] [Google Scholar]
- 81.Nichele, F. Transport Experiments in Two-Dimensional Systems With Strong Spin-Orbit Interaction. PhD thesis, (Eidgenössische Technische Hochschule Zürich, 2014).
- 82.Itskovsky MA, Kventsel GF, Maniv T. Periodic diamagnetic domain structures in metals under a quantizing magnetic field. Phys. Rev. B. 1994;50:6779. doi: 10.1103/PhysRevB.50.6779. [DOI] [PubMed] [Google Scholar]
- 83.Pippard AB. Quantization of coupled orbits in metals. Proc. R. Soc. Lond. Ser. A. 1963;270:1340. [Google Scholar]
- 84.Masuda H, et al. Impact of antiferromagnetic order on Landau-level splitting of quasi-two-dimensional Dirac fermions in EuMnBi2. Phys. Rev. B. 2018;98:161108(R). doi: 10.1103/PhysRevB.98.161108. [DOI] [Google Scholar]
- 85.Ryee S, et al. Quasiparticle self-consistent GW calculation of Sr2RuO4 and SrRuO3. Phys. Rev. B. 2016;93:075125. doi: 10.1103/PhysRevB.93.075125. [DOI] [Google Scholar]
- 86.Katano T, Tsuda N. The Seebeck coefficient of Ca1−xSrxRuO3. J. Phys. Soc. Jpn. 1996;65:207. doi: 10.1143/JPSJ.65.207. [DOI] [Google Scholar]
- 87.Perdew JP, Burke K, Ernzerhof M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996;77:3865. doi: 10.1103/PhysRevLett.77.3865. [DOI] [PubMed] [Google Scholar]
- 88.Kresse G, Joubert D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B. 1999;59:1758. doi: 10.1103/PhysRevB.59.1758. [DOI] [Google Scholar]
- 89.Kresse G, Furthmüller J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B. 1996;54:11169. doi: 10.1103/PhysRevB.54.11169. [DOI] [PubMed] [Google Scholar]
- 90.Monkhorst HJ, Pack, James D. Special points for Brillouin-zone integrations. Phys. Rev. B. 1976;13:5188. doi: 10.1103/PhysRevB.13.5188. [DOI] [Google Scholar]
- 91.Liechtenstein AI, Anisimov VI, Zaanen J. Density-functional theory and strong interactions: Orbital ordering in Mott-Hubbard insulators. Phys. Rev. B. 1995;52:R5467. doi: 10.1103/PhysRevB.52.R5467. [DOI] [PubMed] [Google Scholar]
- 92.Solovyev IV, Dederichs PH, Anisimov VI. Corrected atomic limit in the local-density approximation and the electronic structure of d impurities in Rb. Phys. Rev. B. 1994;50:16861. doi: 10.1103/PhysRevB.50.16861. [DOI] [PubMed] [Google Scholar]
- 93.Rondinelli JM, Caffrey NM, Sanvito S, Spaldin NA. Electronic properties of bulk and thin film SrRuO3: search for the metal-insulator transition. Phys. Rev. B. 2008;78:155107. doi: 10.1103/PhysRevB.78.155107. [DOI] [Google Scholar]
- 94.Grånäs O, et al. Electronic structure, cohesive properties, and magnetism of SrRuO3. Phys. Rev. B. 2014;90:165130. doi: 10.1103/PhysRevB.90.165130. [DOI] [Google Scholar]
- 95.Mostofi AA, et al. An updated version of wannier90: a tool for obtaining maximally-localised Wannier functions. Comput. Phys. Commun. 2014;185:2309. doi: 10.1016/j.cpc.2014.05.003. [DOI] [Google Scholar]
- 96.Gosálbez-M D, Souza I, Vanderbilt D. Chiral degeneracies and Fermi-surface Chern numbers in bcc Fe. Phys. Rev. B. 2015;92:085138. doi: 10.1103/PhysRevB.92.085138. [DOI] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Data Availability Statement
The data that support the findings of this study are available from the corresponding author upon reasonable request.