Abstract
The Sun sporadically produces eruptive events leading to intense fluxes of solar energetic particles (SEPs) that dramatically disrupt the near-Earth radiation environment. Such events have been directly studied for the last decades but little is known about the occurrence and magnitude of rare, extreme SEP events. Presently, a few events that produced measurable signals in cosmogenic radionuclides such as 14C, 10Be and 36Cl have been found. Analyzing annual 14C concentrations in tree-rings from Switzerland, Germany, Ireland, Russia, and the USA we discovered two spikes in atmospheric 14C occurring in 7176 and 5259 BCE. The ~2% increases of atmospheric 14C recorded for both events exceed all previously known 14C peaks but after correction for the geomagnetic field, they are comparable to the largest event of this type discovered so far at 775 CE. These strong events serve as accurate time markers for the synchronization with floating tree-ring and ice core records and provide critical information on the previous occurrence of extreme solar events which may threaten modern infrastructure.
Subject terms: Solar physics, Solar physics
Two extreme solar energetic particle events have been found by carbon isotopes measured in ancient tree rings in 7176 and 5259 BCE. The recorded ~2% increases of atmospheric 14 C for both events exceeds in amplitude of all previously observed events.
Introduction
The Sun sporadically produces eruptive events, such as flares and coronal mass ejection, that can lead to highly intense fluxes of solar energetic particles (SEPs), which escape into the interplanetary space and possibly hit Earth1. When reaching the Earth, SEPs can have a dramatic impact on modern communication, navigation and power systems, satellite life expectancy, the health of astronauts, and aircraft operations2,3. The famous Carrington event was one of the strongest known solar storms that hit the Earth in September 1859 CE, leading to a widespread failure of the telegraph system and observable auroras all over the world3,4. If this, or an even larger event, occurred today the impact on global society and economy would be catastrophic3,5.
The first instrumental observations of SEPs were conducted in the 1940s6. Since then their physical origin, frequency of occurrence, amplitude, and energy distribution have been studied using ground-based and space-borne data7. The strongest measured SEP event (called ground-level-enhancement GLE #5) took place on 23-Feb-1956 (a list of directly measured events is available at the International GLE database (https://gle.oulu.fi)). However, little is known about extreme SEP events whose very existence was unknown until a few years ago8. Even though observational advances such as a worldwide network of neutron monitors established in 1951/1957/1964 made it possible to characterize the Suns’ eruptive behavior9, the temporal coverage of the observational record is not long enough to assess the frequency of extremely rare but highly energetic SEP events. Statistics of sun-like stars suggest that the superflares that produce highly energetic SEPs are extremely rare10,11.
Understanding and ultimately predicting extreme solar events will not only help in mitigating their harmful consequences on modern life and communication systems, but will also help understanding the complex magneto-hydrodynamic behavior of the Sun. In this context, cosmogenic radionuclides provide a powerful tool for reconstructing past solar activity and SEP events12–15.
Cosmogenic radionuclides such as 10Be, 14C and 36Cl are mainly produced by galactic cosmic rays (GCRs) originating from outside our solar system, hitting the Earth’s atmosphere. The flux of GCRs on Earth is modulated (shielded) by the geomagnetic field and the solar magnetic field carried by the solar wind16. As a consequence, enhanced magnetic fields (solar/heliospheric or geomagnetic) reduce cosmogenic nuclide production by GCRs on Earth and vice versa. When hitting the Earth, strong SEPs may cause an additional, short-term increase in cosmogenic nuclide production. Natural archives, such as dendrochronologically dated tree-rings (14C) or polar ice cores (10Be,36Cl), are known to provide precise and temporally accurate records of past cosmogenic radionuclide production12,17,18 giving us a unique opportunity to identify and study the characteristics of strong SEP events over the past thousands of years.
So far, three strong SEP events that led to an abrupt increase of about 1% or more in atmospheric 14C concentrations within less than two years have been unambiguously detected over the past 3,000 years in tree-rings, and confirmed with other cosmogenic radionuclides (10Be, 36Cl) in ice cores, in the years 993 CE, 775 CE and 660 BCE13,14,19,20. More, weaker, yet unconfirmed SEP events have been found in the radionuclide records17,21,22 (Table 1). They are currently considered candidate events either because there is insufficient data coverage and precision to clearly distinguish them from normal solar modulation or because they have not yet been confirmed23. The above mentioned Carrington Event did not lead to a detectable increase in cosmogenic radionuclides17,24,25 implying that associated SEPs either missed the Earth or did not have sufficient energy.
Table 1.
The modeled atmospheric 14C increases, produced 14C and normalized 14C produced at the modern earth magnetic field is given for new and known events.
| Simulated Δ14C increase (‰) | Additional 14C produced (kg) | Geomagnetic field strength (1022Am2) Knudsen | Geomagnetic field strength (1022Am2) Panovska | Normalized additional 14C produced (kg) (M = 7.8 ∙ 1022Am2) Knudsen | Normalized additional 14C produced (kg) (M = 7.8 ∙ 1022Am2) Panovska | |
|---|---|---|---|---|---|---|
| 7176 BCE (This Study) | 19.5 ± 0.6 | 28.7 ± 0.9 | 8.7 ± 1.7 | 7.5 ± 0.4 | 30.1 ± 3.6 | 28.0 ± 1.2 |
| 5410 BCE22 | 5.6 ± 0.8 | 9.0 ± 1.1 | 7.2 ± 0.6 | 7.3 ± 0.4 | 8.6 ± 1.1 | 8.6 ± 1.1 |
| 5259 BCE (This Study) | 19.1 ± 0.6 | 29.2 ± 0.9 | 7.1 ± 0.5 | 7.4 ± 0.4 | 27.6 ± 1.4 | 28.4 ± 1.2 |
| 660 BCE13 | 12.5 ± 1.1 | 19.2 ± 2.1 | 11.4 ± 0.6 | 9.0 ± 0.4 | 23.9 ± 2.7 | 20.9 ± 2.4 |
| 775 CE53 | 17.6 ± 0.5 | 26.2 ± 1.0 | 10.7 ± 0.4 | 9.3 ± 0.5 | 31.5 ± 1.4 | 29.0 ± 1.4 |
| 993 CE53 | 9.6 ± 0.6 | 14.0 ± 1.2 | 10.3 ± 0.4 | 9.0 ± 0.5 | 16.5 ± 1.5 | 15.2 ± 1.4 |
| 1052 CE17 | 5.9 ± 1.1 | 10.1 ± 2.0 | 10.2 ± 0.4 | 9.0 ± 0.4 | 11.8 ± 2.3 | 11.0 ± 2.2 |
| 1279 CE17 | 6.5 ± 1.6 | 9.2 ± 2.7 | 9.6 ± 0.3 | 9.2 ± 0.5 | 10.4 ± 3.0 | 10.1 ± 2.9 |
The longest absolutely dated composite tree-ring record spans the last 12,460 years26. At present, high-precision, annual 14C measurements from tree-rings, which are required for the sensitive detection of strong SEP events, only cover about 2030 years27. Therefore, our ability to study strong SEP events is limited and theoretical estimations on their frequency9 and energy spectra20,28 remain highly uncertain. The extension of the time period covered with annually resolved 14C measurements in tree-rings will provide statistically robust estimates on the magnitude and frequency of strong SEP events.
Currently, the search for strong SEP events mainly relies on existing cosmogenic nuclide records with lower (5–20 years) temporal resolution. The motivation to investigate the annually resolved 14C signal in tree-rings between 7150 BCE and 7200 BCE came from lower resolution, synchronized29,30 10Be and 36Cl data in ice cores31–33 with a resolution of 5 to 20 years and from decadal 14C data (IntCal20)27. Another event in 5259 BCE, was discovered while investigating unexpected difficulties in producing stable Bayesian chronological models for archaeological sites and ceramic sequences dating to the period centering on the 53rd century BCE34–36. With hindsight, this event could not have been detected by the statistical analysis of cosmogenic nuclides from the lower resolution ice core data37.
Here we present two previously unknown 14C production events recorded in two (7176 BCE) and four (5259 BCE) independent, absolutely dated tree-ring chronologies. The magnitudes of the events are assessed by calculating the additional 14C production using a global carbon cycle box model. They are compared to other known 14C events by normalizing their magnitudes to modern geomagnetic shielding. Furthermore, the 7176 BCE event is used as a unique time marker to place a floating (i.e. not absolutely dated) portion of the Bristlecone Pine tree-ring chronology (USA) within 1–2 years on its absolutely dated part.
Results
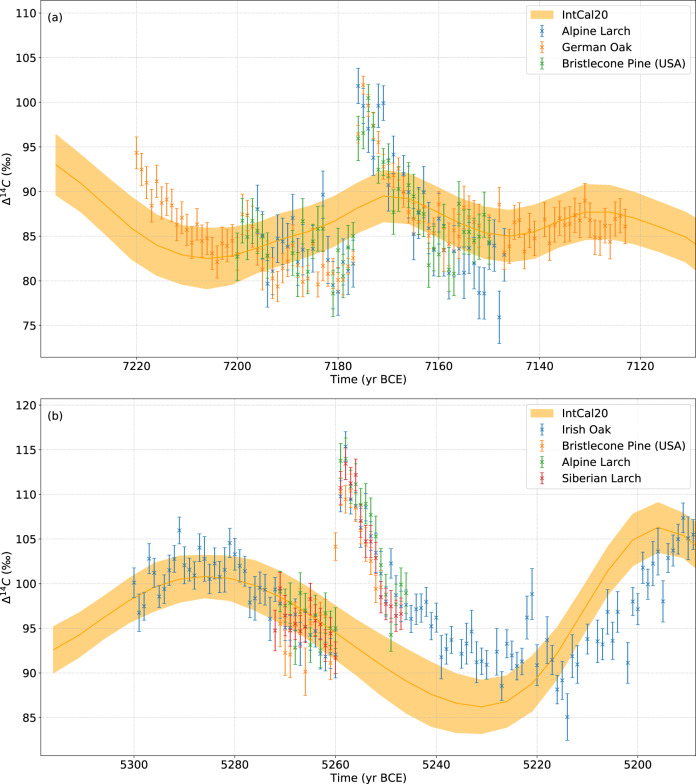
Atmospheric 14C concentrations measured in several independently built tree-ring chronologies increased by nearly 2% within two years in 7176 BCE and 5259 BCE (Fig. 1, Table 1, Supplementary Fig. 1) thereby exceeding the rise in 14C concentration of the largest known event in 775 CE14. For the 7176 BCE event (Fig. 1a) an average increase of (19.5 ± 0.6)‰ in 14C concentrations was observed in the two existing absolutely dated tree-ring chronologies from central Europe covering that time range, the German oak and the Eastern Alpine conifer38,39 chronologies. The 7176 BCE event was also found in a currently floating portion of the Bristlecone Pine chronology from the USA (Supplementary information S1). The 5259 BCE event (Fig. 1b) was found in four absolutely dated tree-ring chronologies from the Alps, Ireland, Russia and the USA showing an average increase in atmospheric 14C concentrations of (19.1 ± 0.6) ‰. A potentially early increase is observed in the Bristlecone Pine in 5260 BCE.
Fig. 1. 14C data of the two events compared to IntCal20.
Annual 14C concentrations reported as Δ14C with 1- σ errors from different trees for the two found events (7176 BCE (a), 5259 BCE (b)) in comparison with the IntCal20 calibration curve27 (orange band).
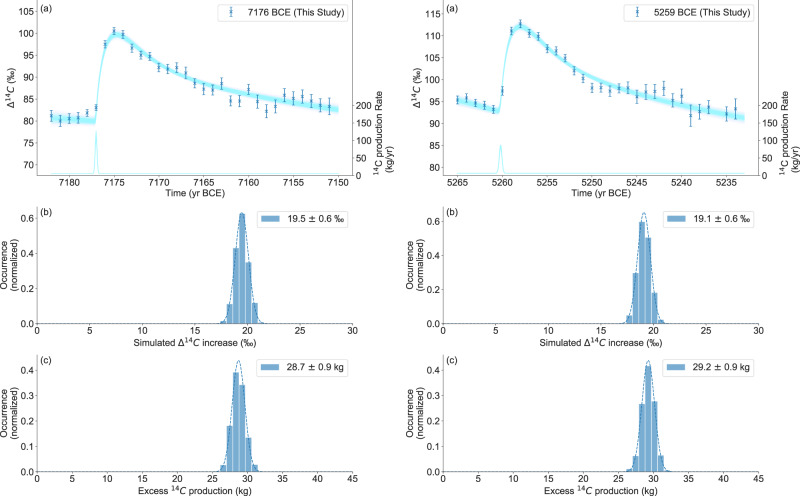
Total and excess 14C productions from the SEP event were calculated using a global carbon cycle model17 (Methods). The excess production (and related uncertainty) was calculated using a Monte–Carlo approach by fitting offset and amplitude of a Gaussian-shaped production spike to the data (Fig. 2, Methods). For each of the 1000 different Monte–Carlo data realizations the amount of additional 14C produced was extracted. The average amount of 14C excess produced during the two events was (29.2 ± 0.9) kg for the 5259 BCE event and (28.7 ± 0.9) kg for the 7176 BCE event (Table 1). This is more than (7176 BCE) or comparable to (5259 BCE) the amount of 14C produced by the strongest 14C event detected so far (775 CE, (26.2 ± 1.0) kg excess 14C). In both cases, a modeled duration of 0.3 year, 2-sigma of the Gaussian, was used to fit the measured 14C increase. This is significantly less than the tree-ring resolution of one year.
Fig. 2. Evaluation procedure of the found events.
a Mean data of the two events (7176 BCE left, 5259 BCE right) with 1-σ errors and result of 1000 Simulations (blue lines) The fitted Gaussian shaped production spikes for all simulations is also shown. b Distribution of the simulated ∆14C increases (blue bars) with a gaussian fit (dashed line). c Distribution of excess 14C production (blue bars) with Gaussian fit (dashed line).
Excess 14C production of all known events (Supplementary Fig. 3) was (re-)calculated using the above described procedure (Fig. 2a). When comparing different events, it is, however, important to note that the same event (in terms of the proton flux and energy distribution) can lead to different excess productions of cosmogenic radionuclides depending on the strength of the Earth’s geomagnetic field at the time of the event. A weak geomagnetic field would result in higher excess production of cosmogenic radionuclides by the SEP event and vice versa. We therefore recalculated the excess of 14C production by normalizing all events relative to the modern geomagnetic dipole moment of 7.8 ∙ 1022 Am2 using two different geomagnetic field records40,41 (see Methods/ Supplementary Figs. 4 and 5 (Fig. 3). The resulting normalized excess 14C productions for the 7176 BCE event are not significantly different from the original value (Table 1). The 5259 BCE event occurred during the period of weaker magnetic field strength and, thus, referring to modern geomagnetic shielding the excess 14C production would be slightly lower. The normalization procedure also significantly changes the excess 14C production of the other known events. For the 775 CE event, the two geomagnetic field reconstructions give different results, while the Knudsen41 reconstruction leads to a 20% increase of excess 14C, using the Panovska40 reconstruction increases it by only 10%. When comparing the events after normalization we observe that the 7176 BCE and the 5259 BCE events are of similar, but slightly weaker magnitude than the 775 CE event. In any case, they are significantly stronger than both 660 BCE and 993 CE events.
Fig. 3. Initial and normalized event productions.
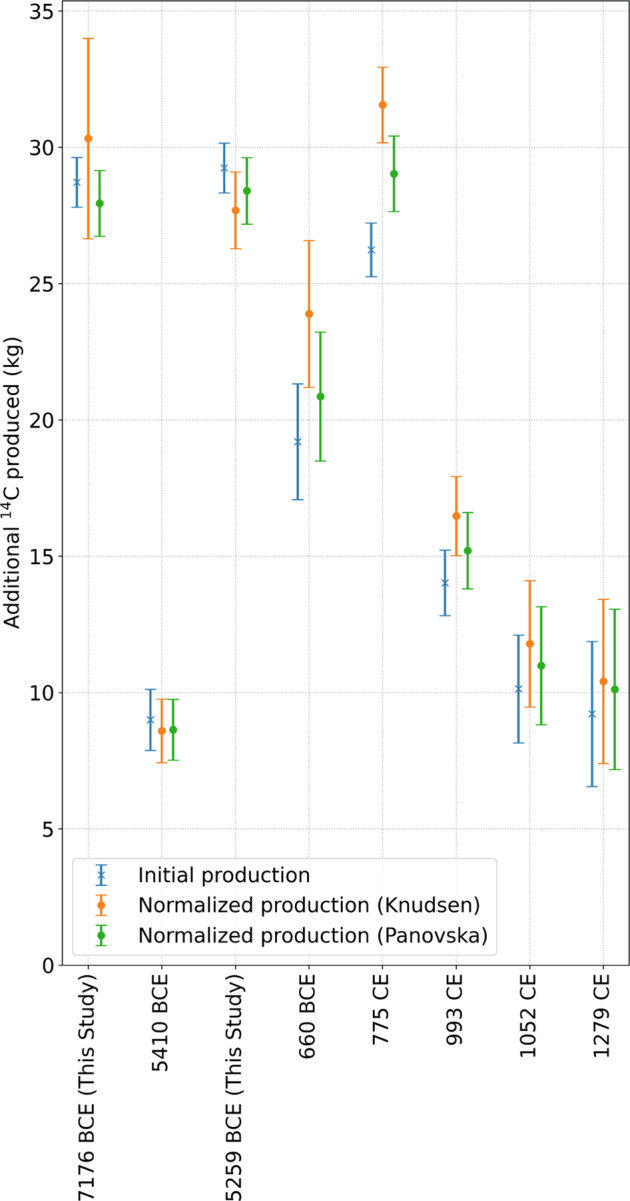
Excess 14C produced during the events (blue) in comparison to the excess 14C production normalized to today’s geomagnetic field strength using two different records (orange Knudsen41, green Panovska40) with 1-σ error bars for all known 14C events.
Discussion
The two identified events are assigned to the years 5259 BCE and 7176 BCE in several independent and absolutely dated tree-ring chronologies. The fact that the events are accurately dated to one year makes them (and all other 14C events) excellent time markers for the synchronization of chronologies. For example, an older floating portion of the USA Bristlecone chronology could not be comprehensively connected to its master chronology using tree-ring dating techniques, due to a very short (not statistically verifiable) period of overlap between the records. Instead, this chronology was assigned possible calendar dates based upon a tentative dendrochronological match within a broader time window suggested by conventional radiocarbon dating42 (see SI for full details). The results of this analysis clearly showed the 7176 BCE event, placing this previously floating sequence on an absolute timescale. Further substantiation of this connection will provide an extension of the Methuselah Walk bristlecone pine chronology beyond 6827 BCE (where the calendar dated portion ends) across a full 10,399 year sequence.
For the 5259 BCE event, we note that the bristlecone record shows a potential early increase in 5260 BCE. There are a number of possible causes for this. Very narrow ring widths (<0.5 mm) during this period, indicating difficult environmental conditions, may have hampered the complete dissection of the rings, or there may be a previously undetected dating error in this earlier calendar dated portion of the record, where cross-checking with other site chronologies is not possible. Alternatively, this anomalous result may relate to regional shifts in growing season or physiological differences between deciduous and indeciduate trees. If, for example, the 14C event occurred in late summer or autumn of 5260 BCE, i.e. after the end of the ring formation period in Ireland, the Alps and the Russian north, but before the end of tree ring formation in California, this would result in increased 14C content of the 5260 BCE bristlecone pine tree ring, but not in the other chronologies. However, this cannot be argued on the basis of published information on the end of today’s growing seasons (Bristlecone pine—late August43,44, Alpine larch—October45, Irish oak – October46, Siberian larch-late August). Furthermore, the fact that the event occurred during the Holocene climatic optimum, which was characterized by a weaker latitudinal temperature gradient47, may mean that more synchronous growing seasons would be more likely. Another possibility may relate to the fact that Bristlecone Pine is the only indeciduate tree in this record. Deciduous trees such as oak and larch store photosynthates produced during the end of growing season to grow the next year’s earlywood48. If the 14C event occurred towards the very end of the deciduous tree’s growth season it is possible that only the indeciduate Bristlecone Pine would register the change in the same year. If this early increase of 14C in Bristlecone Pine can be confirmed by future replicate measurements, the timing of the event would be confined precisely to the end of summer/autumn 5260 BCE.”
Polar ice cores, which are regarded as extremely valuable climate archives, have typically been dated with a precision of about 10 years within the Holocene29 and even less in the transition to the glacial period42. The absolutely dated 14C events in tree-rings can now be used to synchronize the ice core chronologies via cosmogenic 10Be and 36Cl resulting in a precision of about 1–2 years limited by several factors such as weather noise, sampling, and radionuclide transfer times to the ice core43. As a result, chronologies that so far were synchronized with absolutely dated tree-ring records using the low resolution structure of existing 14C or 10Be records29 can now be placed within 1–2 years or about 10 times more precisely around these events using the abrupt change in radionuclide concentrations caused by SEP events.
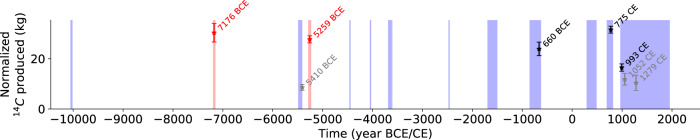
The increasing number of discoveries of strong SEP events that hit Earth over the past 12,000 years indicates that they cannot be considered as extremely rare anymore. So far, only a few sequences covering a total of 2030 yrs of the past 12,400 years (16.5%) have been analyzed with 14C at the annual or biennial resolution, which is required to unambiguously detect SEP events, revealing five strong SEP events (Fig. 4). These findings might lead to the conclusion that strong SEP events hit Earth once every about 400 years on average (i.e. five events in 2030 years). This simple statistic, however, is likely biased by the fact that some studied time periods were not randomly selected for annual analysis, but rather were targeted based on indications from lower-resolution cosmogenic datasets or archaeological evidence that the present multi-annual 14C record might contain more structures than previously visible. In an unlikely case that all major events have already been found over the last 12,400 years, the lower limit of occurrence for strong SEP events can be estimated as five events in 12,400 years or one every ~2400 years. Based on our findings we can constrain the occurrence rate of strong solar events to one every 400–2400 years. We nevertheless expect more events to be discovered as additional annually resolved data becomes available, leading to more precise estimates of the frequency of occurrence and magnitude of strong SEP events. The estimate of the occurrence rate of strong SEP events shows that they are more frequent than suggested by the revised statistics of superflares on sun-like stars (once every 3000--6000 yr)11. However, a direct relation between superflares and strong SEP events remains unknown. The statistical constraints provided by the data will help to test different approaches in solar/stellar physics describing the occurrence and magnitude of extreme events in sun-like stars. Ranking among the three largest short-term 14C production events, the impact of the newly discovered events would have been catastrophic for aircraft, satellites, modern telecommunication and computer systems44–46, if they occurred today.
Fig. 4. Magnitude and occurrence of all known 14C events over time.
Shaded regions mark time periods where IntCal2027 (purple) and our data (red) are based on annual or biennial resolution. Data points show the timing of all known (black) and newly identified (red) 14C events and their normalized additional production with 1-σ errors. Event candidates are indicated in grey.
Materials and Methods
Sample preparation and measurement
For the 7176 BCE event dendrochronologically dated wood samples from Ireland, supplied by the University of Belfast, and from the Alps, supplied by the University of Innsbruck, were dissected into annually resolved samples weighing 30–60 mg.
For the analyses performed at ETHZ, typically 54 tree-ring samples with four wood blanks (2 BC and 2 KB) and 2 1515 CE reference samples47 each weighing 30–60 mg, were prepared in 15 ml glass test tubes together in a batch (making 60 in total). In a slightly modified procedure following Němec et al.48, samples were first soaked in 5 ml 1 M NaOH overnight at 70 °C in an oven. Then the samples were treated with 1 M HCl and 1 M NaOH for 1 hour each at 70°C in a heat block, before they were bleached at a pH of 2–3 with 0.35 M NaClO4 at 70 °C for 2 h. The remaining white holo-cellulose was then freeze-dried overnight.
About 2.5 mg dried holo-cellulose was wrapped in cleaned Al capsules49 and converted to graphite using the automated graphitization line AGE50. A measurement set was made up of three oxalic acid one (OX1) and four oxalic acid two (OX2) standards, 27 samples, two cellulose blanks, two chemical blanks, and two 1515 CE reference samples (individual cellulose preparations of the 1515 CE reference is used for at least two measurements) and measured in the MICADAS accelerator mass spectrometer51. Two measurement sets were typically prepared from one set of samples within a week and subsequently measured. A second graphite sample was subsequently prepared and measured from one third of the prepared cellulose samples for quality control purposes.
Two internal wood reference materials from 1515 CE (Pine and Oak) and two different radiocarbon free wood blanks (Kauri Stage 7, KB, and Brown Coal, BC, from Reichwalde) were repetitively analyzed together with the annual samples. While the wood-blanks were used for blank subtraction in the data evaluation process, the 1515 CE references were used for quality control only.
For the analyses performed in Bristol, tree ring samples (20–30 mg) were processed alongside two KB wood blanks and two oak 1524 CE reference samples in 15 ml glass culture tubes. Samples were pretreated following the standard BRAMS wood pretreatment method (code BABAB; following Němec et al.), as described by Knowles et al.52. Around 2.5 mg dried holo-cellulose was combusted, graphitized, and measured using the Bris-MICADAS AMS. Full details of the procedures employed are described by Knowles et al.52.
Several samples were repetitively measured, and a chi-squared analysis showed that the repeated measurements were generally in good agreement with one another. The resulting chi-squared values of the different chronologies are shown in Table 2. Small observed differences are likely due to wood anatomical effects or due to the limitations of cutting tree-rings accurately for species with narrow tree-rings (Supplements S1). The high overall χ2 value events are likely due to regional offset53 and the earlier increase signal in the Bristlecone Pine chronology in the 5259 BCE event.
Table 2.
Statistical analysis of the repeated measurements of the different chronologies.
| χ2 | Deg. of freedom | P(χ2>) value | |
|---|---|---|---|
| All (7176 BCE) | 255.3 | 202 | 0.01 |
| Alpine Larch (7176 BCE) | 6.3 | 6 | 0.39 |
| German Oak (7176 BCE) | 112.6 | 93 | 0.08 |
| Bristlecone Pine (7176 BCE) | 12.4 | 13 | 0.50 |
| All (5259 BCE) | 254.0 | 216 | 0.04 |
| Irish Oak (5259 BCE) | 89.0 | 82 | 0.28 |
| Alpine Larch(5259 BCE) | 15.9 | 13 | 0.25 |
| Siberian Larch (5259 BCE) | 10.2 | 24 | 0.99 |
| Bristlecone Pine (5259 BCE) | 16.6 | 24 | 0.87 |
Modeling
To model the carbon cycle an improved carbon box model based on the model of Güttler et al.54 was used (Supplementary Fig 2). The model of Güttler uses 11 Boxes to simulate the exchange between the global atmosphere biosphere and Oceans. To model the northern and southern hemispheres separately our model was extended to 22 boxes (11 boxes for each hemisphere). The carbon content of each box is distributed according to the respective relative carbon reservoir masses of the corresponding hemisphere. Radiocarbon is produced in the stratosphere and the troposphere of both hemispheres, where 70% is produced in the Stratosphere and 30 % in the Troposphere. The fluxes were adjusted to ensure a correct ∆14C offset between the northern and southern troposphere. Seasonal variability of fluxes was not considered in the model.
The 12C and 14C content of each box after a time step is calculated with
| 1 |
| 2 |
| 3 |
| 4 |
Here is the content of each box in Gt and λ is the decay constant of 14C. The time step ∆t was chosen to be one month for all the following simulations. The 14C and 12C fluxes are given by the following:
| 5 |
Here are the fluxes given in Supplementary Fig. 2. The fluxes are scaled depending on the deviation from the Holocene steady state . The steady state was computed by simulating 200’000 years with the constant production rate . The model does not consider any isotopic fractionation and thus the 14C fluxes scale just as the 12C fluxes.
With this a general expression for all boxes at any time can be achieved.
| 6 |
| 7 |
| 8 |
The ∆14C of each box for the simulation is calculated by the following expression:
| 9 |
Where are the steady state 12,14C contents of the northern troposphere.
To get a reasonable start state at a given time the production rate for the whole IntCal13 record has been calculated and the simulated state can be loaded at any time before 1950 CE.
The events were evaluated by generating 1000 realizations of the data distributed with the errors of the measurements. For each realization a Gaussian shaped production spike was fitted and the additional production was extracted by integrating the Gaussian production. Since the energy spectrum of the SEPs is softer than the one of the galactic cosmic rays the excess production of the event was mainly produced in the stratosphere (90%) and only 10% in the troposphere. The errors were estimated by using the widths of the resulting distributions.
Geomagnetic field correction
Since the production of 14C in the Earth’s atmosphere is affected by geomagnetic shielding of the flux of SEPs, this effect needs to be accounted for in such a way that all the events are normalized to the same reference standard, specifically to the modern conditions with the dipole moment M = 7.8·1022 A·m2 55. The geomagnetic field has a very complex structure at the Earth’s surface56, but for energetic particles, the dipole moment is the most important since higher momenta decay faster with distance, and the eccentric+tilted dipole approximation is widely used57. Since the true dipole moment is difficult to determine in the past, before the era of direct measurements, an approximation of the virtual axial dipole moment (VADM) is reconstructed from paleo- and archeo-magnetic models58. Accordingly, the geomagnetic-shielding effect on energetic particles is often quantified via the VADM value of M. The modern (epoch 2000) dipole moment is M0 = 7.8·1022 A·m2. Here we normalize the apparent 14C production Q during different events to the standard modern conditions, viz. the production that would be if the event took place nowadays. For that, we modeled the dependence of Q on M using a broad range of SEP spectra measured during the recent decades59. The spectra are bound between the hardest known spectrum of SEP event on 23-Feb-1956 (GLE 0560) and the softest strong-event spectrum of 04-Aug-1972 (GLE 2460), as shown in Supplementary Fig. 4a. The same plot shows also the global yield function, denoted as Yp(E), of 14C for three VADM values of M = 3, 6 and 9 (x1022 A·m2). The global production function is defined as
| 10 |
where Y(E) is the columnar yield function of 14C by protons with energy E in the Earth’s atmosphere61, H(E) is a step-like magnetospheric transmissivity function taking the value of 0 for E < Ec and 1 otherwise, where Ec is the energy corresponding to the local geomagnetic rigidity cutoff defined by the dipole moment M and the polar angle (co-latitude) θ. The production is higher for a weaker geomagnetic field (smaller values of M) and vice versa. The production of 14C by a specific SEP event with a given proton spectrum Jp is defined as the integral of the product of the yield function and the energy spectrum of particles.
| 11 |
The dependence of Q on M is shown in Supplementary Fig. 4b for the two analyzed SEP spectra. In order to compensate for the variable geomagnetic field, we introduce the correction factor, which relates the 14C production at the VADM M to that at the reference field M0:
| 12 |
The correction factor is shown in Supplementary Fig. 4c for the two SEP spectra. Although the spectra are essentially different (panel a) and the isotope production for the two events is different too (panel b), the correction factor appears robust against the exact spectral shape. This correction factor was further applied to compare the strength of all studied events to the standard conditions, as shown in Table 1.
Reconstructions of the geomagnetic field in the past is a difficult task, and the result can be uncertain. To cover a possible range of VADM values we took a conservative approach and considered two paleomagnetic reconstructions for the last millennia, as shown in Supplementary Fig. 5: a recent GGF100k paleomagnetic model by Panovska et al.40 and a reconstruction by Knudsen et al.41 based on the GEOMAGIA50 database. Although there are many other paleomagnetic reconstructions, these two cover the full range of uncertainties.
The uncertainties of the corrected for the VADM changes 14C production (last two columns in Table 1) are mostly defined by the uncertainties of the actual production (column 3) and VADM uncertainties (columns 4 and 5). The resultant uncertainty of the corrected production was assessed using the Monte–Carlo method by randomly picking pairs of M and Q from the normally distributed values within the ranges shown in Table 1 and computing the mean and the standard deviation of the corrected Q values.
Supplementary information
Acknowledgements
The Laboratory of Ion Beam Physics is partially funded by its consortium partners PSI, EAWAG, and EMPA. N.B. is funded by the Swiss National Science Foundation (SNSF grant #SNF 197137). The establishment of the BRAMS Facility was jointly funded by the NERC, BBSRC and the University of Bristol and the measurements in this work were partly funded by an ERC Proof of Concept grant awarded to R.P.E. and financing E.C. postdoctoral contract (LipDat H2020 ERC-2018-PoC/812917). We thank Bisserka Gaydarska for sub-sampling the inter-laboratory replicates from M49, M234, Q2729 and Q2750, Cathy Tyers for reviewing the dating of the Irish and German samples, and Alexander Land for assistance in dating sample M49. P.F. received funding from the SNF Sinergia project CALDERA (no. 183571). R.H. is funded by Russian Science Foundation (grant # 21-14-00330). I.U. acknowledges the support from the Academy of Finland (grant 321882 ESPERA). C.L.P.’s and M.W.S.’s work on bristlecone pine was funded by the M.H. Wiener Foundation (ICCP Project). K.N. acknowledges the support provided by the Austrian Science Fund FWF (grant I-1183-N19).
Source data
Author contributions
L.W., M.C. and N.B. designed the study with input from H.-A.S., R.M. and F.A., A.B., D.B., H.-H.L., K.N, T.P., C.S., C.L.P., M.W.S., D.N., E.C., P.F., R.P.E. and R.H. supplied the annually resolved tree-ring samples and are responsible for the documentation of the dendrochronology. Radiocarbon measurements and analyses were performed by N.B., L.W. and T.K. The modeling and interpretation of the 14C data were done by N.B, M.C., and L.W. with input from I.U., F.A., R.M., H.-A.S., F.M. and C.L.P., N.B, M.C., and L.W. wrote the manuscript with input from all other authors.
Peer review
Peer review information
Nature Communications thanks Brian Thomas and the other, anonymous, reviewer(s) for their contribution to the peer review of this work. Peer reviewer reports are available.
Data availability
The excel data that support the findings of this study are available in PANGEA at doi. Source data are provided with this paper.
Code availability
Any computer code used for evaluation of the results of this study will be available on request.
Competing interests
The authors declare no competing interests.
Footnotes
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Contributor Information
Nicolas Brehm, Email: nbrehm@ethz.ch.
Marcus Christl, Email: mchristl@phys.ethz.ch.
Lukas Wacker, Email: wacker@phys.ethz.ch.
Supplementary information
The online version contains supplementary material available at 10.1038/s41467-022-28804-9.
References
- 1.Desai M, Giacalone J. Large gradual solar energetic particle events. Living Rev. Sol. Phys. 2016;13:3. doi: 10.1007/s41116-016-0002-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 2.Eastwood JP, et al. Quantifying the economic value of space weather forecasting for power grids: an exploratory study. Space Weather. 2018;16:2052–2067. [Google Scholar]
- 3.Council, N. R. Severe Space Weather Events–Understanding Societal and Economic Impacts: A Workshop Report: Extended Summary. (The National Academies Press, 2009).
- 4.Green JL, Boardsen S, Odenwald S, Humble J, Pazamickas KA. Eyewitness reports of the great auroral storm of 1859. Adv. Space Res. 2006;38:145–154. doi: 10.1016/j.asr.2005.08.054. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 5.Fry EK. The risks and impacts of space weather: Policy recommendations and initiatives. Space Policy. 2012;28:180–184. [Google Scholar]
- 6.Forbush SE. Three unusual cosmic-ray increases possibly due to charged particles from the sun. Phys. Rev. 1946;70:771–772. [Google Scholar]
- 7.Vlahos L, Anastasiadis A, Papaioannou A, Kouloumvakos A, Isliker H. Sources of solar energetic particles. Philos. Trans. R. Soc. A: Math., Phys. Eng. Sci. 2019;377:20180095. doi: 10.1098/rsta.2018.0095. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 8.in Extreme Solar Particle Storms: The hostile Sun (eds Fusa Miyake, Ilya Usoskin, & Stepan Poluianov) (IOP Publishing, 2019).
- 9.Usoskin IG, et al. Revisited reference solar proton event of 23 february 1956: assessment of the cosmogenic-isotope method sensitivity to extreme solar events. J. Geophys. Res.: Space Phys. 2020;125:e2020JA027921. [Google Scholar]
- 10.Reinhold T, et al. The sun is less active than other solar-like stars. Science. 2020;368:518–521. doi: 10.1126/science.aay3821. [DOI] [PubMed] [Google Scholar]
- 11.Okamoto S, et al. Statistical properties of superflares on solar-type stars: results using all of the kepler primary mission data. Astrophysical J. 2021;906:72. [Google Scholar]
- 12.Beer J, Vonmoos M, Muscheler R. Solar variability over the past several millennia. Space Sci. Rev. 2006;125:67–79. [Google Scholar]
- 13.Sakurai H, et al. Prolonged production of 14C during the ~660 BCE solar proton event from Japanese tree rings. Sci. Rep. 2020;10:660. doi: 10.1038/s41598-019-57273-2. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 14.Miyake F, Nagaya K, Masuda K, Nakamura T. A signature of cosmic-ray increase in AD 774-775 from tree rings in Japan. Nature. 2012;486:240–242. doi: 10.1038/nature11123. [DOI] [PubMed] [Google Scholar]
- 15.Usoskin IG. A history of solar activity over millennia. Living Rev. Sol. Phys. 2013;10:7–+. [Google Scholar]
- 16.Potgieter MS. Solar modulation of cosmic rays. Living Rev. Sol. Phys. 2013;10:3. [Google Scholar]
- 17.Brehm N, et al. Eleven-year solar cycles over the last millennium revealed by radiocarbon in tree rings. Nat. Geosci. 2021;14:10–15. [Google Scholar]
- 18.Muscheler R, et al. Solar activity during the last 1000 yr inferred from radionuclide records. Quat. Sci. Rev. 2007;26:82–97. [Google Scholar]
- 19.Park J, Southon J, Fahrni S, Creasman PP, Mewaldt R. Relationship between Solar Activity and delta c-14 peaks in AD 775, AD 994, AND 660 BC. Radiocarbon. 2017;59:1147–1156. [Google Scholar]
- 20.Mekhaldi F, et al. Multiradionuclide evidence for the solar origin of the cosmic-ray events of AD 774/5 and 993/4. Nat. Commun. 2015;6:8611. doi: 10.1038/ncomms9611. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 21.Usoskin, I. G., Solanki, S. K., Kovaltsov, G. A., Beer, J. & Kromer, B. Solar proton events in cosmogenic isotope data. Geophys Res Lett33, 10.1029/2006GL026059 (2006)
- 22.Miyake F, et al. A Single-Year Cosmic Ray Event at 5410 BCE Registered in 14C of Tree Rings. Geophys Res Lett. 2021;48:e2021GL093419. doi: 10.1029/2021GL093419. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 23.Timothy Jull AJ, et al. Rapid 14C excursion at 3372-3371 BCE not observed at two different locations. Nat. Commun. 2021;12:712. doi: 10.1038/s41467-020-20695-y. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 24.Berggren, A.-M. et al. A 600-year annual 10Be record from the NGRIP ice core, Greenland. Geophys Res Lett36, 10.1029/2009GL038004 (2009)
- 25.Beer J, et al. Use of 10Be in polar ice to trace the 11-year cycle of solar activity. Nature. 1990;347:164–166. [Google Scholar]
- 26.Friedrich, M. et al. The 12,460-year Hohenheim Oak and Pine Tree-Ring Chronology from Central Europe—A Unique Annual Record for Radiocarbon Calibration and Paleoenvironment Reconstructions. 46, 1111–1122 (2004).
- 27.Reimer PJ, et al. The IntCal20 northern hemisphere radiocarbon age calibration curve (0–55 cal kBP) Radiocarbon. 2020;62:725–757. [Google Scholar]
- 28.O'Hare P, et al. Multiradionuclide evidence for an extreme solar proton event around 2,610 B.P. (approximately 660 BC) Proc. Natl Acad. Sci. USA. 2019;116:5961–5966. doi: 10.1073/pnas.1815725116. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 29.Adolphi F, Muscheler R. Synchronizing the Greenland ice core and radiocarbon timescales over the Holocene – Bayesian wiggle-matching of cosmogenic radionuclide records. Clim. 2016;12:15–30. [Google Scholar]
- 30.Veres D, et al. The Antarctic ice core chronology (AICC2012): an optimized multi-parameter and multi-site dating approach for the last 120 thousand years. Clim. 2013;9:1733–1748. [Google Scholar]
- 31.Yiou F, et al. Beryllium 10 in the Greenland Ice Core Project ice core at Summit, Greenland. J. Geophys. Res.: Oceans. 1997;102:26783–26794. [Google Scholar]
- 32.Steinhilber F, et al. 9,400 years of cosmic radiation and solar activity from ice cores and tree rings. Proc. Natl Acad. Sci. 2012;109:5967. doi: 10.1073/pnas.1118965109. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 33.Muscheler R, et al. Changes in the carbon cycle during the last deglaciation as indicated by the comparison of 10Be and 14C records. Earth Planet. Sci. Lett. 2004;219:325–340. [Google Scholar]
- 34.Denaire A, et al. The cultural project: formal chronological modelling of the early and middle neolithic sequence in lower Alsace. J. Archaeological Method Theory. 2017;24:1072–1149. doi: 10.1007/s10816-016-9307-x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 35.Jakucs J, et al. Between the Vinča and Linearbandkeramik Worlds: The Diversity of Practices and Identities in the 54th–53rd Centuries cal BC in Southwest Hungary and Beyond. J. World Prehistory. 2016;29:267–336. doi: 10.1007/s10963-016-9096-x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 36.Jakucs J, et al. Rows with the neighbours: the short lives of longhouses at the Neolithic site of Versend-Gilencsa. Antiquity. 2018;92:91–117. [Google Scholar]
- 37.Usoskin IG, Kovaltsov GA. Occurrence of extreme solar particle events: assessment from historical proxy data. Astrophysical J. 2012;757:92. [Google Scholar]
- 38.Nicolussi K, et al. A 9111 year long conifer tree-ring chronology for the European Alps: a base for environmental and climatic investigations. Holocene. 2009;19:909–920. [Google Scholar]
- 39.Nicolussi, K., Weber, G., Patzelt, G. & Thurner, A. A question of time: extension of the Eastern Alpine Conifer Chronology back to 10071 b2k. GFZ Potsdam, Scientific Technical Report STR15, Potsdam, 69–73 (2015).
- 40.Panovska S, Constable CG, Korte M. Extending global continuous geomagnetic field reconstructions on timescales beyond human civilization. Geochem., Geophysics, Geosystems. 2018;19:4757–4772. [Google Scholar]
- 41.Knudsen MF, et al. Variations in the geomagnetic dipole moment during the Holocene and the past 50 kyr. Earth Planet. Sci. Lett. 2008;272:319–329. [Google Scholar]
- 42.Adolphi F, et al. Connecting the Greenland ice-core and U∕Th timescales via cosmogenic radionuclides: testing the synchroneity of Dansgaard–Oeschger events. Clim. 2018;14:1755–1781. [Google Scholar]
- 43.Paleari CI, et al. Cosmogenic radionuclides reveal an extreme solar particle storm near a solar minimum 9125 years BP. Nat. Commun. 2022;13:214. doi: 10.1038/s41467-021-27891-4. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 44.Cliver EW, Hayakawa H, Love JJ, Neidig DF. On the size of the flare associated with the solar proton event in 774 AD. Astrophysical J. 2020;903:41. [Google Scholar]
- 45.Dyer CS, Lei F, Clucas SN, Smart DF, Shea MA. Solar particle enhancements of single-event effect rates at aircraft altitudes. IEEE Trans. Nucl. Sci. 2003;50:2038–2045. [Google Scholar]
- 46.Cannon, P., Angling, M., Barclay, L. & Al, E. Extreme space weather:impacts on engineered systems and infrastructure (Royal Academy of Engineering, 2013).
- 47.Sookdeo A, et al. Quality dating: a well-defined protocol implemented at ETH for high-precision 14C dates tested on Late Glacial wood. Radiocarbon. 2020 doi: 10.1017/RDC.2019.132. [DOI] [Google Scholar]
- 48.Němec M, Wacker L, Hajdas I, Gäggeler H. Alternative methods for cellulose preparation for AMS measurement. Radiocarbon. 2016;52:1358–1370. [Google Scholar]
- 49.Welte C, et al. Towards the limits: analysis of microscale C-14 samples using EA-AMS. Nucl. Instrum. Methods Phys. Res. Sect. B-Beam Interact. Mater. At. 2018;437:66–74. [Google Scholar]
- 50.Wacker L, Nemec M, Bourquin J. A revolutionary graphitisation system: fully automated, compact and simple. Nucl. Instrum. Methods Phys. Res. Sect. B: Beam Interact. Mater. At. 2010;268:931–934. [Google Scholar]
- 51.Synal HA, Stocker M, Suter M. MICADAS: a new compact radiocarbon AMS system. Nucl. Instrum. Methods Phys. Res. Sect. B-Beam Interact. Mater. At. 2007;259:7–13. [Google Scholar]
- 52.Knowles TDJ, Monaghan PS, Evershed RP. Radiocarbon sample preparation procedures and the first status report from the bristol radiocarbon AMS (BRAMS) facility. Radiocarbon. 2019;61:1541–1550. [Google Scholar]
- 53.Büntgen, U. et al. Tree rings reveal globally coherent signature of cosmogenic radiocarbon events in 774 and 993 CE. Nature Communications9, 10.1038/s41467-018-06036-0 (2018) [DOI] [PMC free article] [PubMed]
- 54.Guttler D, et al. Rapid increase in cosmogenic 14C in AD 775 measured in New Zealand kauri trees indicates short-lived increase in 14C production spanning both hemispheres. Earth Planet. Sci. Lett. 2015;411:290–297. [Google Scholar]
- 55.Usoskin IG, et al. Solar cyclic activity over the last millennium reconstructed from annual 14C data⋆. AA. 2021;649:A141. [Google Scholar]
- 56.Alken P, et al. International Geomagnetic Reference Field: the thirteenth generation. Earth, Planets Space. 2021;73:49. [Google Scholar]
- 57.Usoskin IG, Mironova IA, Korte M, Kovaltsov GA. Regional millennial trend in the cosmic ray induced ionization of the troposphere. J. Atmos. Sol.-Terrestrial Phys. 2010;72:19–25. [Google Scholar]
- 58.Korte M, Constable CG. The geomagnetic dipole moment over the last 7000 years—new results from a global model. Earth Planet. Sci. Lett. 2005;236:348–358. [Google Scholar]
- 59.Koldobskiy S, Raukunen O, Vainio R, Kovaltsov GA, Usoskin I. New reconstruction of event-integrated spectra (spectral fluences) for major solar energetic particle events⋆. AA. 2021;647:A132. [Google Scholar]
- 60.Raukunen O, et al. Two solar proton fluence models based on ground level enhancement observations. J. Space Weather Space Clim. 2018;8:A04. [Google Scholar]
- 61.Poluianov SV, Kovaltsov GA, Mishev AL, Usoskin IG. Production of cosmogenic isotopes 7Be, 10Be, 14C, 22Na, and 36Cl in the atmosphere: Altitudinal profiles of yield functions. J. Geophys. Res.: Atmospheres. 2016;121:8125–8136. [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Data Availability Statement
The excel data that support the findings of this study are available in PANGEA at doi. Source data are provided with this paper.
Any computer code used for evaluation of the results of this study will be available on request.