Skip to main content
PMC home page
Biochemical Journal logoLink to Biochemical Journal
. 1990 Aug 1;269(3):697–707. doi: 10.1042/bj2690697

Enzyme kinetics and metabolic control. A method to test and quantify the effect of enzymic properties on metabolic variables.

L Acerenza 1, H Kacser 1
PMCID: PMC1131644  PMID: 2390063

Abstract

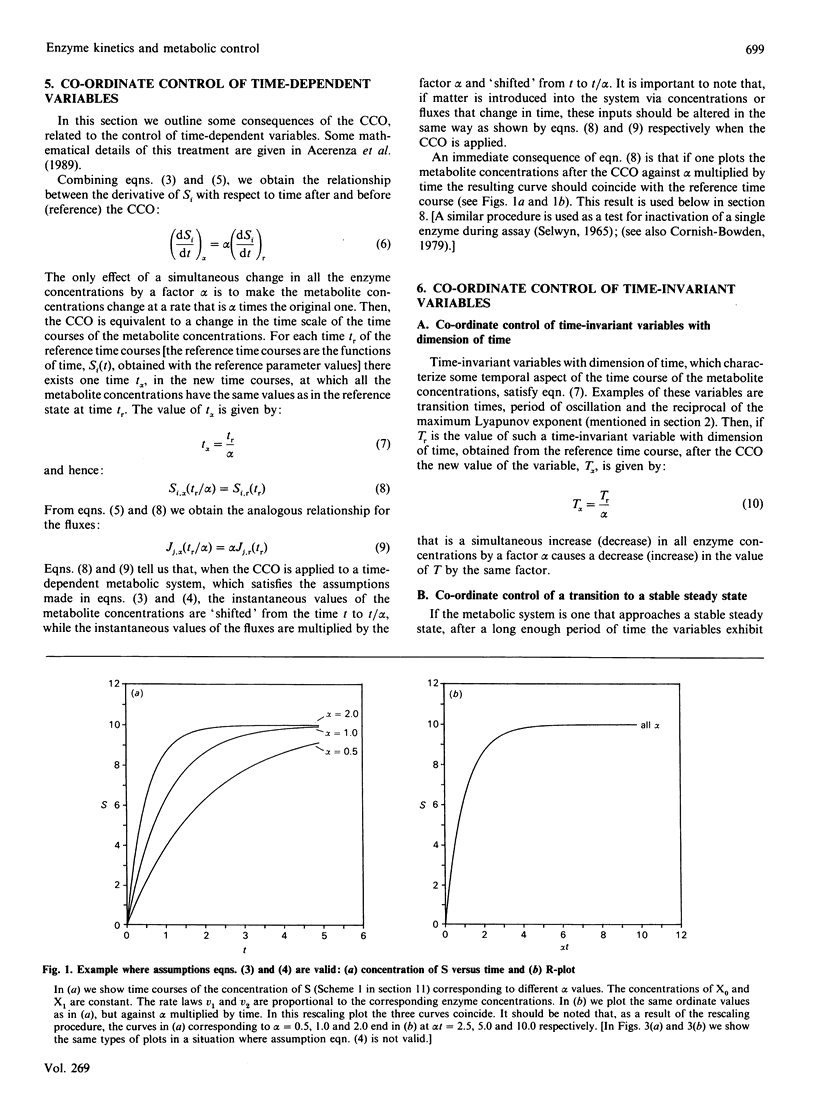
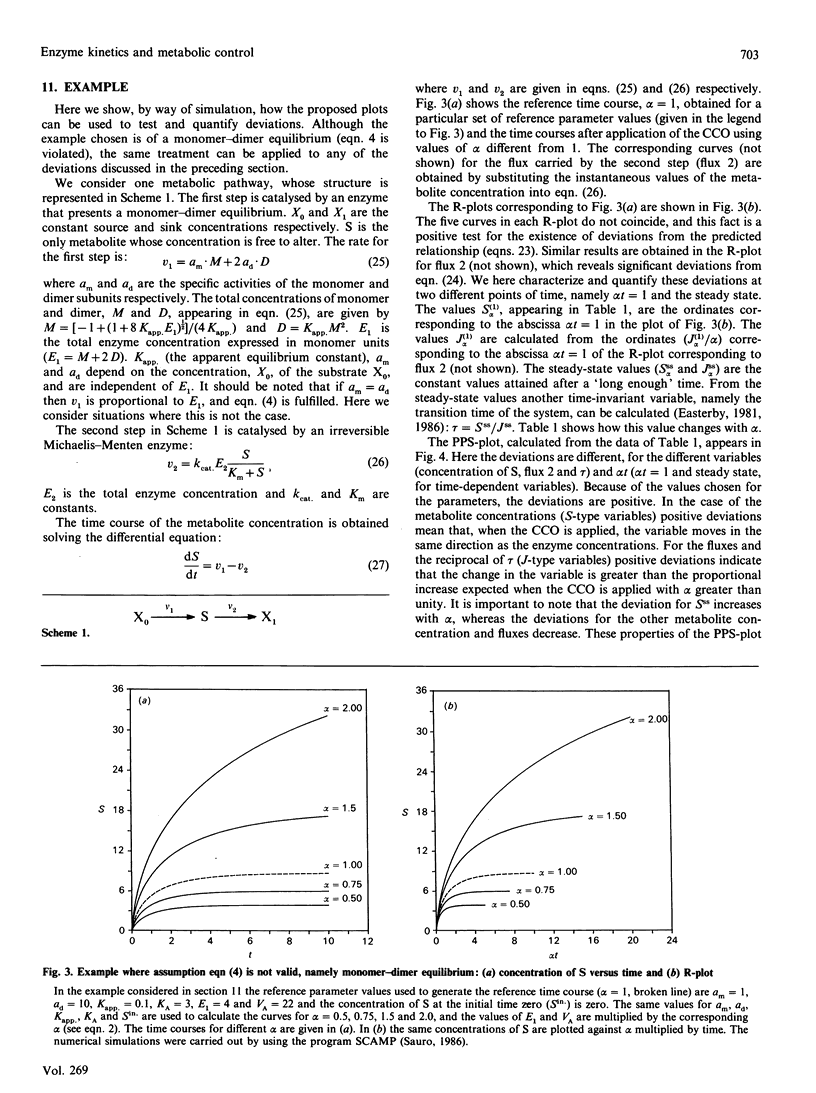
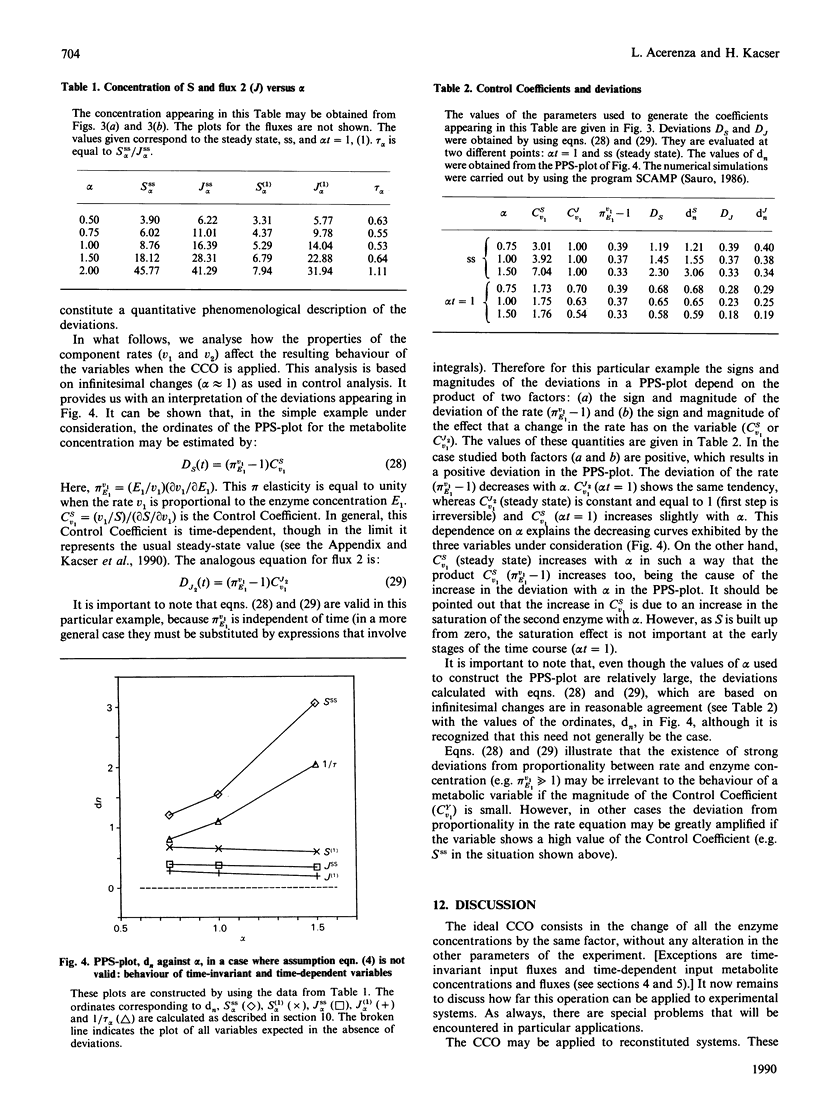
It is usual to study the sensitivity of metabolic variables to small (infinitesimal) changes in the magnitudes of individual parameters such as an enzyme concentration. Here, the effect that a simultaneous change in all the enzyme concentrations by the same factor alpha (Co-ordinate-Control Operation, CCO) has on the variables of time-dependent metabolic systems is investigated. This factor alpha can have any arbitrary large value. First, we assume, for each enzyme measured in isolation, the validity of the steady-state approximation and the proportionality between reaction rate and enzyme concentration. Under these assumptions, any time-invariant variable may behave like a metabolite concentration, i.e. S alpha = Sr (S-type), or like a flux, i.e. J alpha = alpha Jr (J-type). The subscripts r and alpha correspond to the values of the variable before and after the CCO respectively. Similarly, time-dependent variables may behave according to S alpha (t/alpha) = Sr (t) (S-type) or to J alpha (t/alpha) = alpha J r (t) (J-type). A method is given to test these relationships in experimental systems, and to quantify deviations from the predicted behaviour. A positive test for deviations proves the violation of some of the assumptions made. However, the breakdown of the assumptions in an enzyme-catalysed reaction, studied in isolation, may or may not affect significantly the behaviour of the system when the component reaction is embedded in the metabolic network.

Full text

PDF
707

Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. Acerenza L., Sauro H. M., Kacser H. Control analysis of time-dependent metabolic systems. J Theor Biol. 1989 Apr 20;137(4):423–444. doi: 10.1016/s0022-5193(89)80038-4. [DOI] [PubMed] [Google Scholar]
  2. Acerenza L., Sauro H. M., Kacser H. Control analysis of time-dependent metabolic systems. J Theor Biol. 1989 Apr 20;137(4):423–444. doi: 10.1016/s0022-5193(89)80038-4. [DOI] [PubMed] [Google Scholar]
  3. Ciferri O., Sora S., Tiboni O. Effect of gene dosage on tryptophan synthetase activity in Saccharomyces cerevisiae. Genetics. 1969 Mar;61(3):567–576. doi: 10.1093/genetics/61.3.567. [DOI] [PMC free article] [PubMed] [Google Scholar]
  4. Das J., Busse H. G. Long term oscillation in glycolysis. J Biochem. 1985 Mar;97(3):719–727. doi: 10.1093/oxfordjournals.jbchem.a135111. [DOI] [PubMed] [Google Scholar]
  5. Easterby J. S. A generalized theory of the transition time for sequential enzyme reactions. Biochem J. 1981 Oct 1;199(1):155–161. doi: 10.1042/bj1990155. [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Easterby J. S. The effect of feedback on pathway transient response. Biochem J. 1986 Feb 1;233(3):871–875. doi: 10.1042/bj2330871. [DOI] [PMC free article] [PubMed] [Google Scholar]
  7. Eschrich K., Schellenberger W., Hofmann E. In vitro demonstration of alternate stationary states in an open enzyme system containing phosphofructokinase. Arch Biochem Biophys. 1980 Nov;205(1):114–121. doi: 10.1016/0003-9861(80)90089-2. [DOI] [PubMed] [Google Scholar]
  8. Heinrich R., Rapoport T. A. A linear steady-state treatment of enzymatic chains. General properties, control and effector strength. Eur J Biochem. 1974 Feb 15;42(1):89–95. doi: 10.1111/j.1432-1033.1974.tb03318.x. [DOI] [PubMed] [Google Scholar]
  9. Heinrich R., Rapoport T. A. A linear steady-state treatment of enzymatic chains. General properties, control and effector strength. Eur J Biochem. 1974 Feb 15;42(1):89–95. doi: 10.1111/j.1432-1033.1974.tb03318.x. [DOI] [PubMed] [Google Scholar]
  10. Heinrich R., Rapoport T. A. Mathematical analysis of multienzyme systems. II. Steady state and transient control. Biosystems. 1975 Jul;7(1):130–136. doi: 10.1016/0303-2647(75)90050-7. [DOI] [PubMed] [Google Scholar]
  11. Heinrich R., Rapoport T. A. Mathematical analysis of multienzyme systems. II. Steady state and transient control. Biosystems. 1975 Jul;7(1):130–136. doi: 10.1016/0303-2647(75)90050-7. [DOI] [PubMed] [Google Scholar]
  12. Hofmann E., Eschrich K., Schellenberger W. Temporal organization of the phosphofructokinase/fructose-1,6-biphosphatase cycle. Adv Enzyme Regul. 1985;23:331–362. doi: 10.1016/0065-2571(85)90055-x. [DOI] [PubMed] [Google Scholar]
  13. Kacser H., Burns J. A. The control of flux. Symp Soc Exp Biol. 1973;27:65–104. [PubMed] [Google Scholar]
  14. Kacser H., Burns J. A. The control of flux. Symp Soc Exp Biol. 1973;27:65–104. [PubMed] [Google Scholar]
  15. Kacser H., Sauro H. M., Acerenza L. Enzyme-enzyme interactions and control analysis. 1. The case of non-additivity: monomer-oligomer associations. Eur J Biochem. 1990 Feb 14;187(3):481–491. doi: 10.1111/j.1432-1033.1990.tb15329.x. [DOI] [PubMed] [Google Scholar]
  16. MORTIMER R. K. Radiobiological and genetic studies on a polyploid series (haploid to hexaploid) of Saccharomyces cerevisiae. Radiat Res. 1958 Sep;9(3):312–326. [PubMed] [Google Scholar]
  17. Markus M., Kuschmitz D., Hess B. Chaotic dynamics in yeast glycolysis under periodic substrate input flux. FEBS Lett. 1984 Jul 9;172(2):235–238. doi: 10.1016/0014-5793(84)81132-1. [DOI] [PubMed] [Google Scholar]
  18. Meléndez-Hevia E., Torres N. V., Sicilia J., Kacser H. Control analysis of transition times in metabolic systems. Biochem J. 1990 Jan 1;265(1):195–202. doi: 10.1042/bj2650195. [DOI] [PMC free article] [PubMed] [Google Scholar]
  19. Mizraji E., Acerenza L., Hernández J. Time delays in metabolic control systems. Biosystems. 1988;22(1):11–17. doi: 10.1016/0303-2647(88)90046-9. [DOI] [PubMed] [Google Scholar]
  20. Neet K. E., Ainslie G. R., Jr Hysteretic enzymes. Methods Enzymol. 1980;64:192–226. doi: 10.1016/s0076-6879(80)64010-5. [DOI] [PubMed] [Google Scholar]
  21. Salerno C., Ovádi J., Keleti T., Fasella P. Kinetics of coupled reactions catalyzed by aspartate aminotransferase and glutamate dehydrogenase. Eur J Biochem. 1982 Jan;121(3):511–517. doi: 10.1111/j.1432-1033.1982.tb05816.x. [DOI] [PubMed] [Google Scholar]
  22. Sauro H. M., Kacser H. Enzyme-enzyme interactions and control analysis. 2. The case of non-independence: heterologous associations. Eur J Biochem. 1990 Feb 14;187(3):493–500. doi: 10.1111/j.1432-1033.1990.tb15330.x. [DOI] [PubMed] [Google Scholar]
  23. Segel L. A. On the validity of the steady state assumption of enzyme kinetics. Bull Math Biol. 1988;50(6):579–593. doi: 10.1007/BF02460092. [DOI] [PubMed] [Google Scholar]
  24. Selwyn M. J. A simple test for inactivation of an enzyme during assay. Biochim Biophys Acta. 1965 Jul 29;105(1):193–195. doi: 10.1016/s0926-6593(65)80190-4. [DOI] [PubMed] [Google Scholar]
  25. Torres N. V., Souto R., Meléndez-Hevia E. Study of the flux and transition time control coefficient profiles in a metabolic system in vitro and the effect of an external stimulator. Biochem J. 1989 Jun 15;260(3):763–769. doi: 10.1042/bj2600763. [DOI] [PMC free article] [PubMed] [Google Scholar]
  26. Torres N. V., Souto R., Meléndez-Hevia E. Study of the flux and transition time control coefficient profiles in a metabolic system in vitro and the effect of an external stimulator. Biochem J. 1989 Jun 15;260(3):763–769. doi: 10.1042/bj2600763. [DOI] [PMC free article] [PubMed] [Google Scholar]

Articles from Biochemical Journal are provided here courtesy of The Biochemical Society

RESOURCES