The allometry of ornaments and weapons

Abstract

Exaggerated male traits that have evolved under sexual selection include ornaments to attract mates and weapons to deter rivals. Data from studies of many such traits in diverse kinds of organisms show that they almost universally exhibit positive allometries. Both ornaments and weapons increase disproportionately with overall body size, resulting in scaling exponents within species that are consistently >1.0 and usually in the range 1.5–2.5. We show how scaling exponents reflect the relative fitness advantages of ornaments vs. somatic growth by using a simple mathematical model of resource allocation during ontogeny. Because the scaling exponents are similar for the different taxonomic groups, it follows that the fitness advantages of investing in ornaments also are similar. The model also shows how selection for ornaments influences body size at first reproduction and explains why interspecific allometries have consistently lower exponents than intraspecific ones.

Allometry is the study of how characteristics of organisms vary or scale with body size. Such variation has been described traditionally by a so-called allometric equation (1–3) of the form

where Y is a dependent variable, such as antler size or heart rate, Y₀ is a normalization constant or “Y intercept,” X is a measure of body size, typically mass, and b is an allometric or scaling exponent. For physiological and life history traits, b is typically <1 and often a simple multiple of 1/4, such as 1/4, 3/4, or −1/4 (4, 5). For morphological traits, however, so long as Y and X have the same dimensions, b is typically close to 1. This condition is referred to as isometry, whereas when b is >1 or <1, these conditions are referred to as positive or negative allometry, respectively. In 1931, Julian Huxley (6, 7) showed that horns and antlers exhibit positive allometry. Several subsequent authors have suggested that this observation also applies to most morphological structures that function as ornaments to attract mates or as weapons to combat rivals (e.g., 8–12). Indeed, Darwin (13) and many subsequent authors have remarked that sexual selection favors the evolution of “exaggerated” male traits. In 1974, S. J. Gould (6) wrote “The positive allometry of horns and antlers is one of the most pervasive and poorly understood regularities in the study of form and function.”

These positive allometries raise the conceptual question, what are the fitness consequences of allocating energy and materials to ornaments or weapons during ontogenenetic growth and development? It is necessary to understand why, as an individual grows, it should allocate resources differentially to an ornament or weapon, rather than in equal proportions to all structures, which would result in isometric scaling. Additionally, positive allometries often are observed across species of related organisms that differ in average adult body size. The classic examples of exaggerated sexually selected traits are the antlers of Irish elk (Megaloceros giganteus), which are largest in the largest individuals of this giant deer species. So it is also necessary to explain why, as species have evolved larger bodies, they have evolved simultaneously even more exaggerated ornaments and weapons.

The explanation for such positive allometries must lie with the fitness consequences of allocating resources differentially to ornaments or weapons rather than to overall body size. Several authors have addressed this problem (e.g., 8, 9, 12, 14–18). Most have made qualitative verbal arguments rather than mathematical models. Recently, Bonduriansky and Day (19) presented models suggesting that any pattern, isometry or positive or negative allometry, is possible. Here we summarize many of the published empirical studies of ornament allometry, show that the exponents are nearly always substantially >1, and develop a mathematical model that can explain why sexual selection so often leads to such positive allometries.

Patterns of Ornament and Weapon Allometry

We first synthesize data for sexually selected morphological structures in different kinds of organisms. We address three questions: (i) How do the exponents vary among individuals of different sizes within populations, and among populations of different average body size? (ii) How do the exponents vary across traits and taxa? And (iii) are there consistent patterns depending on whether the traits function as ornaments, weapons, or both? Our analysis is based on 13 studies of 9 major taxa and 284 species (Table 1, which is published as supporting information on the PNAS web site). Rather than exhaustively surveying the literature, we focused on studies that present data on multiple samples of individuals, populations, or species and that use standardized methods to measure both body size and the trait of interest. The database includes vertebrates and invertebrates, and terrestrial, freshwater, and marine (intertidal) animals.

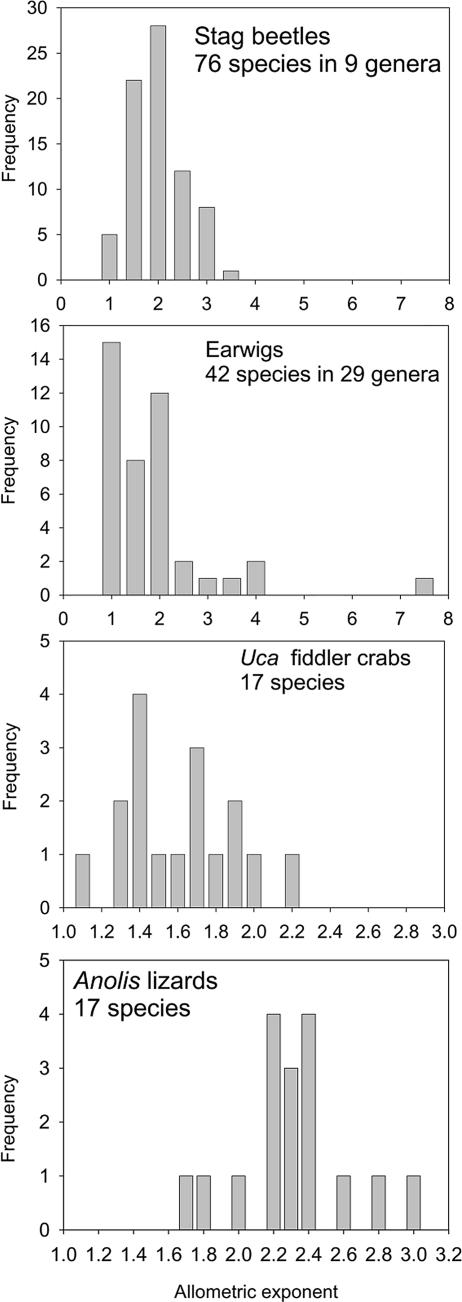
Fig. 1 and Table 1 summarize our compilation of data. Fig. 1 plots frequency distributions for the exponents for intraspecific allometries for the extensive data for horns of stag beetles, claws of fiddler crabs, forceps of earwigs, and dewlaps of Anolis lizards. Table 1 gives additional examples, including the classic deer antlers. Across the entire data set, allometric scaling exponents for individuals within populations range from 0.93–15.7, with a distinct mode between 1.5 and 2.5, so the vast majority of intraspecific exponents are >1.

Fig. 1.

Frequency histograms of allometric exponents obtained by various authors by fitting power functions to data for intraspecific variation in ornament size as a function of body size in different groups of animals: horns of stag beetles (Lucanidae; ref. 20), claws of Uca fiddler crabs (21), forceps of earwigs (Dermaptera; ref. 22), and dewlaps of Anolis lizards (23).

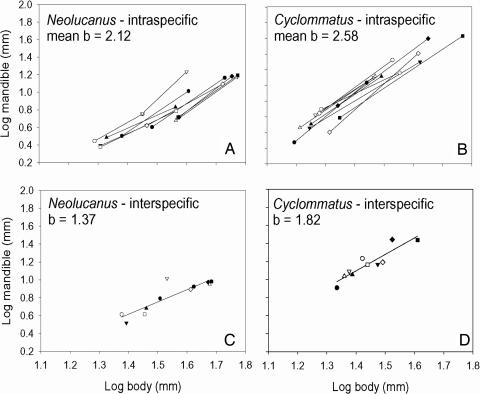
Interspecific allometries nearly always have lower exponents than intraspecific ones for the same trait and taxon (Fig. 2 and Table 1). Nevertheless, they are also consistently >1, ranging from 1.1–3.4 with a mode between 1.5 and 2. Fig. 2 shows examples of relationships for stag beetles, comparing allometries within species of Neolucanus and Cyclommatus and to interspecific allometries for these same two genera.

Fig. 2.

Comparison of intraspecific and interspecific allometries for the horns of stag beetles. (A and B) Intraspecific variation in 11 species of Neolucanus (A) and 10 species of Cyclommatus (B), where each line represents the allometric relationship for a different species, and the average exponent for these relationships is 2.12 for Neolucanus and 2.58 for Cyclommatus. (C and D) Interspecific allometries for the 11 species of Neolucanus (C) and 10 species of Cyclommatus (D), where each data point represents an average value of horn and body length for all measured individuals in a species, and the exponents are 1.37 for Neolucanus and 1.82 for Cyclommatus. Each of the symbols represents a different species: The symbol in the interspecifc plots represents the mean, and the pair of identical symbols connected by a line in the intraspecific plots represents the range of variation (±2 SD). Data were replotted and exponents were recalculated from Kawano (20). In A and B, note that the relationships for larger species are displaced to the right (lower Y₀), reflecting increasing size at first reproduction.

There seem to be no consistent differences in the exponents for ornaments as opposed to weapons: Values for traits that function exclusively as ornaments, e.g., Anolis dewlaps and Poecilia and Xiphophorus fins, bracket most values for traits that function primarily as weapons or have a dual function. We conclude that across a wide variety of traits and taxa, allometric exponents for both ornaments and weapons almost invariably show positive allometries, with exponents typically in the range of 1.5–2.5 and consistently greater for intraspecific comparisons than interspecific ones.

Modeling Investment Allocations and Fitness Payoffs

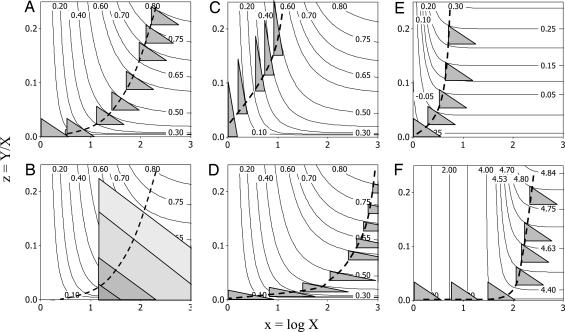
Fig. 3A presents a general model showing how energy or limiting material resources (e.g., mineral nutrients for antlers) are allocated during ontogeny to increase the relative size of an ornament (z = Y/X) as a function of increasing body size (X). While small, the male invests predominately in growth with a concomitant increase in body mass. After reaching reproductive size, in response to sexual selection to acquire mates, the male begins to invest in an ornament or weapon as well as in continued growth. We use the term “reproductive size” loosely, to mean the size at which ornaments become noticeable (e.g., z > 0.05). The outcome of sexual and natural selection is the pattern of resource allocation that maximizes overall fitness. Our model assumes that fitness increases with increasing allocation to both ornament and body, but there is a tradeoff between increasing the relative size of the ornament and increasing overall body size. The tradeoff is specified by the hypotenuse of the right triangle with sides z and log₁₀ X. The payoff is given by a fitness function, shown in the graphical model by plotting contour curves of equal fitness, with increasing fitness toward the upper right (Fig. 3A).

Fig. 3.

Diagram showing how the optimal strategy (dashed line) for allocation to an ornament is calculated. Axes are x = log₁₀ (body size) and z = (ornament size)/(body size) as defined in the text. The triangles indicate allocation tradeoffs between ornament and body, the curved contour lines connect points of equal fitness, and the optimal strategy occurs when the slope of the contour is exactly equal to the slope of the hypotenuse, as derived mathematically in the text. Parameter values are given in Table 2. (A) The case for organisms that continue to grow throughout life. The allocation options each successive year are shown as gray triangles. (B) The analogous case for organisms that start reproducing at different body sizes with different quantities of resources and do not grow thereafter. The similarly shaped allocation triangles comprise a nested set, so that males with fewer resources and smaller body sizes have options triangles that are smaller (darker shading) than males with more resources. Consequently, the optimal allometry (dashed line) is the same as in A. (C and D) Holding the fitness function constant but allocating relatively fewer resources to ornament (C to D) reduces the height of the options triangle and leads to increased body size at first reproduction but does not change the exponent (b = 1.8; see Modeling Investment Allocations and Fitness Payoffs). (E and F) Holding the tradeoff options constant as in A but changing the fitness function can change both size at first reproduction and the allometric exponent. Increasing the relative fitness of males expressing ornaments at large body size (E to F) leads to increased body size at first reproduction, but in this comparison, does not change the allometric exponent. However, changing the shape of the fitness landscape (A to E) changes the allometric exponent, increasing it here from b = 1.8 to b = 3.8.

Our model uses the framework developed by Sibly and Calow (24) and builds on recent theoretical treatments by Bonduriansky and Day (19) and Lindström et al. (25). Fitness, f, is presumed to increase with body mass X and relative ornament size z according to a mathematical function f = f(x, z), where x = log₁₀X and z = Y/X. The model makes the following assumptions: (i) during some period (e.g., 1 year), an individual of body size x and ornament of relative size z has the option of increasing its body size by an amount Δx and its relative ornament size by Δz, subject to; (ii) an allocation tradeoff in which the total energy and material content of body plus ornament is conserved; this principle of allocation gives a negative relationship between Y and X, and a negative relationship between Δx and Δz, so that the options lie on a line that we approximate as the hypotenuse of a right triangle (see gray triangles in Fig. 3A); and (iii) the triangle has a geometrically similar shape (ratio of Δx to Δz) regardless of its position in the (x, z) plane. The absolute sizes of the triangles decrease with increasing body size, because whole-organism production rate scales as the 3/4 power of body mass (e.g., refs. 4 and 26).

To motivate the formal mathematical treatment, we now analyze the example in Fig. 3A. The optimal strategy is that which gives the greatest increase in fitness, given that tradeoffs are constrained to lie on the negatively sloped hypotenuse of the options triangle. The optimal strategy is to allocate resources to the point where a fitness contour just touches the negatively sloped tradeoff line. For immature animals of small size (at the bottom left-hand corner of Fig. 3A), the optimal strategy is to allocate almost all resources to growth. Consequently the options triangle initially moves horizontally to the right. Upon reaching reproductive size, however, the hypotenuse becomes parallel to one of the fitness contours and, thenceforth, resources are increasingly allocated to ornament, so that in each successive year, the options set follows the fitness contours up and to the right (Fig. 3A). Mathematically, this is given by the equation condition

where f_x and f_z denote partial differentiation with respect to x and z respectively, and t is the slope of the hypotenuse (Supporting Text, which is published as supporting information on the PNAS web site). This equation defines the optimal strategy in terms of x and z (see example in Fig. 3A). The quantities f_x and f_z in Eq. 2 indicate the strength of selection on body size and relative ornament size, respectively. Technically f_x is the rate at which fitness increases with x, so it measures the fitness advantage of unit investment in increased body size. Similarly f_z indicates the net benefit of increasing the relative size of the ornament. The slope, t, of the options triangle indicates the terms of the tradeoff: the more negative the slope, the larger the relative allocation to ornament.

In the hypothetical example shown in Fig. 3 A–D, fitness is characterized by a particular mathematical function

In this example, partial differentiation of f with respect to x and y yields

(see Appendix 2), and we assume that t = −0.08. Inserting these values into Eq. 2 yields, after simplification, Y = 0.00434 X ^1.8. So, as the animal grows, its allocation strategy follows the dashed line, which in this example corresponds to an allometric exponent b = 1.8. In general, applying Eq. 2 to the fitness function

gives Y = Y₀X^b, which is Eq. 1. Then, Eq. 4 specifies a fitness function that depends on the parameters x, z, t, Y₀, and b, for which the optimal strategy is the allometric equation. Is the fitness function of Eq. 4 unique in this respect? In fact, any monotonically increasing transformation of f yields Eq. 1 as the optimal strategy, but we conjecture that there are no others. Note that the fitness function f and the parameter values for t, Y₀, and b used in this example (Fig. 3; see also Table 2, which is published as supporting information on the PNAS web site) have not been derived from first principles. Additional research could profitably be devoted to exploring the mechanistic basis and mathematical form of the fitness payoff.

The above model is developed for organisms that continue to grow after reaching reproductive size. In such organisms, it also accounts for within-population variation among individuals, because most of the variation in body size within such a population will be due to variation in age. The same model also can be applied to organisms in which different males start reproducing at different body sizes and do not grow thereafter. Examples include holometabolus insects, such as the stag and rhinoceros beetles in Figs. 1 and 2 and Table 1. In such cases, the tradeoff options are given by a nested series of similar triangles, all with the right angle at the smallest size of reproduction (Fig. 3B). An individual that has accumulated more resources has a proportionately larger options triangle, and the optimal allocation again is given by the point where the hypotenuse is parallel to a fitness contour. So the intraspecific allometric exponent is the slope of a line running through the points of optimal allocation as before. Note that the model for determinant growth gives the same exponent as the version for indeterminant growth so long as the allocation tradeoff and fitness function remain the same (compare Fig. 3 A and B).

What happens if the allocation tradeoffs change? Fig. 3 C and D show the effects of changing the terms of the allocation tradeoff between ornaments and somatic growth, while keeping the fitness functions the same as in Fig. 3 A and B. In Fig. 3C, relative allocation to ornament is greater than before, i.e., a larger ornament for a given sacrifice of somatic growth. The result is taller options triangles than in Fig. 3 A and B. Conversely, in Fig. 3D, relative allocation to ornament is reduced, so the options triangles are shorter. Despite these differences in allocation, however, the allometric exponents remain the same, b = 1.8. Mathematically this relationship is because the fitness contours and Eq. 4 remain the same. In order for this condition to happen, the normalization constant Y₀ has to vary to compensate for the changes in t (see Table 2 for details). The result is that in Fig. 3C, the trajectory starts at z = 0.027 when x = 0, whereas in Fig. 3D, the trajectory does not reach z = 0.027 until body size is 100 times larger and x = 2. So, as the relative allocation to ornament decreases, the optimal strategy is to attain a larger body size before reproducing and investing in ornament.

Now, what happens when the fitness payoffs change? In Fig. 3 E and F, the shapes of the fitness contours change, but the shape of the options triangle remains the same as in Fig. 3A. In Fig. 3E, small animals can get more fitness payoffs from ornaments of a given size than in Fig. 3A. Consequently, the optimal strategy is to start growing ornaments at a smaller size, decreasing the size at first reproduction. The triangles intersect more fitness contours vertically and follow a steeper trajectory, resulting in a higher allometric exponent (b = 3.8 for this example compared with b = 1.8 in Fig. 3A). In contrast, in Fig. 3F, ornaments differentially increase the fitness of larger animals. This relationship leads to increased body size at first reproduction but no change in the allometric exponent, b = 3.8, as in Fig. 3E.

In summary, −t represents the tradeoff between ornament and body, and f_x and f_z indicate the strength of selection on body size and relative ornament size, respectively. The general rule characterizing the optimal strategy is given by Eq. 2. This condition is the mathematical formulation of the rule that optimal allocation occurs when the slope of the fitness contour equals the slope of the tradeoff between ornament and body.

The model above was developed to describe optimal allocation to ornament size within the same individual as body size increases during ontogeny. We now show how it also can be applied across populations that differ in adult body size to explain why interspecific exponents are lower than intraspecific ones. Consider one species that exhibits the fitness contours and allocation tradeoffs shown in Fig. 3E. Now consider a closely related species that has a similar allocation triangle but cannot obtain fitness payoffs from ornaments until it has grown to a larger body size. Its optimal strategy is depicted in Fig. 3F. This second species will be selected to defer reproduction and allocate resources to somatic growth for a longer period. The allometric exponent, b, remains the same, but the normalization constant, Y₀, is decreased, and the allocation trajectory is shifted to the right compared with the closely related species in Fig. 3E. For further details see Supporting Text. Shifting the normalization constant to a lower value in a species of larger body size also reduces the degree of ornament exaggeration in the largest males. Consider the classic case of the Irish elk: The antlers of the largest individuals are indeed very large, but they would have been even larger had the interspecific trajectories simply extended an intraspecific relationship. Others of many possible examples are the stag beetles depicted in Fig. 2, where the interspecific exponent for each genus is the slope fitted to the average values of ornament and body size for each species (see also refs. 20 and 27). The shifts in normalization constants across species may not appear to be very large, but this seemingly small effect is largely a consequence of plotting body size on a logarithmic axis. So the model also can explain why interspecific allometric exponents are consistently lower than intraspecific ones.

Recent models of Bonduriansky and Day (19) and Lindström et al. (25) also develop a framework in which fitness is a mathematical function of trait size and body mass. Bonduriansky and Day's assumptions regarding allocation tradeoffs translate directly into ours: their parameter k is our parameter –t. There are, however, important differences in assumptions, and their model makes different predictions. For example, they predict an irrevocable switch from somatic growth to allocation to the trait at some point in development, rather than the continuous allocation to both that is optimal in our model. More generally, Bonduriansky and Day's models suggest that sexual selection can give rise to a wide variety of both positive and negative allometries. Lindström et al. (25) do not use their model to derive allometric exponents. In contrast to these models, our model not only accounts for the consistently positive allometries of ornaments and weapons but also predicts how allometric exponents and body size at first reproduction vary with allocation tradeoffs and fitness payoffs.

Why Do Ornaments and Weapons Exhibit Positive Allometries?

The empirical evidence shows that the ornaments and weapons used by males to acquire mates almost universally exhibit positive allometries. The exponents of Eq. 1 relating some dimension of ornament size to a comparable measure of overall body size are >1, often in the range of 1.5–2.5, and in a few cases >3. We develop a very simple, general mathematical and graphical model to explain the evolution of such exaggerated structures. The model quantifies the resource allocation tradeoffs and associated fitness payoffs of allocating energy or material resources to increase differentially the size of an ornament or weapon rather than allocating equally to all structures so as to increase overall body size. The main insights provided by the model are that (i) the allocation tradeoff has no effect on the allometric exponent (e.g., compare Fig. 3 A, C, and D, which have the same fitness contours but differ in the shapes of the options triangles). (ii) Instead, the allometric exponent is determined entirely by the shape of the fitness contours (e.g., Fig. 3 E and F have contours of a similar shape, but those of Fig. 3F are displaced to the right. Because the contours have similar shapes, the allometric exponents end up the same; because those of Fig. 3F are displaced to the right, the resultant optimal strategy has a lower normalization constant (Y₀). (iii) The normalization constant is sensitive to both the allocation tradeoff and the position of the fitness contours (compare Fig. 3 C and D). If the tradeoff is invariant, the larger species necessarily have lower normalization constants than smaller ones (Supporting Text). In sum, the shapes of the fitness contours determine the allometric exponent, whereas the normalization constant (Y₀) is determined jointly by the position of the contours and the allocation tradeoff to ornament vs. body. Thus, the allometric exponents alone reflect the relative fitness advantages of ornaments vs. somatic growth for the fitness functions specified by Eq. 4. Turning the argument around, because the allometric exponents are similar for the different taxonomic groups, the fitness advantages of investing in ornaments should also be similar. The suggestion is that in all of the diverse taxa considered here, similar mating advantages are obtained by ornaments of similar cost, in terms of somatic growth, irrespective of the type of the ornament.

The model incorporates, explicitly or implicitly, several important features of biology. First, ornaments and weapons are not cheap. The costs of allocating resources to these traits and to the muscles and other structures required to display the ornaments or wield the weapons are substantial. These costs trade off to limit growth in overall body size. These resource costs and the associated fitness consequences keep the sexually selected structures honest, so they are not subject to cheating (e.g., refs. 14–16). Indeed, comparison of Fig. 3 C and D shows how it is possible to have steep positive allometries, even when only a small fraction of resources is allocated to the ornament. Second, by definition, exaggerated structures exhibit positive allometries. In terms of the model, this exaggeration means that z increases exponentially with x, and relative ornament or weapon size increases exponetially with increasing body size and resources. Note that isometry results when z remains constant as body size increases throughout ontogeny. Third, the exponential scaling of ornament size produces a much more effective signal than if the scaling were isometric. Sensory systems are typically tuned on exponential scales (ref. 28; for example, loudness of sound is measured in decibels, a log₁₀ scale). So, the exponential scale of the male's ornament signal tends to match the exponential scale of the female's sensory response. Fourth, the previous points must be true, even though the size of the ornament or weapon itself represents only a part of the cost of allocating resources to such a structure. Additional anatomical and physiological attributes, not included in the measurement of the ornament or weapon, are required to use the structure in intersexual display or intrasexual combat. These muscles and other traits invariably contribute to the apparent increase in overall body size, so they are often incorporated implicitly in the measurement of the body size variable. So, the z dimensions of the tradeoff triangles underestimate the full cost of allocating to the ornament; additional costs are often hidden in the x dimensions. Fifth, for the above reasons, the positive allometries provide clear examples of condition-dependent, sexually selected traits. Males with more resources produce both absolutely and relatively larger ornaments. Therefore, large ornaments provide an easily detected signal of male condition. Sixth, the model specifies how sexual selection for optimal resource allocation to ornaments affects both the allometric exponent, b, and the normalization constant, Y₀, in Eq. 1. Factors that affect Y₀ cause the intraspecific exponents to be consistently greater than interspecific ones. So, the above fundamental features of allocation tradeoffs and fitness payoffs not only explain positive allometries of ornaments and weapons during the ontogenetic growth of an individual, they also explain the scaling relationships between individuals of different body sizes, both within and between populations of closely related organisms.

To paraphrase Gould (6), the positive allometries of horns and antlers are indeed one of the most pervasive regularities of biological form and function. After several decades of empirical and theoretical investigation, however, these positive allometries are no longer poorly understood. They are the inevitable result of differential allocation to structures that enhance mating success.