Group interest versus self-interest in smallpox vaccination policy

Abstract

The recent threat of bioterrorism has fueled debate on smallpox vaccination policy for the United States. Certain policy proposals call for voluntary mass vaccination; however, if individuals decide whether to vaccinate according to self-interest, the level of herd immunity achieved may differ from what is best for the population as a whole. We present a synthesis of game theory and epidemic modeling that formalizes this conflict between self-interest and group interest and shows that voluntary vaccination is unlikely to reach the group-optimal level. This shortfall results in a substantial increase in expected mortality after an attack.

Smallpox has reemerged recently as a public health issue. Because of the lack of information on smallpox transmission in contemporary populations, mathematical modeling has an especially important role to play in policy development. Suggestions for vaccination policy have ranged from preemptive mass vaccination (1) to post-outbreak ring vaccination (2). The policy of the current Bush administration calls for mandatory vaccination of the armed forces, voluntary vaccination of up to 10 million “first responders” thereafter, and some possibility of voluntary vaccination for the general public (3).

The level of vaccine coverage that is best for the population as a whole (the “group optimum”) can be determined by minimizing a cost function C(p) that expresses the impact on public health (from vaccination and/or bioterrorism) if a proportion p of the population is vaccinated preemptively. In contrast, under a voluntary policy, the coverage level will depend on a number of factors that influence individual behavior including self-interest, religious beliefs, and altruism. If acting according to pure self-interest, individuals would attempt to minimize their risks by weighing the risks of vaccine complications against the risks of bioterrorism. The preferred choice of an individual depends on the choices made by the rest of the population. In the scenario where individuals are motivated by self-interest, we refer to the level achieved under a voluntary program as the “individual equilibrium.”

If there is a significant difference between the group optimum and individual equilibrium, then a policy dilemma results: On the one hand, voluntary vaccination does not protect the population as well as it should; on the other hand, imposing mandatory vaccination to group-optimal levels arguably violates civil rights. This potential dilemma motivates our investigation.

The individual equilibrium can be determined by using game theory, which predicts behavior in situations where the payoff to a strategy depends on the strategy chosen by others. Game theory was first applied to economics and international relations (4) and later to evolution (5). In the context of vaccination, a game-theoretical analysis should be able to predict the expected level of vaccine uptake in a population where individuals act to maximize their payoff (i.e., minimize their probability of death due to smallpox or vaccination).

Intuitively, high levels of voluntary vaccination may be difficult to maintain because an individual has little to gain from being vaccinated if everyone else is already immune. This situation is similar to the classical “prisoner's dilemma” in which cooperative behavior is not a stable strategy (6, 7). However, the “vaccination game” has the added dimension of epidemiological dynamics, which interplay with the self-/group-interest conflict in a potentially complex way. Even with complete knowledge of risks, the level of vaccine uptake that will result from this interaction is not obvious. In this article, we combine epidemic modeling with game theory to explore what differences, if any, exist between individual equilibria and group optima in smallpox vaccination policy.

The Individual Equilibrium

Our vaccination game is a population game (5) or nonatomic game (8), meaning that the payoff to an individual choosing a particular strategy depends on the average behavior of the population. The two basic strategies are “vaccinator” (obtain preemptive vaccination) and “delayer” (decline preemptive vaccination but seek vaccination in the event of an attack). For any strategy, the payoff to an individual is measured in terms of a cost function for the risks of death due to vaccination and/or smallpox bioterrorism.

To solve the game, we seek a Nash equilibrium strategy. In a population where all individuals play such a strategy, it is impossible for a few individuals to increase their payoffs by switching to a different strategy. Vaccinator cannot be a Nash equilibrium for the reason indicated in the introduction (an individual who chooses the delayer strategy when population coverage is at 100% reaps the benefits of high population immunity without suffering the risk of vaccine complications). By comparison, delayer can be a Nash equilibrium under certain conditions, such as when the attack risk is sufficiently low or the risk of death due to vaccination is sufficiently high. In other situations, it might be best for some individuals to be vaccinated preemptively and for others to delay. To allow for this we consider mixed strategies whereby individuals choose the vaccinator strategy with probability P (0 < P < 1) and the delayer strategy otherwise. If all individuals play the mixed strategy P, then a proportion p = P of the population is preemptively vaccinated.

The payoff E_vac to an individual choosing the vaccinator strategy is

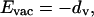1

where d_v is the probability of death from vaccination. We take vaccine efficacy to be 100% [the efficacy of a course of repeated vaccination is nearly perfect (1)].

The effective level of preemptive vaccination will be slightly less than p (the proportion actually vaccinated) due to the death of some vaccinated individuals. Among survivors, the proportion vaccinated is

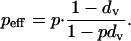2

However, because d_v is of order 10^-6, this effect is negligible, and we ignore it henceforth. With this approximation, the payoff E_del(p) to an individual choosing the delayer strategy is

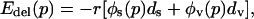3

where r is the risk of attack, ø_s(p) is the probability that a delayer becomes infected with smallpox after an attack, d_s is the probability of death due to a smallpox infection, and ø_v(p) is the probability that a delayer is vaccinated successfully after an attack (if d_v were not small, then we would need to replace p by p_eff on the right-hand side of Eq. 3). We assume that postattack mass vaccination continues until all susceptibles have been vaccinated [a realistic assumption because full coverage can be accomplished within a single generation time of the infection (1)], and hence ø_v(p) = 1 - ø_s(p). Note that ø_s(p) must be a strictly decreasing function of p.

If E_vac > E_del(0), there is a unique Nash equilibrium p_ind (0 < p_ind < 1) that can be found by solving for p_ind in the equation

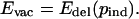4

If E_vac ≤ E_del(0), then the pure delayer strategy (p_ind = 0) is the unique Nash equilibrium (see Existence and Uniqueness of Nash Equilibrium in Appendix, which is published as supporting information on the PNAS web site, www.pnas.org).

The individual equilibrium p_ind predicted by this game-theoretical analysis corresponds to the level of coverage p_ind expected under a voluntary program where individuals act in a rational way to maximize their probability of survival.

The Group Optimum

From the perspective of group interest, we wish to minimize the total number of deaths expected from both vaccination and smallpox infection. If p is the proportion of the population that is preemptively vaccinated, we can express the expected cost C(p) due to vaccination and a potential smallpox epidemic as

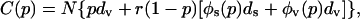5

where N is the population size and the other symbols have the same meaning as in Eq. 3. Because N is merely a scale factor (and therefore can be ignored for the purpose of minimization) and ø_v(p) = 1 - ø_s(p), the cost can be written as

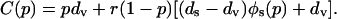6

We now minimize C(p) on the unit interval (0 ≤ p ≤ 1) to determine the group optimum p_gr, which is the coverage level that would have to be imposed to minimize the total expected number of deaths.

SEIDV Epidemic Model

Both the individual equilibrium p_ind and the group optimum p_gr depend on the function ø_s(p), which gives the probability of death from smallpox for any given level of preemptive vaccination. To determine the function ø_s(p), an epidemiological model is required. We developed a compartmental epidemic model in which compartments reflect infection status and the rate of emergence of new cases is proportional to the product of the densities of susceptible and infectious individuals. Such models have been applied to a wide range of infectious diseases (9) including smallpox (1). Our model tracks the time evolution of the densities of individuals who are susceptible (S), infected but not yet infectious (E), infectious (I), removed (dead/immune) due to smallpox infection (D), and removed (dead/immune) due to vaccination (V) (the SEIDV model). The model equations are

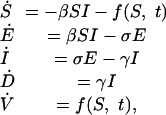7

where β is the mean transmission rate, 1/σ is the mean latent period, and 1/γ is the mean infectious period (here defined as the time between the onset of infectiousness and the isolation of the symptomatic patient). The effect of postattack intervention by health authorities is expressed through the function f(S, t), which is the rate of mass vaccination of susceptible individuals. We assume that mass vaccination is initiated t_res days after the initial smallpox exposure (the “response time”) and that susceptible individuals are vaccinated at a constant rate v until all have been vaccinated; hence

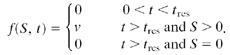8

To estimate the initial attack size we assume that the attack takes the form of aerosolized dispersal in, for instance, a building or airport (10). Therefore the initial number α of individuals exposed to the virus is large, but a proportion p are immune due to preemptive vaccination. As a result, the initial number of infected individuals is (1 - p)α.

Results

The key quantities for assessing policy are the difference in the proportion of the population preemptively vaccinated at the individual equilibrium versus the group optimum,

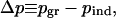9

and the corresponding relative difference in expected mortality,

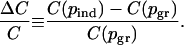10

All parameter values used in the analysis of our model are listed in Table 1. For the parameter values in the third column of that table, we found that the individual equilibrium is p_ind = 0.19 (corresponding to a situation where 19% of the population opts for preemptive vaccination). This falls well below the group optimum p_gr = 0.47. The corresponding relative increase in mortality ΔC/C was 0.54, i.e., 54% more deaths under a voluntary vaccination policy.

Table 1. Parameter values, intervals used in sensitivity analysis, and source references.

Parameter	Definition	Estimated value	Range for sensitivity analysis	Ref.
Ro	Basic reproductive ratio	5	[3.5, 6]	16
1/σ	Mean latent period	11 days	—	17
1/γ	Mean infectious period	3 days	—	17
tres	Vaccinator response time	14 days	[7, 21]	n/a
v	Vaccination rate	0.10 day−1	[0.05, 0.15]	1
dv	Probability of death from vaccine	10−6	—	17
ds	Probability of death from smallpox	0.3	—	17
r	Attack risk (probability)	0.01	[0.001, 0.05]	n/a
α	Attack size (no. of individuals)	5,000	[100, 20,000]	10

The basic reproductive ratio R_o is the number of secondary infections produced by a typical infected individual in a wholly susceptible population; it determines the mean transmission rate β via the relation β ≃ γR_o (9).

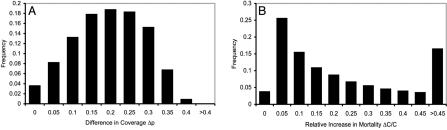
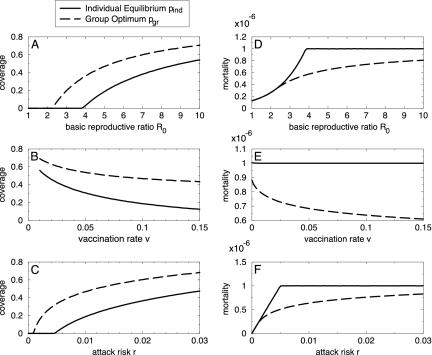
To determine the sensitivity of this result to parameter values we carried out a Monte Carlo sensitivity analysis by randomly selecting values from intervals with realistic bounds (Table 1, fourth column, and Fig. 1). We observed that both p_ind and p_gr usually fall strictly between 0 and 1, and that p_ind ≤ p_gr always (the individual equilibrium never exceeds the group optimum). Furthermore, the difference in the proportion preemptively vaccinated, Δp, was typically large, having an average value of 0.17 across all 10⁵ realizations. The average values of p_ind and p_gr were 0.48 and 0.65, respectively (the variance was substantial in both cases). The ratio of the difference Δp to the group optimum p_gr averaged over all realizations was 35% (this is the expected “error” of the individual equilibrium relative to the group optimum). The relative increase in mortality at the individual equilibrium, ΔC/C, averaged over all realizations was 22%. Both the group optimum and individual equilibrium respond in qualitatively similar ways as the basic reproductive ratio R₀ (see Table 1 legend), postattack vaccination rate v, and attack risk r vary (Fig. 2); p_ind and p_gr exhibit thresholds below which vaccination is too risky and remains at zero.

Fig. 1.

Sensitivity analysis histograms for the difference in vaccine coverage, Δp = p_gr - p_ind (A), and the relative increase in mortality at the individual equilibrium, ΔC/C = [C(p_ind) - C(p_gr)]/C(p_gr)(B). This analysis shows that, for a wide range of parameter values, self-interested decision making can cause coverage levels to fall short of the group-optimal levels, resulting in a significant increase in expected mortality. We analyzed 10⁵ realizations of the model by randomly selecting parameter values from the ranges listed in Table 1. All horizontal axis labels except “0” give upper limits of corresponding bins; lower limits are given by the left adjacent label. The bin corresponding to the “0” label contains outcomes that were precisely zero.

Fig. 2.

Vaccination coverage levels (solid line, individual equilibrium p_ind; dashed line, group optimum p_gr) at various values of basic reproductive ratio R₀ (A), postattack vaccination rate v (B), and attack risk r (C). The dependence of p_ind and p_gr on model parameters is qualitatively similar but quantitatively very different. (D-F) How the expected mortality C(p) varies with model parameters at both the individual equilibrium [C(p_ind)] and the group optimum [C(p_gr)]. Other parameter values were set to the estimated values in Table 1. Because the payoffs for delayer and vaccinator are equal at the mixed Nash equilibrium [E_del(p_ind) = E_vac = -d_v, Eqs. 1 and 4] and C(p) = -pE_vac - (1 - p)E_del(p) (Eq. 5), it follows that C(p_ind) = d_v whenever the Nash equilibrium p_ind is a mixed strategy, which explains why C(p_ind) = 10^-6 over wide parameter ranges in D-F.

We have assumed thus far that there is no residual immunity from the preeradication era; however, the inclusion of residual immunity has little effect on the results (see Fig. 3, which is published as supporting information on the PNAS web site, and Impact of Inclusion of Residual Immunity in Appendix). The impact of vaccine-induced morbidity (such as vaccinia) arising from vaccination can also be incorporated, although a weighting then must be chosen to express the cost of vaccine-induced morbidity relative to fatal complications. If vaccine-induced morbidity is incorporated, the results are again qualitatively unchanged (Fig. 4, which is published as supporting information on the PNAS web site, and Impact of Inclusion of Vaccine-Induced Morbidity in Cost Function in Appendix). Additionally, we carried out a sensitivity analysis with respect to the postattack vaccination rate v and the response time t_res, the two model parameters most readily controlled by the public health authorities (Fig. 5, which is published as supporting information on the PNAS web site, and Sensitivity Analysis for Postattack Vaccination Rate v and Response Time t_res in Appendix). This analysis confirmed that Δp and ΔC/C remain substantial across most of the two-dimensional (v, t_res) parameter space.

Discussion

Our results illustrate how the conflict between group interest and self-interest typically causes large differences between vaccine coverage levels that are best for the group and uptake levels that might actually be achieved under a voluntary vaccination program. Public health education should not ignore appeals to altruism, because, all else being equal, self-interested decision making could lead to seriously suboptimal vaccine uptake levels. More generally, our analysis highlights the potential value of game theory in the development and evaluation of public health policy.

Polls suggest that ≈60% of Americans would choose preemptive smallpox vaccination (11, 12), yet the proportion p_ind predicted by our model is typically <0.60. This apparent discrepancy may be caused by a number of factors. For example, individuals may have an inflated sense of attack risk (r), attack size (α), the rapidity with which a smallpox outbreak would spread (β) relative to the ability of public health authorities to stop the epidemic (v), or a distorted impression of the relationships among transmission potential, interventions, and the probability of being infected. However, building on the current results by using polls specifically designed to test game-theoretical predictions and adapting the models accordingly would help remedy this. For example, for the parameters in Table 1, a value of p_ind = 0.60 is produced by an attack risk r of 4.7%. This result could be compared with polls investigating the public perception of risk.

History details numerous examples of vaccine refusal (13-15). Some of these examples embody the prisoner's dilemma effect. The grounds for refusal vary widely but often are related to perceived risks of vaccination. In many cases, because of the success of the vaccination program itself, certain diseases are rarely seen, and hence individuals tend not to vaccinate because of a low perceived risk. A situation then can be created in which media coverage of potential vaccine risks may cause a scare that drags uptake below critical levels, making large outbreaks possible (14). A notable example was the pertussis vaccine scare of the 1970s in Britain, where a sustained drop in coverage preceded relatively large pertussis outbreaks. Such historical examples, together with the predictions of our game-theoretical model, suggest that persistently high levels of immunization will be difficult to maintain in countries with voluntary vaccination policies.