Calcium-activated nonspecific cation current and synaptic depression promote network-dependent burst oscillations

Abstract

Central pattern generators (CPGs) produce neural-motor rhythms that often depend on specialized cellular or synaptic properties such as pacemaker neurons or alternating phases of synaptic inhibition. Motivated by experimental evidence suggesting that activity in the mammalian respiratory CPG, the preBötzinger complex, does not require either of these components, we present and analyze a mathematical model demonstrating an unconventional mechanism of rhythm generation in which glutamatergic synapses and the short-term depression of excitatory transmission play key rhythmogenic roles. Recurrent synaptic excitation triggers postsynaptic Ca²⁺-activated nonspecific cation current (I_CAN) to initiate a network-wide burst. Robust depolarization due to I_CAN also causes voltage-dependent spike inactivation, which diminishes recurrent excitation and thus attenuates postsynaptic Ca²⁺ accumulation. Consequently, activity-dependent outward currents—produced by Na/K ATPase pumps or other ionic mechanisms—can terminate the burst and cause a transient quiescent state in the network. The recovery of sporadic spiking activity rekindles excitatory interactions and initiates a new cycle. Because synaptic inputs gate postsynaptic burst-generating conductances, this rhythm-generating mechanism represents a new paradigm that can be dubbed a ‘group pacemaker’ in which the basic rhythmogenic unit encompasses a fully interdependent ensemble of synaptic and intrinsic components. This conceptual framework should be considered as an alternative to traditional models when analyzing CPGs for which mechanistic details have not yet been elucidated.

Central pattern generator networks produce neural rhythms that drive motor behaviors (1, 2). Many CPGs function in reduced preparations in vitro, which facilitates studies at the cellular and synaptic levels. Building blocks such as bursting-pacemaker neurons and reciprocal synaptic inhibition often form the core of the rhythmogenic mechanism (3). However, the CPG for inspiratory breathing movements in mammals, the preBötzinger Complex (preBötC) (4, 5), exhibits rhythmicity after removal of synaptic inhibition (6, 7) and after blockade of bursting-pacemaker activity in individual neurons (8, 9).

A group pacemaker has been proposed as the building block of an alternative paradigm for respiratory rhythmogenesis (10). The group pacemaker concept refers to a network that employs recurrent synaptic excitation to boost and spread activity like a conventional network oscillator (3), yet generates bursting oscillations in which cells show a plateau depolarization—or drive potential—during the active phase, as typically associated with intrinsic pacemaker properties. Until recently, the group pacemaker model has remained purely heuristic and hypothetical, without an explicit mathematical instantiation or mechanistic connection to experimental findings.

We tested the viability of an experimentally motivated group pacemaker model, where neurons express a Ca²⁺-activated nonspecific monovalent cation current (I_CAN) with its activation linked to group I metabotropic glutamate receptors (mGluRs) and AMPA receptors that, together, increase intracellular Ca²⁺ (11). I_CAN is normally latent, but this synaptically triggered Ca²⁺ flux evokes I_CAN, which underlies the burst phase of network activity (12, 13). I_CAN may be well suited to play a central role in a group pacemaker because it contributes to plateau depolarizations in diverse contexts such as spinal locomotor networks (14), autonomic motor systems (15), subthalamic neurons (16), and the entorhinal cortex (17), among others.

Our model shows how recurrent excitation and I_CAN generate a burst and, further, predicts that I_CAN hastens burst termination by causing voltage-dependent spike inactivation. The attenuation of spikes diminishes recurrent excitation, yielding a form of synaptic depression that deactivates I_CAN. This deactivation in turn allows activity-dependent outward currents to interrupt spiking and establish a quiescent phase. The eventual resumption of spontaneous spiking restarts recurrent positive feedback and initiates a new cycle, leading to systematic burst oscillations of the network.

With synaptic signals gating burst-generating ion channels in postsynaptic neurons, the group pacemaker model provides a new paradigm for CPG activity in which the rhythmogenic unit is the ensemble of coupled synaptic and intrinsic membrane properties. Physiological studies of respiratory rhythmogenesis guided the model formulation, yet it incorporates general elements that are found across a variety of brain areas. CPGs are ubiquitous throughout the animal kingdom and the rhythmogenic mechanisms are often incompletely understood. Given its relevance to the preBötC and the tendency for successful mechanisms to be harnessed across brain regions and among diverse species, the group pacemaker model presents a viable alternative to bursting-pacemaker neurons, reciprocal inhibition, or conventional network oscillations as the basis for network-level rhythmogenesis.

Results

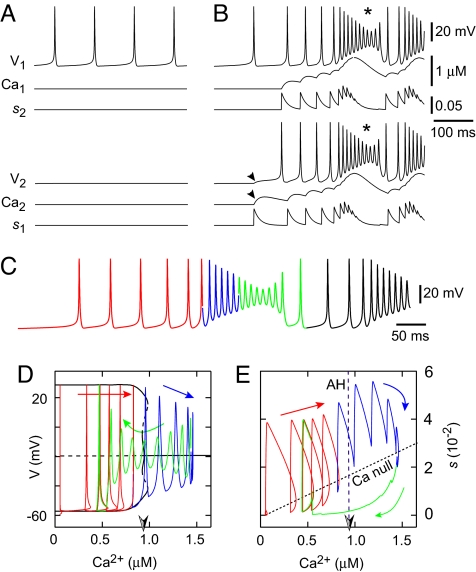
Model neurons with Hodgkin-Huxley-style spike-generating and leak currents, and I_CAN, were mutually coupled via ionotropic AMPA receptors and group I mGluRs, which elevated intracellular Ca²⁺ to activate I_CAN (Fig. S1). We set intrinsic properties such that, in the absence of coupling, one neuron (N₂) was quiescent and the other (N₁) spiked tonically (Fig. 1A). Nevertheless, both neurons had low Ca²⁺ concentrations in the absence of coupling (Ca₁ and Ca₂ ≈ 50 nM).

Fig. 1.

Group pacemaker models. (A and B) Uncoupled (A) and coupled (B) model neurons, showing voltage (V), Ca²⁺, and synaptic dynamics (s). Neuron 1 (N₁, top) is initially tonic (A) and Neuron 2 (N₂, bottom) is initially quiescent (A), before coupling (B). (C) Self-coupled single neuron with dynamics that resemble the 2-neuron case. Color coding applies to phase-plane analyses in D and E. (D) V-Ca²⁺ bifurcation diagram (black) with solution trajectory. Solid (dashed) black curves denote stable (unstable) structures, which are either nodes or the maximal and minimal voltages along families of periodic orbits. These include a curve of steady states at approximately −20 mV, which switches from unstable to stable in a supercritical Andronov-Hopf bifurcation (AH, half-shaded arrowhead; (Ca²⁺)_AH in text) as Ca²⁺ increases, and 3 families of periodic orbits, a stable one that emerges from the AH bifurcation, an unstable family that emerges from the first, and a second stable family of larger amplitude (in maximal and minimal voltage) that emerges from the unstable periodic orbits. Red, blue, and green portions correspond to the voltage trace in C. Voltage spikes follow the large amplitude periodic orbits. Spike attenuation begins when Ca²⁺ becomes so large that this family of periodic orbits no longer exists. Because this occurs very close to (Ca²⁺)_AH, we approximate the point where spike attenuation begins by (Ca²⁺)_AH for simplicity (see text). (E) The s-Ca²⁺ phase plane showing the curve of AH points (the half-shaded arrowhead denotes (Ca²⁺)_AH), and the Ca²⁺ nullcline (Ca null), with the solution trajectory in red, blue, and green.

A synaptic connection enabled N₁ to excite N₂. Spikes in N₁ that initially peaked at 30 mV crossed the synaptic threshold θ_s and incremented the synaptic variable s₁. The increase in s₁ depolarized N₂ via AMPA receptors and caused Ca₂ to increase (Fig. 1B, arrowheads). The rise in Ca₂ evoked I_CAN and N₂ commenced spiking. Recruiting the quiescent cell boosted the synaptic variable s₂, which depolarized N₁,elevated Ca₁, and activated I_CAN in N₁. The positive feedback loop of Ca²⁺ elevation and I_CAN recruitment produced mutual depolarization of ≈30 mV with a superimposed burst of spiking at ≈100 Hz, even though neither neuron produced bursts or high frequency spiking in the absence of coupling.

After a period of rapid spiking, depolarization block subsequently reduced spike amplitudes (Fig. 1B, asterisks). Because V₁ and V₂ failed to reach θ_s, s₁ and s₂ stopped growing and intracellular Ca²⁺ stopped accumulating. Extrusion and sequestration then dominated the Ca²⁺ dynamics and Ca₁ and Ca₂ declined, deactivating I_CAN. After this decline and associated relief of depolarization block, the cells resumed tonic spiking, which quickly ramped up in frequency through reciprocal synaptic excitation, such that the cycle repeated with no significant pauses between bursts (Fig. 1B).

We analyzed how Ca²⁺ and synaptic dynamics govern bursting behavior in the model by computing a bifurcation diagram for a single cell that was tonically active in isolation but, when self-coupled, displayed burst-like oscillations (Fig. 1C) that resemble the 2-cell case (Fig. 1B). We determined the voltage and synaptic dynamics for fixed Ca²⁺ within a range of physiological values, because the rate of change of Ca²⁺ is small compared with voltage and s. The resulting diagram shows that for each sufficiently low level of Ca²⁺, the stable feature is a periodic orbit, corresponding to large-amplitude spiking. As Ca²⁺ increases to a critical value (Ca²⁺)_AH, a supercritical Andronov-Hopf (AH) bifurcation occurs, beyond which the only stable state corresponds to depolarization block (Fig. 1D).

Fig. 1D superimposes the voltage and Ca²⁺ components of the solution trajectory onto the bifurcation diagram. Because the periodic orbit amplitudes remain relatively constant (red) until very close to the AH bifurcation, spike attenuation does not begin until Ca²⁺ reaches (Ca²⁺)_AH (red-blue). Spike amplitudes do not decrease rapidly when Ca²⁺ exceeds (Ca²⁺)_AH (blue-green) because the depolarization block steady state is weakly attracting, i.e., linearization about this stable state yields eigenvalues with negative real parts of small magnitude.

The curve in state space at which the rate of change of Ca²⁺ switches signs is called the Ca²⁺ nullcline and is naturally visualized in the s-Ca²⁺ phase plane because Ca²⁺ changes are synaptically driven (11). During spiking, s and Ca²⁺ increase (Fig. 1E, red). Ca²⁺ eventually exceeds (Ca²⁺)_AH, and spikes diminish (blue). Voltage is not visible in the s-Ca²⁺ plane, but because Fig. 1 D and E share the same Ca²⁺ axis, the voltage in Fig. 1E can be deduced from Fig. 1D based on whether Ca²⁺ is increasing or decreasing along the bifurcation diagram. The Ca²⁺ nullcline is crossed in the downward direction when attenuated spikes no longer boost s. Intracellular Ca²⁺ levels drop (Fig. 1E, green) until Ca²⁺ recrosses (Ca²⁺)_AH and spikes grow to approach the stable large amplitude orbits present for Ca²⁺ < (Ca²⁺)_AH. The reemergence of full-amplitude spikes drives increases in s, causing the Ca²⁺ nullcline to be crossed in the upward direction and allowing Ca²⁺ to increase again. The resulting Ca²⁺ elevation reactivates I_CAN and the burst cycle repeats (Fig. 1 D and E, green-red).

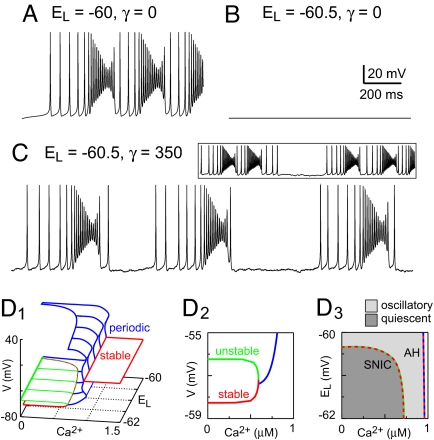
The mechanism described above leads to periodic waxing and waning of spike activity but does not produce extended quiescent periods between bursts (Fig. 2A). Indeed, the branch of periodic orbits born at (Ca²⁺)_AH enables repetitive overshooting spikes that do not depend on I_CAN and persist for all Ca²⁺ < (Ca²⁺)_AH (Fig. 1D). Periods of quiescence that are characteristic of CPG rhythms require the existence of a branch of stable resting states in the bifurcation diagram. Lowering E_L below −60 mV (Fig. 2B) enhanced the K⁺-dominated leak current (I_Leak) and created a branch of stable nodes, at resting potentials akin to V₂ in Fig. 1A, and a branch of saddle points seen at Ca²⁺ < (Ca²⁺)_AH in the V-Ca²⁺ bifurcation diagram (Fig. 2D). Because I_Leak is not dynamic, once the voltage settled onto the stable branch it could not leave, regardless of the strength of self-coupling (Fig. 2B). Adding Gaussian noise to the current-balance equation (see SI Appendix) enabled the system to move spontaneously between the active and quiescent phases. Noise-driven spikes randomly occurring in succession could trigger bursts (Fig. 2C), which evolved with the same dynamics as Fig. 1C. However, with lower E_L and noise fluctuations, the system could spontaneously jump back to the quiescent state when Ca²⁺ was near its nadir, terminating the burst. The dependence of burst termination on noise fluctuations also allowed for back-to-back bursts (Fig. 2C Inset) before burst termination and yielded irregular burst sequences that changed phase erratically, lacking the regularity of CPG-driven rhythmic behaviors.

Fig. 2.

Noise-induced burst oscillations in the self-coupled model. (A) Oscillations and (B) quiescence depend on E_L with no added noise. (C) The addition of membrane noise (γ = 350 pA²) allows irregular bursting for low E_L. (Inset) Back-to-back bursts in this regime. (D₁) Three-dimensional (3D) bifurcation diagram with Ca²⁺ and E_L as bifurcation parameters. The red and green surfaces consist of stable nodes and saddle points, respectively; the blue surface is formed from the maximum and minimum voltages along families of periodic orbits. (D₂) V-Ca²⁺ slice of the 3D graph in D₁ with E_L = −60.5 mV showing the lower stable node branch, the branch of saddle points (which reflect spike threshold), and the minimum voltage from the family of periodic orbits that coalesce in a SNIC bifurcation. (D₃) E_L-Ca²⁺ plane showing the curve of SNIC bifurcation points and the curve of Andronov-Hopf (AH) bifurcation points from which the periodic orbit families emanate, extracted from the 3D graph in D₁. Dark and light gray regions correspond, respectively, to quiescent and oscillatory solutions in the self-coupled model with Ca²⁺ held constant.

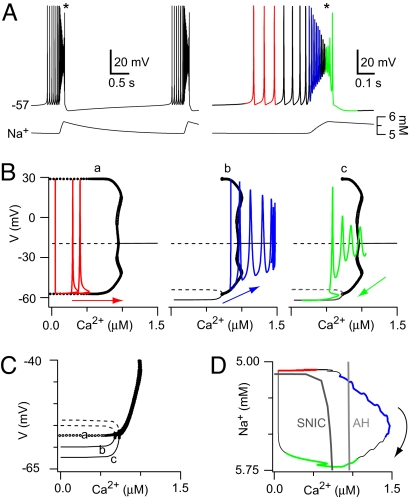
To engender a rhythm with regular quiescent interburst intervals, we added an outward current, I_pump, due to electrogenic Na/K ATPase pumps (Fig. S1) that activate in response to Na⁺ accumulation during the I_CAN-dominated inspiratory burst (18–21). With low initial Na⁺ levels and I_pump deactivated, the self-coupled cell spiked at a low frequency (Fig. 3, red). As shown previously, recurrent excitation and I_CAN activation transformed this activity into a high frequency burst of spikes. Ca²⁺ continued to increase and the trajectory moved rightward in the V-Ca²⁺ bifurcation diagram, yielding a phase of spike attenuation as Ca²⁺ exceeded (Ca²⁺)_AH. These burst dynamics, shown in Fig. 3B, appear similar to Fig. 1D. However, with sufficient Na⁺ accumulation and corresponding I_pump activation, the family of periodic orbits born at (Ca²⁺)_AH terminated at a level of Ca²⁺ below (Ca²⁺)_AH, where the periodic family coalesced with a new branch of stable nodes near −60 mV (Fig. 3Bb). The emergence of these stable nodes and the corresponding unstable saddle points is illustrated in Fig. 3C, which superimposes the V-Ca²⁺ bifurcation structures from Fig. 3B. The periodic family in the low Na⁺ case (curve a) does not terminate at any Ca²⁺ < (Ca²⁺)_AH. However, because Na⁺ accumulates and I_pump activates, the stable branch appears and expands to occupy a greater proportion of the Ca²⁺ space (curves b and c). This I_pump-related branch is analogous to that created by lowering E_L in Fig. 2D. The periodic orbits and stable nodes meet at a saddle-node on an invariant circle (SNIC) bifurcation in the V-Ca²⁺ diagram, and we define the corresponding Ca²⁺ level as (Ca²⁺)_SNIC (Figs. 2D₃ and 3D).

Fig. 3.

Self-coupled model with I_pump. (A) Voltage and Na⁺ traces (Left) with an expanded and color-coded burst (Right). (B) V-Ca²⁺ bifurcation diagrams for fixed Na⁺ values; red, blue, and green trajectories correspond to A. Stable (unstable) points are drawn with solid (dashed) lines, and periodic orbits (maximal and minimal voltages) are shown with circles. The branch near −20 mV is a curve of spiral points, featuring a change in stability at the AH bifurcation from which the periodic orbits emanate. The branches at lower voltages that appear in the Center (b) and Right (c) parts of B, consist of unstable saddles (dashed lines) and stable nodes (solid lines), which intersect at a SNIC bifurcation. (C) Expanded view near the SNIC; a, b, and c refer to Left (a), Center (b), and Right (c) in B, superimposed without trajectories. (D) The burst cycle in Na⁺-Ca²⁺ state space, together with the SNIC and AH curves. Na⁺ is plotted such that lower values on the y axis reflect lower excitability, due to higher Na⁺ evoking greater I_pump (outward) current.

When the stable branch first appeared, it was not visited by the trajectory (Fig. 3Bb). Depolarization block and subsequent synaptic depression led to a drop in Ca²⁺ and changed the direction of the trajectory along the Ca²⁺ axis (Fig. 3Bc). Even as Ca²⁺ declined from its peak value (1.5 μM), Ca²⁺ levels were high enough to maintain sufficient I_CAN activation so that Na⁺ continued accumulating. Therefore, I_pump continued to strengthen, expanding the stable branch of quiescent states until the trajectory, moving leftward relative to the V-Ca²⁺ bifurcation diagram, crossed the SNIC bifurcation and the quiescent state became globally attracting, yielding burst termination (Fig. 3Bc).

The quiescent state persisted until Na⁺ extrusion deactivated I_pump, causing the stable branch to vanish via another SNIC and the family of periodic orbits to once again become globally attracting (a return to Fig. 3Ba). The resulting return to low rate spiking initiated a new burst cycle. The rhythm with I_pump was robust to variations in critical parameters (Fig. S2).

The Ca²⁺ and Na⁺ dynamics during the burst cycle can be examined in the Ca²⁺-Na⁺ plane. For each fixed Na⁺ greater than ≈5.1 mM, the branch of quiescent states extends over a range of Ca²⁺, terminating when Ca²⁺ reaches an upper bound defined by the vertical portion of the SNIC bifurcation curve (Fig. 3D). During the burst, Na⁺ levels exceed 5.1 mM, and thus the quiescent state exists for low Ca²⁺. However, the burst terminates only when the Ca²⁺ decrease that follows depolarization block causes the trajectory to cross the SNIC curve (Fig. 3D, green). Likewise, burst initiation occurs only when a decline in Na⁺ pushes the trajectory back across the horizontal portion of the SNIC curve, out of the region in which the quiescent states exist (Fig. 3D, black-red). Within the burst the trajectory crosses the AH bifurcation curve twice, signifying the start and end of spike attenuation (Fig. 3D, blue-green). The AH curve is vertical in the Ca²⁺-Na⁺ plane because the Na⁺ accumulated from I_CAN does not influence voltage-dependent spike attenuation, although the fast sodium current I_Na may participate.

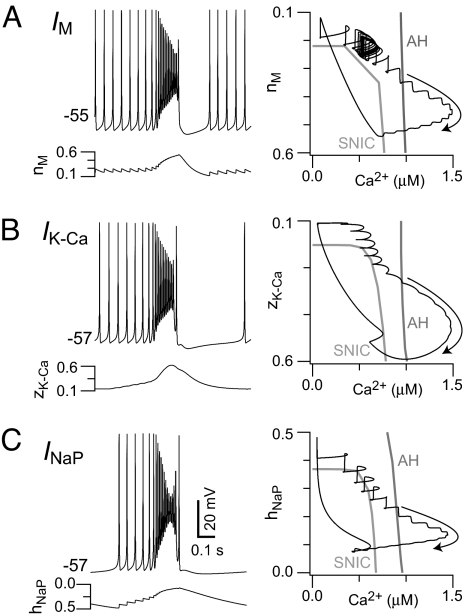
Fig. 3 shows that to prevent repetitive bursts, outward current must create a branch of stable nodes during the burst. Stable nodes capture the trajectory after synaptic depression decreases the Ca²⁺, providing a period of quiescence. We posited that other activity-dependent net outward currents could replace I_pump and subserve this role. We examined 3 candidates that represent different classes of ion channels: a slowly-activating voltage-dependent M-like K⁺ current (I_M, Fig. 4A and Fig. S3B), a Ca²⁺-dependent K⁺ current (I_K-Ca, Fig. 4B and Fig. S3C), and a slowly-inactivating persistent Na⁺ current (I_NaP, Fig. 4C and Fig. S3D). The resulting bursts exhibited similar voltage trajectories regardless of which current we included (Figs. 4 Left and Fig. S3 top traces) and similar flows of Ca²⁺ together with a second slow variable involved in gating the added outward current (Figs. 4 and Fig. S3, blue traces). In all cases, depolarization block and synaptic depression stopped Ca²⁺ influx; these events do not require the activity-dependent net outward current. For I_M, I_K-Ca, and I_NaP, the second slow component played the same role as Na⁺ did for I_pump activation in Fig. 3 and Fig. S3A. Specifically, the gating variables n_M, z_K-Ca, and h_NaP (for I_M, I_K-Ca, and I_NaP) evolved during the burst to evoke net outward currents and thus create a branch of stable quiescent states at low Ca²⁺. In all cases, the trajectory settled onto this stable branch as Ca²⁺ decreased during the burst. The quiescent state disappeared due to deactivation of the outward current (or deinactivation of the inward current I_NaP, Fig. 4C), which restarted recurrent excitation. Although a variety of activity-dependent net outward currents produce quiescent interburst intervals, their gating properties and kinetics contrastingly influence the frequency of the rhythm.

Fig. 4.

Self-coupled model with different outward currents. Voltage (Left) and state-space trajectories with SNIC and AH curves (Right) are shown for M-like K⁺ current (I_M), where n_M is the gating variable in n_M-Ca²⁺ state space (A); Ca²⁺-dependent K⁺ current (I_K-Ca), where z_K-Ca is the gating variable in z_K-Ca-Ca²⁺ state space (B); and persistent Na⁺ current (I_NaP), where h_NaP is the gating variable in h_NaP-Ca²⁺ state space (C). In contrast to A and B, h_NaP values are shown increasing along the y axis (Right) so that all panels on the Right represent more net outward current in the downward direction.

To investigate whether our analysis applied to large networks, we interconnected 200 heterogeneous model neurons. Each neuron had 5 random input connections and 5 random projections to other cells. The full network with I_pump generated a CPG-like rhythm (Fig. S4A). Many neurons discharged sporadically at low rates (5–10 Hz) preceding the active phase because of heterogeneous values of E_L. Recurrent excitation ensured that bursts were globally synchronous, followed by an I_pump-mediated after-hyperpolarization (AHP). The AHP was clearly reflected in the widespread lack of spiking after each burst (Fig. S4A, raster plot), in the voltage trajectory of a typical neuron, and in the average network voltage (Fig. S4A). New cycles began when Na⁺ decayed, I_pump deactivated, and the Ca²⁺ level increased again, accompanied by spontaneous spiking. Therefore, the dynamics of the 200-cell network were analogous to the self-coupled neuron with I_pump in Fig. 3.

Rhythmic bursts also occurred in the 200-neuron network when I_pump was replaced by I_M, I_K-Ca, or I_NaP (Fig. S4 B–D). The resulting full-network rhythm was dynamically equivalent to the paired-cell and single self-coupled neuron simulations displayed in Figs. 3 and 4 and Fig. S3.

Discussion

Mechanism For Burst Oscillations in a Recurrently Connected Excitatory Network.

Here, we demonstrate a viable group pacemaker model, in which a rhythm emerges from an interconnected network of glutamatergic neurons that do not exhibit intrinsic bursting, regardless of applied current levels. Spontaneous spiking engenders positive feedback via recurrent excitation. Glutamate causes postsynaptic depolarization via ionotropic AMPA receptors and acts at group I mGluRs, which collectively increase intracellular Ca²⁺ and evoke I_CAN (11). I_CAN produces the drive potential underlying the burst (12, 13), which is a 10–30 mV envelope of depolarization with concomitant increase in spike rate that reflects burst onset.

Ours is the first CPG model, to our knowledge, that depends on glutamatergic signaling to gate postsynaptic burst-generating ion channels. The intensity of I_CAN in our model causes depolarization block and corresponding spike attenuation (Fig. S5), which has been repeatedly observed in respiratory neurons in vitro (22, 23), in partially intact in situ preparations (24), and in vivo (25). As a potentially rhythmogenic feature, burst-related depolarization block has also been reported in settings as diverse as rat spinal cord oscillations in culture (26) and turtle locomotor rhythms (27). Moreover, because spiking, synaptic transmission, Ca²⁺ influx, and I_CAN activation are directly linked in our model, our analyses lead us to predict that a form of synaptic depression contributes to burst termination in the preBötC.

Bifurcation Mechanisms Underlying Rhythms in a Group Pacemaker.

The rhythm generated by our self-coupled and paired-cell models in the absence of an activity-dependent outward current or lowered E_L (i.e., Figs. 1 and 2A) can be classified mathematically as elliptic bursting (28, 29). Elliptic bursting with depolarization block has been demonstrated in an excitatory self-coupled model (30). In that model, the decay of synaptic excitation after burst termination alleviates depolarization block, allowing spiking to resume and starting the next burst cycle. By contrast, synaptic currents in our model contribute to the onset of depolarization block, but the blocked state can be maintained even if synaptic currents decay to negligible levels; the decay of Ca²⁺ allows spiking to resume.

Incorporating an activity-dependent outward current (I_pump, I_M, I_K-Ca, or I_NaP inactivation) can dramatically change the V-Ca²⁺ bifurcation diagram to include a stable branch of nodes (and a branch of saddle points) at low Ca²⁺, which coalesces with the stable family of periodic orbits in a SNIC bifurcation at (Ca²⁺)_SNIC < (Ca²⁺)_AH (Fig. 3B). The resulting dynamics relies on 2 slow variables (e.g., Na⁺ and Ca²⁺ in Fig. 3) and features 2 crossings of a curve of SNIC bifurcations in the V-Ca²⁺ bifurcation diagram, consistent with parabolic bursting (28, 29, 31). Noise fluctuations could also cause 2 crossings of the SNIC curve with low E_L. In its entirety, the model dynamics is in fact a hybrid of parabolic and elliptic bursting. The active phase begins by passage through a SNIC bifurcation with long initial interspike intervals, as in parabolic bursting, but subsequent I_CAN-mediated depolarization and spike attenuation are characteristic of elliptic bursting. Spike attenuation induces a decay of Ca²⁺ essential for burst termination via the recrossing of the SNIC curve. This more complex activity pattern has not, to our knowledge, been described in any previous model of cell or network burst oscillations.

Burst Initiation in a Group Pacemaker.

We have shown that spontaneous spiking in some constituent neurons is essential to activate I_CAN and initiate bursts. This spiking can come from neurons that are tonically active (Fig. 1A) or via spontaneous fluctuations in silent neurons that spike occasionally when noise is included (Fig. 2C). Tonic neurons and quiescent neurons subject to noise-driven fluctuations provide qualitatively similar kindling for positive feedback in the form of temporal summation of EPSPs that leads to spiking, followed rapidly by I_CAN activation and burst onset. Tonic and noise-driven firing (Fig. S5B), and temporal summation of EPSPs (Fig. S5C), are observed in preBötC neurons. The rate of positive feedback may be influenced by properties not explicitly considered in this model such as K-ATP channels, transient K⁺ currents, or hyperpolarization-activated cationic current (I_h) (see SI Appendix). These factors will impact burst frequency, but not necessarily influence the evolution of the burst as understood via the bifurcation structure in our model.

Burst Termination in a Group Pacemaker.

Because I_CAN underlies the drive potential, bursts end when Ca²⁺ stops increasing and its decay deactivates I_CAN. If unchecked, Ca²⁺ accumulation via recurrent excitation allows for full network-wide I_CAN activation, locking the network in a state of active spiking or depolarization block. At least 3 mechanisms can halt Ca²⁺ build-up during a burst.

First, the loss of spiking from depolarization block may limit recurrent excitation. This is a form of short-term synaptic depression that would reduce Ca²⁺ entry through a variety of possible mechanisms (32–34). Depolarization block during the inspiratory burst is a widespread feature observed in medullary neurons (22–25) that has not, until now, been analyzed from a functional standpoint. Synaptic depression, driven by depolarization block, could contribute to the characteristically abrupt transition from inspiratory burst to expiratory interval.

Second, activating net outward currents could impede Ca²⁺ entry via hyperpolarization. Our analysis shows numerous ionic mechanisms for evoking outward currents, each of which becomes dominant after depolarization block (e.g., Figs. 3 and 4 and Figs. S3 and S4). Na/K ATPase pumps are particularly well suited for this role in the model because I_CAN drives Na⁺ influx.

Finally, Ca²⁺ store receptors may desensitize or the stores may become depleted, which could lead to Ca²⁺ clearance and thus deactivate I_CAN. A burst-terminating role for receptor desensitization on intracellular Ca²⁺ stores has been hypothesized based on cultured preBötC neuron experiments and ring-oscillator models (12).

Burst termination in different group pacemakers could depend on any one of these mechanisms or a mix of all of them. Broadly speaking, it has been far easier in oscillatory cells and networks to experimentally identify the burst-generating mechanisms compared with burst-terminating mechanisms (20, 21), which suggests that multiple, coactive burst termination mechanisms may be common.

A Group Pacemaker May Explain the Neural Origins of Respiratory Rhythm.

CPGs in a wide variety of animals, from mollusks to mammals, depend on reciprocal synaptic inhibition or specialized subpopulations of pacemaker neurons as the key building block of rhythmogenesis. However, respiratory oscillations in the preBötC cannot be attributed to either canonical mechanism. Emergent network oscillations, in which recurrent excitation provides gradual population-wide recruitment followed by activation of outward burst-terminating currents, have been proposed as a general alternative (3). Preliminary efforts to apply this idea to the preBötC were prescient (10) but were not formalized into a mechanistic model. At present, explicit models predominantly depend on the build-up of synaptic drive (35) and are inconsistent with the robust inspiratory bursts that are now known to rely on intrinsic features such as I_CAN (11–13).

I_NaP is universally expressed in the preBötC (36, 37), in CPGs (3, 38–40), and indeed in all central neurons (41). I_NaP was hypothesized to contribute to respiratory rhythm generation because it can give rise to intrinsic bursting-pacemaker properties. Butera et al. developed a seminal model that investigated the role of I_NaP in respiratory rhythm generation, which showed that individual I_NaP-expressing neurons could initiate, maintain and terminate bursts (42, 43). Despite the possible influence of I_NaP on single-cell dynamics, recent reports show that inspiratory rhythms persist under I_NaP blockade and, in the context of network activity, I_NaP plays little role in drive-potential generation (8, 9, 44–46) but see (47). Therefore, we generally study our model without I_NaP to focus on the plausibility of synaptically evoked I_CAN and the group pacemaker as a distinct rhythmogenic mechanism.

Here, we have shown that I_NaP, even when present at levels insufficient to generate bursts in isolated neurons, can contribute to burst termination and subsequent refractoriness (Fig. 4C and Fig. S3D and S4D). Prior models (42, 43, 48–50) with I_NaP showed that strong AMPA receptor-mediated interactions can cause network burst oscillations in the absence of intrinsic pacemaker properties. This mechanism, classified as a network oscillator by Grillner (3), differs from the group pacemaker because AMPA receptors act as charge carriers rather than catalysts for I_CAN activation as in our model.

Breathing patterns result from embedding the preBötC kernel in a larger respiratory network consisting of inhibitory populations in the rostral medulla and the pons (4). I_NaP has been reported to play a significant role in the embedded system, and becomes particularly important under certain physiological states such as gasping (44, 51). An open question is to what extent a CPG model, and the specific roles of I_NaP and I_CAN, influence the resulting motor pattern when the kernel is embedded in this more extensive network.

Implications for Central Pattern Generation.

Efforts to understand CPGs typically focus on abstracting the essential rhythmogenic components. Here, we present a viable group pacemaker mechanism where the basic rhythmogenic unit is the synaptic gating of a cellular burst-generating conductance (I_CAN), coupled to any one of a host of feedback processes that check recurrent excitation. CPGs featuring this burst mechanism would likely exhibit certain properties not arising from more conventional network oscillator models in which recurrent excitation alone recruits network activity. First, recruiting I_CAN amplifies synaptic excitation, implying that once an activity threshold is reached, an abrupt and robust onset of network-wide activity would naturally occur in a group pacemaker (Fig. S4). Such a transition stands in contrast to the saw tooth-like rise in activity, or the bursts with spike frequencies similar to tonic spiking states, which are predicted patterns of activity for recurrent excitatory network oscillator models lacking synaptic gating of an intrinsic inward current. The resulting clarity of phase demarcation in a group pacemaker could prove advantageous for rhythms whose output is muscle contraction. Second, the transition to depolarization block provides an intrinsic mechanism to curtail the positive feedback component of the burst. In terms of breathing, this mechanism could provide a sensory-independent safeguard against apneusis or excessive lung inflation.

Even in CPGs that depend on synaptic inhibition or pacemaker properties, we postulate that incorporating a synaptically activated burst-generating conductance could contribute to enhancing the robustness or influencing the duration of the active phase. If so, such a conductance would be an advantageous target for neuromodulation because its contribution could be modified in response to behavioral imperatives or physiological challenges to alter active phase properties of the pattern and thus change the motor behavior, without jeopardizing the essential underlying rhythm.

Methods

Each neuron was modeled with a current-balance equation, in a simplified framework that makes bifurcation analysis tractable while still capturing the underlying biophysical phenomena (see SI Appendix). We simulated a coupled pair of neurons and a self-coupled neuron. In the 2-cell case, the cells' parameter values were identical except that the leak reversal potential E_L was varied to introduce heterogeneity. We used XPPAUT software for bifurcation analyses and numerical integration with an adjustable time step, 4th-order Runge-Kutta (RK4) method. XPPAUT is freely available for download from G. Bard Ermentrout, Ph.D., Department of Mathematics, The University of Pittsburgh, www.pitt.edu/∼phase/.

Network simulations with 200 interconnected neurons were performed with custom software in C/C++ on Mac OS 10.4, using RK4 with a fixed time step of 0.1 ms. Unix-compatible source code for performing high-speed simulations with arbitrary network topology can be found at http://people.wm.edu/∼cadeln/code.htm. The baseline parameter values were also used in the large network simulations, except for synaptic conductance and a Ca²⁺ flux scaling factor, which were decreased proportional to the number of synaptic inputs per neuron.