Abstract
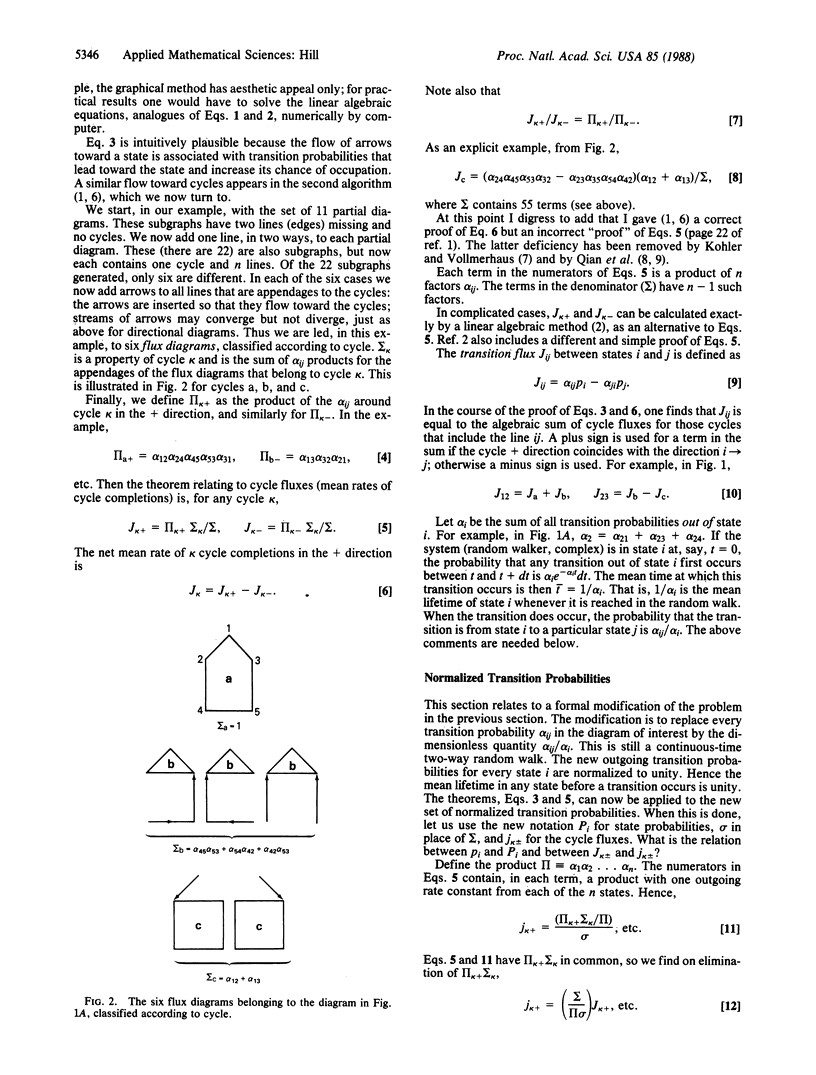
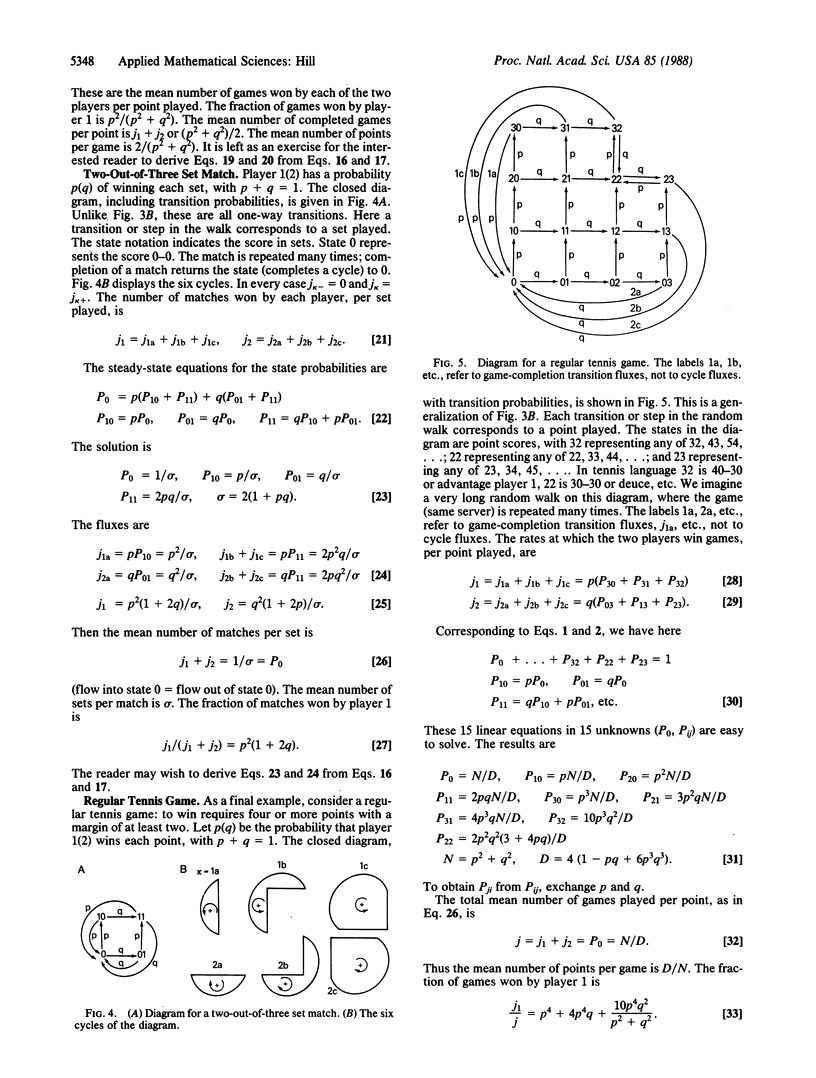
After a review of the diagram method for continuous-time random walks on graphs with cycles, the method is extended to discrete-time random walks. The basic theorems carry over formally from continuous time to discrete time. Three problems in tennis probabilities are used to illustrate random walks on discrete-time diagrams with cycles.
Full text
PDF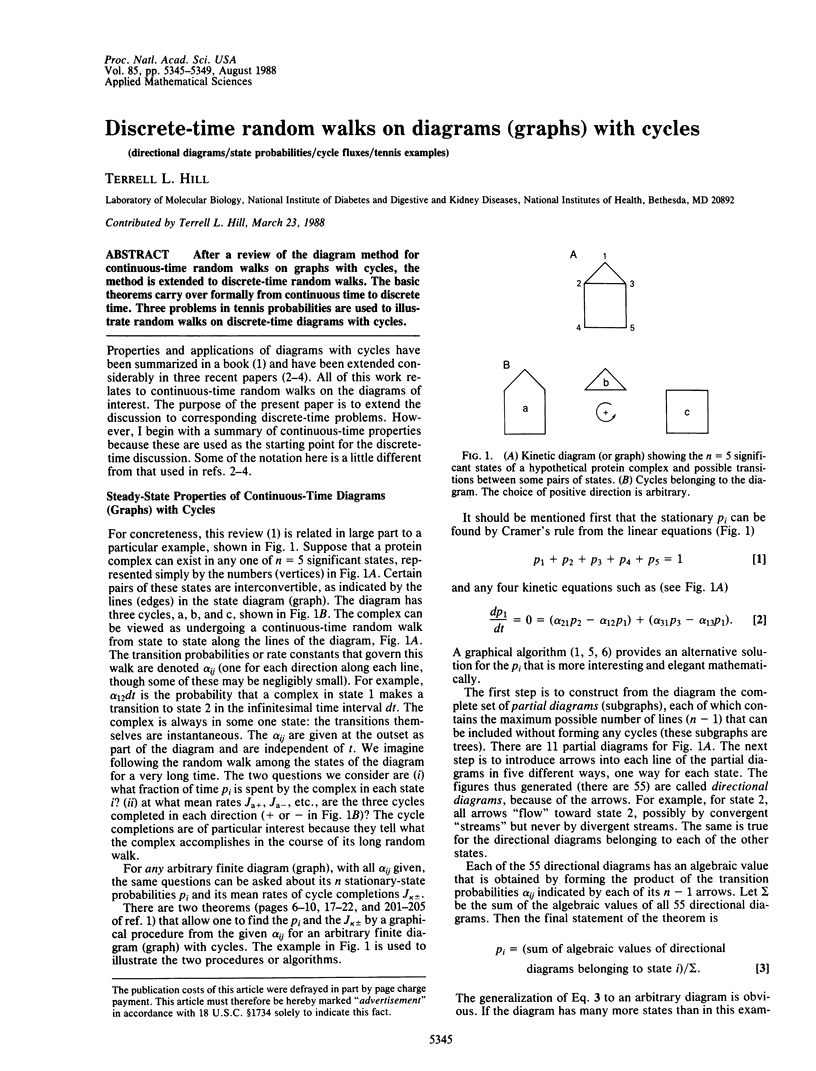


Selected References
These references are in PubMed. This may not be the complete list of references from this article.
- Hill T. L. Further properties of random walks on diagrams (graphs) with and without cycles. Proc Natl Acad Sci U S A. 1988 May;85(10):3271–3275. doi: 10.1073/pnas.85.10.3271. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hill T. L. Interrelations between random walks on diagrams (graphs) with and without cycles. Proc Natl Acad Sci U S A. 1988 May;85(9):2879–2883. doi: 10.1073/pnas.85.9.2879. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hill T. L. Number of visits to a state in a random walk, before absorption, and related topics. Proc Natl Acad Sci U S A. 1988 Jul;85(13):4577–4581. doi: 10.1073/pnas.85.13.4577. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hill T. L. Studies in irreversible thermodynamics. IV. Diagrammatic representation of steady state fluxes for unimolecular systems. J Theor Biol. 1966 Apr;10(3):442–459. doi: 10.1016/0022-5193(66)90137-8. [DOI] [PubMed] [Google Scholar]
- Kohler H. H., Vollmerhaus E. The frequency of cyclic processes in biological multistate systems. J Math Biol. 1980 May;9(3):275–290. doi: 10.1007/BF00276029. [DOI] [PubMed] [Google Scholar]
- Qian C., Qian M., Qian M. P. Markov chain as a model of Hill's theory on circulation. Sci Sin. 1981 Oct;24(10):1431–1448. [PubMed] [Google Scholar]


