Skip to main content
PMC home page
Proceedings of the National Academy of Sciences of the United States of America logoLink to Proceedings of the National Academy of Sciences of the United States of America
. 1996 Apr 30;93(9):4342–4344. doi: 10.1073/pnas.93.9.4342

Dressed polyions, counterion condensation, and adsorption excess in polyelectrolyte solutions.

U Mohanty 1, B W Ninham 1, I Oppenheim 1
PMCID: PMC39538  PMID: 8633067

Abstract

The phenomenon of Manning-Oosawa counterion condensation is given an explicit statistical mechanical and qualitative basis via a dressed polyelectrolyte formalism in connection with the topology of the electrostatic free-energy surface and is derived explicitly in terms of the adsorption excess of ions about the polyion via the nonlinear Poisson-Boltzmann equation. The approach is closely analogous to the theory of ion binding in micelles. Our results not only elucidate a Poisson-Boltzmann analysis, which shows that a fraction of the counterions lie within a finite volume around the polyion even if the volume of the system tends towards infinity, but also provide a direct link between Manning's theta-the number of condensed counterions for each polyion site-and a statistical thermodynamic quantity, namely, the adsorption excess per monomer.

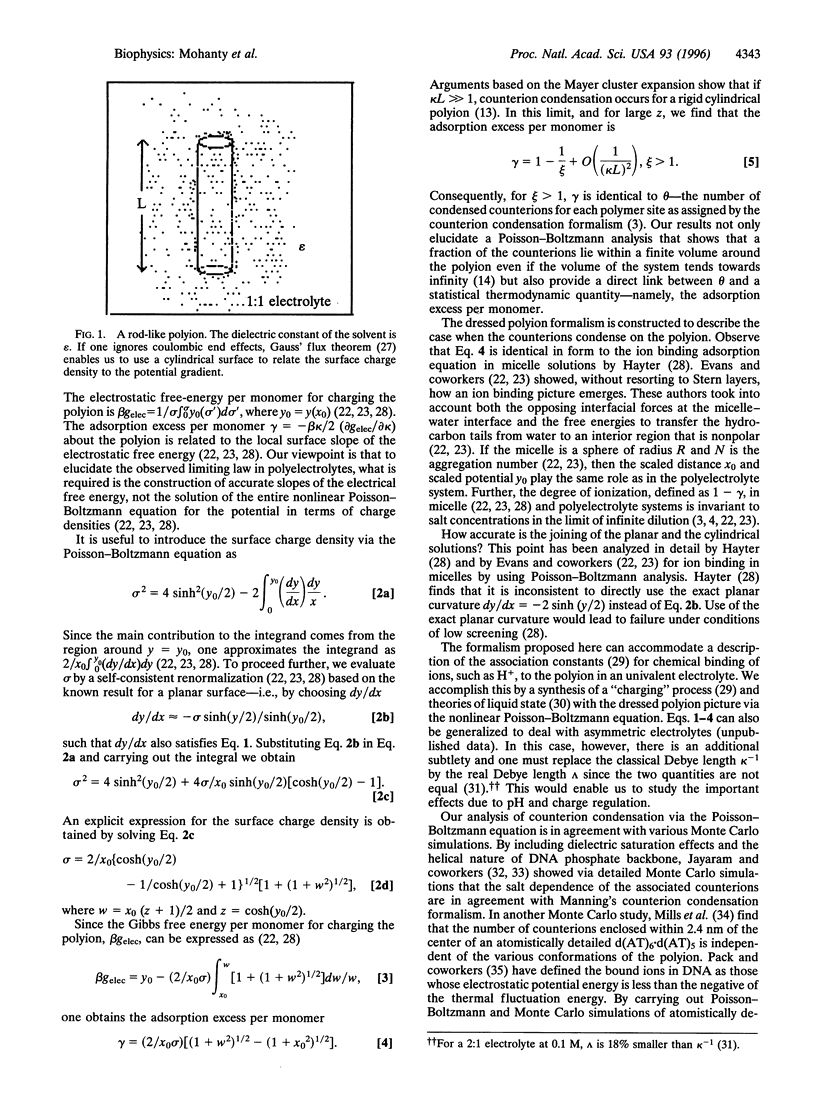
Full text

PDF
4342

Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. Fenley M. O., Manning G. S., Olson W. K. Approach to the limit of counterion condensation. Biopolymers. 1990;30(13-14):1191–1203. doi: 10.1002/bip.360301305. [DOI] [PubMed] [Google Scholar]
  2. Granot J., Feigon J., Kearns D. R. Interactions of DNA with divalent metal ions. I. 31P-NMR studies. Biopolymers. 1982 Jan;21(1):181–201. doi: 10.1002/bip.360210115. [DOI] [PubMed] [Google Scholar]
  3. Lamm G., Wong L., Pack G. R. Monte Carlo and Poisson-Boltzmann calculations of the fraction of counterions bound to DNA. Biopolymers. 1994 Feb;34(2):227–237. doi: 10.1002/bip.360340209. [DOI] [PubMed] [Google Scholar]
  4. Manning G. S. The molecular theory of polyelectrolyte solutions with applications to the electrostatic properties of polynucleotides. Q Rev Biophys. 1978 May;11(2):179–246. doi: 10.1017/s0033583500002031. [DOI] [PubMed] [Google Scholar]
  5. Mills P. A., Rashid A., James T. L. Monte Carlo calculations of ion distributions surrounding the oligonucleotide d(ATATATATAT)2 in the B, A, and wrinkled D conformations. Biopolymers. 1992 Nov;32(11):1491–1501. doi: 10.1002/bip.360321108. [DOI] [PubMed] [Google Scholar]
  6. Mohanty U., Zhao Y. Polarization of counterions in polyelectrolytes. Biopolymers. 1996 Mar;38(3):377–388. doi: 10.1002/(sici)1097-0282(199603)38:3<377::aid-bip10>3.0.co;2-a. [DOI] [PubMed] [Google Scholar]
  7. Record M. T., Jr, Anderson C. F., Lohman T. M. Thermodynamic analysis of ion effects on the binding and conformational equilibria of proteins and nucleic acids: the roles of ion association or release, screening, and ion effects on water activity. Q Rev Biophys. 1978 May;11(2):103–178. doi: 10.1017/s003358350000202x. [DOI] [PubMed] [Google Scholar]
  8. Saif B., Mohr R. K., Montrose C. J., Litovitz T. A. On the mechanism of dielectric relaxation in aqueous DNA solutions. Biopolymers. 1991 Sep;31(10):1171–1180. doi: 10.1002/bip.360311005. [DOI] [PubMed] [Google Scholar]
  9. Stevens MJ, Kremer K. Structure of salt-free linear polyelectrolytes. Phys Rev Lett. 1993 Oct 4;71(14):2228–2231. doi: 10.1103/PhysRevLett.71.2228. [DOI] [PubMed] [Google Scholar]
  10. Zimm B. H., Le Bret M. Counter-ion condensation and system dimensionality. J Biomol Struct Dyn. 1983 Oct;1(2):461–471. doi: 10.1080/07391102.1983.10507455. [DOI] [PubMed] [Google Scholar]

Articles from Proceedings of the National Academy of Sciences of the United States of America are provided here courtesy of National Academy of Sciences

RESOURCES