Generation and confirmation of a (100 × 100)-dimensional entangled quantum system

Significance

Quantum entanglement is one of the key features of quantum mechanics. Quantum systems are the basis of new paradigms in quantum computation, quantum cryptography, or quantum teleportation. By increasing the size of the entangled quantum system, a wider variety of fundamental tests as well as more realistic applications can be performed. The size of the entangled quantum state can increase with the number of particles or, as in the present paper, with the number of involved dimensions. We explore a quantum system that consists of two photons which are 100-dimensionally entangled. The dimensions investigated are the different spatial modes of photons. The result may have potential applications in quantum cryptography and other quantum information tasks.

Abstract

Entangled quantum systems have properties that have fundamentally overthrown the classical worldview. Increasing the complexity of entangled states by expanding their dimensionality allows the implementation of novel fundamental tests of nature, and moreover also enables genuinely new protocols for quantum information processing. Here we present the creation of a (100 × 100)-dimensional entangled quantum system, using spatial modes of photons. For its verification we develop a novel nonlinear criterion which infers entanglement dimensionality of a global state by using only information about its subspace correlations. This allows very practical experimental implementation as well as highly efficient extraction of entanglement dimensionality information. Applications in quantum cryptography and other protocols are very promising.

Quantum entanglement of distant particles leads to correlations that cannot be explained in a local realistic way (1–3). To obtain a deeper understanding of entanglement itself, as well as its application in various quantum information tasks, increasing the complexity of entangled systems is important. Essentially, this can be done in two ways. The first method is to increase the number of particles involved in the entanglement (4). The alternative method is to increase the entanglement dimensionality of a system.

Here we focus on the latter one, namely on the dimension of the entanglement. The text is structured as follows. After a short review of properties and previous experiments, we present a unique method to verify high-dimensional entanglement. Then we show how we experimentally create our high-dimensional two-photon entangled state. We analyze this state with our method and verify a 100 × 100-dimensional entangled quantum system. We conclude with a short outlook to potential future investigations.

High-dimensional entanglement provides a higher information density than conventional two-dimensional (qubit) entangled states, which has important advantages in quantum communication. First, it can be used to increase the channel capacity via superdense coding (5). Second, high-dimensional entanglement enables the implementation of quantum communication tasks in regimes where mere qubit entanglement does not suffice. This involves situations with a high level of noise from the environment (6, 7), or quantum cryptographic systems where an eavesdropper has manipulated the random number generator involved (8). Moreover, the entangled dimensions of the whole Hilbert space also play a very interesting role in quantum computation: high-dimensional systems can be used to simplify the implementation of quantum logic (9). Furthermore, it has been found recently (10) that any continuous measure of entanglement (such as concurrence, entanglement of formation, or negativity) can be very small, while the quantum system still permits an exponential computation speedup over classical machines. This is not the case for the dimension of entanglement—for every quantum computation, it needs to be high (11, 12), which is another hint at the fundamental relevance of the concept.

So far, high-dimensional entanglement has been implemented only in photonic systems. There, different multilevel degrees of freedom, such as spatial modes (13), time-energy (14), path (15, 16), as well as continuous variables (17, 18), have been used. Entanglement of spatial modes of photons has especially attracted much attention in recent years (19–28), because it is readily available from optical nonlinear crystals and the number of involved modes of the entanglement can be very high (29).

In a recent experiment the nonseparability of a two-photon state was shown, by observing Einstein–Podolsky–Rosen correlations of photon pairs in down-conversion (30) (for a similar experiment, see ref. 31). The authors were able to observe entanglement of ∼2,500 spatial states with a camera. In our experiment we go a step further and not only show nonseparability, but we can also extract information about the dimensionality of the entanglement. Precisely, we experimentally verify 100-dimensional entanglement.

One main challenge that remains is the detection and verification of high-dimensional entanglement. For reconstructing the full quantum state via state tomography, the number of required measurements is impractical even for relatively low dimensions because it scales quadratically with the quantum system dimension (24, 27). Even if one had reconstructed the full quantum state, the quantification of the entangled dimensions is a daunting task analytically and even numerically (32). If the full density matrix of the state is not known, it is only possible to give lower bounds of the entangled dimensions. Such methods are usually referred to as a “Schmidt number witness” (33–35).

Results

In our experiment we are in a regime where it is unfeasible to reconstruct the full density matrix because of the required number of measurements due to the high dimension. Therefore, we can only identify lower bounds of the entangled dimensions. Furthermore, all previously published methods for extracting the dimension of entanglement turned out to be impractical for our system. They usually require access to observables on the full Hilbert space, which were not available for our experiment. For these reasons we were required to develop a novel approach.

Strictly speaking, we found a mathematically well-defined and intuitively reasonable method that answers the question, “For a given high-dimensional two-photon state, if correlations between D dimensions of each photon are measured, what is the minimum necessary entanglement dimensionality d required to explain the correlations?” (The precise mathematical formulation of this question is given in SI Text.)

Our approach works such that we define a measurable witness-like quantity W and search for the d-dimensional entangled state maximizing it. When we perform the measurement and exceed the maximal value, we know that the measured quantum state was at least (d + 1)-dimensional entangled. This approach is a generalization of conventional entanglement witnesses, which define a boundary between separable and entangled states. We not only want a boundary between separable and entangled states, but also between different dimensions of entanglement.

The main idea is to look at two-dimensional subspaces, and therewith measure the correlations of the two photons. In analogy to two-dimensional systems (such as photon polarization), we can measure the visibilities in three mutually unbiased bases (MUBs). Mathematically, the visibility is defined as 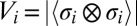, i = {x,y,z}, where σ_i denotes the single-qubit Pauli matrices (for polarization σ_x, σ_y, and σ_z represent measurements in the diagonal/antidiagonal, left/right, and horizontal/vertical basis, respectively). The concept of the measurement is illustrated in Fig. 1. With these measurements, it is possible to detect entanglement between two-dimensional subsystems (35). What we found is one way how such measurements in all two-dimensional subsystems imply a lower bound of the entangled dimensions in the whole quantum system.

Fig. 1.

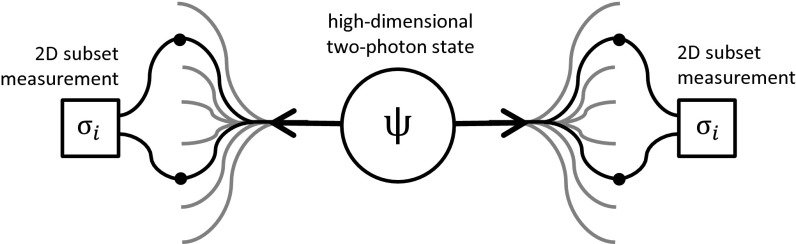
Visualization of the measurement concept. The two photons are sent in two different directions. Each of the photons is in a mixture of many modes. We perform the same two-dimensional subspace measurement on both photons. When we consider all two-dimensional subspaces, we can determine the dimensionality of entanglement.

Our measureable quantity W is the sum of the visibilities in all two-dimensional subspaces

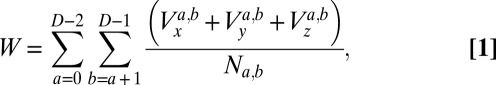where a and b stand for specific states of the photons, D is defined as above (it stands for the number of modes considered), V_i^a,b stands for the visibility in basis i, and N_a,b stands for the normalization. N_a,b is the source of the nonlinearity of W which leads to convenient experimental properties, however makes it very difficult in general to handle mathematically. That nonlinearity is responsible for the fact that the measurement results are automatically normalized (i.e., all visibilities can go up to 1), because by measuring in two-dimensional subspaces, we ignore all other modes (SI Text). Therefore, we do not need to renormalize our measurement results in any way afterward. Nonlinear entanglement witnesses have already been used in earlier experiments and demonstrated specific advantages over linear witnesses (36, 37).

Next we search for the d-dimensional entangled state which is maximizing the quantity W in Eq. 1. The maximization was not yet possible in general (which remains an interesting open problem, especially for more realistic experimental situations). However, we maximized W for a very large and particularly important class which we believe to be sufficient for our experiment. In other words, we used a physical assumption about our state in the derivation, which we will explain in more detail later in this section. The basis of the maximization is a combination of the method of Lagrange multipliers and algebraic considerations. This enables us to find the maximizing d-dimensional quantum state for the quantity W (SI Text), and implies an upper bound on the quantity in Eq. 1 for d-dimensional entangled states, which can be written as

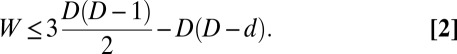If the measurements exceed the bound, the quantum state was at least (d + 1)-dimensionally entangled. Otherwise, if the inequality is fulfilled (W is smaller than the right side of Eq. 2), we cannot make a statement about the dimensionality of entanglement.

The bounds can be understood intuitively. A maximally entangled state in D dimensions will have a visibility of one in all three MUBs in every two-dimensional subspace. This is represented by the first term on the right side. If the entanglement dimensionality of the state is smaller than that of the observed Hilbert space, the maximally reachable value decreases by D for each nonentangled dimension (D − d), which is expressed by the second term.

The quantity in Eq. 1 is remarkable because the number of required measurements scales only linearly with the dimension of the whole Hilbert space, in contrast to state tomography, which scales quadratically. Furthermore, it only involves measurements in two-dimensional subspaces, which are easier to implement than general high-dimensional measurements. Moreover, the quantity W in Eq. 1 is nonlinear, which makes it particularly efficient for nonmaximally entangled quantum states (SI Text).

In our experiment, we apply this unique method to a two-photon quantum system. The photon pair is created by pumping a nonlinear crystal with a laser, where spontaneous parametric down-conversion (SPDC) occurs. For the high-dimensional degree of freedom we use spatial modes of light. Specifically, we use the Laguerre–Gauss (LG) basis to analyze entanglement. LG modes form a basis of solutions of the paraxial wave equation in the cylindrical coordinate system. They are described by two quantum numbers. One quantum number l corresponds to the orbital angular momentum (OAM, or equivalently, the topological charge) of the photon (38, 39). The second quantum number n corresponds to the radial nodes in the intensity profile. Only lately this second degree of freedom has been analyzed theoretically in a quantum mechanical framework (40–42).

In the down-conversion process the angular momentum of the photons is conserved, therefore this degree of freedom is anticorrelated. For the radial quantum number n the situation is more complicated. The full down-conversion process concerning the correlations for the radial quantum number has been analyzed in detail (40) and quasiperfect correlations have been found for specific situations. Recently, these quasiperfect correlations have been demonstrated experimentally (43). The state we expect from down-conversion can then be written as a perfectly (anti-)correlated pure state 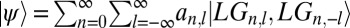with l and n dependent coefficients a. In the derivation of the bounds in Eq. 2 we restricted the states to be perfectly correlated. This means we assume a physical property of our input state, namely perfect (anti-)correlation of the modes. Small deviations from this assumption (which we have observed in the experiment) have been analyzed numerically (SI Text), and we found that it only reduces our observed W, thus justifies the application of the criterion in Eq. 2 in our experiment. The full analytical treatment in the general case is an interesting open problem.

The experimental analysis of the LG modes of the photon pair produced is done by a holographic mode transformation using a spatial light modulator (SLM). With that we can transform any desired mode to a Gauss mode. By using a single-mode fiber (SMF), we filter only for Gauss modes and thereby project the quantum state into the desired mode (39). The setup and exemplary LG modes are shown in Fig. 2.

Fig. 2.

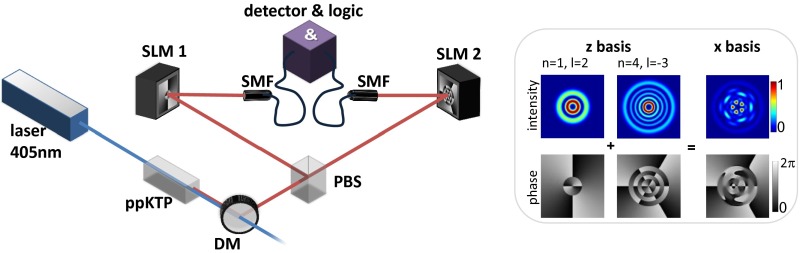
(A) Schematic of the experimental setup. We pump a type-II nonlinear periodically poled potassium titanyl phosphate (ppKTP) crystal with a 405-nm, ∼40-mW single-mode laser. SPDC creates collinear photon pairs with 810-nm wavelength and orthogonal polarizations. We remove the pump beam at a dichroic mirror (DM) and separate the two photons at a polarizing beam splitter (PBS). In both arms of the setup we use SLMs to perform a mode transformation of the photons. The transformation done by a computer-generated hologram at the SLM converts a specific mode into the fundamental Gauss mode. Only the Gauss mode couples into an SMF, thus the SLM + SMF combination acts as a mode filter (39). In the end, we detect the photons with avalanche photodiode based single-photon detectors and analyze the time correlation using a coincidence logic. (B) An example of a two-dimensional subspace is shown. The intensities and phases for two different modes in the z basis are demonstrated, and their superposition leads to a mode in the x basis. The y basis can be constructed similarly.

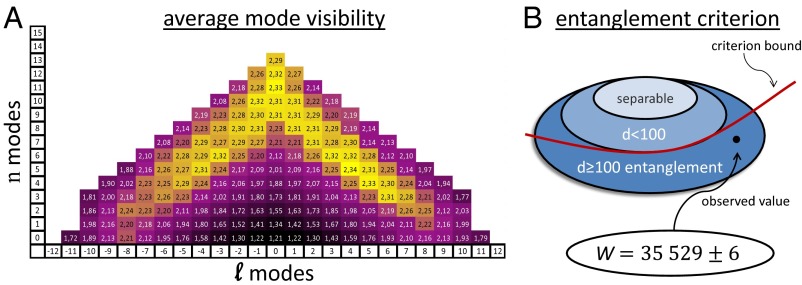
In our experiment we analyze the correlations of 186 modes of two photons (Fig. 3). The number of modes, 186 in our case, corresponds to D in Eq. 2. We use LG modes with an angular quantum number up to l = 11, and a radial quantum number up to n = 13. To calculate the quantity in Eq. 1, we need to measure in every two-dimensional subspace [there are (186 × 185)/2 = 17,205 two-dimensional subspaces] the visibility in x, y, and z basis, which corresponds to 3 × 4 measurement per subspace. Altogether this results in ∼200,000 measurements (with ∼750 million detected photon pairs). For comparison, if we had performed a full state tomography, we would have needed to perform more than 1 billion measurements. When we sum up all of our measured visibilities according to Eq. 1, we find

which corresponds to at least 100-dimensional entanglement according to inequality 2 (101-dimensional: W > 35,619; 100-dimensional: W > 35,433; 99-dimensional: W > 35,247). The confidence interval corresponds to one SD due to the statistical uncertainty. It has been calculated using Monte Carlo simulation assuming Poisson distribution of the count rates. The detailed measurement results and the calculation of [3] can be seen in Fig. 4 and in SI Text. The quantity W in [1] corresponds to measurements of all two-dimensional subspaces in a D × D-dimensional quantum state. It can be seen in Fig. 4A that some modes contribute more to the quantity than others, thus we can try to find a smaller optimal set of modes that shows the higher-dimensional entanglement. We find that by removing 19 modes (that means, not taking into account all two-dimensional subspace measurements with them), we can find at least 103-dimensional entanglement.

Fig. 3.

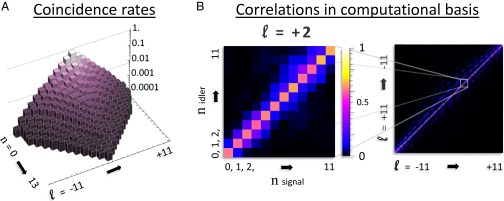
(A) Normalized coincidence rate of different modes (with logarithmic scale), depending on the two mode-numbers (full-field bandwidth). The absolute count rate was 105,500 photon pairs per second for a pump power of 60 mW. To be precise, this is the summed count rate of all 186 modes, not taking into account the inefficiencies of the detectors or imperfect coupling into SMFs. (B) Weighted correlations between different modes in z basis. Due to different probabilities of different modes, in these pictures we weight every correlation with the probability of the modes involved. That means, 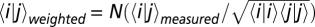, where i and j stand for different two-photon modes, and N is a normalization constant. (Left) The correlation of modes with l = 2 is shown, and reveals good correlation of modes with the same number of radial nodes. (Right) All correlations in the z basis are visualized.

Fig. 4.

(A) The average sum of visibilities (in x, y, and z basis) of a specific mode with all other modes is shown. The observable structure originates from nonmaximally entanglement due to different count rates for different modes (Fig. 3A). The bright regions in the center are modes with a similar probability. The central low-order modes (such as the Gauss mode) have the highest probability, therefore the lowest average visibility. Precisely, the Gauss mode has an average sum of visibilities of 1,21 (which mainly results from the visibilities in z basis). A maximally entangled high-dimensional state would have a summed visibility of 3 for every mode pair. The structure shows that different modes contribute differently to the entanglement criterion. (B) To reveal information about the global, high-dimensional entanglement, we have to calculate the value for the quantity W in Eq. 1. This is done by summing up all visibilities of all subsets. Here, the concept and result of the entanglement dimensionality criterion is visualized. With our criterion in Eq. 2, quantum states are divided into two parts: those with entanglement dimensionality smaller than 100 and those with a larger one (red line). We have observed a value that lies in the lower part, thus we have verified 100-dimensional entanglement.

One way to bring this in relation with other photonic and multipartite entanglement experiments is the following. The dimension of the entangled Hilbert-space scales with dim = d^N, where d stands for the entangled dimensions and N is the number of involved parties. Our experiment shows an entangled Hilbert-space dimension of dim = (103 × 103) ≈ 2^13.4 that is larger than the largest entangled photonic Hilbert space reported so far (with dim = 2¹⁰) (44). Interestingly, it is of similar magnitude as that of the largest quantum systems with multipartite entanglement measured so far, such as 14-qubit ion entanglement with dim = 2¹⁴ (45).

Discussion

Our results show that we can experimentally access a quantum state of two photons which is at least (100 × 100)-dimensionally entangled. This was possible by developing a unique method to analyze efficiently and in an experimentally practical way quantum states with very high dimensions. Furthermore, we exploited the full potential of transverse spatial modes, namely both radial and angular quantum numbers.

Such high-dimensional entanglement offers a great potential for quantum information applications. There are situations where two-dimensional entanglement is no longer sufficient but high-dimensional entangled systems are able to perform the task. In realistic quantum cryptography schemes, for example where noisy environment or manipulated random number generators lead to a breakdown of the system for low-dimensional entangled states, high-dimensionality of the entanglement sustains the security (6–8). The experimental setups as presented here are suitable for such tasks. Additionally, for quantum computation it is necessary to use a large entangled Hilbert space for any quantum speedup. As our result shows that very high-dimensional entangled Hilbert spaces are experimentally accessible, we envision that it will trigger future experiments to solve the next important open question: How to implement experimentally arbitrary controlled transformations between spatial modes to realize quantum computational or similar tasks.