Chatterjee and Templin. 10.1073/pnas.0609975104. |
Fig. 5. Holotype of M. gui (IVPP V13352) as preserved. (Scale bar, 5 cm.) (Inset) Enlarged view of the leg feathers [modified from Xu et al. (1)].
Fig. 6. (A) Articulation of pelvic girdle and femur (length of femur, 12 cm) of Bambiraptor in anterior view showing normal vertical limb posture. (B) The same anterior view; but the femur is put in a horizontal pose, so that it becomes unstable at the joint and dislocated from the hip socket; no dinosaur could assume this horizontal leg posture; in the reconstruction of Microraptor by Xu et al. (figure 1c in ref. 1), the femur is placed in this horizontal fashion, which is anatomically incorrect. (C) Life restoration of Microraptor at the National Museum of Rio de Janeiro, Brazil, where the hindlimb is shown in a vertical pose. However, the leading edge of the asymmetric flight feathers on the metatarsals faces downward (shown by the arrow), which would fail to generate thrust and lift. The orientation of the metatarsal feathers in this pose is incorrect from an aerodynamic point of view.
Fig. 7. (A) Hypothetical taphonomy of M. gui (holotype) just after death to show the orientation of hindlimb feathers; note that the metatarsal feathers were extended in a transverse plane to become an effective airfoil, whereas tibial feathers were directed backward for streamlining. (B) The same animal after burial and fossilization.
Fig. 8. Cladogram illustrating the phylogenetic relationships of Microraptor to other feathered theropods and early birds (modified from refs. 2-4).
1. Xu X, Zhou Z, Wang X, Kuang X, Zhang F, Du X (2003) Nature 421:335-340.
2. Holtz TR (2005) Nat Hist 114:40-41.
3. Xu, X., Zhang, F. (2005) Naturwissenschaften 92:173-177.
4. Zhang F, Zhou Z (2004) Nature 431:925.
SI Text
Hindlimb Posture of Theropods.
The parasagittal hindlimb posture of Microraptor is beautifully preserved in the holotype specimen (1) (Fig. 1). In all theropods (including birds), the hip, knee, and ankle joints are stable and fully congruent during parasagittal motion of the hindlimbs, permitting a wide range of flexion and extension but little abduction and adduction. Manipulation of the hip and femoral joint of Bambiraptor (2), a close relative of Microraptor, endorses the parasagittal limb motion in dromaeosaurs (Fig. 6A). The hip joint quickly becomes incongruent as the femur is abducted horizontally from the parasagittal plane (Fig. 6B).The vertical hindlimb posture has been corrected in a recent life restoration of Microraptor at the National Museum of Rio de Janeiro, Brazil, but the orientation of the asymmetric feathers on the metatarsus still remains problematic in this posture because the rear wings have been held vertically under the body, where the leading edge of the asymmetric feathers faces downward like a rudder that would fail to generate any lift (Fig. 6C). The gliding performance of Microraptor would have suffered if the hindlimbs were not part of the lifting system. These flight feathers should be horizontal and face forward against the wind direction to generate lift.
Taphonomy of Microraptor.
The orientation of the metatarsal feathers in the holotype specimen appears to be a preservational artifact along the bedding plane (Fig. 5). Because they are asymmetric flight feathers like those of the forewings, the narrow, leading edge should face forward against the direction of airflow to gain lift. We suggest that these feathers were oriented in life in a transverse horizontal plane like those of the hand section to be an effective airfoil during flight (Fig. 7A) but that they collapsed backward during fossilization (Fig. 7B).Methodology.
To analyze the flight performance of Microraptor, we used two computer algorithms, ANFLTPWR (animal flight power) and ANFLTSIM (animal flight simulation), which are based on the streamtube model (6). In all cases, the body and wing drag coefficients are computed as functions of the Reynolds number (see refs. 6 and 7 for detailed methodology). Reynolds number is a dimensionless quantity combining fluid density, viscosity, and the size and speed of a body immersed in the fluid; it is a measure of scale effects on fluid flow and body force coefficients.Most functional analyses of flight performances of flying animals have centered on birds, taking advantage of aerodynamic equations used in aircraft design. The most common method for approximate performance estimation is an aircraft-like model (8), which uses familiar parameters such as lift and drag coefficients, wing area, wing aspect ratio, and span efficiency. With this method, the power required to maintain steady level flight is calculated as the product of aerodynamic drag and flight airspeed. The total drag is calculated as the sum of two components: the so-called induced drag, which is the penalty that must be paid for the production of aerodynamic lift, and a component (sometimes called zero-lift drag) assumed independent of lift, comprising pressure drag and surface skin friction. This simple method has a limitation: it predicts infinite induced drag and power at zero airspeed and therefore cannot be used without modification for performance estimation in hovering or near-hovering flight. A second model, known as momentum streamtube theory, was originally proposed for straight wings by Prandtl and Tietjens (see ref. 9) and developed further by helicopter designers (13) and adapted to animal flight (6, 7). It avoids the zero-speed problem by making the assumption that a cylindrical tube of air having a cross-section area (A) with diameter approximately equal to the wingspan (b), or rotor diameter, initially approaching the wing or rotor at flight speed (V), is deflected downward through an angle (ø), which may vary from 90° in hovering at zero speed to a small angle in cruising and high-speed flight. The flight power is equated to the change in kinetic energy flow in the streamtube from far upstream to far downstream, which is required to balance lift and aerodynamic drag. Compared with the aircraft method, the streamtube model has its own drawbacks: unfamiliarity to most animal flight researchers and greater numerical complexity in application. However, it is important to note that the two methods produce essentially identical results in powered flight at speeds that are usually >5-10 m/s.
Gliding Flight.
Microraptor's wing loading (70 N/m2) is relatively high compared with that of birds of similar size (11), but it compares more closely with that of gliding vertebrates. As a rule, gliders tend to have much higher wing loadings (up to two or three times higher) than flapping flyers of the same mass (11), and Microraptor conforms to this pattern. The gliding speed would range from 12 m/s (to stay up as long as possible or to make best use of a thermal) to 15 m/s (to glide the greatest horizontal distances). Microraptor was able to maintain significant stability with the help of a long tail terminating in a frond-like horizontal flap. The dorsal wing was probably used for controlling gliding through motions such as sweep and twist, and perhaps through moderate flapping. In the eventual evolution of avian flight, stability would be killed in favor of greater maneuverability by the loss of the long tail (12).Several "flying" fishes, gliding lizards, flying squirrels, and colugos have evolved broad horizontal membranes to catch an airstream (13), but their wing is fundamentally different in form and function from that of Microraptor. The pros and cons of the suggested biplane wing configuration of Microraptor are difficult to sort out. Wings farther apart and nearer equal length would have given a better lift/drag ratio, but, given the limits on the span of the lower wing, a higher chord (lower aspect ratio) on the lower wing may have been beneficial.
The total induced drag of a biplane is theoretically lower than that of a monoplane of the same span and of the same total lift. The lowest induced drag in a biplane is by having equal wingspans and a large vertical separation between the wings. In Microraptor, although the vertical spacing is large, the ventral wing is shorter than the dorsal wing. For that configuration, Prandtl and Tietjens (figure 179 in ref. 9) suggested that the induced drag reduction is only »5%. Thus the biplane wing configuration may have conferred some aerodynamic fitness in the initial stage of avian flight, enough advantage for a start-up glider like Microraptor.
Takeoff from a Perch.
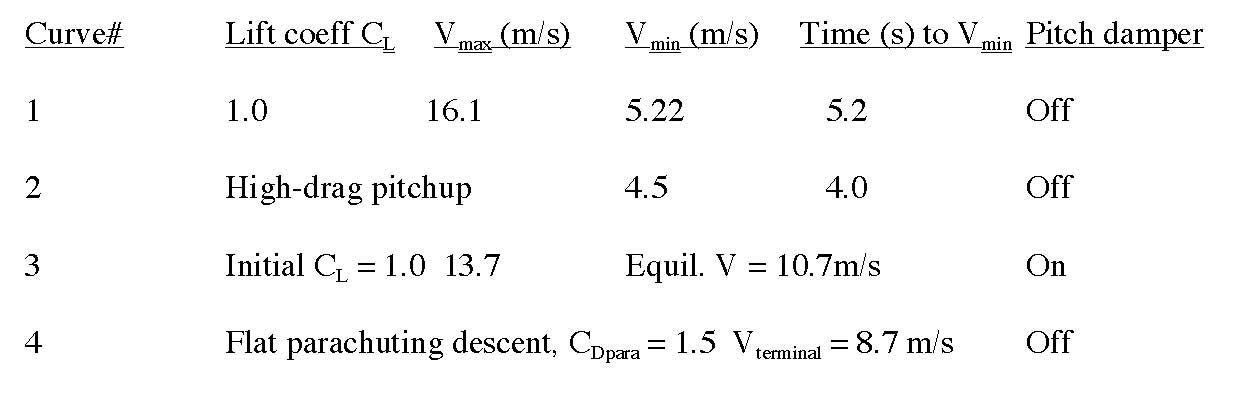
Whenever birds with a mass greater than »0.1 kg take off from the ground, they need speed to become airborne (8, 11). For Microraptor, with an estimated mass of 1 kg, takeoff from the ground would be impossible because it would need a long run, a short burst of anaerobic power, and vigorous dorsoventral flapping of the wing about the glenoid-humeral joint. Anatomical evidence indicates that Microraptor was not capable of ground or running takeoff because it lacked the supracoracoideus pulley to elevate the wings. As a result, it could not flap its wings quickly for lift generation. Moreover, running takeoff would damage the ventral metatarsal wings (1). In addition to its relatively high wing loading, Microraptor lacked the necessary power to lift off from the ground. Flight simulations of powered takeoffs from the ground indicate that this is impractical. At estimated maximum available power, equilibrium level flight may be marginally possible (see Fig. 3A), but takeoff distances are indefinitely long. Moreover, the forewing area appears to be too small to absorb any significant anaerobic power above the continuous level without exceeding maximum lift limits. For this reason, most living birds prefer to take off from an elevated perch to secure elevated lift (11).We have calculated the takeoff capability of Microraptor from a perch using both streamtube (6) and aircraft (8) models (Fig. 3C). Curve 1 shows phugoid gliding. Curve 2 shows an alternative method for rapid deceleration immediately before landing: rapid pitchup or steepening of the body and wing, which induces reduction in lift and a large increase in drag. The short "hook" shows such a trajectory for Microraptor, initiated after the path has flattened. Curve 3 shows a gliding path with pitch damper on. The lift coefficient was held constant at 1.0 in the undamped trajectory, but when the pitch damper is activated, the lift coefficient varies continuously as speed changes during the glide, until it eventually settles down to the original specified value. The "safe" landing speed for flying animals is »5 m/s (6). Curve 4 shows a parachuting trajectory. Parameters for the numbered curves are as follows:

Phugoid Gliding.
The term "phugoid" (Greek word, meaning take flight) was coined in the early days of aircraft flight to denote a characteristic pitching oscillation. These oscillations occur whenever any winged animal (or aircraft) finds itself in a nonequilibrium flight, such as after being launched without sufficient wing lift to balance its weight. In gliding flight, the motion is eventually damped to a steady glide and, in fact, the rate of damping is inversely proportional to the lift-drag (L/D) ratio.Streamlining of the Tibia.
Drag could be dramatically reduced in a gliding Microraptor by streamlining the vertically held tibia with feathers so that the turbulent spaces behind are filled in and the front areas are rounded or tapered (Fig. 2E-G). The greater the turbulence, the greater the drag, which could be reduced considerably by streamlining. In fact, filling in the turbulent area behind the object improves streamlining more effectively than does altering the front. Using ANFLTPWR, we computed the zero-lift drag and converted this to the so-called parasite drag area, which is the area of a circular disk facing forward with a drag coefficient equal to 1.0 that would have the same drag. At 10 m/s airspeed (without tibia) this was 0.002 m2 (a disk with a diameter of »4 cm). We measured the tibia diameter of the holotype specimen at »0.6 cm (0.006 m) and tibia length as 13 cm (1) (Fig. 1A). The frontal area of two tibiae is thus »0.0016 m2. The Reynolds number of the tibia is »4,000, in the range where the drag coefficient of a circular cylinder is close to 1.0. Thus the ratio of the tibia drag to that of the animal without tibia is 0.0016/0.0020 = 0.8 (hence the nearly 40% increase in animal drag with an unstreamlined tibia). The large performance advantage obtained by retracting undercarriages has been long known by aircraft designers. Even in small personal aircraft, which leave the undercarriage exposed, the supporting struts are airfoil-shaped, and wheels are usually enclosed in streamlined spats.1. Xu X, Zhou Z, Wang X, Kuang X, Zhang F, Du X (2003) Nature 421:335-340.
2. Holtz TR (2005) Nat Hist 114:40-41.
3. Xu, X., Zhang, F. (2005) Naturwissenschaften 92:173-177.
4. Zhang F, Zhou Z (2004) Nature 431:925.
5. Burnham DA, Derstler KL, Currie PJ, Bakker RT, Zhou Z, Ostrom JH (2000) Univ Kansas Paleont Contr (new series) 13:1-14.
6. Templin RJ (2000) Prog Aeronaut Sci 36:393-436.
7. Chatterjee S, Templin RJ (2004) Geol Soc Am Sp Pap 376:1-64.
8. Pennycuick CJ (1975) Mechanics of Flight in Avian Biology, eds Farner DS, King JR (Academic, New York), Vol 5, pp 1-75.
9. Prandtl L, Tietjens OG (1934) Applied Hydro- and Aeromechanics (McGraw-Hill, New York).
10. Stepniewski WZ, Keys CM (1984) Rotary-Wing Aerodynamics (Dover, Minneola, NY).
11. Tennekes H (1996) The Simple Science of Flight (MIT Press, Cambridge, MA).
12. Chatterjee S (1997) The Rise of Birds (Johns Hopkins Univ Press, Baltimore).
13. Alexander DE (2002) Nature's Flyers (Johns Hopkins Univ Press, Baltimore).