Skip to main content
PMC home page
Biophysical Journal logoLink to Biophysical Journal
. 1997 Dec;73(6):3192–3210. doi: 10.1016/S0006-3495(97)78345-0

Statistical mechanics of simple models of protein folding and design.

V S Pande 1, A Y Grosberg 1, T Tanaka 1
PMCID: PMC1181222  PMID: 9414231

Abstract

It is now believed that the primary equilibrium aspects of simple models of protein folding are understood theoretically. However, current theories often resort to rather heavy mathematics to overcome some technical difficulties inherent in the problem or start from a phenomenological model. To this end, we take a new approach in this pedagogical review of the statistical mechanics of protein folding. The benefit of our approach is a drastic mathematical simplification of the theory, without resort to any new approximations or phenomenological prescriptions. Indeed, the results we obtain agree precisely with previous calculations. Because of this simplification, we are able to present here a thorough and self contained treatment of the problem. Topics discussed include the statistical mechanics of the random energy model (REM), tests of the validity of REM as a model for heteropolymer freezing, freezing transition of random sequences, phase diagram of designed ("minimally frustrated") sequences, and the degree to which errors in the interactions employed in simulations of either folding and design can still lead to correct folding behavior.

Full text

PDF

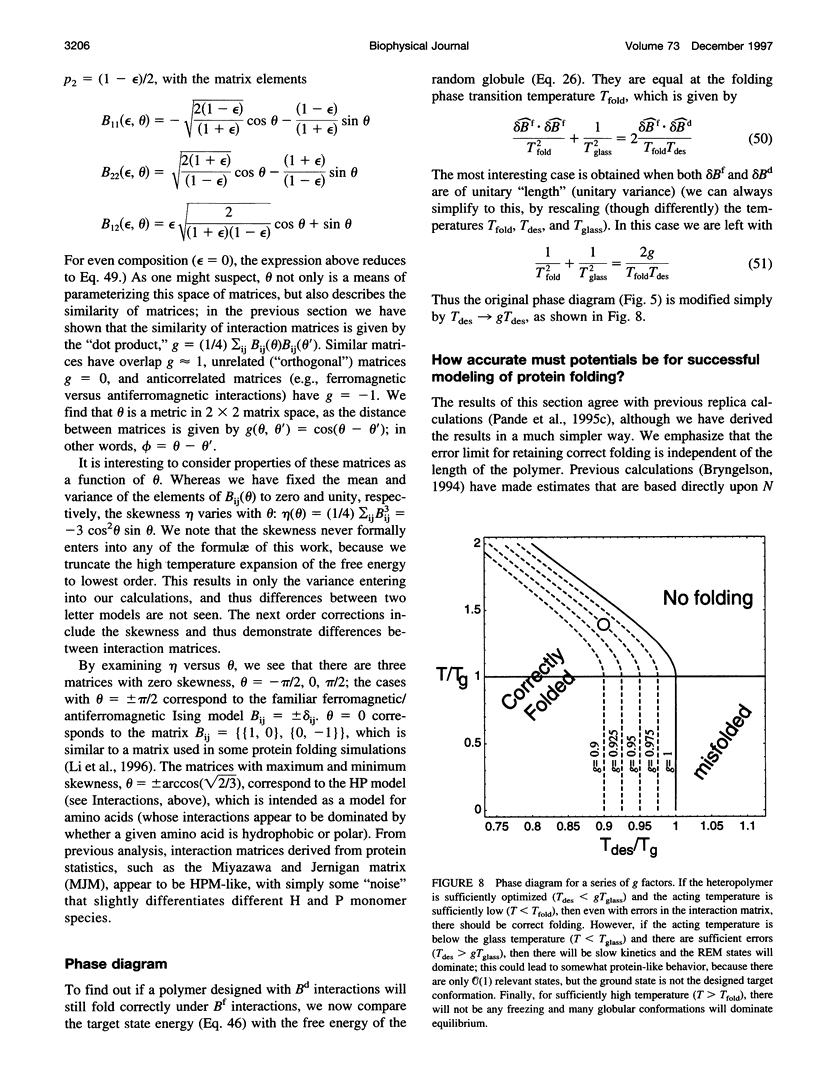
Images in this article

3197FIGURE 2
on p.3197
3198FIGURE 3
on p.3198
3202FIGURE 4
on p.3202
3203FIGURE 6
on p.3203

Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. Abkevich V. I., Gutin A. M., Shakhnovich E. I. Specific nucleus as the transition state for protein folding: evidence from the lattice model. Biochemistry. 1994 Aug 23;33(33):10026–10036. doi: 10.1021/bi00199a029. [DOI] [PubMed] [Google Scholar]
  2. Bryngelson J. D., Wolynes P. G. Spin glasses and the statistical mechanics of protein folding. Proc Natl Acad Sci U S A. 1987 Nov;84(21):7524–7528. doi: 10.1073/pnas.84.21.7524. [DOI] [PMC free article] [PubMed] [Google Scholar]
  3. Hecht M. H., Richardson J. S., Richardson D. C., Ogden R. C. De novo design, expression, and characterization of Felix: a four-helix bundle protein of native-like sequence. Science. 1990 Aug 24;249(4971):884–891. doi: 10.1126/science.2392678. [DOI] [PubMed] [Google Scholar]
  4. Klimov DK, Thirumalai D. Criterion that determines the foldability of proteins. Phys Rev Lett. 1996 May 20;76(21):4070–4073. doi: 10.1103/PhysRevLett.76.4070. [DOI] [PubMed] [Google Scholar]
  5. Li H., Helling R., Tang C., Wingreen N. Emergence of preferred structures in a simple model of protein folding. Science. 1996 Aug 2;273(5275):666–669. doi: 10.1126/science.273.5275.666. [DOI] [PubMed] [Google Scholar]
  6. Onuchic J. N., Wolynes P. G., Luthey-Schulten Z., Socci N. D. Toward an outline of the topography of a realistic protein-folding funnel. Proc Natl Acad Sci U S A. 1995 Apr 11;92(8):3626–3630. doi: 10.1073/pnas.92.8.3626. [DOI] [PMC free article] [PubMed] [Google Scholar]
  7. Panchenko A. R., Luthey-Schulten Z., Wolynes P. G. Foldons, protein structural modules, and exons. Proc Natl Acad Sci U S A. 1996 Mar 5;93(5):2008–2013. doi: 10.1073/pnas.93.5.2008. [DOI] [PMC free article] [PubMed] [Google Scholar]
  8. Pande V. S., Grosberg AYu, Tanaka T. On the theory of folding kinetics for short proteins. Fold Des. 1997;2(2):109–114. doi: 10.1016/s1359-0278(97)00015-1. [DOI] [PubMed] [Google Scholar]
  9. Pande V. S., Grosberg A. Y., Tanaka T. Nonrandomness in protein sequences: evidence for a physically driven stage of evolution? Proc Natl Acad Sci U S A. 1994 Dec 20;91(26):12972–12975. doi: 10.1073/pnas.91.26.12972. [DOI] [PMC free article] [PubMed] [Google Scholar]
  10. Pande V. S., Grosberg A. Y., Tanaka T. Thermodynamic procedure to synthesize heteropolymers that can renature to recognize a given target molecule. Proc Natl Acad Sci U S A. 1994 Dec 20;91(26):12976–12979. doi: 10.1073/pnas.91.26.12976. [DOI] [PMC free article] [PubMed] [Google Scholar]
  11. Pande VS, Grosberg AY, Joerg C, Kardar M, Tanaka T. Freezing Transition of Compact Polyampholytes. Phys Rev Lett. 1996 Oct 21;77(17):3565–3568. doi: 10.1103/PhysRevLett.77.3565. [DOI] [PubMed] [Google Scholar]
  12. Pande VS, Grosberg AY, Joerg C, Tanaka T. Is heteropolymer freezing well described by the random energy model? Phys Rev Lett. 1996 May 20;76(21):3987–3990. doi: 10.1103/PhysRevLett.76.3987. [DOI] [PubMed] [Google Scholar]
  13. Pande VS, Grosberg AY, Tanaka T. Freezing transition of random heteropolymers consisting of an arbitrary set of monomers. Phys Rev E Stat Phys Plasmas Fluids Relat Interdiscip Topics. 1995 Apr;51(4):3381–3392. doi: 10.1103/physreve.51.3381. [DOI] [PubMed] [Google Scholar]
  14. Plotkin SS, Wang J, Wolynes PG. Correlated energy landscape model for finite, random heteropolymers. Phys Rev E Stat Phys Plasmas Fluids Relat Interdiscip Topics. 1996 Jun;53(6):6271–6296. doi: 10.1103/physreve.53.6271. [DOI] [PubMed] [Google Scholar]
  15. Ptitsyn O. B., Volkenstein M. V. Protein structure and neutral theory of evolution. J Biomol Struct Dyn. 1986 Aug;4(1):137–156. doi: 10.1080/07391102.1986.10507651. [DOI] [PubMed] [Google Scholar]
  16. Ramanathan S, Shakhnovich E. Statistical mechanics of proteins with "evolutionary selected" sequences. Phys Rev E Stat Phys Plasmas Fluids Relat Interdiscip Topics. 1994 Aug;50(2):1303–1312. doi: 10.1103/physreve.50.1303. [DOI] [PubMed] [Google Scholar]
  17. Sfatos CD, Gutin AM, Shakhnovich EI. Phase diagram of random copolymers. Phys Rev E Stat Phys Plasmas Fluids Relat Interdiscip Topics. 1993 Jul;48(1):465–475. doi: 10.1103/physreve.48.465. [DOI] [PubMed] [Google Scholar]
  18. Shakhnovich E. I., Gutin A. M. Engineering of stable and fast-folding sequences of model proteins. Proc Natl Acad Sci U S A. 1993 Aug 1;90(15):7195–7199. doi: 10.1073/pnas.90.15.7195. [DOI] [PMC free article] [PubMed] [Google Scholar]
  19. Shakhnovich E. I., Gutin A. M. Formation of unique structure in polypeptide chains. Theoretical investigation with the aid of a replica approach. Biophys Chem. 1989 Nov;34(3):187–199. doi: 10.1016/0301-4622(89)80058-4. [DOI] [PubMed] [Google Scholar]
  20. Yue K., Fiebig K. M., Thomas P. D., Chan H. S., Shakhnovich E. I., Dill K. A. A test of lattice protein folding algorithms. Proc Natl Acad Sci U S A. 1995 Jan 3;92(1):325–329. doi: 10.1073/pnas.92.1.325. [DOI] [PMC free article] [PubMed] [Google Scholar]

Articles from Biophysical Journal are provided here courtesy of The Biophysical Society

RESOURCES