A proposal for robust temperature compensation of circadian rhythms

Abstract

The internal circadian rhythms of cells and organisms coordinate their physiological properties to the prevailing 24-h cycle of light and dark on earth. The mechanisms generating circadian rhythms have four defining characteristics: they oscillate endogenously with period close to 24 h, entrain to external signals, suffer phase shifts by aberrant pulses of light or temperature, and compensate for changes in temperature over a range of 10°C or more. Most theoretical descriptions of circadian rhythms propose that the underlying mechanism generates a stable limit cycle oscillation (in constant darkness or dim light), because limit cycles quite naturally possess the first three defining properties of circadian rhythms. On the other hand, the period of a limit cycle oscillator is typically very sensitive to kinetic rate constants, which increase markedly with temperature. Temperature compensation is therefore not a general property of limit cycle oscillations but must be imposed by some delicate balance of temperature dependent effects. However, “delicate balances” are unlikely to be robust to mutations. On the other hand, if circadian rhythms arise from a mechanism that concentrates sensitivity into a few rate constants, then the “balancing act” is likely to be more robust and evolvable. We propose a switch-like mechanism for circadian rhythms that concentrates period sensitivity in just two parameters, by forcing the system to alternate between a stable steady state and a stable limit cycle.

Since the breakthrough discovery of the period (per) gene by Konopka and Benzer in 1971 (1), molecular biologists have identified many new circadian rhythm genes and have uncovered a complex network of interacting feedback loops which comprise the control system. In the consensus view, an endogenous daily rhythm is created by a negative feedback loop whereby the PERIOD (PER) protein inhibits its own expression by interfering with transcription factors (2, 3). This mechanism has been studied in great detail theoretically by many authors (4–12). The time-delayed negative feedback loop generates limit cycle oscillations with many properties characteristic of physiological daily rhythms, except for one: the autonomous circadian period (T) is quite insensitive to variations of the kinetic constants, a property that is not characteristic of generic limit cycle oscillators. This insensitivity shows up in two ways: (i) T varies little among individual organisms even though individuals show considerable genetic and/or proteomic variability that translates into variations of kinetic parameters, and (ii) T is temperature compensated, even though kinetic constants are strongly temperature dependent. Physiologically, this robustness of the period (T ≈ 24 h despite genetic variability and environmental fluctuations) is essential to circadian physiology. If the autonomous period of the clock drifts too far from 24 h, then the circadian rhythm would not reliably entrain to the external 24 h light/dark Zeitgeber.

(i) Consider first the tight distribution of T [24 ± 1 h, coefficient of variation (CV) = 4%] across populations of fruit flies (13, 14). In general, the period of a limit cycle oscillator depends on many rate constants (k_i), and the variability of T as a result of rate constant variations is given by

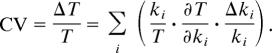Now, the relative variability of rate constants between individuals is likely to be very large (e.g., 50% in the case of heterozygosity for a loss-of-function mutation), and the variability in one parameter is surely independent of the variability in another parameter. So, the only way that CV can be small in the face of arbitrary, large Δk_i values is for T somehow to be independent of most rate constants in the mechanism.

(ii) Temperature compensation leads us to the same conclusion. Rate constants depend on temperature (θ) according to Arrhenius' law, k_i = α_ie^−Eirθ, where R is the universal gas constant, α_i determines the value of k_i at θ = 298 K, and E_i is the activation energy of the ith reaction. Ruoff and colleagues (15) pointed out that a limit cycle oscillator would be temperature compensated if, according to the chain rule,

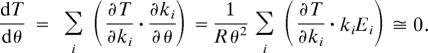This sum is a balance of positive and negative terms (because ∂T/∂k_i is positive for some i and negative for others), and it can always be set close to zero by choosing a suitable set of activation energies. The proposal of Ruoff and colleagues is a reasonable and popular explanation of temperature compensation (16–19). If Ruoff's balance hypothesis is correct, we would expect that most mutations of circadian rhythm genes (which change kinetic constants and activation energies in the underlying control system) are likely to disrupt this balance and, therefore, to exhibit failures in temperature compensation of the circadian rhythm. As expected, there are mutants with defective temperature compensation in both Neurospora crassa and Drosophila melanogaster (Table 1). However, more intriguingly, geneticists (13, 14, 20–23) have identified many circadian rhythm mutations (aberrant period) that leave temperature compensation intact; indeed, 60–70% of the representative mutants in Table 1 maintain temperature compensation. In order for temperature compensation to survive in the face of a variety of mutations at many different places in the mechanism, many terms in the balance equation must be negligible, i.e., ∂T/∂k_i · k_iE_i ≅ 0 for many i. It is unlikely that E_i ≅ 0 for many kinetic constants, leading us to the conclusion that ∂T/∂k_i ≅ 0 for many k_i values. Hence, the mechanism of circadian rhythms must somehow generate a 24-h period independently of the precise values of many of the rate constants in the mechanism. Based on the fact that the majority of circadian rhythm mutants maintain temperature compensation, we suggest that temperature compensation is not the result of a delicate balance of opposing influences of all of the rate constants in the mechanism, but rather that temperature compensation is embedded directly in the molecular machinery, analogous to the way perfect adaptation appears to be embedded in the mechanism of bacterial chemotaxis (24).

Table 1.

Representative list of clock mutants in N. crassa (20, 23) and D. melanogaster (13, 14, 21, 22)

Gene	Allele name	Period* at 25°C, h	Temperature compensation†
N. crassa
frequency	frq (wild type)	21.5	Yes
	frq1	16.5	Yes
	frq2	19.3	Yes
	frq3	24.0	No
	frq7	29.0	No
period-1	prd-1	25.8	No
period-2	prd-2	25.5	Yes
period-3	prd-3	25.1	No
period-4	prd-4	18.0	No
period-6	prd-6	18.0 at 22°C	Yes
chrono	chr	23.5	Yes
white collar-2	wc-2 ER24	29.7	No
arginine-13	arg-13	19.0	Yes
chain elongation	cel	Variable	No
choline-1	chol-1	Variable	No
cytochrome a-5	cya-5	19.0	Yes
cytochrome b-2	cyb-2	18.0	Yes
cytochrome b-3	cyb-3	20.0	Yes
cytochrome-4	cyt-4	20.0	Yes
cysteine-4	cys-4	19.0	Yes
cysteine-9	cys-9	Variable	No
cysteine-12	cys-12	19.0	Yes
maternally inherited	mi-2, mi-3, mi-5	18–19	Yes
oligomycin resistant	oliR	18–19	Yes
phenylalanine-1	phe-1	19.0	Yes
Double mutants in
N. crassa
	prd-2; prd-3	32.7	No
	prd-3; prd-6	18.6	Yes
D. melanogaster
period	per (wild type)	23.4	0.99
	perS	18.9–19.7	1.09
	perL	28.3–29.1	0.88
	perSLIH	27.2–28.1	1.05–1.08
double time	dbtL	26.8	1.08
	dbtS	18.7	1.04
timeless	timS2	21.9	0.96
	timL2	26.5	1.00
	timL1	28.1	1.02
	timUL	32.9	0.98
	timrit	25.5 at 24°C	0.91 (15–24°C)
			0.62 (24–30°C)
Double mutants in
D. melanogaster
	perL; dbtS	20.9	0.89
	perT; perSLIH	23.5 at 18°C	1.06
	perCLK; perSLIH	24.8 at 18°C	0.99
	per+; perSLIH	26.0 at 18°C	1.04
	per01; perSLIH	28.7 at 18°C	1.03
	per04; perSLIH	28.6 at 18°C	1.04
	perL; perSLIH	27.3 at 18°C	0.98
	timrit; perL	32.9 at 24°C	0.81 (15–24°C)
			0.46 (24–30°C)
	timrit; perS	22 at 24°C	1.05 (15–24°C)
			0.67 (24–30°C)
	timSL; perL	25.5	1.0

Most of N. crassa mutant list is adapted from Bell-Pedersen (20).

*Period at 25°C, unless otherwise indicated. A range of period is given when there are variations of period from different sources.

^†Values of Q₁₀ are given when they are available from literature or if there are data points that allow us to calculate Q₁₀ = (T₂/T₁)^{10/(θ1−θ2)} (43). A range of Q₁₀ is given when there are different values of Q₁₀ from different sources. Matsumoto et al. (22) use two different values of Q₁₀, below and above 24°C. If a value of Q₁₀ is not available, simple “Yes” or “No” is used to represent either presence or absence of temperature compensation in N. crassa where Bell-Pedersen (20) used “+” or “−” to represent the data. “Yes” indicates that the period changes are <20% over a 10°C range in temperature.

The cell division cycle (25) has a similar kind of compensation embedded in its control mechanism: it is a periodic process governed by a complex regulatory network, but the period of the cell cycle is completely independent of the rate constants in the underlying network. For unicellular organisms, like yeast, the period of the cell cycle is always equal to the mass doubling time of the culture, T_d = ln(2)/μ_g, where μ_g = specific growth rate, which is a function of the nutritional value of the growth medium, not the rate constants for the kinase and phosphatase reactions that dominate the control network. This physiological property of “balanced growth and division,” which is essential to the reproduction of unicellular organisms, is a consequence of the control mechanism, which triggers cell division (2-fold reduction of cell mass) every time growth increases cell mass by 2-fold (26). A similar “resetting mechanism” for the circadian rhythm might be responsible for minimizing the number of rate constants that strongly influence its period.

If ∂T/∂k_i ≅ 0 for most k_i valuess, then the robustness of circadian period is readily understood. (i) CV = ΔT/T is small because most terms in the sum,

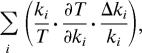are zero; and (ii) ∂T/∂θ ≅ 0 because most terms in the sum,

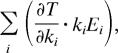are also zero. Only mutations in specific genes i, for which ∂T/∂k_i ≠ 0, will have strong effects on circadian period and temperature compensation. Identifying these mutations may lead us to that part of the mechanism responsible for robustness of circadian period.

“Resetting” Mechanism for Circadian Rhythms

Supporting Information.

For further details, see supporting information (SI) Text, Tables 2 and 3, and Figs. 5–7.

A Simple Model.

To illustrate the resetting hypothesis, we use an overly simplified model (Appendix) of the circadian rhythm control system (10), to focus on fundamental ideas rather than to be distracted by mechanistic details. The fundamental idea behind the resetting hypothesis is based on a generic bifurcation diagram and is in no way dependent on special features of the illustrative model. In SI, we present a more complex and realistic circadian rhythm model that has the same sort of bifurcation diagram and, hence, the same general properties as the simple model. Better models than these, as long as they contain the right sort of interplay among positive and negative feedback loops, will have the same potential for generating robust oscillations by resetting.

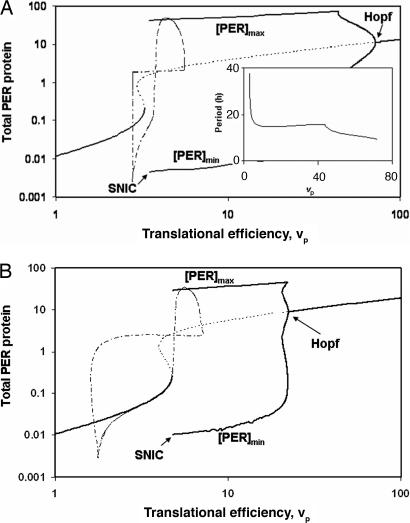
Our simple model (Appendix) supplements the basic negative feedback loop (PER protein inhibits its own production by interfering with factors that promote per gene transcription) with a positive feedback loop (PER protein inhibits its own degradation by forming homodimers that are less susceptible to proteolysis). The interplay between these feedback loops creates the potential for the control system to switch between a stable steady state of low PER abundance and a limit-cycle oscillation during which PER protein reaches very high abundance. To see this switching potential, we plot in Fig. 1A a one-parameter bifurcation diagram for the differential equations (Appendix) describing per mRNA and protein dynamics. As a function of translational efficiency, v_p, we plot [PER]_ss, the steady state concentration of total PER protein (the S-shaped curve), and [PER]_max and [PER]_min during limit cycle oscillations. Limit cycles are found for v_p values between 3.28 and 72. At v_p = 72, limit cycles arise by a Hopf bifurcation (small amplitude, finite frequency); at v_p = 3.28, they arise by a saddle-node on an invariant circle (SNIC) bifurcation (small frequency, finite amplitude). For a small range of translational efficiencies, 2.98 < v_p < 3.28, the control system has three steady-state solutions (one stable and two unstable).

Fig. 1.

One-parameter bifurcation diagrams for the differential equations (B1) and (B2), described in Appendix. (A) Parameter values: v_m = 2, k_m = 0.2, k_p1 = 53.36, k_p2 = 0.06, k_p3 = 0.2, K_eq = 1, P_crit = 0.6, J_p = 0.05. (B) All rate constants increased 2-fold. For each value of the bifurcation parameter, v_p, we plot the value of [PER] on recurrent solutions of the differential equations (steady states and limit cycle oscillations). Solid curve, stable steady state; dashed curve, unstable steady state. Curves labeled [PER]_max and [PER]_min indicate the range of an oscillatory solution at fixed value of v_p. At the Hopf bifurcation, the steady state changes stability and small amplitude, stable limit cycle oscillations arise. At the SNIC bifurcation, two steady states (a stable node and an unstable saddle) annihilate each other and are replaced by a large amplitude limit cycle. (A Inset) The period of oscillation at the SNIC bifurcation is infinite, but drops quickly to a value of ≈15 h. Superimposed on the bifurcation diagrams are the trajectories (dashed/dotted line) generated by the resetting hypothesis (see text). Although the locations of the bifurcation points depend strongly on parameter values, as do the shapes of the resetting trajectories (dashed/dotted line), the period of the two trajectories is precisely 24 h.

The Resetting Hypothesis.

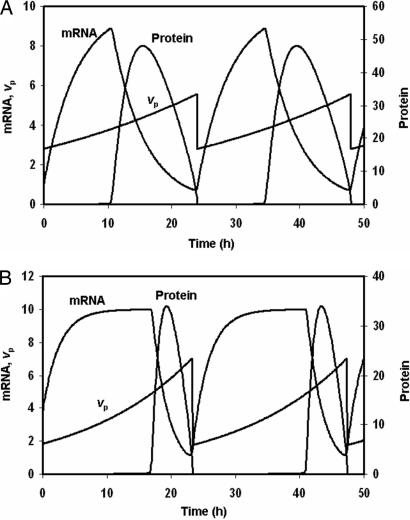
At this point, the usual approach would be to choose v_p in the oscillatory region, say v_p = 30, and model circadian rhythms as a limit cycle oscillation. The resetting hypothesis is more subtle: it posits (in this case) that per mRNA translation rate is not constant but a regulated variable of the mechanism (more on this assumption later). That is, v_p is reinterpreted as a time-dependent variable rather than a rate constant. Suppose that v_p(t) starts at a value <3.28 and increases exponentially, i.e., dv_p/dt = μ·v_p, for some constant value of μ. As long as v_p < 3.28, the control system is attracted to the stable steady state with low [PER]. However, when v_p passes through the SNIC bifurcation point, the stable steady state is lost and the control system begins an oscillation in [PER]. We assume that, when [PER] drops below a threshold level, v_p is reset by a factor σ < 1, which brings v_p back below 3.28 (see the dash-dot curve in Fig. 1A). In Fig. 2A, we display endogenous oscillations of the resetting mechanism, plotting per mRNA, protein and v_p as functions of time.

Fig. 2.

Time courses of per mRNA and protein, and the resetting parameter, v_p, for the mechanism described in the text. (A) Parameter values as in Fig. 1A, plus P_thresh = 2, μ = 0.0288, σ = 0.5. (B) Parameter values as in Fig. 1B, plus P_thresh = 2, μ = 0.0576, σ = 0.25.

In the resetting model, the period of the oscillation is given exactly by T = μ⁻¹·lnσ⁻¹. Temperature compensation requires only that we balance the effects of μ and σ,

the other rate constants in the mechanism may change considerably as a consequence of mutation without disturbing this balance. For instance, the period of oscillation (T = 24.07 h) is unchanged by a 2-fold increase or decrease of any rate constant in the mechanism, except μ and σ (naturally) and k_m. (If k_m is decreased below 0.17, then [PER] never drops below the threshold value, so v_p is never reset; v_p increases to some large value and the control system settles onto a stable steady state.) To illustrate this property of the resetting model, we plot the bifurcation diagram and time courses of the system (in Figs. 1B and 2B) when all of the rate constants have been increased 2-fold (rough simulation of a 10°C rise in temperature). The parameter σ is decreased from 0.5 to 0.25 to compensate for the rise in μ. Notice that the bifurcation points of the system move considerably, and the time courses are much changed [e.g., v_p(t) now increases and decreases 4-fold during an oscillation], but the period of the clock is still 24 h.

Is it reasonable to assume that PER translation rate might fluctuate over 24 h, as envisioned by the resetting hypothesis? The efficiency with which PER protein is produced from mRNA may well be regulated by specific per mRNA-binding proteins (27) or microRNAs (28). Circadian oscillations of a translation-activating protein or a translation-silencing microRNA might carry v_p back and forth across the bifurcation point, as envisioned in the resetting model in Figs. 1 and 2. However, there is no experimental evidence at present for such translational regulation of the circadian rhythm.

Robust Behavior of the Resetting Mechanism in the Face of Genetic Variability.

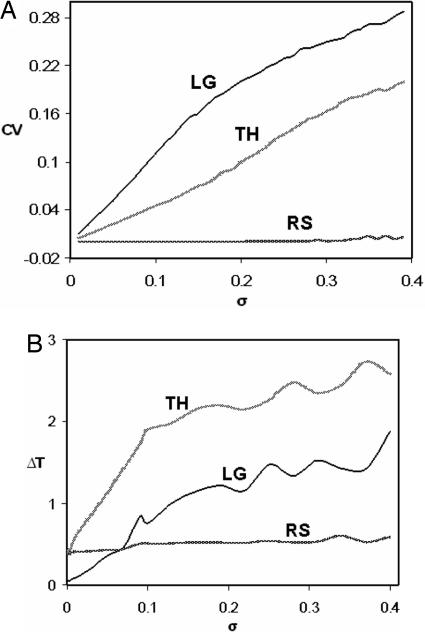
To claim that the resetting hypothesis gives a better account of properties i and ii than do limit cycle models, we must compare the behavior of the resetting model to some reasonable limit-cycle models of circadian rhythms. We choose the Leloup–Goldbeter (LG) (18) and Tyson–Hong (TH) (10) models in the limit-cycle regime. For a fair comparison, we must give each limit cycle model the advantage of an arbitrary “compensation relation” between any two of its most period-determining rate constants, analogous to the relation σ = e^−24μ required of the resetting (RS) model. As explained in SI, we take these compensation relations to be v_m = 0.43·(k_s − 0.3)^−0.16 for the LG model and J_p = 3.2 × 10⁻⁵·km−3.2 for the TH model. For each of the three models (RS, LG, and TH), we perform two tests: (test A) variability of circadian period with respect to simultaneous random perturbations of all of the kinetic constants in the model (i.e., variability across individuals), and (test B) ability to maintain temperature compensation in the face of single mutations (which cause random changes in α_i and E_i for some i).

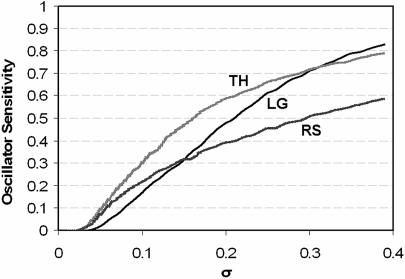
For each test, we generate a large sample of randomly perturbed individuals. In test A, an individual is generated by multiplying each basal parameter value (although not violating the compensation relation) by a new random number drawn from N(1, σ_p), the normal distribution with mean 1.0 and standard deviation σ_p. In test B, a “mutant” organism is created by randomly selecting a rate constant k_i = α_ie^−Ei/Rθ and altering both α_i and E_i by random multiplicative factors drawn from N(1, σ_p). Then the mutant organism's period is computed for θ = 293, 294,…, 303 K and its ability to temperature compensate is measured as ΔT = T_max − T_min. Both tests are run for many values of σ_p between 0.01 and 0.4, and the results (Fig. 3) plot the coefficient of variation of the period, CV = SD of period/mean of period (for test A) or the average value of ΔT (for test B) versus σ_p. For test A (Fig. 3A), for CV to be ≈5% (as observed), we must constrain the rate constant perturbations to be <5% for LG and <12% for TH, but there are no such constraints for RS. For test B (Fig. 3B), we see that both LG and TH quickly lose the ability to temperature compensate as mutations alter catalytic properties of circadian rhythm components, but RS is robustly temperature compensated. These results are a direct consequence of the fact that the limit cycle models spread out control of the period to a large number of parameters. We can also note that many perturbations for tests A and B cause a loss of oscillation (or represent a transition into more complex rhythmic behavior), giving us a separate measure of robustness (see Fig. 4).^¶

Fig. 3.

Robustness of compensated oscillator period as a function of perturbation strength (σ_p) for the models LG, TH and RS. (A) The CV of the period is plotted vs. σ_p, indicating each model's response to test A. RS is virtually unaffected (extremely robust), whereas LG and TH fail to compensate the period to different degrees. (B) ΔT is averaged over all possible single reaction mutants (Test B) for a given perturbation strength, σ_p. We see that RS is robustly temperature compensated for such mutations (very low ΔT), whereas both LG and TH fail to compensate over the given temperature range.

Fig. 4.

Sensitivity of oscillation (% of samples that lose oscillation) as a function of perturbation strength (σ_p) in test A. At σ_p = 0.2, LG loses oscillation ≈47% of the time, TH ≈59% of the time, and RS ≈40% of the time. Oscillations are most robust to small perturbations for LG, whereas RS is considerably more robust than either LG or TH for large perturbations.

General Requirements for Resetting.

The resetting mechanism does not depend on the specific assumptions we introduced to compute Fig. 1 or to make v_p (the translational efficiency of per mRNA) increase and decrease. It relies instead on having a regulatory network of sufficient richness to generate a bifurcation that carries the system from a stable steady state to a large amplitude oscillation, and on having a resettable parameter that can carry the control system back and forth across the bifurcation. Both SNIC bifurcations and subcritical Hopf bifurcations (26, 29) are suitable for this purpose, and they are both commonly observed in regulatory networks with positive and negative feedback.

For resetting to be consistent with a 24-h clock, the period of oscillation close to the bifurcation point must be <24 h, because the control system needs to spend some part of the 24-h cycle on the branch of stable steady states and the rest of the cycle traversing (part of) the limit cycle. This would seem to be a problem for a SNIC bifurcation because the period of the limit cycle oscillation diverges to infinity as the bifurcation parameter approaches the bifurcation point. However, it is often the case that the period of oscillation decreases rapidly as the bifurcation parameter moves away from a SNIC bifurcation, and so it is possible to satisfy the timing requirement. In our case, for v_p increasing beyond 3.28, the period drops precipitously to a value of about 15 h (Fig. 1A Inset). Hence, the amount of time necessary for [PER] to increase to its maximum value and then drop again below the threshold, when v_p increases above 3.28, is ≈12 h. The control system spends about half the day in the stable steady state region and the other half in the oscillatory region (Fig. 2A). If the minimum period of oscillations in this region is larger than ≈20 h, then the resetting mechanism will not maintain simple periodic repetitions of 24 h.

These bifurcations are generic (their existence does not depend on delicate mechanistic assumptions), and many different parameters in the mechanism are candidates for the resetting role. Note that the resetting hypothesis depends on the exponential change of some control parameter, v, followed by proportional resetting of v (multiplication by a factor σ). In the cell cycle context, these requirements are quite natural, because cell size increases nearly exponentially and is decreased by a factor of 0.5 at cell division. In the context of circadian rhythms, proportional resetting of v is unlikely to be an abrupt, stepwise change, but rather a rapid, continuous adjustment, governed by some terms in a differential equation for dv/dt. When the resetting step is smoothed out in this fashion, the oscillation can now be thought of as a limit cycle for a system of n + 1 differential equations

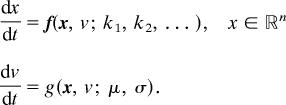Nonetheless, the period of this limit cycle will be determined largely by the dynamics of v, i.e., by parameters μ and σ, and only very weakly by the rate constants k₁, k₂,…, etc., governing the dynamics of x. Circadian period will be robustly regulated ≈24 h if μ and σ satisfy a compensation relation like σ = e^−24μ.

It has been suggested (30) that an increase in the complexity of the loop structure of a model (i.e., the addition of more positive and negative feedback loops) leads to an increased ability to meet several simultaneous evolutionary constraints, such as temperature compensation and robustness to parameter perturbations. The resetting paradigm described here achieves some of the same goals with a simple mechanism comprising one positive and one negative feedback loop.

A More Realistic Model.

In SI, we consider a model of PER dynamics in fruit flies, including interactions with TIM, dCLK, and CYC proteins, nuclear transport, and additional feedback loops. This model also displays a SNIC bifurcation (SI Fig. 6), with the bifurcation parameter equal to the rate constant for nuclear transport of PER/TIM complexes. In this context, resetting could operate if the nuclear transport rate decreases exponentially during the cycle, and is then reactivated when [PER] drops below some threshold. We can imagine the following scenario: nuclear entry of PER is progressively slowed down by posttranslational modification (e.g., phosphorylation) and/or by forming complexes with TIM, until a certain phase of the cycle when PER's structure or phosphorylation state changes to a form that enters the nucleus rapidly.

Recent evidence indicates that PER translocation between cytoplasm and nucleus is regulated during the circadian cycle. Meyer et al. (31) showed that PER and TIM rapidly form complexes and accumulate in the cytoplasm, and after a delay of ≈6 h, they abruptly dissociate and move into the nucleus. This perplexing behavior is consistent with the resetting picture in SI Fig. 6, where the rate of nuclear entry of PER decreases steadily during the circadian cycle and then increases abruptly. Furthermore, PER translocation is intimately connected to circadian period and temperature compensation. The per^L allele encodes a mutant protein PER^L with a single amino acid substitution, resulting in long-period rhythms (≈28 h) (32) that are not temperature compensated (14). In mutant cells, nuclear translocation of PER^L is delayed (31, 33). Meyer et al. (31) did not test for the timing of nuclear accumulation of PER as a function of temperature in wild-type PER vs. PER^L expressing cells. We predict that the onset of PER nuclear translocation is further delayed with increasing temperature in PER^L expressing cells (hence longer periods at higher temperatures in per^L cells; ref. 13), whereas the delay is invariant in wild-type PER-expressing cells. In light of the results of Meyer et al. and other evidence of regulated PER nuclear entry involving TIM, DBT, and various kinases (14, 34–36), we favor regulated nuclear import and/or export as a likely candidate for our resetting variable.

In contrast to delayed nuclear entry of PER^L, TIM^UL expressing cells exhibit advanced nuclear entry of PER compared with wild-type cells (37). (The tim^UL mutation, for which temperature compensation is intact, is a single amino acid substitution that causes prolonged accumulation of PER/TIM^UL in the nucleus, with an extended phase of repression of per and tim, which lengthens the period to 33 h.) It appears as though the tim^UL mutation causes a longer period not by changing the onset of nuclear accumulation of PER, but by altering the nuclear import and/or export rate followed by delayed closure of the negative feedback loop. In the context of our model, the tim^UL mutation might be causing changes in μ and/or σ to lengthen T, whereas maintaining the balance between μ and σ as a function of temperature variations.

Discussion

In summary, we found that Ruoff's equation is not robust to mutation if it requires delicate balancing of many rate constants in a limit cycle model for the circadian rhythm mechanism. We propose that temperature compensation and other indicators of the robustness of circadian period to genetic variation are more likely the results of a molecular mechanism for which only a few control parameters significantly affect the period of oscillation, and we suggest a resetting hypothesis as a candidate mechanism. Resetting works by moving an effective rate constant back and forth across a SNIC bifurcation. SNIC bifurcations are common features of regulatory networks with both positive and negative feedback loops, of which the circadian machinery is richly endowed. In general, many different rate constants in the mechanism can serve the resetting role.

Our modeling presumes that circadian rhythm properties such as robust 24-h period and temperature compensation are determined at the level of single pacesetting cells. We have not considered any role for intercellular communication in determining the period or the temperature-independence of circadian rhythms. That circadian period mutants commonly leave temperature compensation intact (Table 1) may reflect a difference in levels of organization for these properties. For example, oscillator period may be temperature-compensated at the cellular level exactly as proposed by Ruoff and colleagues, but the overt period of the rhythm in a multicellular organism may be determined, in addition, by intercellular couplings that are insensitive to temperature changes. If that were the case, then mutations affecting intracellular interactions might change the period without upsetting temperature compensation. Although this alternative explanation may apply to Drosophila, it is unlikely for Neurospora.

The oscillatory period of a cell-autonomous, limit-cycle model, based on detailed biochemical interactions among circadian genes and proteins, is a complicated function of all of the rate constants in the mechanism. Because reaction rate constants increase rapidly with temperature, the period-lengthening and period-shortening effects of the parameters must be delicately balanced to achieve temperature compensation. Consequently, temperature-independence of circadian period (in this paradigm) should be fragile with respect to mutation. By contrast, our resetting hypothesis concentrates all of the period-determining effects on just two parameters (μ and σ), which makes temperature compensation easier to achieve. Although the temporal dynamics of the underlying reactions are still strongly temperature dependent (within the resetting paradigm), the control system switches back and forth between the domain of attraction of a stable steady state and the domain of attraction of a stable limit cycle. As temperature changes, any alterations in the relative timing of events in the limit-cycle region are made up for by compensatory changes in the time spent under attraction of the stable steady state. The 24-h period is determined solely by the rules for switching between the two domains.

On the other hand, the resetting hypothesis may appear to be too robust: only mutations that alter μ and σ impinge significantly on period and temperature compensation. We are not proposing that the circadian rhythm mechanism is such a simple process that only two parameters dictate the period of the system. We suppose that, in reality, μ and σ are functions of other molecular processes (phosphorylation, ubiquitination, complex formation, etc.) and that mutations that disrupt any of these processes may interfere with temperature compensation.

The resetting hypothesis makes a number of testable predictions.

Prediction 1.

For many reasons independent of our theory, it seems reasonable to do a thorough screen for genetic mutations that disrupt temperature compensation. Are such mutations common and broadly distributed across the circadian rhythm regulatory network, as the limit cycle hypothesis would suggest, or are they rare and concentrated among a few components of the network, as the resetting hypothesis would suggest?

Prediction 2.

Resetting requires a dynamic system with both positive and negative feedback loops that operates in a region of parameter space exhibiting both multiple steady states (bistability) and limit cycle oscillations (see Fig. 1). Hysteresis in our model relies on the autocatalytic increase of PER based on its homodimerization and stabilization. One could test this assumption directly by measuring the half-lives of monomeric PER (by disrupting the PAS-binding domain) compared with PER–PER complexes. One could also test for hysteresis directly, along the same lines that proved successful in demonstrating bistability in the mitotic control of frog eggs (38, 39), in a per-null mutant with the wild-type per gene under the control of an inducible promoter (e.g., the Tet On/Off system). When PER synthesis is ramped up from low rates, there should be an abrupt increase in the PER expression level at a certain threshold synthesis rate. Once the system is in the PER-high state, it will stay there as the PER synthesis rate is ramped back down, until it falls below a lower threshold synthesis rate for turning the bistable switch off.

Prediction 3.

If bistability can be demonstrated in the circadian rhythm control system, then it is likely on theoretical grounds that if the positive feedback loop is genetically severed, then oscillations continue with shorter period and smaller amplitude. This effect has been observed experimentally in the analogous case for M-phase promoting factor in frog egg extracts (40).

Abbreviation

CV

coefficient of variation.

Appendix: A Simple Model of PER Dynamics

To illustrate the resetting hypothesis, we use a two-variable model of the dynamics of per mRNA (M) and total PER protein (P_T) described in an earlier publication (10). We assume that PER molecules exist in monomeric and dimeric states, in equilibrium,

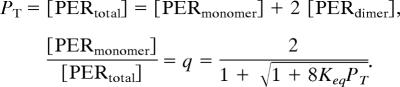The dimeric form enters the nucleus and inhibits transcription of the per gene

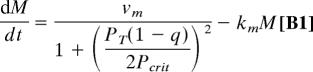In addition to “background” degradation of PER (the term −k_p3P_T), there is additional degradation associated with phosphorylation of PER by a kinase called DBT (doubletime). Based on evidence in refs. 41 and 42, we assume that DBT phosphorylates PER monomers faster than PER dimers (k_p1 > k_p2).